Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 100216 by Rio Michael last updated on 25/Jun/20

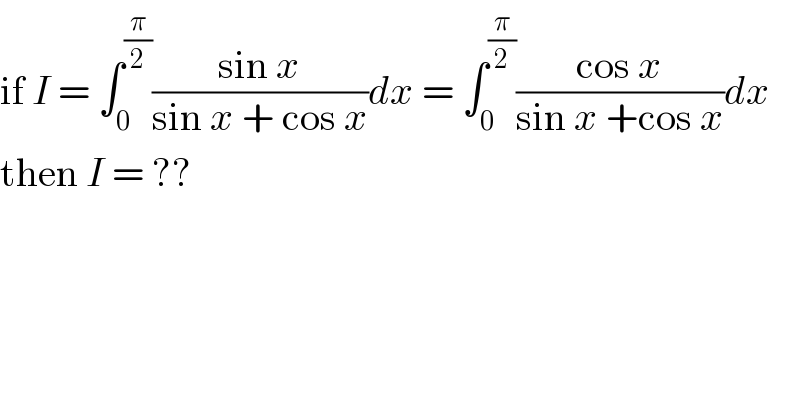
if I = ∫_0 ^(π/2) ((sin x)/(sin x + cos x))dx = ∫_0 ^(π/2) ((cos x)/(sin x +cos x))dx then I = ??

$$\mathrm{if}\:{I}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sin}\:{x}}{\mathrm{sin}\:{x}\:+\:\mathrm{cos}\:{x}}{dx}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cos}\:{x}}{\mathrm{sin}\:{x}\:+\mathrm{cos}\:{x}}{dx}\: \\ $$$$\mathrm{then}\:{I}\:=\:?? \\ $$

Commented by Dwaipayan Shikari last updated on 25/Jun/20

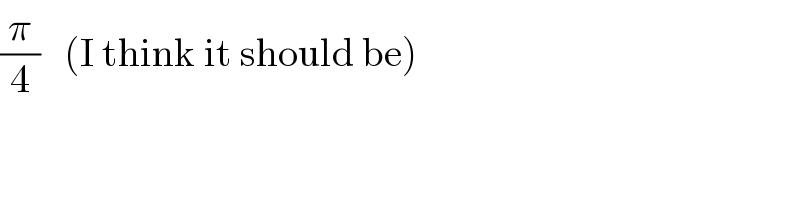
(π/4) (I think it should be)

$$\frac{\pi}{\mathrm{4}}\:\:\:\left(\mathrm{I}\:\mathrm{think}\:\mathrm{it}\:\mathrm{should}\:\mathrm{be}\right) \\ $$

Commented by Dwaipayan Shikari last updated on 25/Jun/20

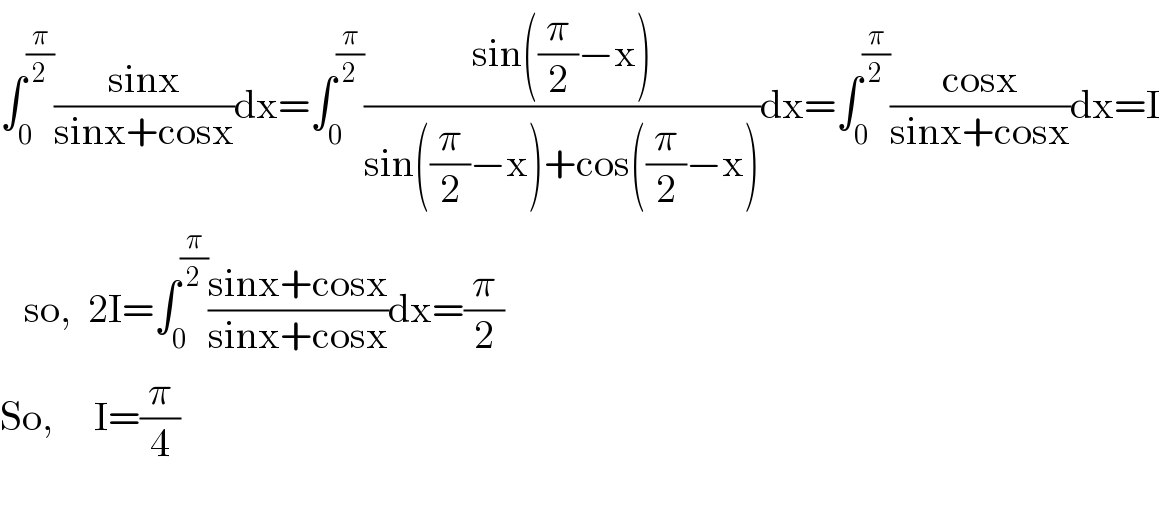
∫_0 ^(π/2) ((sinx)/(sinx+cosx))dx=∫_0 ^(π/2) ((sin((π/2)−x))/(sin((π/2)−x)+cos((π/2)−x)))dx=∫_0 ^(π/2) ((cosx)/(sinx+cosx))dx=I so, 2I=∫_0 ^(π/2) ((sinx+cosx)/(sinx+cosx))dx=(π/2) So, I=(π/4)

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sinx}}{\mathrm{sinx}+\mathrm{cosx}}\mathrm{dx}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sin}\left(\frac{\pi}{\mathrm{2}}−\mathrm{x}\right)}{\mathrm{sin}\left(\frac{\pi}{\mathrm{2}}−\mathrm{x}\right)+\mathrm{cos}\left(\frac{\pi}{\mathrm{2}}−\mathrm{x}\right)}\mathrm{dx}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cosx}}{\mathrm{sinx}+\mathrm{cosx}}\mathrm{dx}=\mathrm{I} \\ $$$$\:\:\:\mathrm{so},\:\:\mathrm{2I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sinx}+\mathrm{cosx}}{\mathrm{sinx}+\mathrm{cosx}}\mathrm{dx}=\frac{\pi}{\mathrm{2}} \\ $$$$\mathrm{So},\:\:\:\:\:\mathrm{I}=\frac{\pi}{\mathrm{4}} \\ $$$$ \\ $$

Answered by Ar Brandon last updated on 25/Jun/20

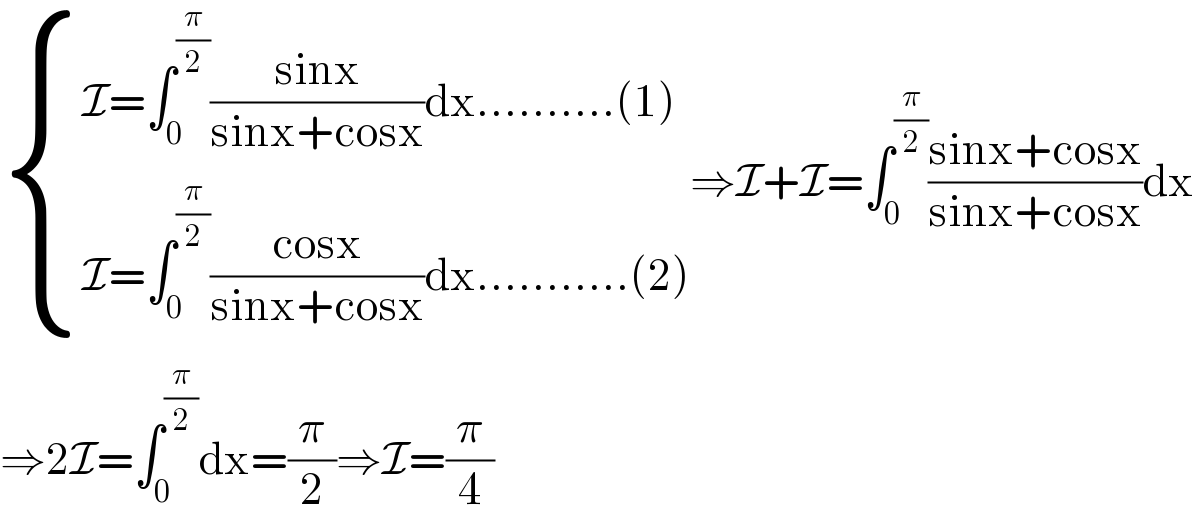
{ ((I=∫_0 ^(π/2) ((sinx)/(sinx+cosx))dx..........(1))),((I=∫_0 ^(π/2) ((cosx)/(sinx+cosx))dx...........(2))) :}⇒I+I=∫_0 ^(π/2) ((sinx+cosx)/(sinx+cosx))dx ⇒2I=∫_0 ^(π/2) dx=(π/2)⇒I=(π/4)

$$\begin{cases}{\mathcal{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sinx}}{\mathrm{sinx}+\mathrm{cosx}}\mathrm{dx}..........\left(\mathrm{1}\right)}\\{\mathcal{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cosx}}{\mathrm{sinx}+\mathrm{cosx}}\mathrm{dx}...........\left(\mathrm{2}\right)}\end{cases}\Rightarrow\mathcal{I}+\mathcal{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sinx}+\mathrm{cosx}}{\mathrm{sinx}+\mathrm{cosx}}\mathrm{dx} \\ $$$$\Rightarrow\mathrm{2}\mathcal{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{dx}=\frac{\pi}{\mathrm{2}}\Rightarrow\mathcal{I}=\frac{\pi}{\mathrm{4}} \\ $$

Commented by Coronavirus last updated on 25/Jun/20

clean

$${clean} \\ $$

Commented by Ar Brandon last updated on 25/Jun/20

Ouais

Commented by Rio Michael last updated on 26/Jun/20

thank you′ll

$$\mathrm{thank}\:\mathrm{you}'\mathrm{ll} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com