solve the differential equations 1- xcos (ln (x/y))dy−ydx=0 2- ydx+2xdy =2y((√x)/(cos^2 (y)))dy y(0)=π


Question and Answers Forum

All Questions Topic List
Differential Equation Questions
Previous in All Question Next in All Question
Previous in Differential Equation Next in Differential Equation
Question Number 100594 by Coronavirus last updated on 27/Jun/20

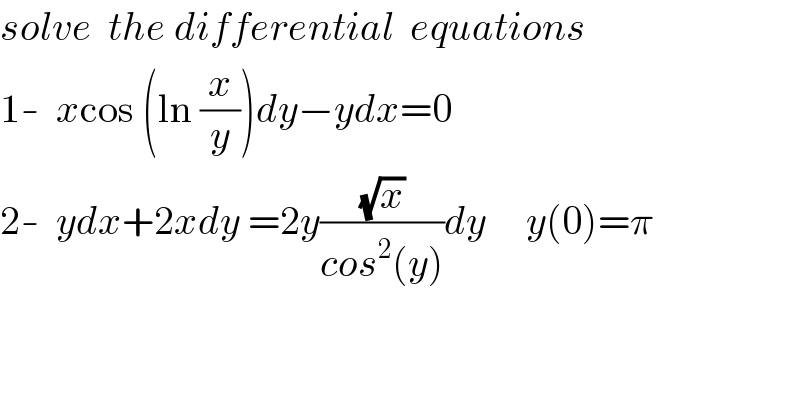
$s o l v e t h e d i f f e r e n t i a l e q u a t i o n s$ $1 - x \cos (\ln \frac{x}{y}) d y - y d x = 0$ $2 - y d x + 2 x d y = 2 y \frac{\sqrt{x}}{{c o s}^{2} (y)} d y y (0) = π$
	Answered by smridha last updated on 27/Jun/20

	$2 . [\frac{d x}{2 \sqrt{x}} + \frac{\sqrt{x}}{y} d y = {s e c}^{2} (y) d y]$ $m u l t : b y y b o t h s i d e s a n d i n t e g r a t i n g$ $\int d (y . \sqrt{x}) = \int y . {s e c}^{2} (y) d y$ $\Rightarrow y \sqrt{x} = y t a n (y) + l n [c o s (y)] + c$ $p u t t h e c o n d i t i o n y (0) = π$ $\Rightarrow 0 = 0 + l n (- 1) + c$ $s o c = \underline{+} i π$ $s o t h e s o l u t i o n$ $y \sqrt{x} = y t a n (y) + l n [c o s (y)] \underline{+} i π$
	Answered by smridha last updated on 28/Jun/20

	$(1) . \frac{d x}{d y} = \frac{x}{2 y} [{(\frac{x}{y})}^{i} + {(\frac{x}{y})}^{- i}]$ $l e t \frac{x}{y} = v s o \frac{d x}{d y} = v + y . \frac{d v}{d y}$ $n o w$ $v + y . \frac{d v}{d y} = \frac{v}{2} [\frac{v^{2 i} + 1}{v^{i}}]$ $y . \frac{d v}{d y} = v [\frac{{(v^{i} - 1)}^{2}}{2 v^{i}}]$ $\frac{d y}{y} = \frac{2 v^{i - 1}}{{(v^{i} - 1)}^{2}} d v$ $i n t e g r a t i n g b o t h s i d e s w e g e t . .$ $l n (y) = 2 i . \frac{1}{(v^{i} - 1)} + l n (c)$ $s o y = c . e^{\frac{2 i}{[{(\frac{x}{y})}^{i} - 1]}}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum