
Question Number 101828 by floor(10²Eta[1]) last updated on 05/Jul/20
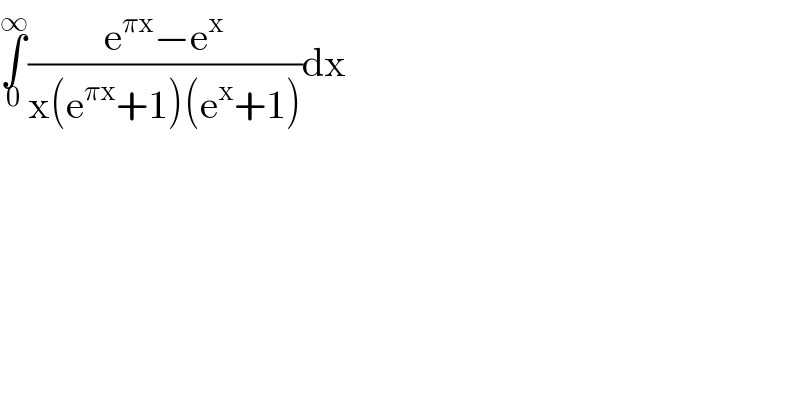
$$\underset{\mathrm{0}} {\overset{\infty} {\int}}\frac{\mathrm{e}^{\pi\mathrm{x}} −\mathrm{e}^{\mathrm{x}} }{\mathrm{x}\left(\mathrm{e}^{\pi\mathrm{x}} +\mathrm{1}\right)\left(\mathrm{e}^{\mathrm{x}} +\mathrm{1}\right)}\mathrm{dx} \\ $$
Answered by maths mind last updated on 05/Jul/20

$$=\int_{\mathrm{0}} ^{+\infty} \frac{\mathrm{1}}{{x}}\left\{\frac{\mathrm{1}}{\left({e}^{{x}} +\mathrm{1}\right)}−\frac{\mathrm{1}}{{e}^{\pi{x}} +\mathrm{1}}\right\}{dx} \\ $$$${let}\:{f}\:{bee}\:{lik}\:{t}\geqslant\mathrm{0}\:{well}\:{defind}\:{C}_{\infty} \\ $$$${f}\left({t}\right)=\int_{\mathrm{0}} ^{+\infty} \frac{\mathrm{1}}{{x}}\left\{\frac{\mathrm{1}}{{e}^{{x}} +\mathrm{1}}−\frac{\mathrm{1}}{{e}^{\pi{x}} +\mathrm{1}}\right\}{e}^{−{xt}} {dx} \\ $$$${f}'\left({t}\right)=\int_{\mathrm{0}} ^{+\infty} −\frac{{e}^{−{xt}} }{{e}^{{x}} +\mathrm{1}}{dx}+\int_{\mathrm{0}} ^{+\infty} \frac{{e}^{−{xt}} }{{e}^{\pi{x}} +\mathrm{1}}{dx} \\ $$$$=−\int_{\mathrm{0}} ^{\infty} {e}^{−{x}\left({t}+\mathrm{1}\right)} \underset{{k}\geqslant\mathrm{0}} {\sum}\left(−{e}^{−{x}} \right)^{{k}} {dx}+\int_{\mathrm{0}} ^{+\infty} {e}^{−\left({t}+\pi\right)} \Sigma\left(−{e}^{−\pi{x}} \right)^{{k}} {dx} \\ $$$$=\underset{{k}\geqslant\mathrm{0}} {\sum}\left(−\mathrm{1}\right)^{{k}+\mathrm{1}} .\frac{\mathrm{1}}{{k}+{t}+\mathrm{1}}+\underset{{k}\geqslant\mathrm{0}} {\sum}\frac{\left(−\mathrm{1}\right)^{{k}} }{\pi{k}+{t}+\pi} \\ $$$$=\underset{{k}\geqslant\mathrm{0}} {\sum}\left(\frac{\left(−\mathrm{1}\right)^{{k}} }{{k}+{t}+\pi}−\frac{\left(−\mathrm{1}\right)^{{k}} }{{k}+{t}+\mathrm{1}}\right) \\ $$$$=\underset{{k}\geqslant\mathrm{0}} {\sum}\left(\frac{\mathrm{1}}{\mathrm{2}{k}+{t}+\pi}−\frac{\mathrm{1}}{\mathrm{2}{k}+{t}+\mathrm{1}}\right)+\underset{{k}\geqslant\mathrm{0}} {\sum}\left(\frac{\mathrm{1}}{\mathrm{2}{k}+\mathrm{2}+{t}}−\frac{\mathrm{1}}{\mathrm{2}{k}+{t}+\pi+\mathrm{1}}\right) \\ $$$$\frac{\mathrm{1}}{{t}+\pi}−\frac{\mathrm{1}}{{t}+\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}+{t}}−\frac{\mathrm{1}}{{t}+\pi+\mathrm{1}}+\underset{{k}\geqslant\mathrm{1}} {\sum}\left(−\frac{\mathrm{1}}{\mathrm{2}{k}}+\frac{\mathrm{1}}{\mathrm{2}{k}+{t}+\pi}+\frac{\mathrm{1}}{\mathrm{2}{k}}−\frac{\mathrm{1}}{\mathrm{2}{k}+{t}+\mathrm{1}}\right) \\ $$$$+\underset{{k}\geqslant\mathrm{1}} {\sum}\left(\frac{\mathrm{1}}{\mathrm{2}{k}+\mathrm{2}+{t}}−\frac{\mathrm{1}}{\mathrm{2}{k}}+\frac{\mathrm{1}}{\mathrm{2}{k}}−\frac{\mathrm{1}}{\mathrm{2}{k}+{t}+\pi+\mathrm{1}}\right) \\ $$$${we}\:{have}\:{H}\left({z}\right)=\underset{{k}\geqslant\mathrm{1}} {\sum}\left(\frac{\mathrm{1}}{{k}}−\frac{\mathrm{1}}{{k}+{z}}\bar {\right)}\forall{z}\in\mathbb{C}−\left\{\mathbb{Z}_{−} \right\} \\ $$$${we}\:{get}\:{f}'\left({t}\right)=\frac{\mathrm{1}}{{t}+\pi}−\frac{\mathrm{1}}{{t}+\mathrm{1}}+\frac{\mathrm{1}}{{t}+\mathrm{2}}−\frac{\mathrm{1}}{{t}+\pi+\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{2}}{H}\left(\frac{{t}+\pi}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{2}}{H}\left(\frac{{t}+\mathrm{1}}{\mathrm{2}}\right) \\ $$$$−\frac{\mathrm{1}}{\mathrm{2}}{H}\left(\frac{{t}+\mathrm{2}}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{2}}{H}\left(\frac{{t}+\pi+\mathrm{1}}{\mathrm{2}}\right) \\ $$$${H}\left({z}\right)=\Psi\left({z}+\mathrm{1}\right)+\gamma \\ $$$${we}\:{get} \\ $$$${f}'\left({t}\right)=\frac{\mathrm{1}}{{t}+\pi}−\frac{\mathrm{1}}{{t}+\mathrm{1}}+\frac{\mathrm{1}}{{t}+\mathrm{2}}−\frac{\mathrm{1}}{{t}+\pi+\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}}\left(\Psi\left(\frac{{t}+\mathrm{3}}{\mathrm{2}}\right)−\Psi\left(\frac{{t}+\pi+\mathrm{2}}{\mathrm{2}}\right)+\Psi\left(\frac{{t}+\pi+\mathrm{1}}{\mathrm{2}}\right)−\Psi\left(\frac{{t}+\mathrm{2}}{\mathrm{2}}\right)\right) \\ $$$${f}\left({t}\right)={ln}\left(\frac{{t}+\pi}{{t}+\pi+\mathrm{1}}\right)+{ln}\left(\frac{{t}+\mathrm{2}}{{t}+\mathrm{1}}\right)+{log}\left(\frac{\Gamma\left(\frac{{t}+\mathrm{3}}{\mathrm{2}}\right)}{\Gamma\left(\frac{{t}+\mathrm{2}}{\mathrm{2}}\right)}\right)+{log}\left(\frac{\Gamma\left(\frac{{t}+\pi+\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left(\frac{{t}+\pi+\mathrm{2}}{\mathrm{2}}\right)}\right)+{c} \\ $$$${wr}\:{used}\int\Psi\left({z}\right){dz}={log}\left(\Gamma\left({z}\right)\right)+{c} \\ $$$$\underset{{t}\rightarrow\infty} {\mathrm{lim}}{f}\left({t}\right)=\mathrm{0} \\ $$$${we}\:{need}\:{too}\:{finde}\:{this}\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{\Gamma\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left({x}\right)} \\ $$$$\Gamma\left({z}+{a}\right)\sim\sqrt{\mathrm{2}\pi{z}}.{z}^{{z}+{a}−\frac{\mathrm{1}}{\mathrm{2}}} {e}^{−{z}} \\ $$$${f}\left({t}\right)\rightarrow\mathrm{0} \\ $$$${f}\left(\mathrm{0}\right)=\int_{\mathrm{0}} ^{+\infty} \frac{{e}^{\pi{x}} −{e}^{{x}} }{{x}\left({e}^{{x}} +\mathrm{1}\right)\left({e}^{\pi{x}} +\mathrm{1}\right)}{dx}={ln}\left(\frac{\pi}{\pi+\mathrm{1}}\right)+{ln}\left(\mathrm{2}\right)+{log}\left(\frac{\Gamma\left(\frac{\mathrm{3}}{\mathrm{2}}\right)\Gamma\left(\frac{\pi+\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left(\mathrm{1}\right)\Gamma\left(\frac{\pi+\mathrm{2}}{\mathrm{2}}\right)}\right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by floor(10²Eta[1]) last updated on 05/Jul/20

$$\mathrm{someone}\:\mathrm{help}\:\mathrm{me}\:\mathrm{with}\:\mathrm{the}\:\mathrm{solution}: \\ $$$$\mathrm{I}\left(\mathrm{t}\right)=\underset{\mathrm{0}} {\overset{\infty} {\int}}\frac{\mathrm{e}^{\mathrm{tx}} −\mathrm{e}^{\mathrm{x}} }{\mathrm{x}\left(\mathrm{e}^{\mathrm{tx}} +\mathrm{1}\right)\left(\mathrm{e}^{\mathrm{x}} +\mathrm{1}\right)}\mathrm{dx} \\ $$$$\mathrm{now}\:\mathrm{i}'\mathrm{m}\:\mathrm{gonna}\:\mathrm{derivate}\:\mathrm{and}\:\mathrm{integrate}\:\mathrm{again}: \\ $$$$\mathrm{I}'\left(\mathrm{t}\right)=\underset{\mathrm{0}} {\overset{\infty} {\int}}\frac{\partial}{\partial\mathrm{t}}\left(\frac{\mathrm{e}^{\mathrm{tx}} −\mathrm{e}^{\mathrm{x}} }{\mathrm{x}\left(\mathrm{e}^{\mathrm{tx}} +\mathrm{1}\right)\left(\mathrm{e}^{\mathrm{x}} +\mathrm{1}\right)}\right)\mathrm{dx} \\ $$$$=\underset{\mathrm{0}} {\overset{\infty} {\int}}\frac{\mathrm{e}^{\mathrm{tx}} }{\left(\mathrm{e}^{\mathrm{tx}} +\mathrm{1}\right)^{\mathrm{2}} }\mathrm{dx},\:\mathrm{u}=\mathrm{e}^{\mathrm{tx}} +\mathrm{1}\Rightarrow\frac{\mathrm{du}}{\mathrm{t}}=\mathrm{e}^{\mathrm{tx}} \mathrm{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{t}}\underset{\mathrm{2}} {\overset{\infty} {\int}}\frac{\mathrm{du}}{\mathrm{u}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2t}} \\ $$$$\Rightarrow\mathrm{I}'\left(\mathrm{t}\right)=\frac{\mathrm{1}}{\mathrm{2t}}\Rightarrow\mathrm{I}\left(\mathrm{t}\right)=\int\frac{\mathrm{1}}{\mathrm{2t}}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\mathrm{t}\mid+\mathrm{C} \\ $$$$\mathrm{I}\left(\mathrm{1}\right)=\mathrm{0}=\mathrm{C}\Rightarrow\mathrm{I}\left(\mathrm{t}\right)=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\mathrm{t}\mid \\ $$$$\Rightarrow\mathrm{I}\left(\pi\right)=\mathrm{ln}\left(\sqrt{\pi}\right) \\ $$
Answered by mathmax by abdo last updated on 05/Jul/20
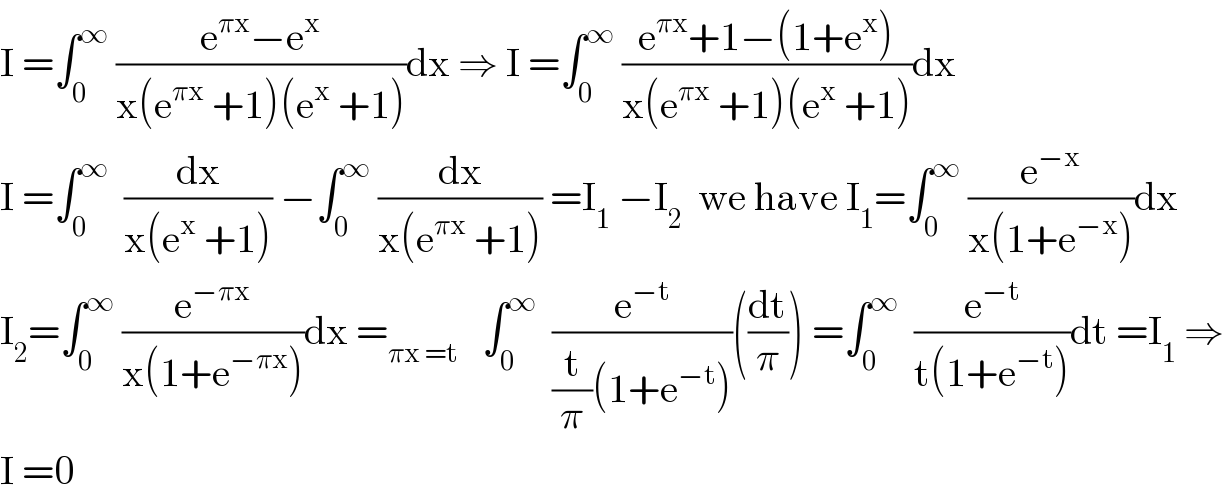
$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{\pi\mathrm{x}} −\mathrm{e}^{\mathrm{x}} }{\mathrm{x}\left(\mathrm{e}^{\pi\mathrm{x}} \:+\mathrm{1}\right)\left(\mathrm{e}^{\mathrm{x}} \:+\mathrm{1}\right)}\mathrm{dx}\:\Rightarrow\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{\pi\mathrm{x}} +\mathrm{1}−\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)}{\mathrm{x}\left(\mathrm{e}^{\pi\mathrm{x}} \:+\mathrm{1}\right)\left(\mathrm{e}^{\mathrm{x}} \:+\mathrm{1}\right)}\mathrm{dx} \\ $$$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{dx}}{\mathrm{x}\left(\mathrm{e}^{\mathrm{x}} \:+\mathrm{1}\right)}\:−\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{dx}}{\mathrm{x}\left(\mathrm{e}^{\pi\mathrm{x}} \:+\mathrm{1}\right)}\:=\mathrm{I}_{\mathrm{1}} \:−\mathrm{I}_{\mathrm{2}} \:\:\mathrm{we}\:\mathrm{have}\:\mathrm{I}_{\mathrm{1}} =\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{−\mathrm{x}} }{\mathrm{x}\left(\mathrm{1}+\mathrm{e}^{−\mathrm{x}} \right)}\mathrm{dx} \\ $$$$\mathrm{I}_{\mathrm{2}} =\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{−\pi\mathrm{x}} }{\mathrm{x}\left(\mathrm{1}+\mathrm{e}^{−\pi\mathrm{x}} \right)}\mathrm{dx}\:=_{\pi\mathrm{x}\:=\mathrm{t}} \:\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{e}^{−\mathrm{t}} }{\frac{\mathrm{t}}{\pi}\left(\mathrm{1}+\mathrm{e}^{−\mathrm{t}} \right)}\left(\frac{\mathrm{dt}}{\pi}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{e}^{−\mathrm{t}} }{\mathrm{t}\left(\mathrm{1}+\mathrm{e}^{−\mathrm{t}} \right)}\mathrm{dt}\:=\mathrm{I}_{\mathrm{1}} \:\Rightarrow \\ $$$$\mathrm{I}\:=\mathrm{0} \\ $$
Commented by maths mind last updated on 05/Jul/20

$$\:{I}_{\mathrm{1},} {I}_{\mathrm{2}} \:{didnt}\:\:{cv}\: \\ $$
Commented by mathmax by abdo last updated on 05/Jul/20
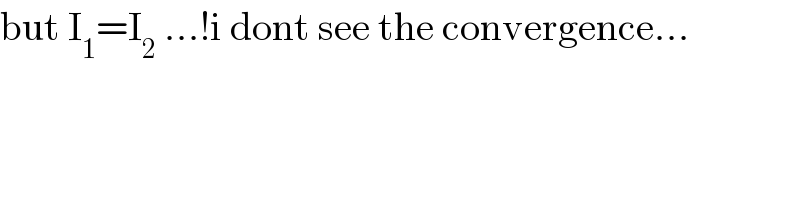
$$\mathrm{but}\:\mathrm{I}_{\mathrm{1}} =\mathrm{I}_{\mathrm{2}} \:...!\mathrm{i}\:\mathrm{dont}\:\mathrm{see}\:\mathrm{the}\:\mathrm{convergence}... \\ $$
