
Question Number 102183 by bemath last updated on 07/Jul/20
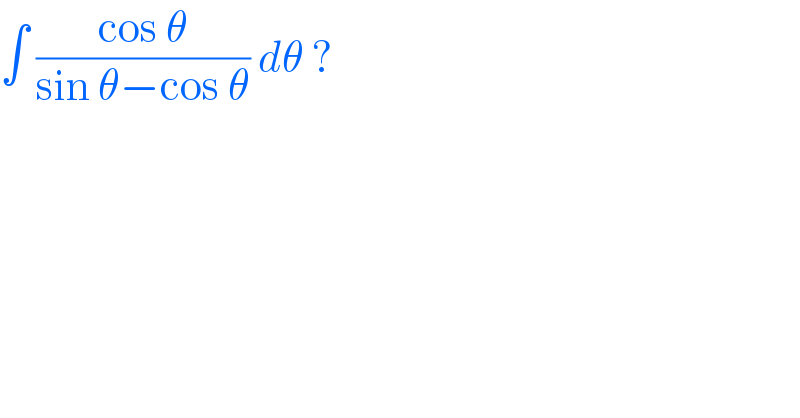
$$\int\:\frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta−\mathrm{cos}\:\theta}\:{d}\theta\:? \\ $$
Answered by Dwaipayan Shikari last updated on 07/Jul/20
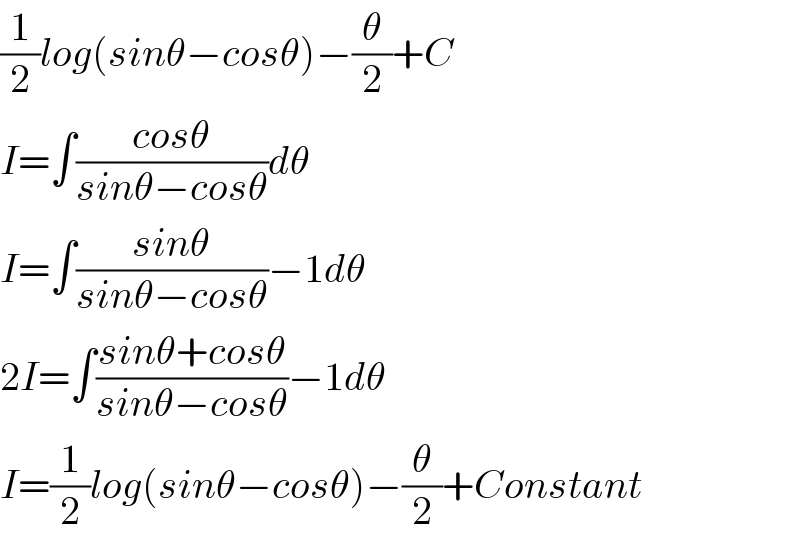
$$\frac{\mathrm{1}}{\mathrm{2}}{log}\left({sin}\theta−{cos}\theta\right)−\frac{\theta}{\mathrm{2}}+{C} \\ $$$${I}=\int\frac{{cos}\theta}{{sin}\theta−{cos}\theta}{d}\theta \\ $$$${I}=\int\frac{{sin}\theta}{{sin}\theta−{cos}\theta}−\mathrm{1}{d}\theta \\ $$$$\mathrm{2}{I}=\int\frac{{sin}\theta+{cos}\theta}{{sin}\theta−{cos}\theta}−\mathrm{1}{d}\theta \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}{log}\left({sin}\theta−{cos}\theta\right)−\frac{\theta}{\mathrm{2}}+{Constant} \\ $$
Answered by bobhans last updated on 07/Jul/20
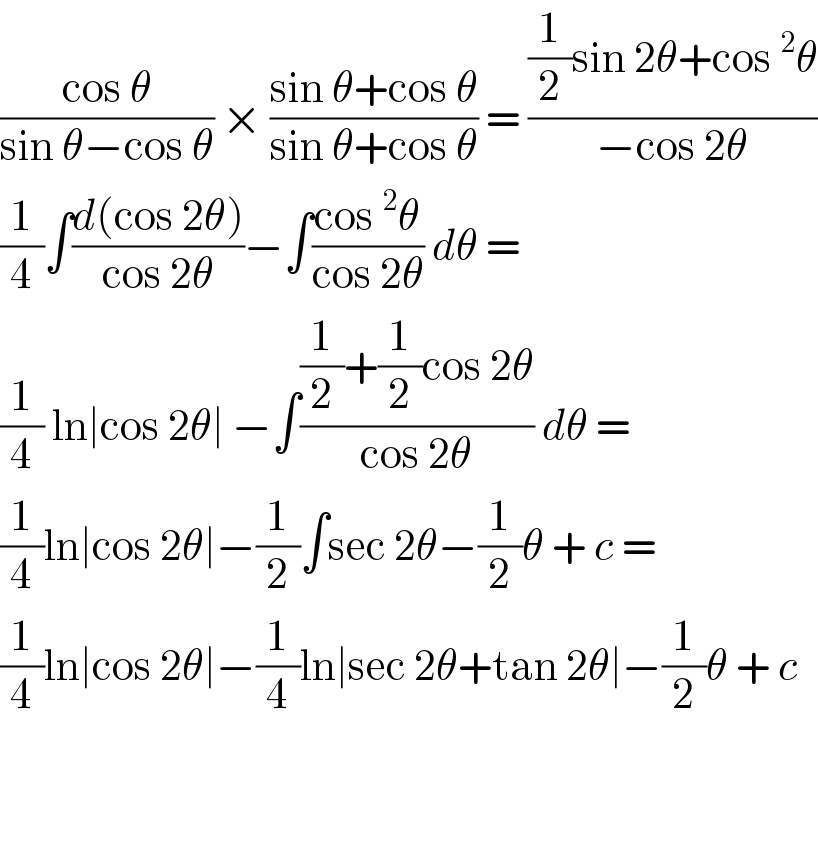
$$\frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta−\mathrm{cos}\:\theta}\:×\:\frac{\mathrm{sin}\:\theta+\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta+\mathrm{cos}\:\theta}\:=\:\frac{\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}\:\mathrm{2}\theta+\mathrm{cos}\:^{\mathrm{2}} \theta}{−\mathrm{cos}\:\mathrm{2}\theta} \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\int\frac{{d}\left(\mathrm{cos}\:\mathrm{2}\theta\right)}{\mathrm{cos}\:\mathrm{2}\theta}−\int\frac{\mathrm{cos}\:^{\mathrm{2}} \theta}{\mathrm{cos}\:\mathrm{2}\theta}\:{d}\theta\:=\: \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{ln}\mid\mathrm{cos}\:\mathrm{2}\theta\mid\:−\int\frac{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\mathrm{2}\theta}{\mathrm{cos}\:\mathrm{2}\theta}\:{d}\theta\:= \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\mathrm{cos}\:\mathrm{2}\theta\mid−\frac{\mathrm{1}}{\mathrm{2}}\int\mathrm{sec}\:\mathrm{2}\theta−\frac{\mathrm{1}}{\mathrm{2}}\theta\:+\:{c}\:= \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\mathrm{cos}\:\mathrm{2}\theta\mid−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\mathrm{sec}\:\mathrm{2}\theta+\mathrm{tan}\:\mathrm{2}\theta\mid−\frac{\mathrm{1}}{\mathrm{2}}\theta\:+\:{c} \\ $$$$ \\ $$$$ \\ $$
Answered by 1549442205 last updated on 07/Jul/20
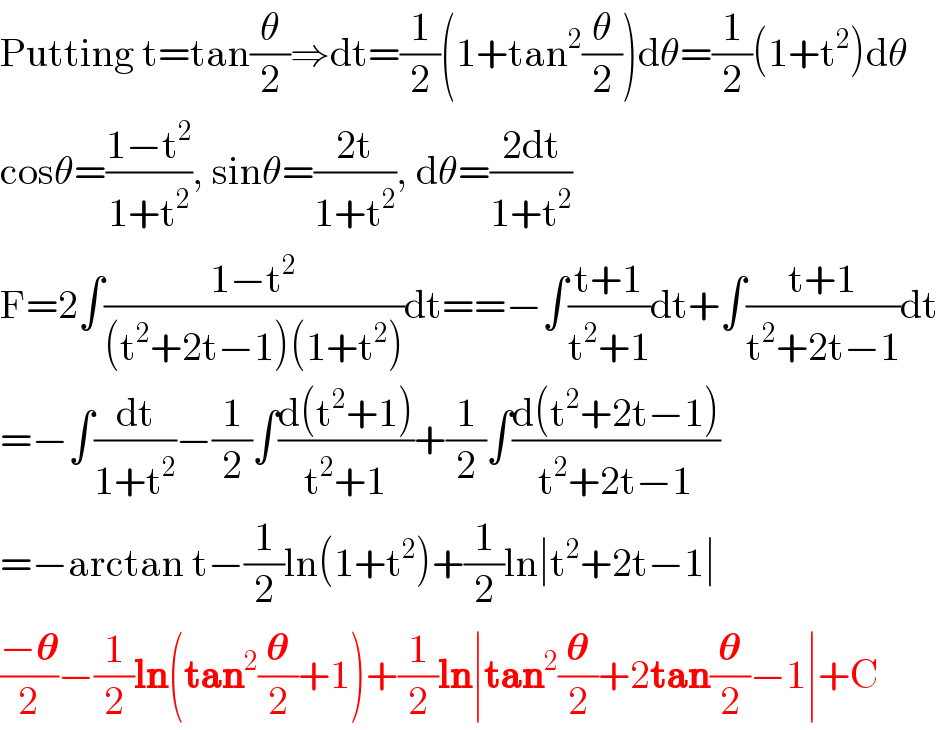
$$\mathrm{Putting}\:\mathrm{t}=\mathrm{tan}\frac{\theta}{\mathrm{2}}\Rightarrow\mathrm{dt}=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}\right)\mathrm{d}\theta=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\mathrm{d}\theta \\ $$$$\mathrm{cos}\theta=\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} },\:\mathrm{sin}\theta=\frac{\mathrm{2t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} },\:\mathrm{d}\theta=\frac{\mathrm{2dt}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} } \\ $$$$\mathrm{F}=\mathrm{2}\int\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\left(\mathrm{t}^{\mathrm{2}} +\mathrm{2t}−\mathrm{1}\right)\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)}\mathrm{dt}==−\int\frac{\mathrm{t}+\mathrm{1}}{\mathrm{t}^{\mathrm{2}} +\mathrm{1}}\mathrm{dt}+\int\frac{\mathrm{t}+\mathrm{1}}{\mathrm{t}^{\mathrm{2}} +\mathrm{2t}−\mathrm{1}}\mathrm{dt} \\ $$$$=−\int\frac{\mathrm{dt}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{d}\left(\mathrm{t}^{\mathrm{2}} +\mathrm{1}\right)}{\mathrm{t}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{d}\left(\mathrm{t}^{\mathrm{2}} +\mathrm{2t}−\mathrm{1}\right)}{\mathrm{t}^{\mathrm{2}} +\mathrm{2t}−\mathrm{1}} \\ $$$$=−\mathrm{arctan}\:\mathrm{t}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\mathrm{t}^{\mathrm{2}} +\mathrm{2t}−\mathrm{1}\mid \\ $$$$\frac{−\boldsymbol{\theta}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\boldsymbol{\mathrm{ln}}\left(\boldsymbol{\mathrm{tan}}^{\mathrm{2}} \frac{\boldsymbol{\theta}}{\mathrm{2}}+\mathrm{1}\right)+\frac{\mathrm{1}}{\mathrm{2}}\boldsymbol{\mathrm{ln}}\mid\boldsymbol{\mathrm{tan}}^{\mathrm{2}} \frac{\boldsymbol{\theta}}{\mathrm{2}}+\mathrm{2}\boldsymbol{\mathrm{tan}}\frac{\boldsymbol{\theta}}{\mathrm{2}}−\mathrm{1}\mid+\mathrm{C} \\ $$
Answered by mathmax by abdo last updated on 08/Jul/20
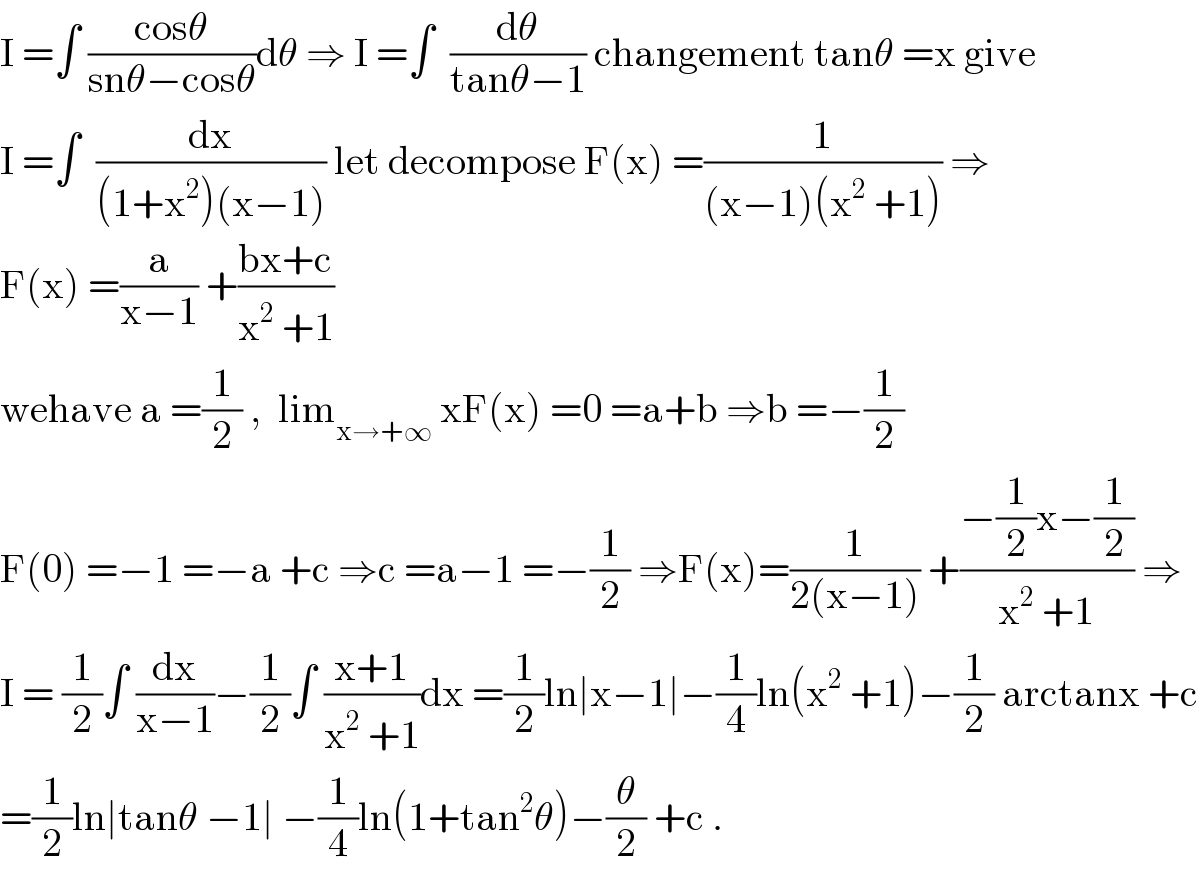
$$\mathrm{I}\:=\int\:\frac{\mathrm{cos}\theta}{\mathrm{sn}\theta−\mathrm{cos}\theta}\mathrm{d}\theta\:\Rightarrow\:\mathrm{I}\:=\int\:\:\frac{\mathrm{d}\theta}{\mathrm{tan}\theta−\mathrm{1}}\:\mathrm{changement}\:\mathrm{tan}\theta\:=\mathrm{x}\:\mathrm{give} \\ $$$$\mathrm{I}\:=\int\:\:\frac{\mathrm{dx}}{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\left(\mathrm{x}−\mathrm{1}\right)}\:\mathrm{let}\:\mathrm{decompose}\:\mathrm{F}\left(\mathrm{x}\right)\:=\frac{\mathrm{1}}{\left(\mathrm{x}−\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)}\:\Rightarrow \\ $$$$\mathrm{F}\left(\mathrm{x}\right)\:=\frac{\mathrm{a}}{\mathrm{x}−\mathrm{1}}\:+\frac{\mathrm{bx}+\mathrm{c}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$$\mathrm{wehave}\:\mathrm{a}\:=\frac{\mathrm{1}}{\mathrm{2}}\:,\:\:\mathrm{lim}_{\mathrm{x}\rightarrow+\infty} \:\mathrm{xF}\left(\mathrm{x}\right)\:=\mathrm{0}\:=\mathrm{a}+\mathrm{b}\:\Rightarrow\mathrm{b}\:=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{F}\left(\mathrm{0}\right)\:=−\mathrm{1}\:=−\mathrm{a}\:+\mathrm{c}\:\Rightarrow\mathrm{c}\:=\mathrm{a}−\mathrm{1}\:=−\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{2}\left(\mathrm{x}−\mathrm{1}\right)}\:+\frac{−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$$\mathrm{I}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\int\:\frac{\mathrm{dx}}{\mathrm{x}−\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{2}}\int\:\frac{\mathrm{x}+\mathrm{1}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\mathrm{x}−\mathrm{1}\mid−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{arctanx}\:+\mathrm{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\mathrm{tan}\theta\:−\mathrm{1}\mid\:−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \theta\right)−\frac{\theta}{\mathrm{2}}\:+\mathrm{c}\:. \\ $$
