Question and Answers Forum

All Questions      Topic List

Differential Equation Questions

Previous in All Question      Next in All Question      

Previous in Differential Equation      Next in Differential Equation      

Question Number 103009 by bobhans last updated on 12/Jul/20

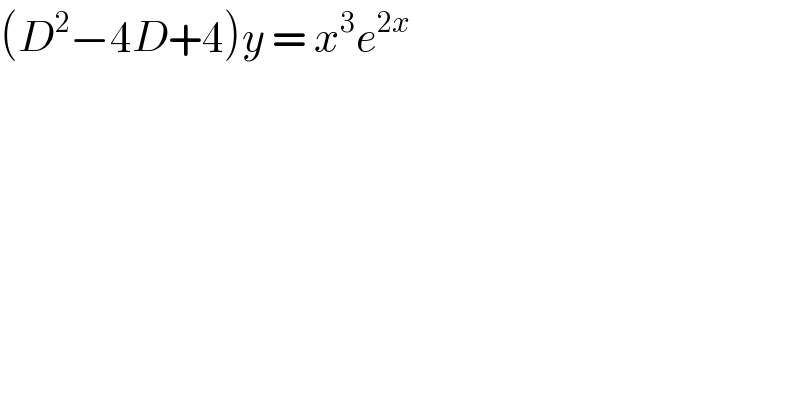
(D^2 −4D+4)y = x^3 e^(2x)

$$\left({D}^{\mathrm{2}} −\mathrm{4}{D}+\mathrm{4}\right){y}\:=\:{x}^{\mathrm{3}} {e}^{\mathrm{2}{x}} \: \\ $$

Answered by bramlex last updated on 12/Jul/20

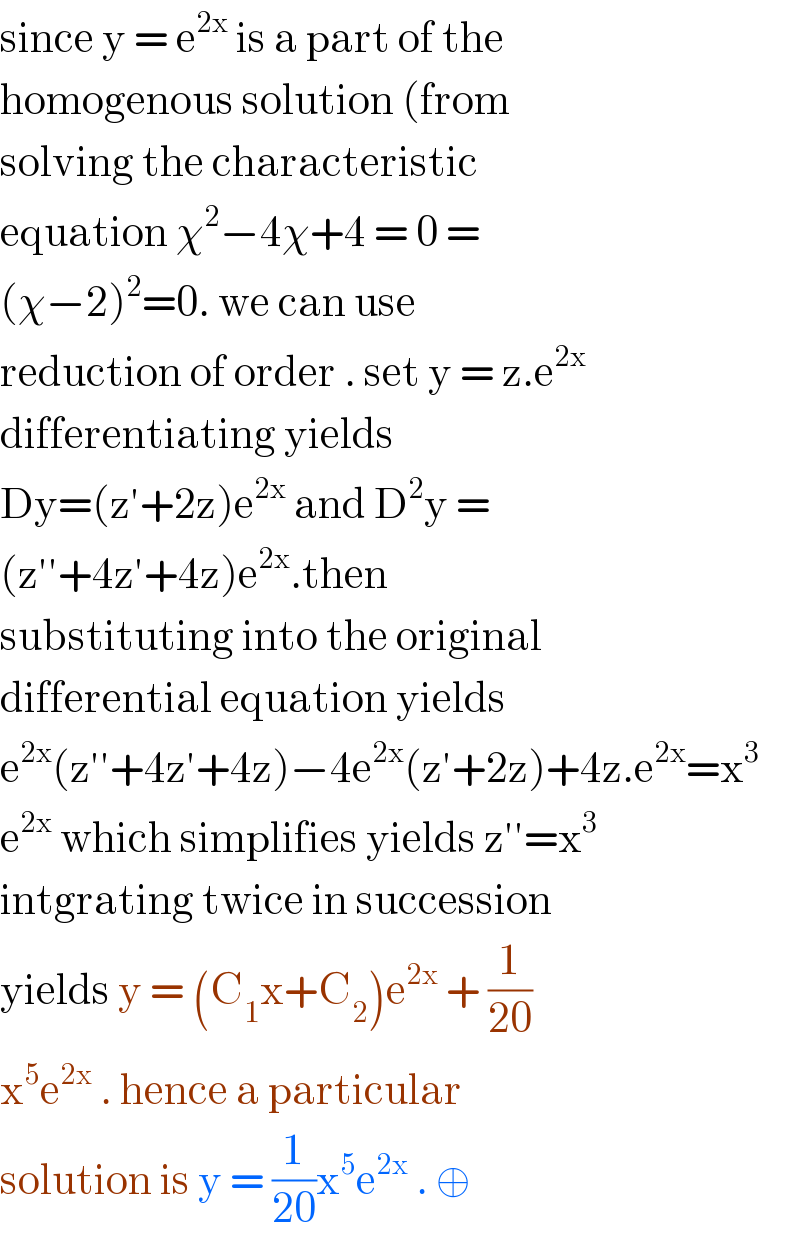
since y = e^(2x) is a part of the homogenous solution (from solving the characteristic equation χ^2 −4χ+4 = 0 = (χ−2)^2 =0. we can use reduction of order . set y = z.e^(2x) differentiating yields Dy=(z′+2z)e^(2x) and D^2 y = (z′′+4z′+4z)e^(2x) .then substituting into the original differential equation yields e^(2x) (z′′+4z′+4z)−4e^(2x) (z′+2z)+4z.e^(2x) =x^3 e^(2x) which simplifies yields z′′=x^3 intgrating twice in succession yields y = (C_1 x+C_2 )e^(2x) + (1/(20)) x^5 e^(2x) . hence a particular solution is y = (1/(20))x^5 e^(2x) . ⊕

$$\mathrm{since}\:\mathrm{y}\:=\:\mathrm{e}^{\mathrm{2x}} \:\mathrm{is}\:\mathrm{a}\:\mathrm{part}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{homogenous}\:\mathrm{solution}\:\left(\mathrm{from}\right. \\ $$$$\mathrm{solving}\:\mathrm{the}\:\mathrm{characteristic} \\ $$$$\mathrm{equation}\:\chi^{\mathrm{2}} −\mathrm{4}\chi+\mathrm{4}\:=\:\mathrm{0}\:= \\ $$$$\left(\chi−\mathrm{2}\right)^{\mathrm{2}} =\mathrm{0}.\:\mathrm{we}\:\mathrm{can}\:\mathrm{use} \\ $$$$\mathrm{reduction}\:\mathrm{of}\:\mathrm{order}\:.\:\mathrm{set}\:\mathrm{y}\:=\:\mathrm{z}.\mathrm{e}^{\mathrm{2x}} \\ $$$$\mathrm{differentiating}\:\mathrm{yields} \\ $$$$\mathrm{Dy}=\left(\mathrm{z}'+\mathrm{2z}\right)\mathrm{e}^{\mathrm{2x}} \:\mathrm{and}\:\mathrm{D}^{\mathrm{2}} \mathrm{y}\:= \\ $$$$\left(\mathrm{z}''+\mathrm{4z}'+\mathrm{4z}\right)\mathrm{e}^{\mathrm{2x}} .\mathrm{then} \\ $$$$\mathrm{substituting}\:\mathrm{into}\:\mathrm{the}\:\mathrm{original} \\ $$$$\mathrm{differential}\:\mathrm{equation}\:\mathrm{yields}\: \\ $$$$\mathrm{e}^{\mathrm{2x}} \left(\mathrm{z}''+\mathrm{4z}'+\mathrm{4z}\right)−\mathrm{4e}^{\mathrm{2x}} \left(\mathrm{z}'+\mathrm{2z}\right)+\mathrm{4z}.\mathrm{e}^{\mathrm{2x}} =\mathrm{x}^{\mathrm{3}} \\ $$$$\mathrm{e}^{\mathrm{2x}} \:\mathrm{which}\:\mathrm{simplifies}\:\mathrm{yields}\:\mathrm{z}''=\mathrm{x}^{\mathrm{3}} \\ $$$$\mathrm{intgrating}\:\mathrm{twice}\:\mathrm{in}\:\mathrm{succession} \\ $$$$\mathrm{yields}\:\mathrm{y}\:=\:\left(\mathrm{C}_{\mathrm{1}} \mathrm{x}+\mathrm{C}_{\mathrm{2}} \right)\mathrm{e}^{\mathrm{2x}} \:+\:\frac{\mathrm{1}}{\mathrm{20}} \\ $$$$\mathrm{x}^{\mathrm{5}} \mathrm{e}^{\mathrm{2x}} \:.\:\mathrm{hence}\:\mathrm{a}\:\mathrm{particular} \\ $$$$\mathrm{solution}\:\mathrm{is}\:\mathrm{y}\:=\:\frac{\mathrm{1}}{\mathrm{20}}\mathrm{x}^{\mathrm{5}} \mathrm{e}^{\mathrm{2x}} \:.\:\oplus \\ $$

Commented by bobhans last updated on 12/Jul/20

yes...

$${yes}... \\ $$

Answered by mathmax by abdo last updated on 12/Jul/20

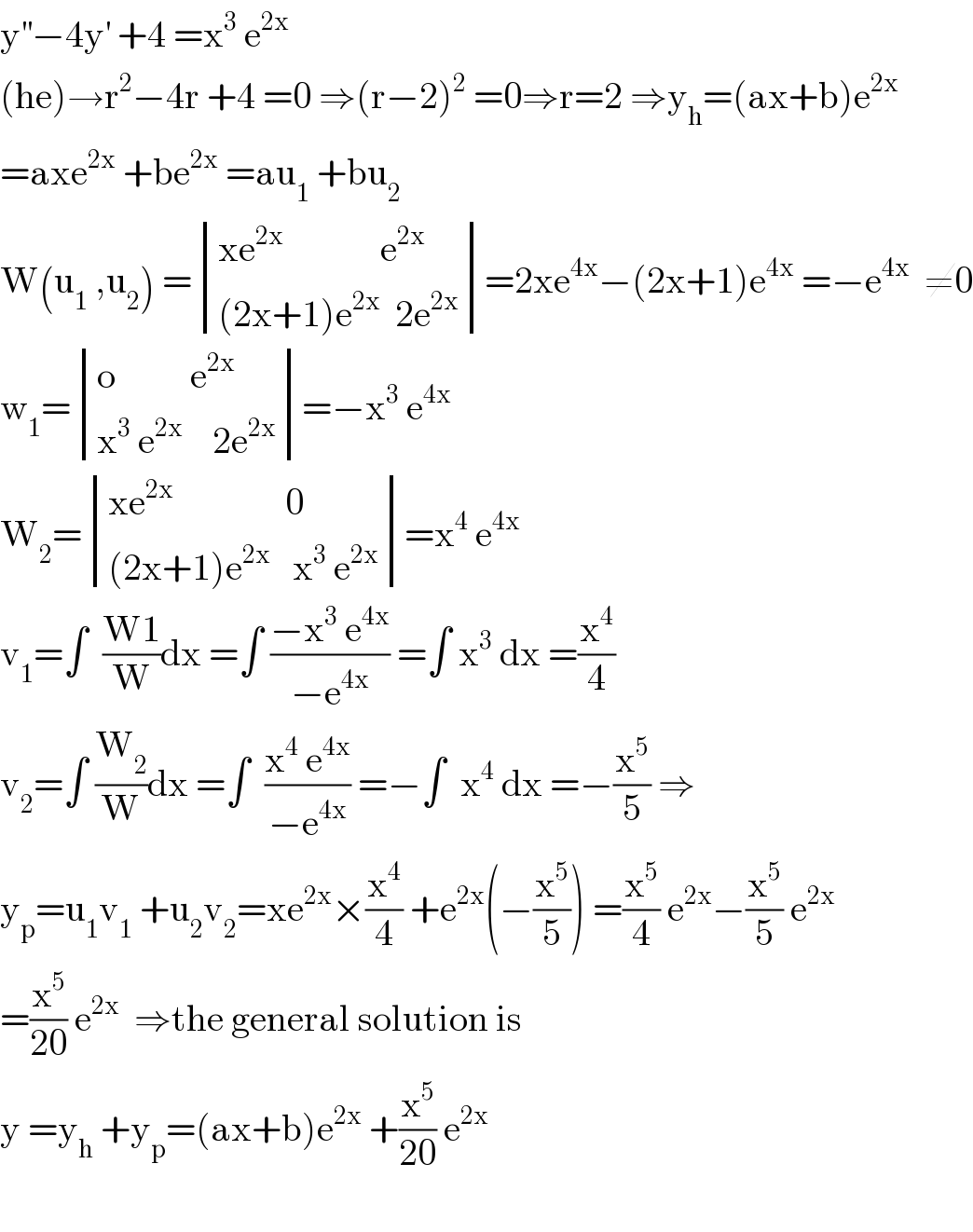
y^(′′) −4y^′ +4 =x^3 e^(2x) (he)→r^2 −4r +4 =0 ⇒(r−2)^2 =0⇒r=2 ⇒y_h =(ax+b)e^(2x) =axe^(2x) +be^(2x) =au_1 +bu_2 W(u_1 ,u_2 ) = determinant (((xe^(2x ) e^(2x) )),(((2x+1)e^(2x) 2e^(2x) )))=2xe^(4x) −(2x+1)e^(4x) =−e^(4x) ≠0 w_1 = determinant (((o e^(2x) )),((x^3 e^(2x) 2e^(2x) )))=−x^3 e^(4x) W_2 = determinant (((xe^(2x) 0)),(((2x+1)e^(2x) x^3 e^(2x) )))=x^4 e^(4x) v_1 =∫ ((W1)/W)dx =∫ ((−x^3 e^(4x) )/(−e^(4x) )) =∫ x^3 dx =(x^4 /4) v_2 =∫ (W_2 /W)dx =∫ ((x^4 e^(4x) )/(−e^(4x) )) =−∫ x^4 dx =−(x^5 /5) ⇒ y_p =u_1 v_1 +u_2 v_2 =xe^(2x) ×(x^4 /4) +e^(2x) (−(x^5 /5)) =(x^5 /4) e^(2x) −(x^5 /5) e^(2x) =(x^5 /(20)) e^(2x) ⇒the general solution is y =y_h +y_p =(ax+b)e^(2x) +(x^5 /(20)) e^(2x)

$$\mathrm{y}^{''} −\mathrm{4y}^{'} \:+\mathrm{4}\:=\mathrm{x}^{\mathrm{3}} \:\mathrm{e}^{\mathrm{2x}} \\ $$$$\left(\mathrm{he}\right)\rightarrow\mathrm{r}^{\mathrm{2}} −\mathrm{4r}\:+\mathrm{4}\:=\mathrm{0}\:\Rightarrow\left(\mathrm{r}−\mathrm{2}\right)^{\mathrm{2}} \:=\mathrm{0}\Rightarrow\mathrm{r}=\mathrm{2}\:\Rightarrow\mathrm{y}_{\mathrm{h}} =\left(\mathrm{ax}+\mathrm{b}\right)\mathrm{e}^{\mathrm{2x}} \\ $$$$=\mathrm{axe}^{\mathrm{2x}} \:+\mathrm{be}^{\mathrm{2x}} \:=\mathrm{au}_{\mathrm{1}} \:+\mathrm{bu}_{\mathrm{2}} \\ $$$$\mathrm{W}\left(\mathrm{u}_{\mathrm{1}} \:,\mathrm{u}_{\mathrm{2}} \right)\:=\begin{vmatrix}{\mathrm{xe}^{\mathrm{2x}\:\:} \:\:\:\:\:\:\:\:\:\:\:\:\mathrm{e}^{\mathrm{2x}} }\\{\left(\mathrm{2x}+\mathrm{1}\right)\mathrm{e}^{\mathrm{2x}} \:\:\mathrm{2e}^{\mathrm{2x}} }\end{vmatrix}=\mathrm{2xe}^{\mathrm{4x}} −\left(\mathrm{2x}+\mathrm{1}\right)\mathrm{e}^{\mathrm{4x}} \:=−\mathrm{e}^{\mathrm{4x}} \:\:\neq\mathrm{0} \\ $$$$\mathrm{w}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{o}\:\:\:\:\:\:\:\:\:\:\mathrm{e}^{\mathrm{2x}} }\\{\mathrm{x}^{\mathrm{3}} \:\mathrm{e}^{\mathrm{2x}} \:\:\:\:\mathrm{2e}^{\mathrm{2x}} }\end{vmatrix}=−\mathrm{x}^{\mathrm{3}} \:\mathrm{e}^{\mathrm{4x}} \\ $$$$\mathrm{W}_{\mathrm{2}} =\begin{vmatrix}{\mathrm{xe}^{\mathrm{2x}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{0}}\\{\left(\mathrm{2x}+\mathrm{1}\right)\mathrm{e}^{\mathrm{2x}} \:\:\:\mathrm{x}^{\mathrm{3}} \:\mathrm{e}^{\mathrm{2x}} }\end{vmatrix}=\mathrm{x}^{\mathrm{4}} \:\mathrm{e}^{\mathrm{4x}} \\ $$$$\mathrm{v}_{\mathrm{1}} =\int\:\:\frac{\mathrm{W1}}{\mathrm{W}}\mathrm{dx}\:=\int\:\frac{−\mathrm{x}^{\mathrm{3}} \:\mathrm{e}^{\mathrm{4x}} }{−\mathrm{e}^{\mathrm{4x}} }\:=\int\:\mathrm{x}^{\mathrm{3}} \:\mathrm{dx}\:=\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{4}} \\ $$$$\mathrm{v}_{\mathrm{2}} =\int\:\frac{\mathrm{W}_{\mathrm{2}} }{\mathrm{W}}\mathrm{dx}\:=\int\:\:\frac{\mathrm{x}^{\mathrm{4}} \:\mathrm{e}^{\mathrm{4x}} }{−\mathrm{e}^{\mathrm{4x}} }\:=−\int\:\:\mathrm{x}^{\mathrm{4}} \:\mathrm{dx}\:=−\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{5}}\:\Rightarrow \\ $$$$\mathrm{y}_{\mathrm{p}} =\mathrm{u}_{\mathrm{1}} \mathrm{v}_{\mathrm{1}} \:+\mathrm{u}_{\mathrm{2}} \mathrm{v}_{\mathrm{2}} =\mathrm{xe}^{\mathrm{2x}} ×\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{4}}\:+\mathrm{e}^{\mathrm{2x}} \left(−\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{5}}\right)\:=\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{4}}\:\mathrm{e}^{\mathrm{2x}} −\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{5}}\:\mathrm{e}^{\mathrm{2x}} \\ $$$$=\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{20}}\:\mathrm{e}^{\mathrm{2x}} \:\:\Rightarrow\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\:\mathrm{is} \\ $$$$\mathrm{y}\:=\mathrm{y}_{\mathrm{h}} \:+\mathrm{y}_{\mathrm{p}} =\left(\mathrm{ax}+\mathrm{b}\right)\mathrm{e}^{\mathrm{2x}} \:+\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{20}}\:\mathrm{e}^{\mathrm{2x}} \\ $$$$ \\ $$

Commented by bemath last updated on 12/Jul/20

sir Abdo & sir Bramlex coll

$${sir}\:{Abdo}\:\&\:{sir}\:{Bramlex}\:{coll}\: \\ $$

Commented by mathmax by abdo last updated on 13/Jul/20

thanks sir

$$\mathrm{thanks}\:\mathrm{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com