if an object moves along a straight line according to the relationship( x(t)=((1/2)t^2 −t+2)) find (1) the average speed between (x=(3/2) , x=(7/2)) (2) the pelvic velocity between (x=(7/2))


Question and Answers Forum

All Questions Topic List
None Questions
Previous in All Question Next in All Question
Previous in None Next in None
Question Number 103703 by mohammad17 last updated on 16/Jul/20

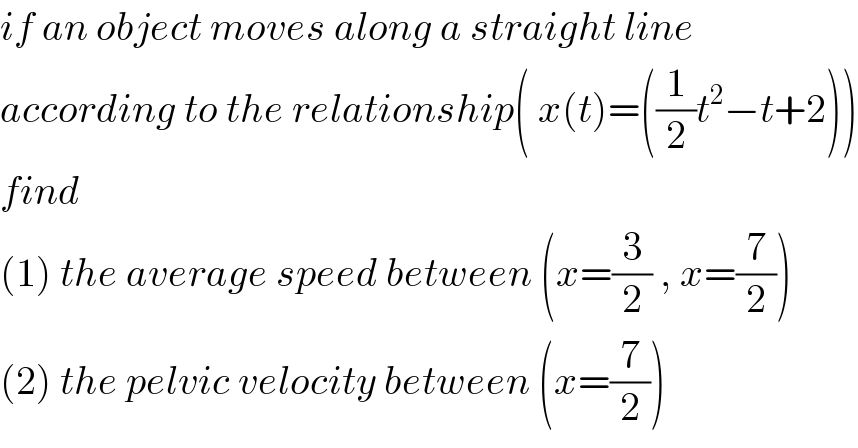
$i f a n o b j e c t m o v e s a l o n g a s t r a i g h t l i n e$ $a c c o r d i n g t o t h e r e l a t i o n s h i p (x (t) = (\frac{1}{2} t^{2} - t + 2))$ $f i n d$ $(1) t h e a v e r a g e s p e e d b e t w e e n (x = \frac{3}{2}, x = \frac{7}{2})$ $(2) t h e p e l v i c v e l o c i t y b e t w e e n (x = \frac{7}{2})$
	Answered by Dwaipayan Shikari last updated on 16/Jul/20

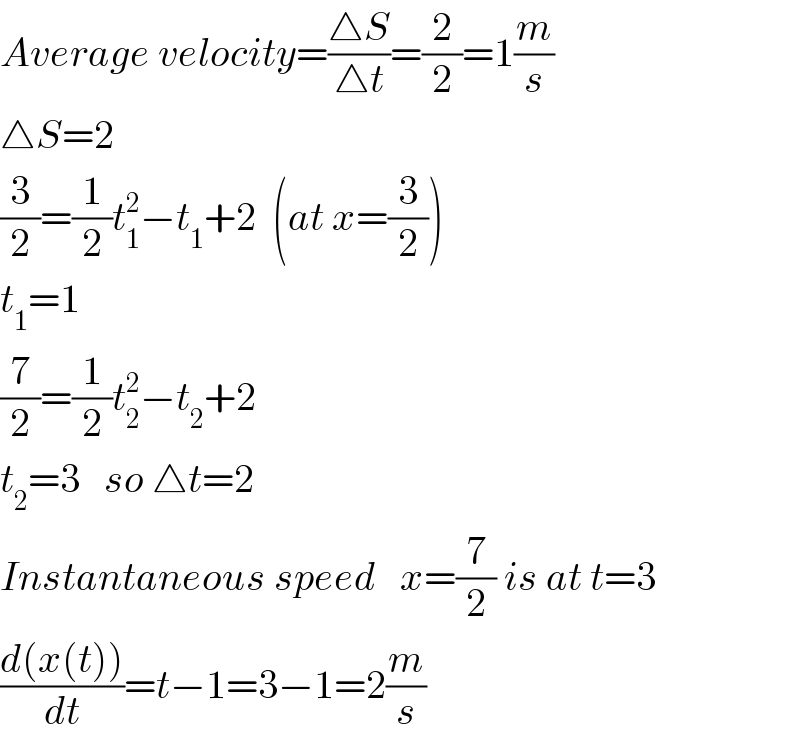
	$A v e r a g e v e l o c i t y = \frac{△ S}{△ t} = \frac{2}{2} = 1 \frac{m}{s}$ $△ S = 2$ $\frac{3}{2} = \frac{1}{2} t_{1}^{2} - t_{1} + 2 (a t x = \frac{3}{2})$ $t_{1} = 1$ $\frac{7}{2} = \frac{1}{2} t_{2}^{2} - t_{2} + 2$ $t_{2} = 3 s o △ t = 2$ $I n s t a n t a n e o u s s p e e d x = \frac{7}{2} i s a t t = 3$ $\frac{d (x (t))}{d t} = t - 1 = 3 - 1 = 2 \frac{m}{s}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum