Question and Answers Forum

All Questions      Topic List

Differential Equation Questions

Previous in All Question      Next in All Question      

Previous in Differential Equation      Next in Differential Equation      

Question Number 104595 by Ar Brandon last updated on 22/Jul/20

Solve the DE x′′(t)+2x′(t)+x(t)=te^(−t)

$$\mathrm{Solve}\:\mathrm{the}\:\mathcal{DE} \\ $$$$\mathrm{x}''\left(\mathrm{t}\right)+\mathrm{2x}'\left(\mathrm{t}\right)+\mathrm{x}\left(\mathrm{t}\right)=\mathrm{te}^{−\mathrm{t}} \\ $$

Answered by mathmax by abdo last updated on 22/Jul/20

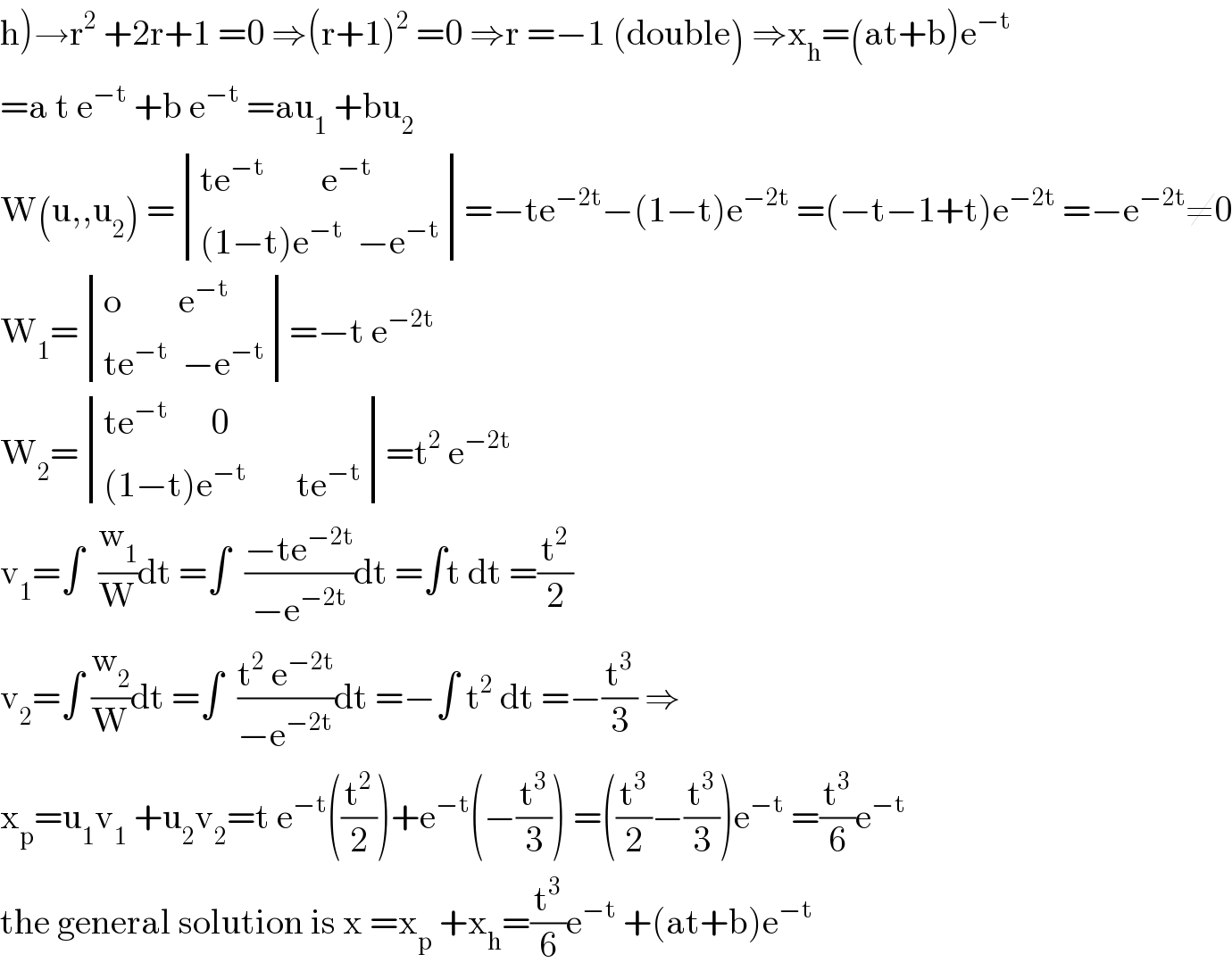
h)→r^2 +2r+1 =0 ⇒(r+1)^2 =0 ⇒r =−1 (double) ⇒x_h =(at+b)e^(−t) =a t e^(−t) +b e^(−t) =au_1 +bu_2 W(u,,u_2 ) = determinant (((te^(−t) e^(−t) )),(((1−t)e^(−t) −e^(−t) )))=−te^(−2t) −(1−t)e^(−2t) =(−t−1+t)e^(−2t) =−e^(−2t) ≠0 W_1 = determinant (((o e^(−t) )),((te^(−t) −e^(−t) )))=−t e^(−2t) W_2 = determinant (((te^(−t) 0)),(((1−t)e^(−t) te^(−t) )))=t^2 e^(−2t) v_1 =∫ (w_1 /W)dt =∫ ((−te^(−2t) )/(−e^(−2t) ))dt =∫t dt =(t^2 /2) v_2 =∫ (w_2 /W)dt =∫ ((t^2 e^(−2t) )/(−e^(−2t) ))dt =−∫ t^2 dt =−(t^3 /3) ⇒ x_p =u_1 v_1 +u_2 v_2 =t e^(−t) ((t^2 /2))+e^(−t) (−(t^3 /3)) =((t^3 /2)−(t^3 /3))e^(−t) =(t^3 /6)e^(−t) the general solution is x =x_p +x_h =(t^3 /6)e^(−t) +(at+b)e^(−t)

$$\left.\mathrm{h}\right)\rightarrow\mathrm{r}^{\mathrm{2}} \:+\mathrm{2r}+\mathrm{1}\:=\mathrm{0}\:\Rightarrow\left(\mathrm{r}+\mathrm{1}\right)^{\mathrm{2}} \:=\mathrm{0}\:\Rightarrow\mathrm{r}\:=−\mathrm{1}\:\left(\mathrm{double}\right)\:\Rightarrow\mathrm{x}_{\mathrm{h}} =\left(\mathrm{at}+\mathrm{b}\right)\mathrm{e}^{−\mathrm{t}} \\ $$$$=\mathrm{a}\:\mathrm{t}\:\mathrm{e}^{−\mathrm{t}} \:+\mathrm{b}\:\mathrm{e}^{−\mathrm{t}} \:=\mathrm{au}_{\mathrm{1}} \:+\mathrm{bu}_{\mathrm{2}} \\ $$$$\mathrm{W}\left(\mathrm{u},,\mathrm{u}_{\mathrm{2}} \right)\:=\begin{vmatrix}{\mathrm{te}^{−\mathrm{t}} \:\:\:\:\:\:\:\:\mathrm{e}^{−\mathrm{t}} }\\{\left(\mathrm{1}−\mathrm{t}\right)\mathrm{e}^{−\mathrm{t}} \:\:−\mathrm{e}^{−\mathrm{t}} }\end{vmatrix}=−\mathrm{te}^{−\mathrm{2t}} −\left(\mathrm{1}−\mathrm{t}\right)\mathrm{e}^{−\mathrm{2t}} \:=\left(−\mathrm{t}−\mathrm{1}+\mathrm{t}\right)\mathrm{e}^{−\mathrm{2t}} \:=−\mathrm{e}^{−\mathrm{2t}} \neq\mathrm{0} \\ $$$$\mathrm{W}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{o}\:\:\:\:\:\:\:\:\mathrm{e}^{−\mathrm{t}} }\\{\mathrm{te}^{−\mathrm{t}} \:\:−\mathrm{e}^{−\mathrm{t}} }\end{vmatrix}=−\mathrm{t}\:\mathrm{e}^{−\mathrm{2t}} \\ $$$$\mathrm{W}_{\mathrm{2}} =\begin{vmatrix}{\mathrm{te}^{−\mathrm{t}} \:\:\:\:\:\:\mathrm{0}}\\{\left(\mathrm{1}−\mathrm{t}\right)\mathrm{e}^{−\mathrm{t}} \:\:\:\:\:\:\:\mathrm{te}^{−\mathrm{t}} }\end{vmatrix}=\mathrm{t}^{\mathrm{2}} \:\mathrm{e}^{−\mathrm{2t}} \\ $$$$\mathrm{v}_{\mathrm{1}} =\int\:\:\frac{\mathrm{w}_{\mathrm{1}} }{\mathrm{W}}\mathrm{dt}\:=\int\:\:\frac{−\mathrm{te}^{−\mathrm{2t}} }{−\mathrm{e}^{−\mathrm{2t}} }\mathrm{dt}\:=\int\mathrm{t}\:\mathrm{dt}\:=\frac{\mathrm{t}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\mathrm{v}_{\mathrm{2}} =\int\:\frac{\mathrm{w}_{\mathrm{2}} }{\mathrm{W}}\mathrm{dt}\:=\int\:\:\frac{\mathrm{t}^{\mathrm{2}} \:\mathrm{e}^{−\mathrm{2t}} }{−\mathrm{e}^{−\mathrm{2t}} }\mathrm{dt}\:=−\int\:\mathrm{t}^{\mathrm{2}} \:\mathrm{dt}\:=−\frac{\mathrm{t}^{\mathrm{3}} }{\mathrm{3}}\:\Rightarrow \\ $$$$\mathrm{x}_{\mathrm{p}} =\mathrm{u}_{\mathrm{1}} \mathrm{v}_{\mathrm{1}} \:+\mathrm{u}_{\mathrm{2}} \mathrm{v}_{\mathrm{2}} =\mathrm{t}\:\mathrm{e}^{−\mathrm{t}} \left(\frac{\mathrm{t}^{\mathrm{2}} }{\mathrm{2}}\right)+\mathrm{e}^{−\mathrm{t}} \left(−\frac{\mathrm{t}^{\mathrm{3}} }{\mathrm{3}}\right)\:=\left(\frac{\mathrm{t}^{\mathrm{3}} }{\mathrm{2}}−\frac{\mathrm{t}^{\mathrm{3}} }{\mathrm{3}}\right)\mathrm{e}^{−\mathrm{t}} \:=\frac{\mathrm{t}^{\mathrm{3}} }{\mathrm{6}}\mathrm{e}^{−\mathrm{t}} \\ $$$$\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\:\mathrm{is}\:\mathrm{x}\:=\mathrm{x}_{\mathrm{p}} \:+\mathrm{x}_{\mathrm{h}} =\frac{\mathrm{t}^{\mathrm{3}} }{\mathrm{6}}\mathrm{e}^{−\mathrm{t}} \:+\left(\mathrm{at}+\mathrm{b}\right)\mathrm{e}^{−\mathrm{t}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com