Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 108217 by john santu last updated on 15/Aug/20

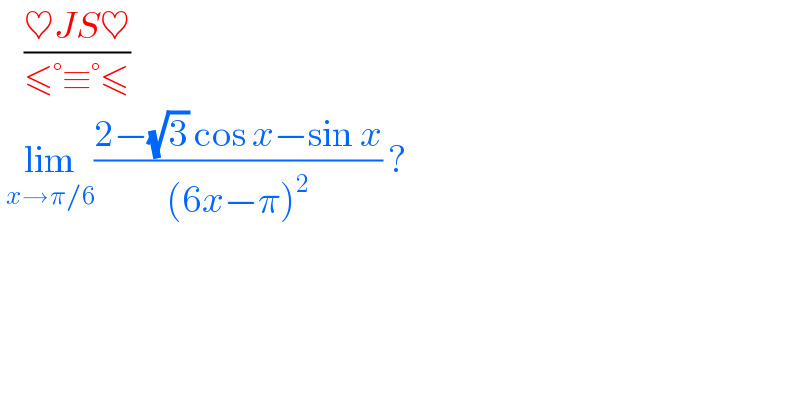
((♥JS♥)/(≤°≡°≤)) lim_(x→π/6) ((2−(√3) cos x−sin x)/((6x−π)^2 )) ?

$$\:\:\:\:\frac{\heartsuit{JS}\heartsuit}{\leqslant°\equiv°\leqslant} \\ $$$$\:\underset{{x}\rightarrow\pi/\mathrm{6}} {\mathrm{lim}}\frac{\mathrm{2}−\sqrt{\mathrm{3}}\:\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}{\left(\mathrm{6}{x}−\pi\right)^{\mathrm{2}} }\:?\: \\ $$

Commented by john santu last updated on 15/Aug/20

good all sir...

$${good}\:{all}\:{sir}... \\ $$

Answered by bemath last updated on 15/Aug/20

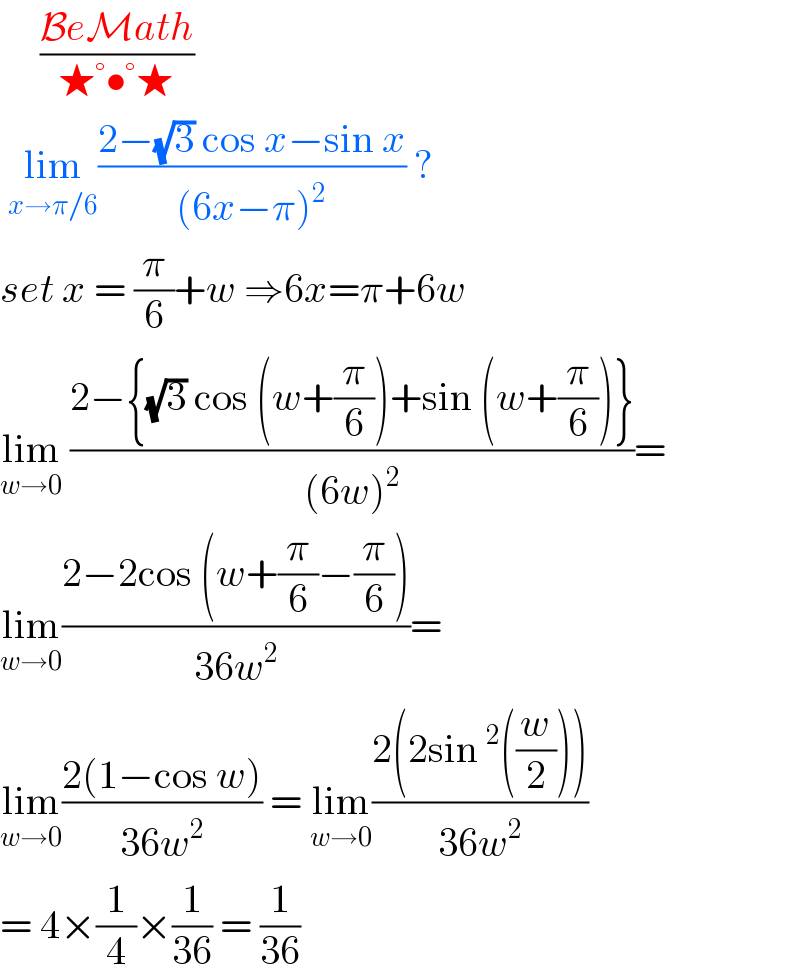
((BeMath)/(★°•°★)) lim_(x→π/6) ((2−(√3) cos x−sin x)/((6x−π)^2 )) ? set x = (π/6)+w ⇒6x=π+6w lim_(w→0) ((2−{(√3) cos (w+(π/6))+sin (w+(π/6))})/((6w)^2 ))= lim_(w→0) ((2−2cos (w+(π/6)−(π/6)))/(36w^2 ))= lim_(w→0) ((2(1−cos w))/(36w^2 )) = lim_(w→0) ((2(2sin^2 ((w/2))))/(36w^2 )) = 4×(1/4)×(1/(36)) = (1/(36))

$$\:\:\:\:\:\frac{\mathcal{B}{e}\mathcal{M}{ath}}{\bigstar°\bullet°\bigstar} \\ $$$$\:\underset{{x}\rightarrow\pi/\mathrm{6}} {\mathrm{lim}}\frac{\mathrm{2}−\sqrt{\mathrm{3}}\:\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}{\left(\mathrm{6}{x}−\pi\right)^{\mathrm{2}} }\:? \\ $$$${set}\:{x}\:=\:\frac{\pi}{\mathrm{6}}+{w}\:\Rightarrow\mathrm{6}{x}=\pi+\mathrm{6}{w} \\ $$$$\underset{{w}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{2}−\left\{\sqrt{\mathrm{3}}\:\mathrm{cos}\:\left({w}+\frac{\pi}{\mathrm{6}}\right)+\mathrm{sin}\:\left({w}+\frac{\pi}{\mathrm{6}}\right)\right\}}{\left(\mathrm{6}{w}\right)^{\mathrm{2}} }= \\ $$$$\underset{{w}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}−\mathrm{2cos}\:\left({w}+\frac{\pi}{\mathrm{6}}−\frac{\pi}{\mathrm{6}}\right)}{\mathrm{36}{w}^{\mathrm{2}} }= \\ $$$$\underset{{w}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}\left(\mathrm{1}−\mathrm{cos}\:{w}\right)}{\mathrm{36}{w}^{\mathrm{2}} }\:=\:\underset{{w}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}\left(\mathrm{2sin}\:^{\mathrm{2}} \left(\frac{{w}}{\mathrm{2}}\right)\right)}{\mathrm{36}{w}^{\mathrm{2}} } \\ $$$$=\:\mathrm{4}×\frac{\mathrm{1}}{\mathrm{4}}×\frac{\mathrm{1}}{\mathrm{36}}\:=\:\frac{\mathrm{1}}{\mathrm{36}} \\ $$

Answered by 1549442205PVT last updated on 15/Aug/20

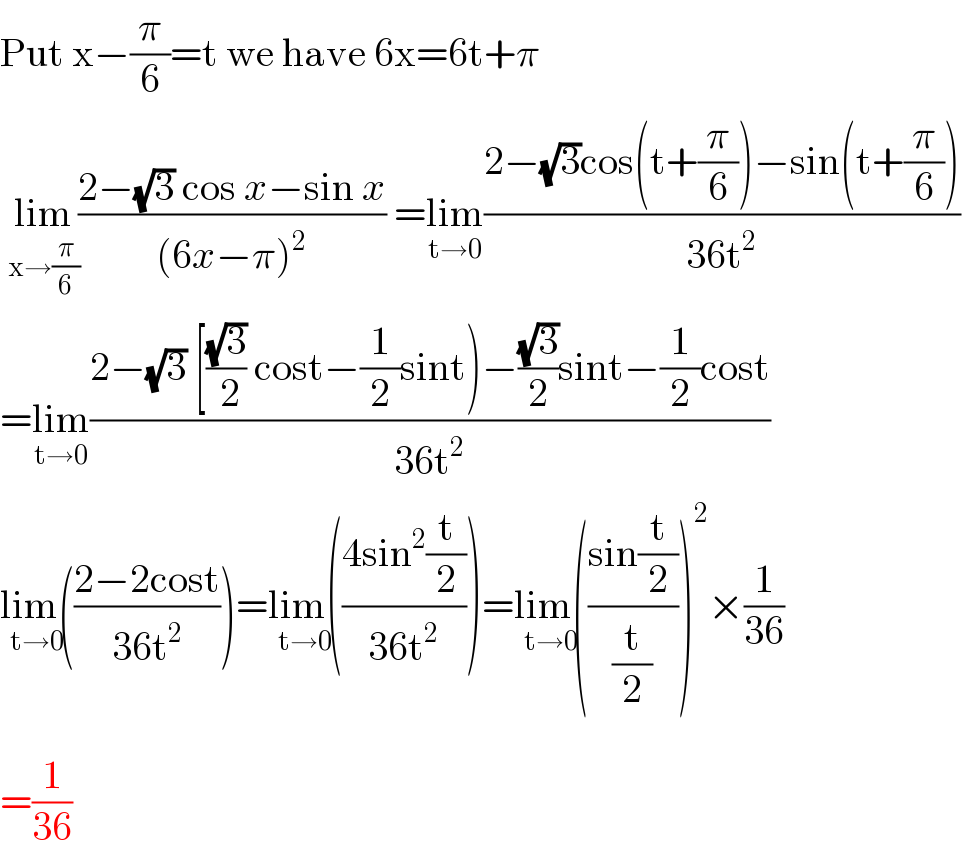
Put x−(π/6)=t we have 6x=6t+π lim_(x→(π/6)) ((2−(√3) cos x−sin x)/((6x−π)^2 )) =lim_(t→0) ((2−(√3)cos(t+(π/6))−sin(t+(π/6)))/(36t^2 )) =lim_(t→0) ((2−(√3) [((√3)/( 2)) cost−(1/2)sint)−((√3)/2)sint−(1/2)cost)/(36t^2 )) lim(_(t→0) ((2−2cost)/(36t^2 )))=lim(_(t→0) ((4sin^2 (t/2))/(36t^2 )))=lim(_(t→0) ((sin(t/2))/(t/2)))^2 ×(1/(36)) =(1/(36))

$$\mathrm{Put}\:\mathrm{x}−\frac{\pi}{\mathrm{6}}=\mathrm{t}\:\mathrm{we}\:\mathrm{have}\:\mathrm{6x}=\mathrm{6t}+\pi \\ $$$$\:\underset{\mathrm{x}\rightarrow\frac{\pi}{\mathrm{6}}} {\mathrm{lim}}\frac{\mathrm{2}−\sqrt{\mathrm{3}}\:\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}{\left(\mathrm{6}{x}−\pi\right)^{\mathrm{2}} }\:=\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}−\sqrt{\mathrm{3}}\mathrm{cos}\left(\mathrm{t}+\frac{\pi}{\mathrm{6}}\right)−\mathrm{sin}\left(\mathrm{t}+\frac{\pi}{\mathrm{6}}\right)}{\mathrm{36t}^{\mathrm{2}} } \\ $$$$=\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}−\sqrt{\mathrm{3}}\:\left[\frac{\sqrt{\mathrm{3}}}{\:\mathrm{2}}\:\mathrm{cost}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sint}\right)−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{sint}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cost}}{\mathrm{36t}^{\mathrm{2}} } \\ $$$$\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}\left(}\frac{\mathrm{2}−\mathrm{2cost}}{\mathrm{36t}^{\mathrm{2}} }\right)=\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}\left(}\frac{\mathrm{4sin}^{\mathrm{2}} \frac{\mathrm{t}}{\mathrm{2}}}{\mathrm{36t}^{\mathrm{2}} }\right)=\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}\left(}\frac{\mathrm{sin}\frac{\mathrm{t}}{\mathrm{2}}}{\frac{\mathrm{t}}{\mathrm{2}}}\right)^{\mathrm{2}} ×\frac{\mathrm{1}}{\mathrm{36}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{36}} \\ $$

Answered by Dwaipayan Shikari last updated on 15/Aug/20

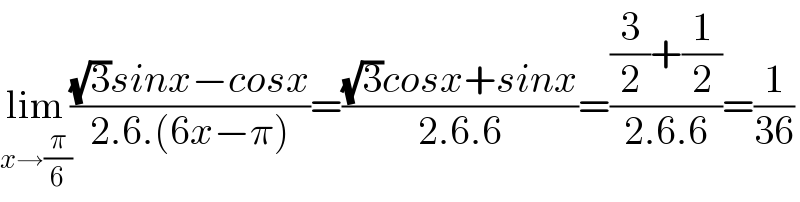
lim_(x→(π/6)) (((√3)sinx−cosx)/(2.6.(6x−π)))=(((√3)cosx+sinx)/(2.6.6))=(((3/2)+(1/2))/(2.6.6))=(1/(36))

$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{6}}} {\mathrm{lim}}\frac{\sqrt{\mathrm{3}}{sinx}−{cosx}}{\mathrm{2}.\mathrm{6}.\left(\mathrm{6}{x}−\pi\right)}=\frac{\sqrt{\mathrm{3}}{cosx}+{sinx}}{\mathrm{2}.\mathrm{6}.\mathrm{6}}=\frac{\frac{\mathrm{3}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}}{\mathrm{2}.\mathrm{6}.\mathrm{6}}=\frac{\mathrm{1}}{\mathrm{36}} \\ $$

Answered by mathmax by abdo last updated on 15/Aug/20

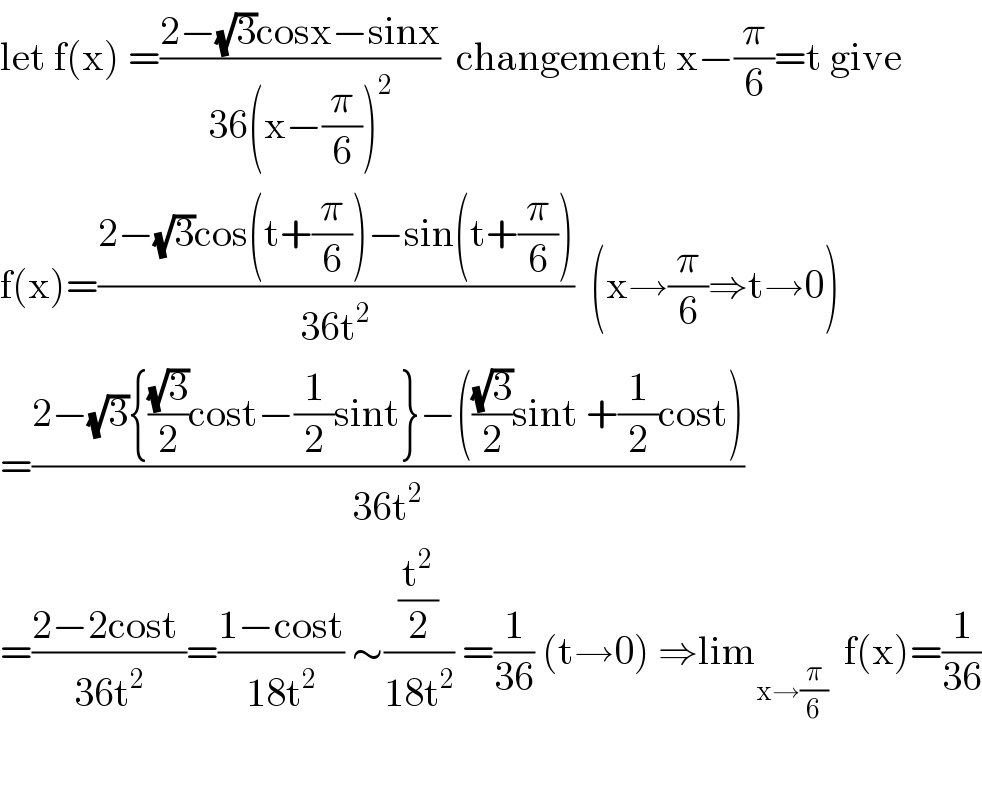
let f(x) =((2−(√3)cosx−sinx)/(36(x−(π/6))^2 )) changement x−(π/6)=t give f(x)=((2−(√3)cos(t+(π/6))−sin(t+(π/6)))/(36t^2 )) (x→(π/6)⇒t→0) =((2−(√3){((√3)/2)cost−(1/2)sint}−(((√3)/2)sint +(1/2)cost))/(36t^2 )) =((2−2cost )/(36t^2 ))=((1−cost)/(18t^2 )) ∼((t^2 /2)/(18t^2 )) =(1/(36)) (t→0) ⇒lim_(x→(π/6)) f(x)=(1/(36))

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)\:=\frac{\mathrm{2}−\sqrt{\mathrm{3}}\mathrm{cosx}−\mathrm{sinx}}{\mathrm{36}\left(\mathrm{x}−\frac{\pi}{\mathrm{6}}\right)^{\mathrm{2}} }\:\:\mathrm{changement}\:\mathrm{x}−\frac{\pi}{\mathrm{6}}=\mathrm{t}\:\mathrm{give} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{2}−\sqrt{\mathrm{3}}\mathrm{cos}\left(\mathrm{t}+\frac{\pi}{\mathrm{6}}\right)−\mathrm{sin}\left(\mathrm{t}+\frac{\pi}{\mathrm{6}}\right)}{\mathrm{36t}^{\mathrm{2}} }\:\:\left(\mathrm{x}\rightarrow\frac{\pi}{\mathrm{6}}\Rightarrow\mathrm{t}\rightarrow\mathrm{0}\right) \\ $$$$=\frac{\mathrm{2}−\sqrt{\mathrm{3}}\left\{\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{cost}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sint}\right\}−\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{sint}\:+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cost}\right)}{\mathrm{36t}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{2}−\mathrm{2cost}\:}{\mathrm{36t}^{\mathrm{2}} }=\frac{\mathrm{1}−\mathrm{cost}}{\mathrm{18t}^{\mathrm{2}} }\:\sim\frac{\frac{\mathrm{t}^{\mathrm{2}} }{\mathrm{2}}}{\mathrm{18t}^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{36}}\:\left(\mathrm{t}\rightarrow\mathrm{0}\right)\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow\frac{\pi}{\mathrm{6}}} \:\:\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{36}} \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com