
Previous in Differential Equation Next in Differential Equation
Question Number 108473 by subhankar10 last updated on 17/Aug/20

$$\mathrm{3x}^{\mathrm{3}} +\mathrm{4x}−\mathrm{3}=\mathrm{0} \\ $$$$\mathrm{solve}\:\mathrm{this}\:\mathrm{problem}. \\ $$
Answered by Sarah85 last updated on 17/Aug/20
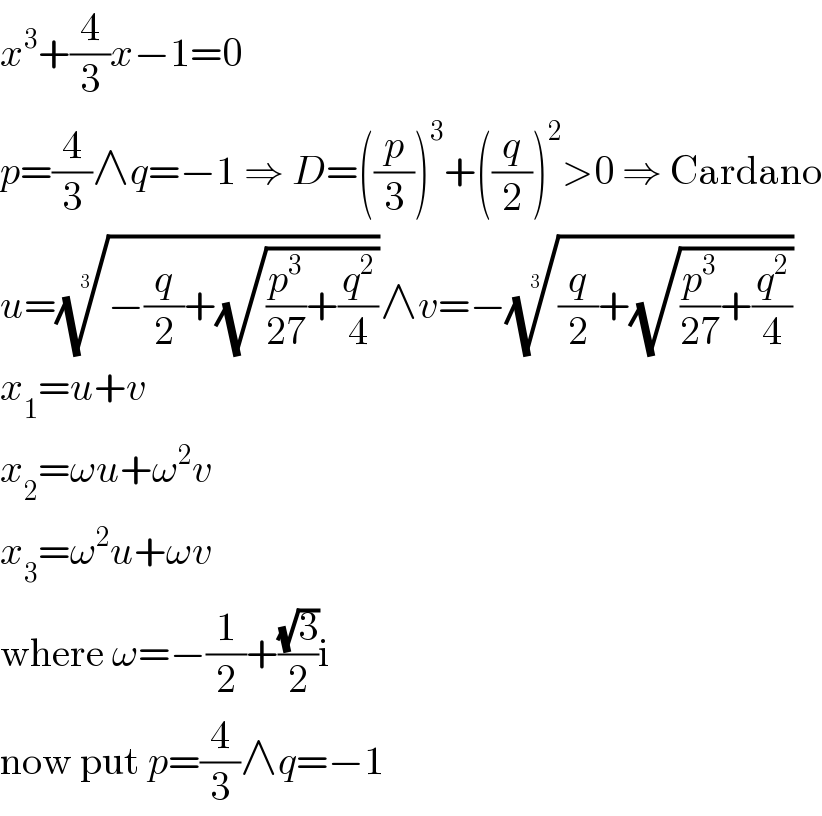
$${x}^{\mathrm{3}} +\frac{\mathrm{4}}{\mathrm{3}}{x}−\mathrm{1}=\mathrm{0} \\ $$$${p}=\frac{\mathrm{4}}{\mathrm{3}}\wedge{q}=−\mathrm{1}\:\Rightarrow\:{D}=\left(\frac{{p}}{\mathrm{3}}\right)^{\mathrm{3}} +\left(\frac{{q}}{\mathrm{2}}\right)^{\mathrm{2}} >\mathrm{0}\:\Rightarrow\:\mathrm{Cardano} \\ $$$${u}=\sqrt[{\mathrm{3}}]{−\frac{{q}}{\mathrm{2}}+\sqrt{\frac{{p}^{\mathrm{3}} }{\mathrm{27}}+\frac{{q}^{\mathrm{2}} }{\mathrm{4}}}}\wedge{v}=−\sqrt[{\mathrm{3}}]{\frac{{q}}{\mathrm{2}}+\sqrt{\frac{{p}^{\mathrm{3}} }{\mathrm{27}}+\frac{{q}^{\mathrm{2}} }{\mathrm{4}}}} \\ $$$${x}_{\mathrm{1}} ={u}+{v} \\ $$$${x}_{\mathrm{2}} =\omega{u}+\omega^{\mathrm{2}} {v} \\ $$$${x}_{\mathrm{3}} =\omega^{\mathrm{2}} {u}+\omega{v} \\ $$$$\mathrm{where}\:\omega=−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{i} \\ $$$$\mathrm{now}\:\mathrm{put}\:{p}=\frac{\mathrm{4}}{\mathrm{3}}\wedge{q}=−\mathrm{1} \\ $$
Answered by 1549442205PVT last updated on 17/Aug/20
![3x^3 +4x−3=0(1) Put x=(2/3)y then (1)⇔(8/9)y^3 +(8/3)y−3=0 ⇔y^3 +3y−((27)/8)=0 (2).Put y=z−(1/z) (2)⇔z^3 −(1/z^3 )−3(z−(1/z))+3(z−(1/z))−((27)/8)=0 ⇔z^3 −(1/z^3 )−((27)/8)=0.Put z^3 =t we get t−(1/t)−((27)/8)=0⇔8t^2 −27t−8=0 Δ=27^2 +4.8^2 =729+256=985 ⇒z^3 =t=((27±(√(985)))/(16))⇒z=^3 (√((27±(√(985)))/(16))) ⇒(1/z)=^3 (√((16)/(27±(√(985))))) i)For z=^3 (√((27+(√(985)))/(16)))⇒(1/z)=^3 (√((16)/(27+(√(985))))) we get x_1 =(2/3)y=(2/3)(z−(1/z))= =(2/3)[^3 (√((27+(√(985)))/(16)))−^3 (√((16)/(27+(√(985)))))] ii)For z=^3 (√((27−(√(985)))/(16)))⇒(1/z)=^3 (√((16)/(27−(√(985))))) z−(1/z)= [3(√((27−(√(985)))/(16)))−^3 (√((16)/(27−(√(985)))))] =[^3 (√((−256)/(16(27+(√(985)))))−^3 (√((16(27+(√(985))))/(−256))) =^3 (√((−16)/(27+(√(985)))))−^3 (√((27+(√(985)))/(−16))) =^3 (√((27+(√(985)))/(16)))−^3 (√((16)/(27+(√(985))))) we get x_2 =(2/3)y=(2/3)(z−(1/z))=x_1 Thus,the given equation has unique solution x=(2/3)[3(√((27+(√(985)))/(16)))−^3 (√((16)/(27+(√(985)))))]](Q108511.png)
$$\mathrm{3x}^{\mathrm{3}} +\mathrm{4x}−\mathrm{3}=\mathrm{0}\left(\mathrm{1}\right) \\ $$$$\mathrm{Put}\:\mathrm{x}=\frac{\mathrm{2}}{\mathrm{3}}\mathrm{y}\:\mathrm{then}\:\left(\mathrm{1}\right)\Leftrightarrow\frac{\mathrm{8}}{\mathrm{9}}\mathrm{y}^{\mathrm{3}} +\frac{\mathrm{8}}{\mathrm{3}}\mathrm{y}−\mathrm{3}=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{y}^{\mathrm{3}} +\mathrm{3y}−\frac{\mathrm{27}}{\mathrm{8}}=\mathrm{0}\:\left(\mathrm{2}\right).\mathrm{Put}\:\mathrm{y}=\mathrm{z}−\frac{\mathrm{1}}{\mathrm{z}} \\ $$$$\left(\mathrm{2}\right)\Leftrightarrow\mathrm{z}^{\mathrm{3}} −\frac{\mathrm{1}}{\mathrm{z}^{\mathrm{3}} }−\mathrm{3}\left(\mathrm{z}−\frac{\mathrm{1}}{\mathrm{z}}\right)+\mathrm{3}\left(\mathrm{z}−\frac{\mathrm{1}}{\mathrm{z}}\right)−\frac{\mathrm{27}}{\mathrm{8}}=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{z}^{\mathrm{3}} −\frac{\mathrm{1}}{\mathrm{z}^{\mathrm{3}} }−\frac{\mathrm{27}}{\mathrm{8}}=\mathrm{0}.\mathrm{Put}\:\mathrm{z}^{\mathrm{3}} =\mathrm{t}\:\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{t}−\frac{\mathrm{1}}{\mathrm{t}}−\frac{\mathrm{27}}{\mathrm{8}}=\mathrm{0}\Leftrightarrow\mathrm{8t}^{\mathrm{2}} −\mathrm{27t}−\mathrm{8}=\mathrm{0} \\ $$$$\Delta=\mathrm{27}^{\mathrm{2}} +\mathrm{4}.\mathrm{8}^{\mathrm{2}} =\mathrm{729}+\mathrm{256}=\mathrm{985} \\ $$$$\Rightarrow\mathrm{z}^{\mathrm{3}} =\mathrm{t}=\frac{\mathrm{27}\pm\sqrt{\mathrm{985}}}{\mathrm{16}}\Rightarrow\mathrm{z}=\:^{\mathrm{3}} \sqrt{\frac{\mathrm{27}\pm\sqrt{\mathrm{985}}}{\mathrm{16}}} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{z}}=\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{27}\pm\sqrt{\mathrm{985}}}} \\ $$$$\left.\mathrm{i}\right)\mathrm{For}\:\mathrm{z}=\:^{\mathrm{3}} \sqrt{\frac{\mathrm{27}+\sqrt{\mathrm{985}}}{\mathrm{16}}}\Rightarrow\frac{\mathrm{1}}{\mathrm{z}}=\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{27}+\sqrt{\mathrm{985}}}} \\ $$$$\mathrm{we}\:\mathrm{get}\:\:\mathrm{x}_{\mathrm{1}} =\frac{\mathrm{2}}{\mathrm{3}}\mathrm{y}=\frac{\mathrm{2}}{\mathrm{3}}\left(\mathrm{z}−\frac{\mathrm{1}}{\mathrm{z}}\right)= \\ $$$$=\frac{\mathrm{2}}{\mathrm{3}}\left[\:^{\mathrm{3}} \sqrt{\frac{\mathrm{27}+\sqrt{\mathrm{985}}}{\mathrm{16}}}−\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{27}+\sqrt{\mathrm{985}}}}\right] \\ $$$$\left.\mathrm{ii}\right)\mathrm{For}\:\mathrm{z}=\:^{\mathrm{3}} \sqrt{\frac{\mathrm{27}−\sqrt{\mathrm{985}}}{\mathrm{16}}}\Rightarrow\frac{\mathrm{1}}{\mathrm{z}}=\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{27}−\sqrt{\mathrm{985}}}} \\ $$$$\mathrm{z}−\frac{\mathrm{1}}{\mathrm{z}}=\:\left[\mathrm{3}\sqrt{\frac{\mathrm{27}−\sqrt{\mathrm{985}}}{\mathrm{16}}}−\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{27}−\sqrt{\mathrm{985}}}}\right] \\ $$$$=\left[\:^{\mathrm{3}} \sqrt{\frac{−\mathrm{256}}{\mathrm{16}\left(\mathrm{27}+\sqrt{\mathrm{985}}\right.}}−\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}\left(\mathrm{27}+\sqrt{\mathrm{985}}\right)}{−\mathrm{256}}}\right. \\ $$$$=\:^{\mathrm{3}} \sqrt{\frac{−\mathrm{16}}{\mathrm{27}+\sqrt{\mathrm{985}}}}−\:^{\mathrm{3}} \sqrt{\frac{\mathrm{27}+\sqrt{\mathrm{985}}}{−\mathrm{16}}} \\ $$$$=^{\mathrm{3}} \sqrt{\frac{\mathrm{27}+\sqrt{\mathrm{985}}}{\mathrm{16}}}−\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{27}+\sqrt{\mathrm{985}}}} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{x}_{\mathrm{2}} =\frac{\mathrm{2}}{\mathrm{3}}\mathrm{y}=\frac{\mathrm{2}}{\mathrm{3}}\left(\mathrm{z}−\frac{\mathrm{1}}{\mathrm{z}}\right)=\mathrm{x}_{\mathrm{1}} \\ $$$$\mathrm{Thus},\mathrm{the}\:\mathrm{given}\:\mathrm{equation}\:\mathrm{has}\:\mathrm{unique} \\ $$$$\mathrm{solution}\:\boldsymbol{\mathrm{x}}=\frac{\mathrm{2}}{\mathrm{3}}\left[\mathrm{3}\sqrt{\frac{\mathrm{27}+\sqrt{\mathrm{985}}}{\mathrm{16}}}−\:^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{27}+\sqrt{\mathrm{985}}}}\right] \\ $$
Commented by Sarah85 last updated on 17/Aug/20

$$\mathrm{we}'\mathrm{ve}\:\mathrm{got}\:\mathrm{Cardano}'\mathrm{s}\:\mathrm{solution}\:\mathrm{formula}\:\mathrm{and} \\ $$$$\mathrm{for}\:\mathrm{the}\:\mathrm{cases}\:\mathrm{where}\:{D}<\mathrm{0}\:\mathrm{there}'\mathrm{s}\:\mathrm{a}\:\mathrm{trigonometric} \\ $$$$\mathrm{solution}\:\mathrm{formula}.\:\mathrm{no}\:\mathrm{need}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{each}\:\mathrm{single} \\ $$$$\mathrm{case}\:\mathrm{like}\:\mathrm{this}. \\ $$$$\mathrm{and}\:\mathrm{btw}\:\mathrm{a}\:\mathrm{3rd}\:\mathrm{degree}\:\mathrm{polynomial}\:{always}\:\mathrm{has} \\ $$$$\mathrm{three}\:\mathrm{solutions} \\ $$
Commented by 1549442205PVT last updated on 17/Aug/20

$$\mathrm{The}\:\mathrm{above}\:\mathrm{solution}\:\mathrm{only}\:\mathrm{mention}\:\mathrm{to} \\ $$$$\mathrm{real}\:\mathrm{roots}.\mathrm{If}\:\mathrm{you}\:\mathrm{want}\:\mathrm{to}\:\mathrm{find}\:\mathrm{two}\:\mathrm{other} \\ $$$$\mathrm{complex}\:\mathrm{roots}\:\mathrm{then}\:\mathrm{they}\:\mathrm{are}\:\:\mathrm{in}\:\mathrm{the}\:\mathrm{cubic}\: \\ $$$$\mathrm{root}\:\mathrm{of}\:\mathrm{z}^{\mathrm{3}} =\frac{\mathrm{27}\pm\sqrt{\mathrm{985}}}{\mathrm{16}}\:\mathrm{because}\:\mathrm{the}\:\mathrm{cubic}\:\: \\ $$$$\mathrm{root}\:\mathrm{of}\:\mathrm{an}\:\mathrm{arbitrary}\:\mathrm{number}\:\:\mathrm{has}\:\mathrm{always}\: \\ $$$$\mathrm{three}\:\mathrm{different}\:\:\mathrm{values}\:\mathrm{in}\:\mathrm{field}\:\mathrm{of}\: \\ $$$$\mathrm{complex}\:\mathrm{number} \\ $$
