Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 109022 by mathdave last updated on 20/Aug/20

Answered by Dwaipayan Shikari last updated on 20/Aug/20

∫_0 ^∞ ((x+1)/((1+x)^3 ))−(1/((1+x)^3 ))dx −[(1/(1+x))]_0 ^∞ +[(1/(2(1+x)^2 ))]_0 ^∞ =1−(1/2)=(1/2)

$$\int_{\mathrm{0}} ^{\infty} \frac{{x}+\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{\mathrm{3}} }−\frac{\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{\mathrm{3}} }{dx} \\ $$$$−\left[\frac{\mathrm{1}}{\mathrm{1}+{x}}\right]_{\mathrm{0}} ^{\infty} +\left[\frac{\mathrm{1}}{\mathrm{2}\left(\mathrm{1}+{x}\right)^{\mathrm{2}} }\right]_{\mathrm{0}} ^{\infty} =\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Answered by Dwaipayan Shikari last updated on 20/Aug/20

4)∫_0 ^2 (1/(1+x^2 ))+((x^4 +x^2 )/(1+x^2 ))−(x^2 /(1+x^2 )) [tan^(−1) x+(x^3 /3)−x+tan^(−1) x]_0 ^2 =(2/3)+2tan^(−1) 2

$$\left.\mathrm{4}\right)\int_{\mathrm{0}} ^{\mathrm{2}} \frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }+\frac{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }−\frac{{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\left[{tan}^{−\mathrm{1}} {x}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}}−{x}+{tan}^{−\mathrm{1}} {x}\right]_{\mathrm{0}} ^{\mathrm{2}} =\frac{\mathrm{2}}{\mathrm{3}}+\mathrm{2}{tan}^{−\mathrm{1}} \mathrm{2} \\ $$

Answered by mathmax by abdo last updated on 20/Aug/20

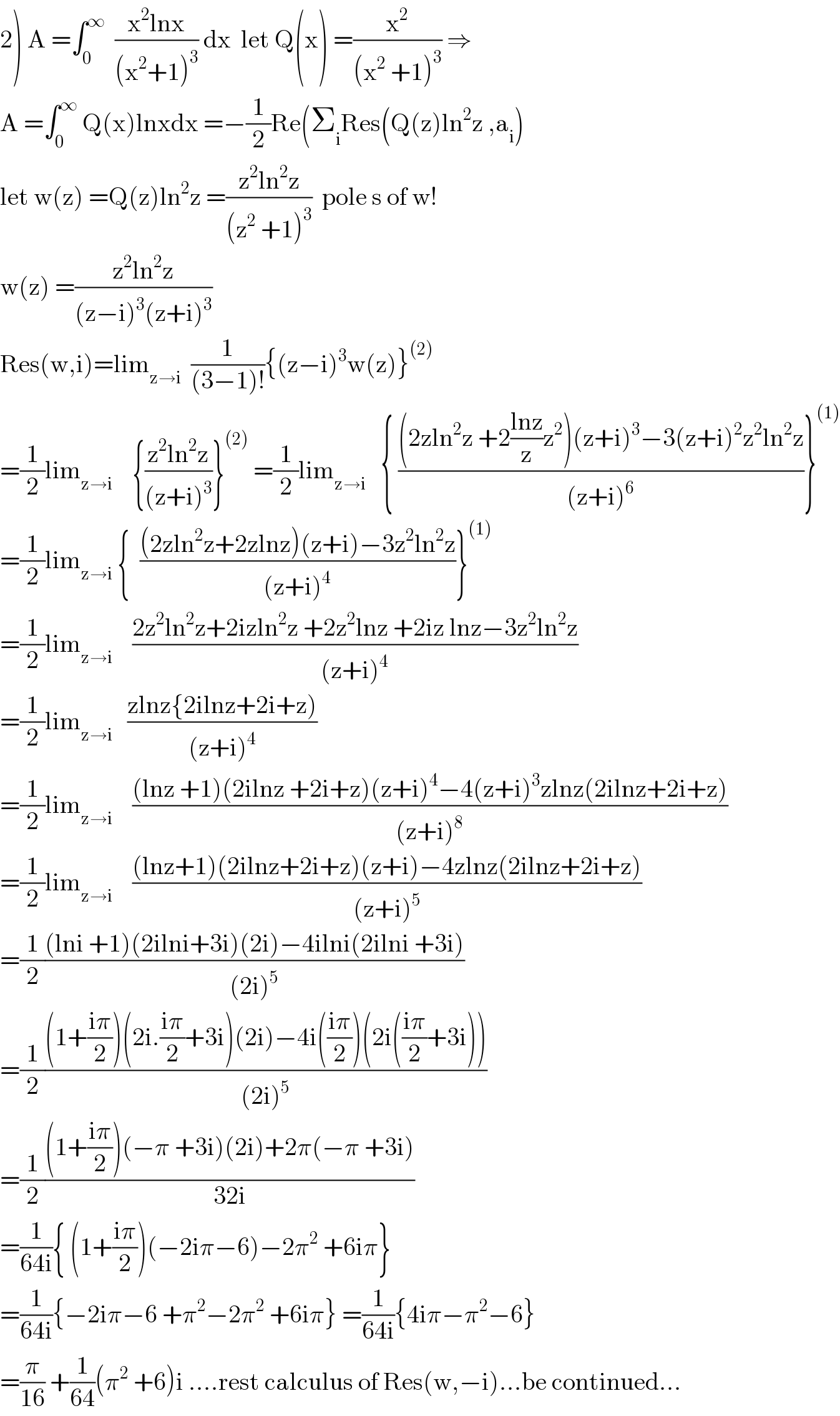
2) A =∫_0 ^∞ ((x^2 lnx)/((x^2 +1)^3 )) dx let Q(x) =(x^2 /((x^2 +1)^3 )) ⇒ A =∫_0 ^∞ Q(x)lnxdx =−(1/2)Re(Σ_i Res(Q(z)ln^2 z ,a_i ) let w(z) =Q(z)ln^2 z =((z^2 ln^2 z)/((z^2 +1)^3 )) pole s of w! w(z) =((z^2 ln^2 z)/((z−i)^3 (z+i)^3 )) Res(w,i)=lim_(z→i) (1/((3−1)!)){(z−i)^3 w(z)}^((2)) =(1/2)lim_(z→i) {((z^2 ln^2 z)/((z+i)^3 ))}^((2)) =(1/2)lim_(z→i) { (((2zln^2 z +2((lnz)/z)z^2 )(z+i)^3 −3(z+i)^2 z^2 ln^2 z)/((z+i)^6 ))}^((1)) =(1/2)lim_(z→i) { (((2zln^2 z+2zlnz)(z+i)−3z^2 ln^2 z)/((z+i)^4 ))}^((1)) =(1/2)lim_(z→i) ((2z^2 ln^2 z+2izln^2 z +2z^2 lnz +2iz lnz−3z^2 ln^2 z)/((z+i)^4 )) =(1/2)lim_(z→i) ((zlnz{2ilnz+2i+z))/((z+i)^4 )) =(1/2)lim_(z→i) (((lnz +1)(2ilnz +2i+z)(z+i)^4 −4(z+i)^3 zlnz(2ilnz+2i+z))/((z+i)^8 )) =(1/2)lim_(z→i) (((lnz+1)(2ilnz+2i+z)(z+i)−4zlnz(2ilnz+2i+z))/((z+i)^5 )) =(1/2)(((lni +1)(2ilni+3i)(2i)−4ilni(2ilni +3i))/((2i)^5 )) =(1/2)(((1+((iπ)/2))(2i.((iπ)/2)+3i)(2i)−4i(((iπ)/2))(2i(((iπ)/2)+3i)))/((2i)^5 )) =(1/2)(((1+((iπ)/2))(−π +3i)(2i)+2π(−π +3i))/(32i)) =(1/(64i)){ (1+((iπ)/2))(−2iπ−6)−2π^2 +6iπ} =(1/(64i)){−2iπ−6 +π^2 −2π^2 +6iπ} =(1/(64i)){4iπ−π^2 −6} =(π/(16)) +(1/(64))(π^2 +6)i ....rest calculus of Res(w,−i)...be continued...

$$\left.\mathrm{2}\right)\:\mathrm{A}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{x}^{\mathrm{2}} \mathrm{lnx}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} }\:\mathrm{dx}\:\:\mathrm{let}\:\mathrm{Q}\left(\mathrm{x}\right)\:=\frac{\mathrm{x}^{\mathrm{2}} }{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} }\:\Rightarrow \\ $$$$\mathrm{A}\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{Q}\left(\mathrm{x}\right)\mathrm{lnxdx}\:=−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{Re}\left(\sum_{\mathrm{i}} \mathrm{Res}\left(\mathrm{Q}\left(\mathrm{z}\right)\mathrm{ln}^{\mathrm{2}} \mathrm{z}\:,\mathrm{a}_{\mathrm{i}} \right)\right. \\ $$$$\mathrm{let}\:\mathrm{w}\left(\mathrm{z}\right)\:=\mathrm{Q}\left(\mathrm{z}\right)\mathrm{ln}^{\mathrm{2}} \mathrm{z}\:=\frac{\mathrm{z}^{\mathrm{2}} \mathrm{ln}^{\mathrm{2}} \mathrm{z}}{\left(\mathrm{z}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} }\:\:\mathrm{pole}\:\mathrm{s}\:\mathrm{of}\:\mathrm{w}! \\ $$$$\mathrm{w}\left(\mathrm{z}\right)\:=\frac{\mathrm{z}^{\mathrm{2}} \mathrm{ln}^{\mathrm{2}} \mathrm{z}}{\left(\mathrm{z}−\mathrm{i}\right)^{\mathrm{3}} \left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{3}} } \\ $$$$\mathrm{Res}\left(\mathrm{w},\mathrm{i}\right)=\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\:\frac{\mathrm{1}}{\left(\mathrm{3}−\mathrm{1}\right)!}\left\{\left(\mathrm{z}−\mathrm{i}\right)^{\mathrm{3}} \mathrm{w}\left(\mathrm{z}\right)\right\}^{\left(\mathrm{2}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\:\:\:\left\{\frac{\mathrm{z}^{\mathrm{2}} \mathrm{ln}^{\mathrm{2}} \mathrm{z}}{\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{3}} }\right\}^{\left(\mathrm{2}\right)} \:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\:\:\left\{\:\frac{\left(\mathrm{2zln}^{\mathrm{2}} \mathrm{z}\:+\mathrm{2}\frac{\mathrm{lnz}}{\mathrm{z}}\mathrm{z}^{\mathrm{2}} \right)\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{3}} −\mathrm{3}\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{2}} \mathrm{z}^{\mathrm{2}} \mathrm{ln}^{\mathrm{2}} \mathrm{z}}{\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{6}} }\right\}^{\left(\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\left\{\:\:\frac{\left(\mathrm{2zln}^{\mathrm{2}} \mathrm{z}+\mathrm{2zlnz}\right)\left(\mathrm{z}+\mathrm{i}\right)−\mathrm{3z}^{\mathrm{2}} \mathrm{ln}^{\mathrm{2}} \mathrm{z}}{\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{4}} }\right\}^{\left(\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\:\:\:\frac{\mathrm{2z}^{\mathrm{2}} \mathrm{ln}^{\mathrm{2}} \mathrm{z}+\mathrm{2izln}^{\mathrm{2}} \mathrm{z}\:+\mathrm{2z}^{\mathrm{2}} \mathrm{lnz}\:+\mathrm{2iz}\:\mathrm{lnz}−\mathrm{3z}^{\mathrm{2}} \mathrm{ln}^{\mathrm{2}} \mathrm{z}}{\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{4}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\:\:\frac{\mathrm{zlnz}\left\{\mathrm{2ilnz}+\mathrm{2i}+\mathrm{z}\right)}{\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{4}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\:\:\:\frac{\left(\mathrm{lnz}\:+\mathrm{1}\right)\left(\mathrm{2ilnz}\:+\mathrm{2i}+\mathrm{z}\right)\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{4}} −\mathrm{4}\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{3}} \mathrm{zlnz}\left(\mathrm{2ilnz}+\mathrm{2i}+\mathrm{z}\right)}{\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{8}} }\:\:\:\: \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{i}} \:\:\:\:\frac{\left(\mathrm{lnz}+\mathrm{1}\right)\left(\mathrm{2ilnz}+\mathrm{2i}+\mathrm{z}\right)\left(\mathrm{z}+\mathrm{i}\right)−\mathrm{4zlnz}\left(\mathrm{2ilnz}+\mathrm{2i}+\mathrm{z}\right)}{\left(\mathrm{z}+\mathrm{i}\right)^{\mathrm{5}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\frac{\left(\mathrm{lni}\:+\mathrm{1}\right)\left(\mathrm{2ilni}+\mathrm{3i}\right)\left(\mathrm{2i}\right)−\mathrm{4ilni}\left(\mathrm{2ilni}\:+\mathrm{3i}\right)}{\left(\mathrm{2i}\right)^{\mathrm{5}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\frac{\left(\mathrm{1}+\frac{\mathrm{i}\pi}{\mathrm{2}}\right)\left(\mathrm{2i}.\frac{\mathrm{i}\pi}{\mathrm{2}}+\mathrm{3i}\right)\left(\mathrm{2i}\right)−\mathrm{4i}\left(\frac{\mathrm{i}\pi}{\mathrm{2}}\right)\left(\mathrm{2i}\left(\frac{\mathrm{i}\pi}{\mathrm{2}}+\mathrm{3i}\right)\right)}{\left(\mathrm{2i}\right)^{\mathrm{5}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\frac{\left(\mathrm{1}+\frac{\mathrm{i}\pi}{\mathrm{2}}\right)\left(−\pi\:+\mathrm{3i}\right)\left(\mathrm{2i}\right)+\mathrm{2}\pi\left(−\pi\:+\mathrm{3i}\right)}{\mathrm{32i}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{64i}}\left\{\:\left(\mathrm{1}+\frac{\mathrm{i}\pi}{\mathrm{2}}\right)\left(−\mathrm{2i}\pi−\mathrm{6}\right)−\mathrm{2}\pi^{\mathrm{2}} \:+\mathrm{6i}\pi\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{64i}}\left\{−\mathrm{2i}\pi−\mathrm{6}\:+\pi^{\mathrm{2}} −\mathrm{2}\pi^{\mathrm{2}} \:+\mathrm{6i}\pi\right\}\:=\frac{\mathrm{1}}{\mathrm{64i}}\left\{\mathrm{4i}\pi−\pi^{\mathrm{2}} −\mathrm{6}\right\} \\ $$$$=\frac{\pi}{\mathrm{16}}\:+\frac{\mathrm{1}}{\mathrm{64}}\left(\pi^{\mathrm{2}} \:+\mathrm{6}\right)\mathrm{i}\:....\mathrm{rest}\:\mathrm{calculus}\:\mathrm{of}\:\mathrm{Res}\left(\mathrm{w},−\mathrm{i}\right)...\mathrm{be}\:\mathrm{continued}... \\ $$

Answered by mathmax by abdo last updated on 20/Aug/20

3) I =∫_0 ^∞ (x/((1+x)^3 )) dx changement 1+x=t give I =∫_1 ^∞ ((t−1)/t^3 )dt =∫_1 ^∞ ((1/t^2 )−(1/t^3 ))dt =[−(1/t)+(1/(2t^2 ))]_1 ^∞ =1−(1/2)=(1/2)

$$\left.\mathrm{3}\right)\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{x}}{\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{3}} }\:\mathrm{dx}\:\mathrm{changement}\:\mathrm{1}+\mathrm{x}=\mathrm{t}\:\mathrm{give} \\ $$$$\mathrm{I}\:=\int_{\mathrm{1}} ^{\infty} \:\frac{\mathrm{t}−\mathrm{1}}{\mathrm{t}^{\mathrm{3}} }\mathrm{dt}\:\:=\int_{\mathrm{1}} ^{\infty} \left(\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{3}} }\right)\mathrm{dt}\:=\left[−\frac{\mathrm{1}}{\mathrm{t}}+\frac{\mathrm{1}}{\mathrm{2t}^{\mathrm{2}} }\right]_{\mathrm{1}} ^{\infty} =\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Answered by mathmax by abdo last updated on 20/Aug/20

4) I =∫_0 ^2 ((1+x^4 )/(1+x^2 ))dx ⇒ I =∫_0 ^2 ((x^2 (x^2 +1)−x^2 +1)/(x^2 +1))dx =∫_0 ^(2 ) x^2 dx −∫_0 ^(2 ) ((x^2 −1)/(x^2 +1))dx =[(x^3 /3)]_0 ^2 −∫_0 ^2 ((x^2 +1−2)/(x^2 +1))dx =(8/3)−2 +2 [arctanx]_0 ^2 =(2/3) +2arctan(2)

$$\left.\mathrm{4}\right)\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\mathrm{2}} \:\frac{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\Rightarrow\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\mathrm{2}} \:\frac{\mathrm{x}^{\mathrm{2}} \left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)−\mathrm{x}^{\mathrm{2}} +\mathrm{1}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{2}\:} \:\mathrm{x}^{\mathrm{2}} \mathrm{dx}\:−\int_{\mathrm{0}} ^{\mathrm{2}\:} \:\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx}\:=\left[\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}\right]_{\mathrm{0}} ^{\mathrm{2}} −\int_{\mathrm{0}} ^{\mathrm{2}} \:\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{1}−\mathrm{2}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx} \\ $$$$=\frac{\mathrm{8}}{\mathrm{3}}−\mathrm{2}\:+\mathrm{2}\:\left[\mathrm{arctanx}\right]_{\mathrm{0}} ^{\mathrm{2}} \:=\frac{\mathrm{2}}{\mathrm{3}}\:+\mathrm{2arctan}\left(\mathrm{2}\right) \\ $$

Answered by mathmax by abdo last updated on 20/Aug/20

6) I =∫_0 ^1 ((xdx)/(1−x^2 )) ⇒ I =(1/2)∫_0 ^1 ((1/(1−x))−(1/(1+x)))dx =lim_(ξ→1) (1/2)[ln∣((1−x)/(1+x))∣]_0 ^ξ =+∞ this integral is divergent...! ∫ (√(tanx))dx is solved see the platform

$$\left.\mathrm{6}\right)\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{xdx}}{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:\:\:\Rightarrow\:\mathrm{I}\:=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{1}−\mathrm{x}}−\frac{\mathrm{1}}{\mathrm{1}+\mathrm{x}}\right)\mathrm{dx} \\ $$$$=\mathrm{lim}_{\xi\rightarrow\mathrm{1}} \frac{\mathrm{1}}{\mathrm{2}}\left[\mathrm{ln}\mid\frac{\mathrm{1}−\mathrm{x}}{\mathrm{1}+\mathrm{x}}\mid\right]_{\mathrm{0}} ^{\xi} \:=+\infty\:\mathrm{this}\:\mathrm{integral}\:\mathrm{is}\:\mathrm{divergent}...! \\ $$$$\int\:\sqrt{\mathrm{tanx}}\mathrm{dx}\:\mathrm{is}\:\mathrm{solved}\:\mathrm{see}\:\mathrm{the}\:\mathrm{platform} \\ $$

Answered by Dwaipayan Shikari last updated on 20/Aug/20

−(1/2)∫_0 ^1 ((−2x)/((1−x^2 )))dx=−(1/2)[log(1−x^2 )]_0 ^1 →∞

$$−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{−\mathrm{2}{x}}{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{dx}=−\frac{\mathrm{1}}{\mathrm{2}}\left[{log}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)\right]_{\mathrm{0}} ^{\mathrm{1}} \rightarrow\infty \\ $$

Answered by mathmax by abdo last updated on 20/Aug/20

1) A =∫_0 ^1 ln(((4+3sinx)/(4+3cosx)))dx ⇒A =∫_0 ^1 ln(4+3sinx)dx −∫_0 ^1 ln(4+3cosx)dx =2ln(2)+∫_0 ^1 ln(1+(3/4)sinx)dx−2ln(2) −∫_0 ^1 ln(1+(3/4)cosx)dx =∫_0 ^1 ln(1+(3/4)sinx)−∫_0 ^1 ln(1+(3/4)cosx) let f(a) =∫_0 ^1 ln(1+asinx) with0<a<1 ⇒ f^′ (a) =∫_0 ^1 ((sinx)/(1+asinx))dx =(1/a)∫_0 ^1 ((1+asinx−1)/(1+asinx))dx =(1/a)−(1/a)∫_0 ^1 (dx/(1+asinx)) we have ∫_0 ^1 (dx/(1+asinx)) =_(tan((x/2))=t) ∫_0 ^(tan((1/2))) ((2dt)/((1+t^2 )(1+a((2t)/(1+t^2 ))))) ∫_0 ^(tan((1/2))) ((2dt)/(1+t^2 +2at)) =2 ∫_0 ^(tan((1/2))) (dt/(t^2 +2at +1)) =2∫_0 ^(tan((1/2))) (dt/(t^2 +2at +a^2 +1−a^2 )) =2∫_0 ^(tan((1/2))) (dt/((t+a)^(2 ) +1−a^2 )) =_(t+a =(√(1−a^2 ))u) 2 ∫_(a/(√(1−a^2 ))) ^((tan((1/2))+a)/(√(1−a^2 ))) (((√(1−a^2 ))du)/((1−a^2 )(1+u^2 ))) =(2/(√(1−a^2 ))) [arctanu]_(a/(√(1−a^2 ))) ^((a+tan((1/2)))/(√(1−a^2 ))) =(2/(√(1−a^2 ))){ arctan(((a+tan((1/2)))/(√(1−a^2 )))) −arctan((a/(√(1−a^2 ))))} ⇒ f^′ (a) =(1/a)−(2/(a(√(1−a^2 ))))(arctan(((a+tan((1/2)))/(√(1−a^2 ))))−arctan((a/(√(1−a^2 ))))) ⇒ f(a) =lna−2 ∫_1 ^a (1/(t(√(1−t^2 ))))(arctan(((t+tg((1/2)))/(√(1−t^2 ))))−arctan((t/(√(1−t^2 )))))dt +C c =f(1) ....be continued....

$$\left.\mathrm{1}\right)\:\mathrm{A}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{ln}\left(\frac{\mathrm{4}+\mathrm{3sinx}}{\mathrm{4}+\mathrm{3cosx}}\right)\mathrm{dx}\:\Rightarrow\mathrm{A}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{4}+\mathrm{3sinx}\right)\mathrm{dx} \\ $$$$−\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{4}+\mathrm{3cosx}\right)\mathrm{dx}\:=\mathrm{2ln}\left(\mathrm{2}\right)+\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{1}+\frac{\mathrm{3}}{\mathrm{4}}\mathrm{sinx}\right)\mathrm{dx}−\mathrm{2ln}\left(\mathrm{2}\right) \\ $$$$−\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{1}+\frac{\mathrm{3}}{\mathrm{4}}\mathrm{cosx}\right)\mathrm{dx}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{1}+\frac{\mathrm{3}}{\mathrm{4}}\mathrm{sinx}\right)−\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{1}+\frac{\mathrm{3}}{\mathrm{4}}\mathrm{cosx}\right) \\ $$$$\mathrm{let}\:\mathrm{f}\left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{1}+\mathrm{asinx}\right)\:\:\mathrm{with0}<\mathrm{a}<\mathrm{1}\:\Rightarrow \\ $$$$\mathrm{f}^{'} \left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{sinx}}{\mathrm{1}+\mathrm{asinx}}\mathrm{dx}\:=\frac{\mathrm{1}}{\mathrm{a}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{1}+\mathrm{asinx}−\mathrm{1}}{\mathrm{1}+\mathrm{asinx}}\mathrm{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{a}}−\frac{\mathrm{1}}{\mathrm{a}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{dx}}{\mathrm{1}+\mathrm{asinx}}\:\mathrm{we}\:\mathrm{have}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{dx}}{\mathrm{1}+\mathrm{asinx}}\:=_{\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)=\mathrm{t}} \:\int_{\mathrm{0}} ^{\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)} \:\frac{\mathrm{2dt}}{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{a}\frac{\mathrm{2t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\right)} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)} \:\frac{\mathrm{2dt}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} +\mathrm{2at}}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)} \:\frac{\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} \:+\mathrm{2at}\:+\mathrm{1}}\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)} \:\frac{\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} \:+\mathrm{2at}\:+\mathrm{a}^{\mathrm{2}} \:+\mathrm{1}−\mathrm{a}^{\mathrm{2}} } \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)} \:\frac{\mathrm{dt}}{\left(\mathrm{t}+\mathrm{a}\right)^{\mathrm{2}\:} \:+\mathrm{1}−\mathrm{a}^{\mathrm{2}} }\:=_{\mathrm{t}+\mathrm{a}\:=\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }\mathrm{u}} \:\:\mathrm{2}\:\int_{\frac{\mathrm{a}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}} ^{\frac{\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)+\mathrm{a}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}} \:\:\frac{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }\mathrm{du}}{\left(\mathrm{1}−\mathrm{a}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\:\left[\mathrm{arctanu}\right]_{\frac{\mathrm{a}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}} ^{\frac{\mathrm{a}+\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}} \:=\frac{\mathrm{2}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\left\{\:\mathrm{arctan}\left(\frac{\mathrm{a}+\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\right)\right. \\ $$$$\left.−\mathrm{arctan}\left(\frac{\mathrm{a}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\right)\right\}\:\Rightarrow \\ $$$$\mathrm{f}^{'} \left(\mathrm{a}\right)\:=\frac{\mathrm{1}}{\mathrm{a}}−\frac{\mathrm{2}}{\mathrm{a}\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\left(\mathrm{arctan}\left(\frac{\mathrm{a}+\mathrm{tan}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\right)−\mathrm{arctan}\left(\frac{\mathrm{a}}{\sqrt{\mathrm{1}−\mathrm{a}^{\mathrm{2}} }}\right)\right)\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{a}\right)\:=\mathrm{lna}−\mathrm{2}\:\int_{\mathrm{1}} ^{\mathrm{a}} \:\:\frac{\mathrm{1}}{\mathrm{t}\sqrt{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }}\left(\mathrm{arctan}\left(\frac{\mathrm{t}+\mathrm{tg}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\sqrt{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }}\right)−\mathrm{arctan}\left(\frac{\mathrm{t}}{\sqrt{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }}\right)\right)\mathrm{dt}\:+\mathrm{C} \\ $$$$\mathrm{c}\:=\mathrm{f}\left(\mathrm{1}\right)\:....\mathrm{be}\:\mathrm{continued}.... \\ $$$$ \\ $$

Commented by mathmax by abdo last updated on 20/Aug/20

let determine f(a)=∫_0 ^1 ln(1+asinx)dx at form of serie (o<a<1 we have (d/du)ln(1+u) =(1/(1+u)) =Σ_(n=0) ^∞ (−1)^n u^(n ) for ∣u∣<1⇒ ln(1+u) =Σ_(n=0) ^∞ (((−1)^n u^(n+1) )/(n+1)) +c (c=0)=Σ_(n=1) ^∞ (((−1)^(n−1) u^n )/n) ⇒ f(a) =∫_0 ^1 Σ_(n=1) ^∞ (((−1)^(n−1) )/n)(asinx)^n dx =Σ_(n=1) ^∞ (((−1)^(n−1) )/n) a^n ∫_0 ^1 sin^n xdx =Σ_(n=1) ^∞ (((−1)^(n−1) )/n)a^n w_n with w_n =∫_0 ^(1 ) sin^n xdx (wallis integral on [0,1].... w_n canbe calculsted by recurrence...

$$\mathrm{let}\:\mathrm{determine}\:\mathrm{f}\left(\mathrm{a}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left(\mathrm{1}+\mathrm{asinx}\right)\mathrm{dx}\:\:\mathrm{at}\:\mathrm{form}\:\mathrm{of}\:\mathrm{serie}\:\left(\mathrm{o}<\mathrm{a}<\mathrm{1}\right. \\ $$$$\mathrm{we}\:\mathrm{have}\:\frac{\mathrm{d}}{\mathrm{du}}\mathrm{ln}\left(\mathrm{1}+\mathrm{u}\right)\:=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{u}}\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{u}^{\mathrm{n}\:} \:\mathrm{for}\:\mid\mathrm{u}\mid<\mathrm{1}\Rightarrow \\ $$$$\mathrm{ln}\left(\mathrm{1}+\mathrm{u}\right)\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} \mathrm{u}^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}+\mathrm{1}}\:+\mathrm{c}\:\:\left(\mathrm{c}=\mathrm{0}\right)=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} \mathrm{u}^{\mathrm{n}} }{\mathrm{n}}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}}\left(\mathrm{asinx}\right)^{\mathrm{n}} \mathrm{dx} \\ $$$$=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}}\:\mathrm{a}^{\mathrm{n}} \:\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{sin}^{\mathrm{n}} \mathrm{xdx}\:=\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}−\mathrm{1}} }{\mathrm{n}}\mathrm{a}^{\mathrm{n}} \:\mathrm{w}_{\mathrm{n}} \\ $$$$\mathrm{with}\:\mathrm{w}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}\:} \mathrm{sin}^{\mathrm{n}} \mathrm{xdx}\:\:\left(\mathrm{wallis}\:\mathrm{integral}\:\mathrm{on}\:\left[\mathrm{0},\mathrm{1}\right]....\right. \\ $$$$\mathrm{w}_{\mathrm{n}} \mathrm{canbe}\:\mathrm{calculsted}\:\mathrm{by}\:\mathrm{recurrence}... \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com