Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 113004 by malwan last updated on 10/Sep/20

prove that _0 ∫^( ∞) cos(x^2 )dx = _0 ∫^( ∞) sin(x^2 )dx =((√π)/(2(√2)))

$${prove}\:{that} \\ $$$$\:_{\mathrm{0}} \int^{\:\infty} \:{cos}\left({x}^{\mathrm{2}} \right){dx}\:=\:\:_{\mathrm{0}} \int^{\:\infty} {sin}\left({x}^{\mathrm{2}} \right){dx}\:=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$

Answered by mathdave last updated on 10/Sep/20

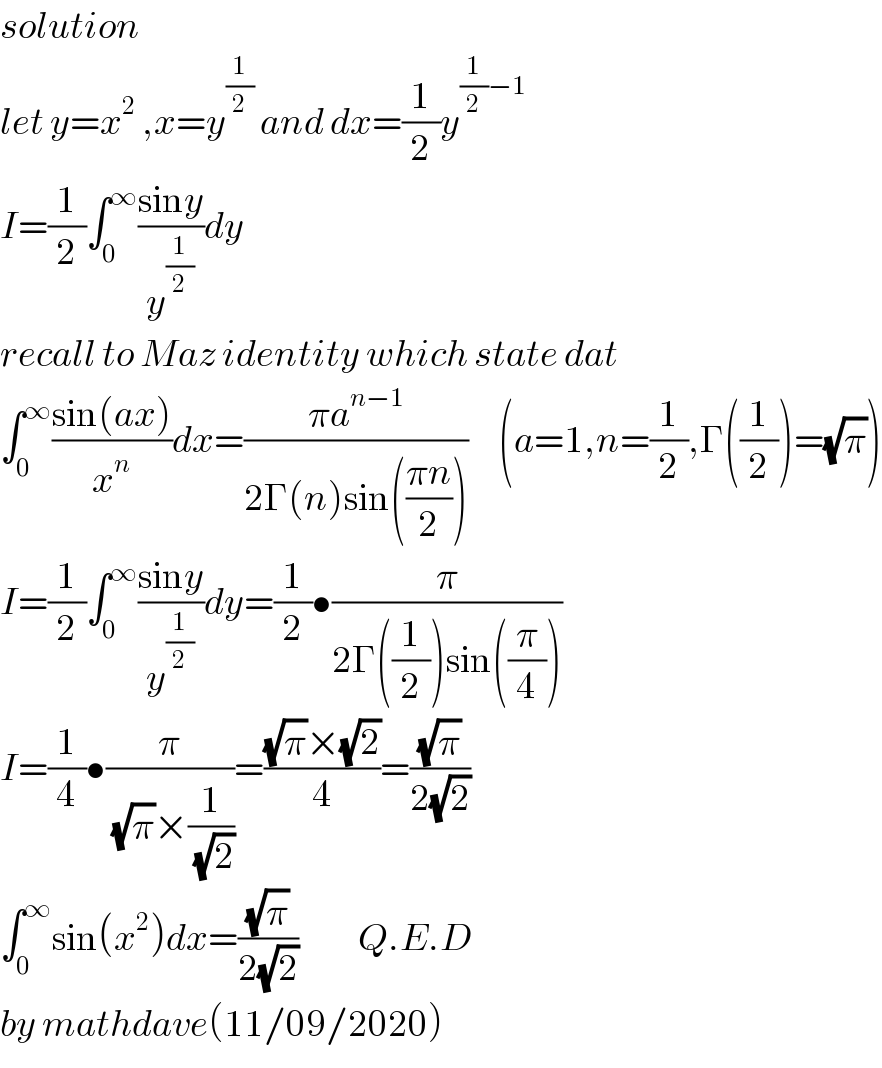
solution let y=x^2 ,x=y^(1/2) and dx=(1/2)y^((1/2)−1) I=(1/2)∫_0 ^∞ ((siny)/y^(1/2) )dy recall to Maz identity which state dat ∫_0 ^∞ ((sin(ax))/x^n )dx=((πa^(n−1) )/(2Γ(n)sin(((πn)/2)))) (a=1,n=(1/2),Γ((1/2))=(√π)) I=(1/2)∫_0 ^∞ ((siny)/y^(1/2) )dy=(1/2)•(π/(2Γ((1/2))sin((π/4)))) I=(1/4)•(π/((√π)×(1/(√2))))=(((√π)×(√2))/4)=((√π)/(2(√2))) ∫_0 ^∞ sin(x^2 )dx=((√π)/(2(√2))) Q.E.D by mathdave(11/09/2020)

$${solution}\: \\ $$$${let}\:{y}={x}^{\mathrm{2}} \:,{x}={y}^{\frac{\mathrm{1}}{\mathrm{2}}} \:{and}\:{dx}=\frac{\mathrm{1}}{\mathrm{2}}{y}^{\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}{y}}{{y}^{\frac{\mathrm{1}}{\mathrm{2}}} }{dy} \\ $$$${recall}\:{to}\:{Maz}\:{identity}\:{which}\:{state}\:{dat} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\left({ax}\right)}{{x}^{{n}} }{dx}=\frac{\pi{a}^{{n}−\mathrm{1}} }{\mathrm{2}\Gamma\left({n}\right)\mathrm{sin}\left(\frac{\pi{n}}{\mathrm{2}}\right)}\:\:\:\:\:\left({a}=\mathrm{1},{n}=\frac{\mathrm{1}}{\mathrm{2}},\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=\sqrt{\pi}\right) \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}{y}}{{y}^{\frac{\mathrm{1}}{\mathrm{2}}} }{dy}=\frac{\mathrm{1}}{\mathrm{2}}\bullet\frac{\pi}{\mathrm{2}\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\mathrm{sin}\left(\frac{\pi}{\mathrm{4}}\right)} \\ $$$${I}=\frac{\mathrm{1}}{\mathrm{4}}\bullet\frac{\pi}{\sqrt{\pi}×\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}}=\frac{\sqrt{\pi}×\sqrt{\mathrm{2}}}{\mathrm{4}}=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \mathrm{sin}\left({x}^{\mathrm{2}} \right){dx}=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\:\:\:\:\:\:\:\:\:{Q}.{E}.{D} \\ $$$${by}\:{mathdave}\left(\mathrm{11}/\mathrm{09}/\mathrm{2020}\right) \\ $$

Commented by malwan last updated on 11/Sep/20

thank you so much

$${thank}\:{you}\:{so}\:{much} \\ $$

Commented by Tawa11 last updated on 06/Sep/21

great sir

$$\mathrm{great}\:\mathrm{sir} \\ $$

Answered by mathmax by abdo last updated on 10/Sep/20

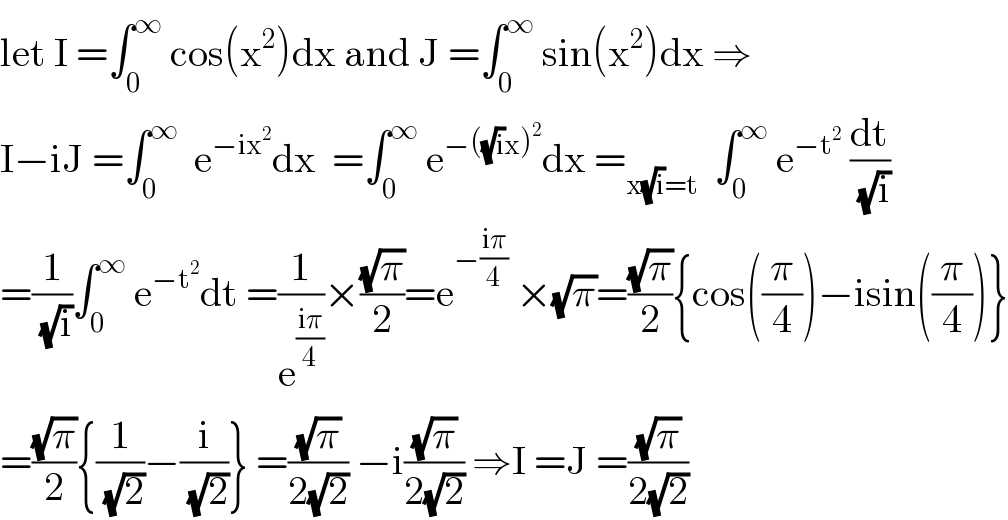
let I =∫_0 ^∞ cos(x^2 )dx and J =∫_0 ^∞ sin(x^2 )dx ⇒ I−iJ =∫_0 ^∞ e^(−ix^2 ) dx =∫_0 ^∞ e^(−((√i)x)^2 ) dx =_(x(√i)=t) ∫_0 ^∞ e^(−t^2 ) (dt/(√i)) =(1/(√i))∫_0 ^∞ e^(−t^2 ) dt =(1/e^((iπ)/4) )×((√π)/2)=e^(−((iπ)/4)) ×(√π)=((√π)/2){cos((π/4))−isin((π/4))} =((√π)/2){(1/(√2))−(i/(√2))} =((√π)/(2(√2))) −i((√π)/(2(√2))) ⇒I =J =((√π)/(2(√2)))

$$\mathrm{let}\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{cos}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{dx}\:\mathrm{and}\:\mathrm{J}\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{sin}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{I}−\mathrm{iJ}\:=\int_{\mathrm{0}} ^{\infty} \:\:\mathrm{e}^{−\mathrm{ix}^{\mathrm{2}} } \mathrm{dx}\:\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\left(\sqrt{\mathrm{i}}\mathrm{x}\right)^{\mathrm{2}} } \mathrm{dx}\:=_{\mathrm{x}\sqrt{\mathrm{i}}=\mathrm{t}} \:\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \:\frac{\mathrm{dt}}{\sqrt{\mathrm{i}}} \\ $$$$=\frac{\mathrm{1}}{\sqrt{\mathrm{i}}}\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{dt}\:=\frac{\mathrm{1}}{\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} }×\frac{\sqrt{\pi}}{\mathrm{2}}=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \:×\sqrt{\pi}=\frac{\sqrt{\pi}}{\mathrm{2}}\left\{\mathrm{cos}\left(\frac{\pi}{\mathrm{4}}\right)−\mathrm{isin}\left(\frac{\pi}{\mathrm{4}}\right)\right\} \\ $$$$=\frac{\sqrt{\pi}}{\mathrm{2}}\left\{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}−\frac{\mathrm{i}}{\sqrt{\mathrm{2}}}\right\}\:=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{\mathrm{2}}}\:−\mathrm{i}\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\Rightarrow\mathrm{I}\:=\mathrm{J}\:=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$

Commented by malwan last updated on 11/Sep/20

great Sir thank you

$${great}\:{Sir} \\ $$$${thank}\:{you} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com