Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 116503 by bemath last updated on 04/Oct/20

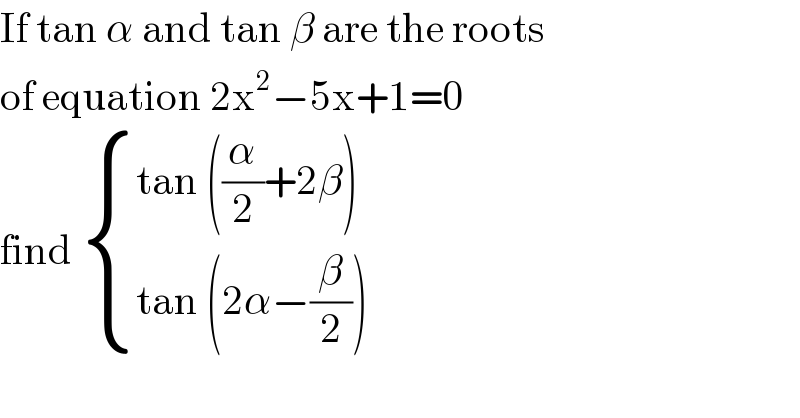
If tan α and tan β are the roots of equation 2x^2 −5x+1=0 find { ((tan ((α/2)+2β))),((tan (2α−(β/2)))) :}

$$\mathrm{If}\:\mathrm{tan}\:\alpha\:\mathrm{and}\:\mathrm{tan}\:\beta\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\: \\ $$$$\mathrm{of}\:\mathrm{equation}\:\mathrm{2x}^{\mathrm{2}} −\mathrm{5x}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{find}\:\begin{cases}{\mathrm{tan}\:\left(\frac{\alpha}{\mathrm{2}}+\mathrm{2}\beta\right)}\\{\mathrm{tan}\:\left(\mathrm{2}\alpha−\frac{\beta}{\mathrm{2}}\right)}\end{cases} \\ $$

Answered by bobhans last updated on 04/Oct/20

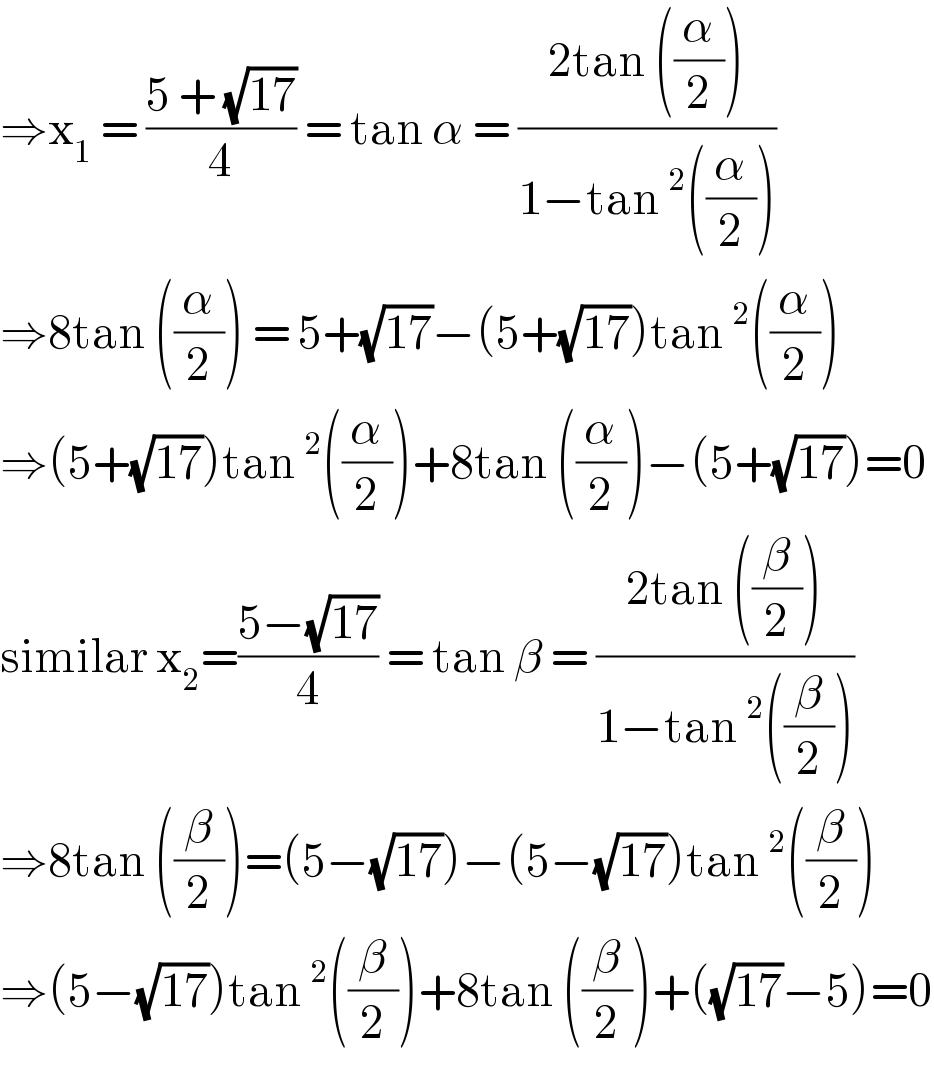
⇒x_1 = ((5 + (√(17)))/4) = tan α = ((2tan ((α/2)))/(1−tan^2 ((α/2)))) ⇒8tan ((α/2)) = 5+(√(17))−(5+(√(17)))tan^2 ((α/2)) ⇒(5+(√(17)))tan^2 ((α/2))+8tan ((α/2))−(5+(√(17)))=0 similar x_2 =((5−(√(17)))/4) = tan β = ((2tan ((β/2)))/(1−tan^2 ((β/2)))) ⇒8tan ((β/2))=(5−(√(17)))−(5−(√(17)))tan^2 ((β/2)) ⇒(5−(√(17)))tan^2 ((β/2))+8tan ((β/2))+((√(17))−5)=0

$$\Rightarrow\mathrm{x}_{\mathrm{1}} \:=\:\frac{\mathrm{5}\:+\:\sqrt{\mathrm{17}}}{\mathrm{4}}\:=\:\mathrm{tan}\:\alpha\:=\:\frac{\mathrm{2tan}\:\left(\frac{\alpha}{\mathrm{2}}\right)}{\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\alpha}{\mathrm{2}}\right)} \\ $$$$\Rightarrow\mathrm{8tan}\:\left(\frac{\alpha}{\mathrm{2}}\right)\:=\:\mathrm{5}+\sqrt{\mathrm{17}}−\left(\mathrm{5}+\sqrt{\mathrm{17}}\right)\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\alpha}{\mathrm{2}}\right) \\ $$$$\Rightarrow\left(\mathrm{5}+\sqrt{\mathrm{17}}\right)\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\alpha}{\mathrm{2}}\right)+\mathrm{8tan}\:\left(\frac{\alpha}{\mathrm{2}}\right)−\left(\mathrm{5}+\sqrt{\mathrm{17}}\right)=\mathrm{0} \\ $$$$\mathrm{similar}\:\mathrm{x}_{\mathrm{2}} =\frac{\mathrm{5}−\sqrt{\mathrm{17}}}{\mathrm{4}}\:=\:\mathrm{tan}\:\beta\:=\:\frac{\mathrm{2tan}\:\left(\frac{\beta}{\mathrm{2}}\right)}{\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\beta}{\mathrm{2}}\right)} \\ $$$$\Rightarrow\mathrm{8tan}\:\left(\frac{\beta}{\mathrm{2}}\right)=\left(\mathrm{5}−\sqrt{\mathrm{17}}\right)−\left(\mathrm{5}−\sqrt{\mathrm{17}}\right)\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\beta}{\mathrm{2}}\right) \\ $$$$\Rightarrow\left(\mathrm{5}−\sqrt{\mathrm{17}}\right)\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\beta}{\mathrm{2}}\right)+\mathrm{8tan}\:\left(\frac{\beta}{\mathrm{2}}\right)+\left(\sqrt{\mathrm{17}}−\mathrm{5}\right)=\mathrm{0} \\ $$

Answered by TANMAY PANACEA last updated on 04/Oct/20

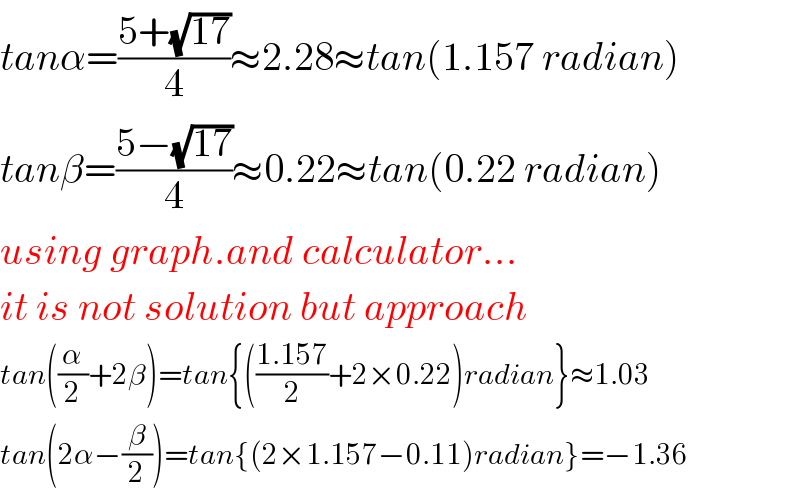
tanα=((5+(√(17)))/4)≈2.28≈tan(1.157 radian) tanβ=((5−(√(17)))/4)≈0.22≈tan(0.22 radian) using graph.and calculator... it is not solution but approach tan((α/2)+2β)=tan{(((1.157)/2)+2×0.22)radian}≈1.03 tan(2α−(β/2))=tan{(2×1.157−0.11)radian}=−1.36

$${tan}\alpha=\frac{\mathrm{5}+\sqrt{\mathrm{17}}}{\mathrm{4}}\approx\mathrm{2}.\mathrm{28}\approx{tan}\left(\mathrm{1}.\mathrm{157}\:{radian}\right) \\ $$$${tan}\beta=\frac{\mathrm{5}−\sqrt{\mathrm{17}}}{\mathrm{4}}\approx\mathrm{0}.\mathrm{22}\approx{tan}\left(\mathrm{0}.\mathrm{22}\:{radian}\right) \\ $$$${using}\:{graph}.{and}\:{calculator}... \\ $$$${it}\:{is}\:{not}\:{solution}\:{but}\:{approach} \\ $$$${tan}\left(\frac{\alpha}{\mathrm{2}}+\mathrm{2}\beta\right)={tan}\left\{\left(\frac{\mathrm{1}.\mathrm{157}}{\mathrm{2}}+\mathrm{2}×\mathrm{0}.\mathrm{22}\right){radian}\right\}\approx\mathrm{1}.\mathrm{03} \\ $$$${tan}\left(\mathrm{2}\alpha−\frac{\beta}{\mathrm{2}}\right)={tan}\left\{\left(\mathrm{2}×\mathrm{1}.\mathrm{157}−\mathrm{0}.\mathrm{11}\right){radian}\right\}=−\mathrm{1}.\mathrm{36} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com