
Question Number 118435 by bramlexs22 last updated on 17/Oct/20
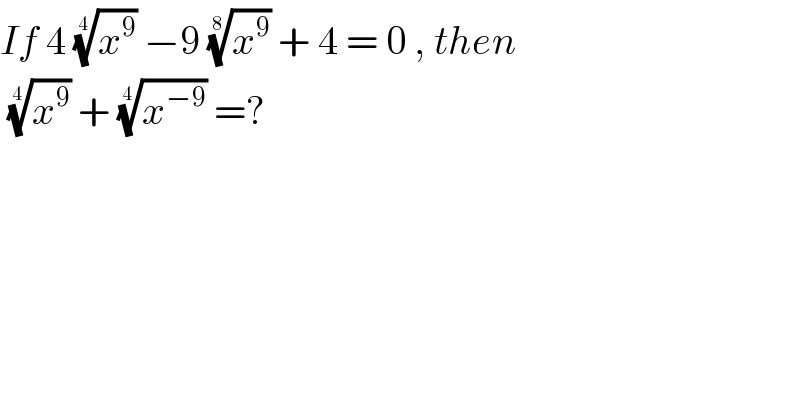
$${If}\:\mathrm{4}\:\sqrt[{\mathrm{4}\:}]{{x}^{\mathrm{9}} }\:−\mathrm{9}\:\sqrt[{\mathrm{8}\:}]{{x}^{\mathrm{9}} }\:+\:\mathrm{4}\:=\:\mathrm{0}\:,\:{then}\: \\ $$$$\:\sqrt[{\mathrm{4}\:}]{{x}^{\mathrm{9}} }\:+\:\sqrt[{\mathrm{4}\:}]{{x}^{−\mathrm{9}} }\:=? \\ $$
Answered by benjo_mathlover last updated on 18/Oct/20
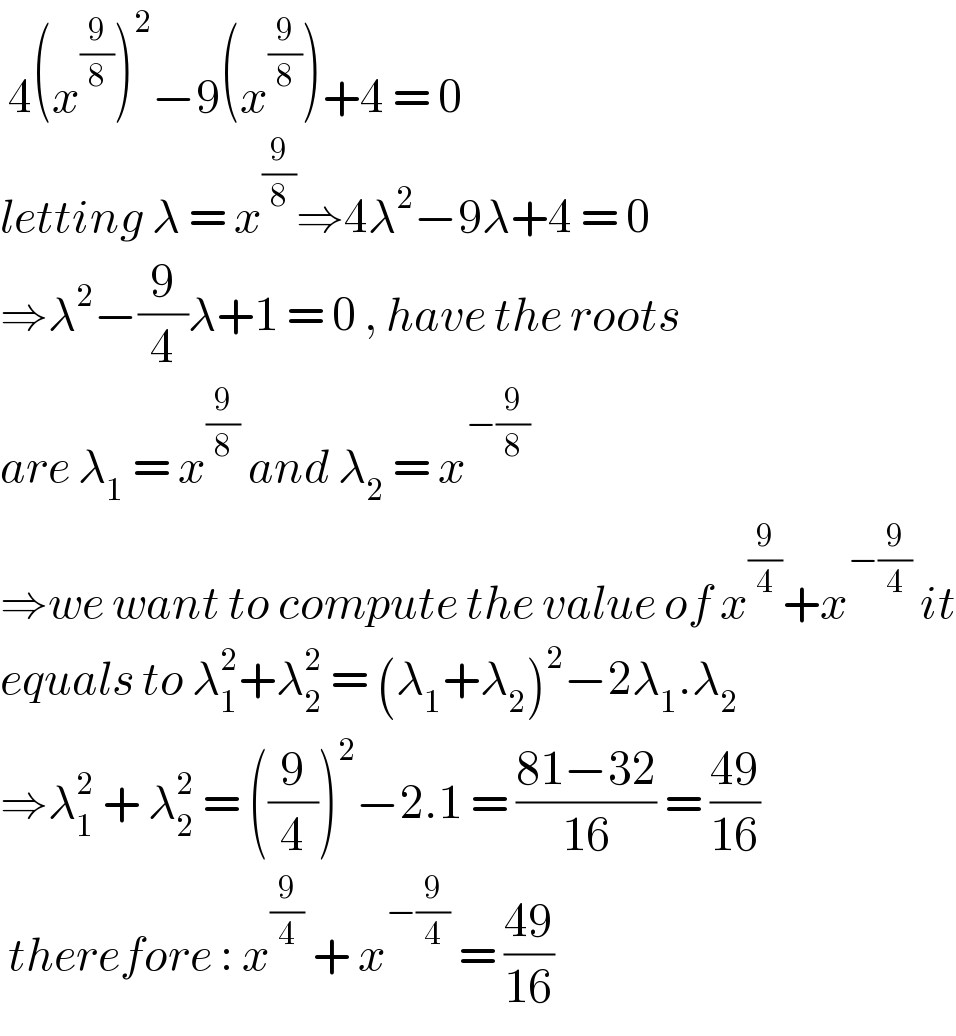
$$\:\mathrm{4}\left({x}^{\frac{\mathrm{9}}{\mathrm{8}}} \right)^{\mathrm{2}} −\mathrm{9}\left({x}^{\frac{\mathrm{9}}{\mathrm{8}}} \right)+\mathrm{4}\:=\:\mathrm{0} \\ $$$${letting}\:\lambda\:=\:{x}^{\frac{\mathrm{9}}{\mathrm{8}}} \Rightarrow\mathrm{4}\lambda^{\mathrm{2}} −\mathrm{9}\lambda+\mathrm{4}\:=\:\mathrm{0} \\ $$$$\Rightarrow\lambda^{\mathrm{2}} −\frac{\mathrm{9}}{\mathrm{4}}\lambda+\mathrm{1}\:=\:\mathrm{0}\:,\:{have}\:{the}\:{roots} \\ $$$${are}\:\lambda_{\mathrm{1}} \:=\:{x}^{\frac{\mathrm{9}}{\mathrm{8}}} \:{and}\:\lambda_{\mathrm{2}} \:=\:{x}^{−\frac{\mathrm{9}}{\mathrm{8}}} \\ $$$$\Rightarrow{we}\:{want}\:{to}\:{compute}\:{the}\:{value}\:{of}\:{x}^{\frac{\mathrm{9}}{\mathrm{4}}} +{x}^{−\frac{\mathrm{9}}{\mathrm{4}}} \:{it} \\ $$$${equals}\:{to}\:\lambda_{\mathrm{1}} ^{\mathrm{2}} +\lambda_{\mathrm{2}} ^{\mathrm{2}} \:=\:\left(\lambda_{\mathrm{1}} +\lambda_{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{2}\lambda_{\mathrm{1}} .\lambda_{\mathrm{2}} \\ $$$$\Rightarrow\lambda_{\mathrm{1}} ^{\mathrm{2}} \:+\:\lambda_{\mathrm{2}} ^{\mathrm{2}} \:=\:\left(\frac{\mathrm{9}}{\mathrm{4}}\right)^{\mathrm{2}} −\mathrm{2}.\mathrm{1}\:=\:\frac{\mathrm{81}−\mathrm{32}}{\mathrm{16}}\:=\:\frac{\mathrm{49}}{\mathrm{16}} \\ $$$$\:{therefore}\::\:{x}^{\frac{\mathrm{9}}{\mathrm{4}}} \:+\:{x}^{−\frac{\mathrm{9}}{\mathrm{4}}} \:=\:\frac{\mathrm{49}}{\mathrm{16}} \\ $$
Commented by MJS_new last updated on 17/Oct/20

$$\mathrm{answer}\:\mathrm{is}\:\frac{\mathrm{49}}{\mathrm{16}} \\ $$
Commented by bramlexs22 last updated on 17/Oct/20

$${how}\:{sir}? \\ $$
Commented by MJS_new last updated on 17/Oct/20
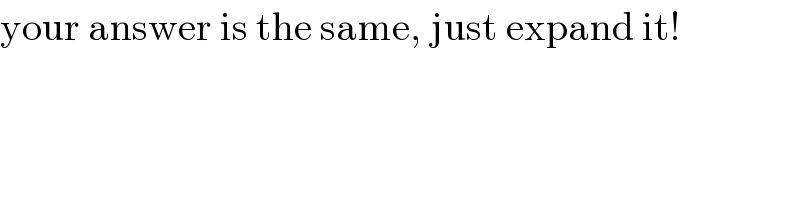
$$\mathrm{your}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{the}\:\mathrm{same},\:\mathrm{just}\:\mathrm{expand}\:\mathrm{it}! \\ $$
Answered by MJS_new last updated on 17/Oct/20

$$\mathrm{we}\:\mathrm{don}'\mathrm{t}\:\mathrm{have}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{for}\:{x} \\ $$$$\mathrm{let}\:{t}={x}^{\mathrm{9}/\mathrm{8}} \\ $$$$\Rightarrow\:\mathrm{the}\:\mathrm{problem}\:\mathrm{turns}\:\mathrm{to} \\ $$$$\mathrm{if}\:\mathrm{4}{t}^{\mathrm{2}} −\mathrm{9}{t}+\mathrm{4}=\mathrm{0}\:\mathrm{then}\:{t}^{\mathrm{2}} +{t}^{−\mathrm{2}} =? \\ $$$$\mathrm{4}{t}^{\mathrm{2}} −\mathrm{9}{t}+\mathrm{4}=\mathrm{0} \\ $$$${t}^{\mathrm{2}} −\frac{\mathrm{9}}{\mathrm{4}}{t}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{the}\:\mathrm{solutions}\:\mathrm{of}\:{t}^{\mathrm{2}} +{pt}+\mathrm{1}\:\mathrm{are} \\ $$$${t}_{\mathrm{1}} =\frac{−{p}−\sqrt{{p}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}}\:\mathrm{and}\:{t}_{\mathrm{2}} ={t}_{\mathrm{1}} ^{−\mathrm{1}} \\ $$$$\Rightarrow\:{t}^{\mathrm{2}} +{t}^{−\mathrm{2}} ={t}_{\mathrm{1}} ^{\mathrm{2}} +{t}_{\mathrm{2}} ^{\mathrm{2}} \\ $$$${t}_{\mathrm{1}} ^{\mathrm{2}} =\frac{{p}^{\mathrm{2}} −\mathrm{2}+{p}\sqrt{{p}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}}\wedge{t}_{\mathrm{2}} ^{\mathrm{2}} =\frac{{p}^{\mathrm{2}} −\mathrm{2}+{p}\sqrt{{p}^{\mathrm{2}} −\mathrm{4}}}{\mathrm{2}} \\ $$$$\Rightarrow\:{t}^{\mathrm{2}} +{t}^{−\mathrm{2}} ={t}_{\mathrm{1}} ^{\mathrm{2}} +{t}_{\mathrm{2}} ^{\mathrm{2}} ={p}^{\mathrm{2}} −\mathrm{2} \\ $$$${p}=−\frac{\mathrm{9}}{\mathrm{4}}\:\Rightarrow\:\mathrm{answer}\:\mathrm{is}\:\frac{\mathrm{49}}{\mathrm{16}} \\ $$
