Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 119921 by bramlexs22 last updated on 28/Oct/20

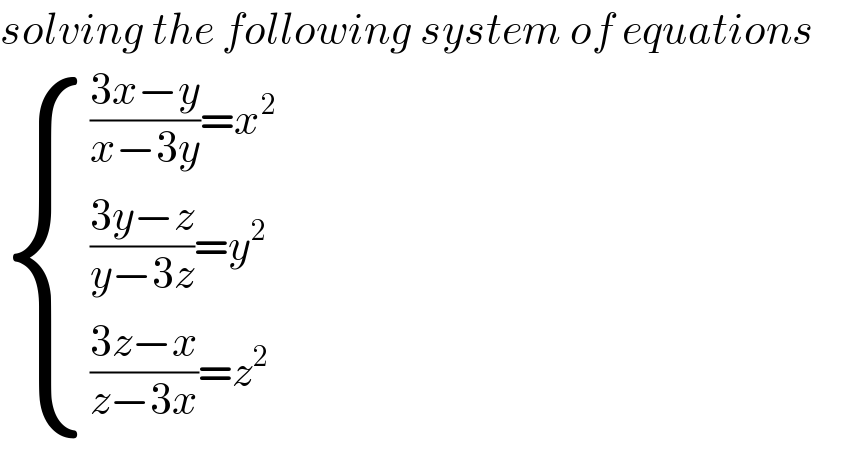
solving the following system of equations { ((((3x−y)/(x−3y))=x^2 )),((((3y−z)/(y−3z))=y^2 )),((((3z−x)/(z−3x))=z^2 )) :}

$${solving}\:{the}\:{following}\:{system}\:{of}\:{equations} \\ $$$$\begin{cases}{\frac{\mathrm{3}{x}−{y}}{{x}−\mathrm{3}{y}}={x}^{\mathrm{2}} }\\{\frac{\mathrm{3}{y}−{z}}{{y}−\mathrm{3}{z}}={y}^{\mathrm{2}} }\\{\frac{\mathrm{3}{z}−{x}}{{z}−\mathrm{3}{x}}={z}^{\mathrm{2}} }\end{cases} \\ $$

Answered by mindispower last updated on 28/Oct/20

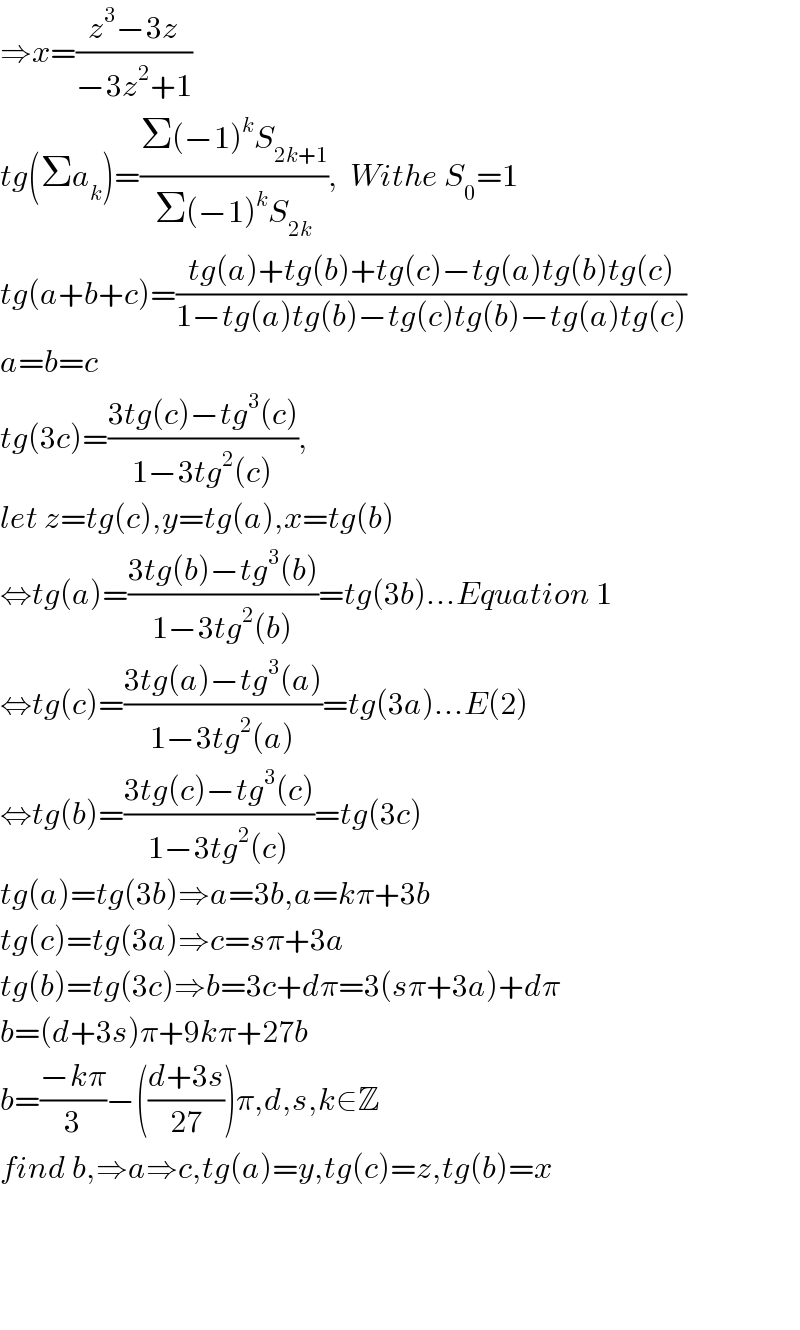
⇒x=((z^3 −3z)/(−3z^2 +1)) tg(Σa_k )=((Σ(−1)^k S_(2k+1) )/(Σ(−1)^k S_(2k) )), Withe S_0 =1 tg(a+b+c)=((tg(a)+tg(b)+tg(c)−tg(a)tg(b)tg(c))/(1−tg(a)tg(b)−tg(c)tg(b)−tg(a)tg(c))) a=b=c tg(3c)=((3tg(c)−tg^3 (c))/(1−3tg^2 (c))), let z=tg(c),y=tg(a),x=tg(b) ⇔tg(a)=((3tg(b)−tg^3 (b))/(1−3tg^2 (b)))=tg(3b)...Equation 1 ⇔tg(c)=((3tg(a)−tg^3 (a))/(1−3tg^2 (a)))=tg(3a)...E(2) ⇔tg(b)=((3tg(c)−tg^3 (c))/(1−3tg^2 (c)))=tg(3c) tg(a)=tg(3b)⇒a=3b,a=kπ+3b tg(c)=tg(3a)⇒c=sπ+3a tg(b)=tg(3c)⇒b=3c+dπ=3(sπ+3a)+dπ b=(d+3s)π+9kπ+27b b=((−kπ)/3)−(((d+3s)/(27)))π,d,s,k∈Z find b,⇒a⇒c,tg(a)=y,tg(c)=z,tg(b)=x

$$\Rightarrow{x}=\frac{{z}^{\mathrm{3}} −\mathrm{3}{z}}{−\mathrm{3}{z}^{\mathrm{2}} +\mathrm{1}} \\ $$$${tg}\left(\Sigma{a}_{{k}} \right)=\frac{\Sigma\left(−\mathrm{1}\right)^{{k}} {S}_{\mathrm{2}{k}+\mathrm{1}} }{\Sigma\left(−\mathrm{1}\right)^{{k}} {S}_{\mathrm{2}{k}} },\:\:{Withe}\:{S}_{\mathrm{0}} =\mathrm{1} \\ $$$${tg}\left({a}+{b}+{c}\right)=\frac{{tg}\left({a}\right)+{tg}\left({b}\right)+{tg}\left({c}\right)−{tg}\left({a}\right){tg}\left({b}\right){tg}\left({c}\right)}{\mathrm{1}−{tg}\left({a}\right){tg}\left({b}\right)−{tg}\left({c}\right){tg}\left({b}\right)−{tg}\left({a}\right){tg}\left({c}\right)} \\ $$$${a}={b}={c} \\ $$$${tg}\left(\mathrm{3}{c}\right)=\frac{\mathrm{3}{tg}\left({c}\right)−{tg}^{\mathrm{3}} \left({c}\right)}{\mathrm{1}−\mathrm{3}{tg}^{\mathrm{2}} \left({c}\right)}, \\ $$$${let}\:{z}={tg}\left({c}\right),{y}={tg}\left({a}\right),{x}={tg}\left({b}\right) \\ $$$$\Leftrightarrow{tg}\left({a}\right)=\frac{\mathrm{3}{tg}\left({b}\right)−{tg}^{\mathrm{3}} \left({b}\right)}{\mathrm{1}−\mathrm{3}{tg}^{\mathrm{2}} \left({b}\right)}={tg}\left(\mathrm{3}{b}\right)...{Equation}\:\mathrm{1} \\ $$$$\Leftrightarrow{tg}\left({c}\right)=\frac{\mathrm{3}{tg}\left({a}\right)−{tg}^{\mathrm{3}} \left({a}\right)}{\mathrm{1}−\mathrm{3}{tg}^{\mathrm{2}} \left({a}\right)}={tg}\left(\mathrm{3}{a}\right)...{E}\left(\mathrm{2}\right) \\ $$$$\Leftrightarrow{tg}\left({b}\right)=\frac{\mathrm{3}{tg}\left({c}\right)−{tg}^{\mathrm{3}} \left({c}\right)}{\mathrm{1}−\mathrm{3}{tg}^{\mathrm{2}} \left({c}\right)}={tg}\left(\mathrm{3}{c}\right) \\ $$$${tg}\left({a}\right)={tg}\left(\mathrm{3}{b}\right)\Rightarrow{a}=\mathrm{3}{b},{a}={k}\pi+\mathrm{3}{b} \\ $$$${tg}\left({c}\right)={tg}\left(\mathrm{3}{a}\right)\Rightarrow{c}={s}\pi+\mathrm{3}{a} \\ $$$${tg}\left({b}\right)={tg}\left(\mathrm{3}{c}\right)\Rightarrow{b}=\mathrm{3}{c}+{d}\pi=\mathrm{3}\left({s}\pi+\mathrm{3}{a}\right)+{d}\pi \\ $$$${b}=\left({d}+\mathrm{3}{s}\right)\pi+\mathrm{9}{k}\pi+\mathrm{27}{b} \\ $$$${b}=\frac{−{k}\pi}{\mathrm{3}}−\left(\frac{{d}+\mathrm{3}{s}}{\mathrm{27}}\right)\pi,{d},{s},{k}\in\mathbb{Z} \\ $$$${find}\:{b},\Rightarrow{a}\Rightarrow{c},{tg}\left({a}\right)={y},{tg}\left({c}\right)={z},{tg}\left({b}\right)={x} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Answered by MJS_new last updated on 28/Oct/20

one solution is x=y=z=±i for the other solutions let y=px∧z=qx ⇒ { ((((p−3)/(3p−1))=x^2 )),((((3p−q)/(p−3q))=p^2 x^2 )),((((3q−1)/(q−3))=q^2 x^2 )) :} ⇒ ((p−3)/(3p−1))=((3p−q)/(p^2 (p−3q)))=((3q−1)/(q^2 (q−3))) we can eliminate one of the two unknown and get a polynome of degree 13 with one solution =1. the other 12 are ∈R but I only can approximate q=((p(p^3 −3p^2 −9p+3))/(3p^3 −9p^2 −3p+1)) (p−1)((p^(12) +1)−20(p^(11) +p)+34(p^(10) +p^2 )+700(p^9 +p^3 )−2705(p^8 +p^4 )−680(p^7 +p^5 )+9436p^6 )=0 p_1 =1 p_2 ≈−5.87781 p_3 ≈−1.63530 p_4 ≈−.611508 p_5 ≈−.170131 p_6 ≈.0637272 p_7 ≈.199046 p_8 ≈.278216 p_9 ≈.320163 p_(10) ≈3.12340 p_(11) ≈3.59433 p_(12) ≈5.02397 p_(13) ≈15.6919 please do the rest for yourself

$$\mathrm{one}\:\mathrm{solution}\:\mathrm{is}\:{x}={y}={z}=\pm\mathrm{i} \\ $$$$\mathrm{for}\:\mathrm{the}\:\mathrm{other}\:\mathrm{solutions}\:\mathrm{let} \\ $$$${y}={px}\wedge{z}={qx} \\ $$$$\Rightarrow \\ $$$$\begin{cases}{\frac{{p}−\mathrm{3}}{\mathrm{3}{p}−\mathrm{1}}={x}^{\mathrm{2}} }\\{\frac{\mathrm{3}{p}−{q}}{{p}−\mathrm{3}{q}}={p}^{\mathrm{2}} {x}^{\mathrm{2}} }\\{\frac{\mathrm{3}{q}−\mathrm{1}}{{q}−\mathrm{3}}={q}^{\mathrm{2}} {x}^{\mathrm{2}} }\end{cases} \\ $$$$\Rightarrow \\ $$$$\frac{{p}−\mathrm{3}}{\mathrm{3}{p}−\mathrm{1}}=\frac{\mathrm{3}{p}−{q}}{{p}^{\mathrm{2}} \left({p}−\mathrm{3}{q}\right)}=\frac{\mathrm{3}{q}−\mathrm{1}}{{q}^{\mathrm{2}} \left({q}−\mathrm{3}\right)} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{eliminate}\:\mathrm{one}\:\mathrm{of}\:\mathrm{the}\:\mathrm{two}\:\mathrm{unknown} \\ $$$$\mathrm{and}\:\mathrm{get}\:\mathrm{a}\:\mathrm{polynome}\:\mathrm{of}\:\mathrm{degree}\:\mathrm{13}\:\mathrm{with}\:\mathrm{one} \\ $$$$\mathrm{solution}\:=\mathrm{1}.\:\mathrm{the}\:\mathrm{other}\:\mathrm{12}\:\mathrm{are}\:\in\mathbb{R}\:\mathrm{but}\:\mathrm{I}\:\mathrm{only} \\ $$$$\mathrm{can}\:\mathrm{approximate} \\ $$$${q}=\frac{{p}\left({p}^{\mathrm{3}} −\mathrm{3}{p}^{\mathrm{2}} −\mathrm{9}{p}+\mathrm{3}\right)}{\mathrm{3}{p}^{\mathrm{3}} −\mathrm{9}{p}^{\mathrm{2}} −\mathrm{3}{p}+\mathrm{1}} \\ $$$$\left({p}−\mathrm{1}\right)\left(\left({p}^{\mathrm{12}} +\mathrm{1}\right)−\mathrm{20}\left({p}^{\mathrm{11}} +{p}\right)+\mathrm{34}\left({p}^{\mathrm{10}} +{p}^{\mathrm{2}} \right)+\mathrm{700}\left({p}^{\mathrm{9}} +{p}^{\mathrm{3}} \right)−\mathrm{2705}\left({p}^{\mathrm{8}} +{p}^{\mathrm{4}} \right)−\mathrm{680}\left({p}^{\mathrm{7}} +{p}^{\mathrm{5}} \right)+\mathrm{9436}{p}^{\mathrm{6}} \right)=\mathrm{0} \\ $$$${p}_{\mathrm{1}} =\mathrm{1} \\ $$$${p}_{\mathrm{2}} \approx−\mathrm{5}.\mathrm{87781} \\ $$$${p}_{\mathrm{3}} \approx−\mathrm{1}.\mathrm{63530} \\ $$$${p}_{\mathrm{4}} \approx−.\mathrm{611508} \\ $$$${p}_{\mathrm{5}} \approx−.\mathrm{170131} \\ $$$${p}_{\mathrm{6}} \approx.\mathrm{0637272} \\ $$$${p}_{\mathrm{7}} \approx.\mathrm{199046} \\ $$$${p}_{\mathrm{8}} \approx.\mathrm{278216} \\ $$$${p}_{\mathrm{9}} \approx.\mathrm{320163} \\ $$$${p}_{\mathrm{10}} \approx\mathrm{3}.\mathrm{12340} \\ $$$${p}_{\mathrm{11}} \approx\mathrm{3}.\mathrm{59433} \\ $$$${p}_{\mathrm{12}} \approx\mathrm{5}.\mathrm{02397} \\ $$$${p}_{\mathrm{13}} \approx\mathrm{15}.\mathrm{6919} \\ $$$$\mathrm{please}\:\mathrm{do}\:\mathrm{the}\:\mathrm{rest}\:\mathrm{for}\:\mathrm{yourself} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com