Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 125054 by micelle last updated on 08/Dec/20

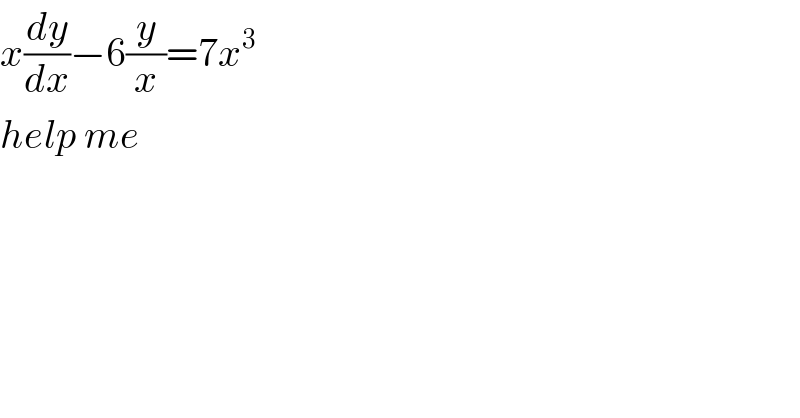
x(dy/dx)−6(y/x)=7x^3 help me

$${x}\frac{{dy}}{{dx}}−\mathrm{6}\frac{{y}}{{x}}=\mathrm{7}{x}^{\mathrm{3}} \\ $$$${help}\:{me} \\ $$

Answered by Dwaipayan Shikari last updated on 08/Dec/20

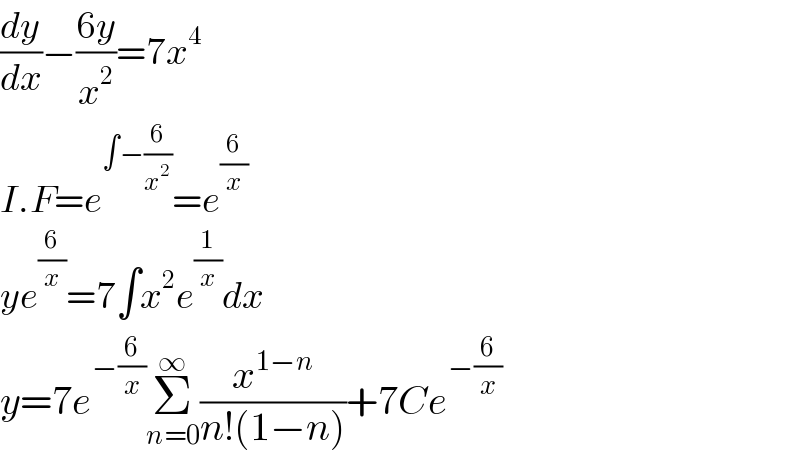
(dy/dx)−((6y)/x^2 )=7x^4 I.F=e^(∫−(6/x^2 )) =e^(6/x) ye^(6/x) =7∫x^2 e^(1/x) dx y=7e^(−(6/x)) Σ_(n=0) ^∞ (x^(1−n) /(n!(1−n)))+7Ce^(−(6/x))

$$\frac{{dy}}{{dx}}−\frac{\mathrm{6}{y}}{{x}^{\mathrm{2}} }=\mathrm{7}{x}^{\mathrm{4}} \\ $$$${I}.{F}={e}^{\int−\frac{\mathrm{6}}{{x}^{\mathrm{2}} }} ={e}^{\frac{\mathrm{6}}{{x}}} \:\:\:\:\:\:\:\: \\ $$$${ye}^{\frac{\mathrm{6}}{{x}}} =\mathrm{7}\int{x}^{\mathrm{2}} {e}^{\frac{\mathrm{1}}{{x}}} {dx} \\ $$$${y}=\mathrm{7}{e}^{−\frac{\mathrm{6}}{{x}}} \underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{x}^{\mathrm{1}−{n}} }{{n}!\left(\mathrm{1}−{n}\right)}+\mathrm{7}{Ce}^{−\frac{\mathrm{6}}{{x}}} \\ $$

Answered by TITA last updated on 08/Dec/20

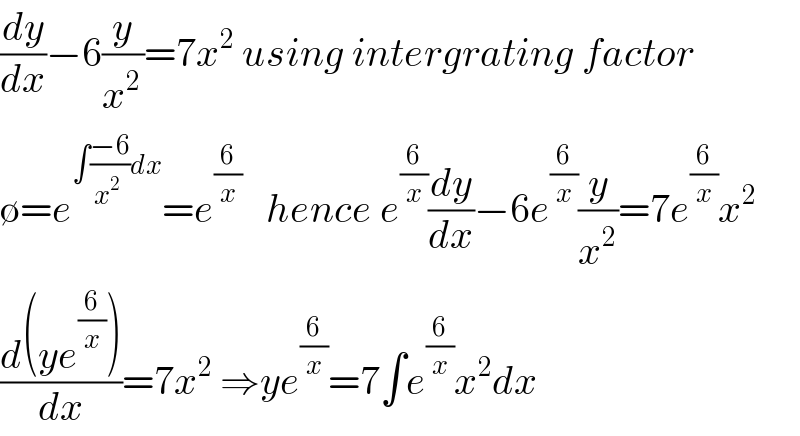
(dy/dx)−6(y/x^(2 ) )=7x^2 using intergrating factor ∅=e^(∫((−6)/x^(2 ) )dx) =e^(6/x) hence e^(6/x) (dy/dx)−6e^(6/x) (y/x^2 )=7e^(6/x) x^2 ((d(ye^(6/x) ))/dx)=7x^2 ⇒ye^(6/x) =7∫e^(6/x) x^2 dx

$$\frac{{dy}}{{dx}}−\mathrm{6}\frac{{y}}{{x}^{\mathrm{2}\:} }=\mathrm{7}{x}^{\mathrm{2}} \:{using}\:{intergrating}\:{factor} \\ $$$$\emptyset={e}^{\int\frac{−\mathrm{6}}{{x}^{\mathrm{2}\:\:} }{dx}} ={e}^{\frac{\mathrm{6}}{{x}}} \:\:\:{hence}\:{e}^{\frac{\mathrm{6}}{{x}}} \frac{{dy}}{{dx}}−\mathrm{6}{e}^{\frac{\mathrm{6}}{{x}}} \frac{{y}}{{x}^{\mathrm{2}} }=\mathrm{7}{e}^{\frac{\mathrm{6}}{{x}}} {x}^{\mathrm{2}} \\ $$$$\frac{{d}\left({ye}^{\frac{\mathrm{6}}{{x}}} \right)}{{dx}}=\mathrm{7}{x}^{\mathrm{2}} \:\Rightarrow{ye}^{\frac{\mathrm{6}}{{x}}} =\mathrm{7}\int{e}^{\frac{\mathrm{6}}{{x}}} {x}^{\mathrm{2}} {dx} \\ $$

Commented by TITA last updated on 08/Dec/20

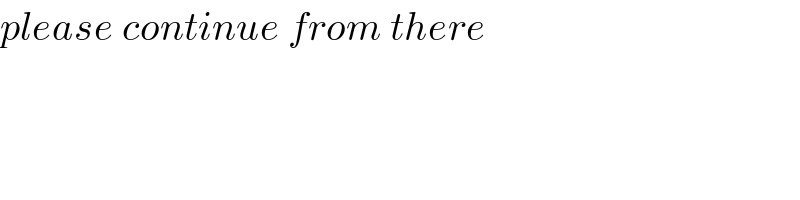
please continue from there

$${please}\:{continue}\:{from}\:{there} \\ $$

Commented by benjo_mathlover last updated on 08/Dec/20

it should be ye^(6/x) = ∫ 7x^2 e^(6/x) dx. your solution is wrong

$${it}\:{should}\:{be}\:{ye}^{\frac{\mathrm{6}}{{x}}} \:=\:\int\:\mathrm{7}{x}^{\mathrm{2}} {e}^{\frac{\mathrm{6}}{{x}}} \:{dx}. \\ $$$${your}\:{solution}\:{is}\:{wrong} \\ $$

Commented by TITA last updated on 08/Dec/20

ok thanks

$${ok}\:{thanks} \\ $$

Commented by mohammad17 last updated on 08/Dec/20

false solution

$${false}\:{solution} \\ $$

Answered by Bird last updated on 08/Dec/20

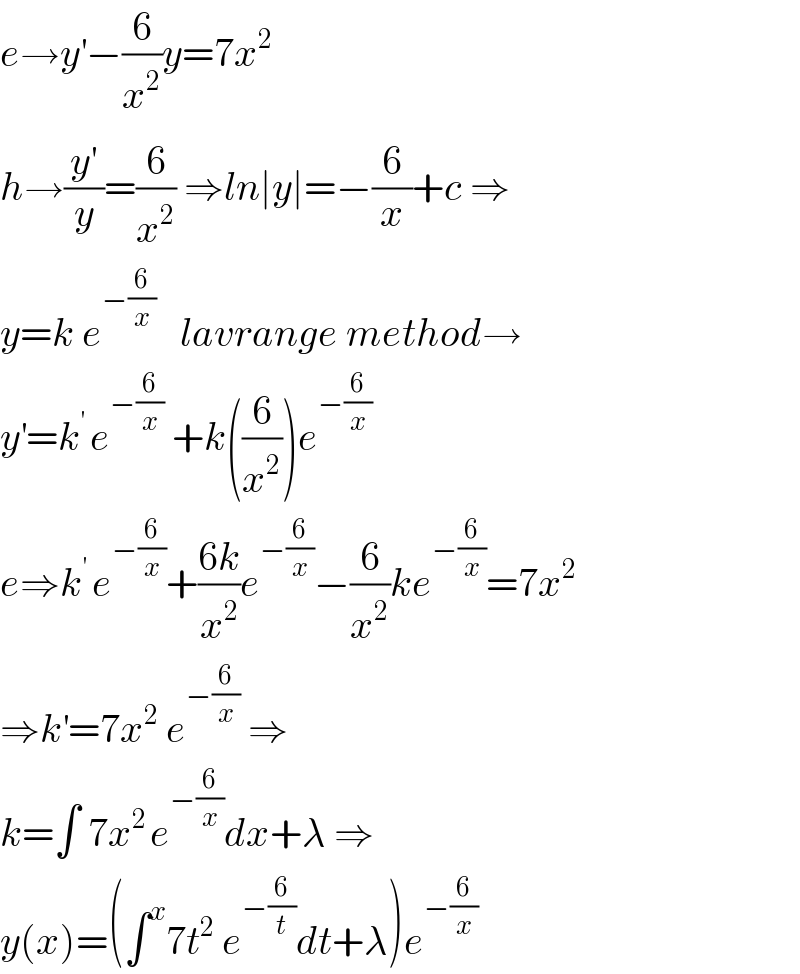
e→y^′ −(6/x^2 )y=7x^2 h→(y^′ /y)=(6/x^2 ) ⇒ln∣y∣=−(6/x)+c ⇒ y=k e^(−(6/x)) lavrange method→ y^′ =k^(′ ) e^(−(6/x)) +k((6/x^2 ))e^(−(6/x)) e⇒k^(′ ) e^(−(6/x)) +((6k)/x^2 )e^(−(6/x)) −(6/x^2 )ke^(−(6/x)) =7x^2 ⇒k^′ =7x^2 e^(−(6/x)) ⇒ k=∫ 7x^(2 ) e^(−(6/x)) dx+λ ⇒ y(x)=(∫^x 7t^2 e^(−(6/t)) dt+λ)e^(−(6/x))

$${e}\rightarrow{y}^{'} −\frac{\mathrm{6}}{{x}^{\mathrm{2}} }{y}=\mathrm{7}{x}^{\mathrm{2}} \\ $$$${h}\rightarrow\frac{{y}^{'} }{{y}}=\frac{\mathrm{6}}{{x}^{\mathrm{2}} }\:\Rightarrow{ln}\mid{y}\mid=−\frac{\mathrm{6}}{{x}}+{c}\:\Rightarrow \\ $$$${y}={k}\:{e}^{−\frac{\mathrm{6}}{{x}}} \:\:\:{lavrange}\:{method}\rightarrow \\ $$$${y}^{'} ={k}^{'\:} {e}^{−\frac{\mathrm{6}}{{x}}} \:+{k}\left(\frac{\mathrm{6}}{{x}^{\mathrm{2}} }\right){e}^{−\frac{\mathrm{6}}{{x}}} \\ $$$${e}\Rightarrow{k}^{'\:} {e}^{−\frac{\mathrm{6}}{{x}}} +\frac{\mathrm{6}{k}}{{x}^{\mathrm{2}} }{e}^{−\frac{\mathrm{6}}{{x}}} −\frac{\mathrm{6}}{{x}^{\mathrm{2}} }{ke}^{−\frac{\mathrm{6}}{{x}}} =\mathrm{7}{x}^{\mathrm{2}} \\ $$$$\Rightarrow{k}^{'} =\mathrm{7}{x}^{\mathrm{2}} \:{e}^{−\frac{\mathrm{6}}{{x}}} \:\Rightarrow \\ $$$${k}=\int\:\mathrm{7}{x}^{\mathrm{2}\:} {e}^{−\frac{\mathrm{6}}{{x}}} {dx}+\lambda\:\Rightarrow \\ $$$${y}\left({x}\right)=\left(\int^{{x}} \mathrm{7}{t}^{\mathrm{2}} \:{e}^{−\frac{\mathrm{6}}{{t}}} {dt}+\lambda\right){e}^{−\frac{\mathrm{6}}{{x}}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com