Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 125482 by joki last updated on 11/Dec/20

Answered by MJS_new last updated on 11/Dec/20

∫((4x^3 +x^2 +1)/((x^2 −2)^3 ))dx= [Ostrogradski′s Method] =((x^3 −64x^2 −14x+64)/(32(x^2 −2)^2 ))+(1/(32))∫(dx/(x^2 −2))= =((x^3 −64x^2 −14x+64)/(32(x^2 −2)^2 ))+((√2)/(128))ln ∣(((√2)x−2)/( (√2)x+2))∣ +C

$$\int\frac{\mathrm{4}{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +\mathrm{1}}{\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{3}} }{dx}= \\ $$$$\:\:\:\:\:\left[\mathrm{Ostrogradski}'\mathrm{s}\:\mathrm{Method}\right] \\ $$$$=\frac{{x}^{\mathrm{3}} −\mathrm{64}{x}^{\mathrm{2}} −\mathrm{14}{x}+\mathrm{64}}{\mathrm{32}\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{32}}\int\frac{{dx}}{{x}^{\mathrm{2}} −\mathrm{2}}= \\ $$$$=\frac{{x}^{\mathrm{3}} −\mathrm{64}{x}^{\mathrm{2}} −\mathrm{14}{x}+\mathrm{64}}{\mathrm{32}\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} }+\frac{\sqrt{\mathrm{2}}}{\mathrm{128}}\mathrm{ln}\:\mid\frac{\sqrt{\mathrm{2}}{x}−\mathrm{2}}{\:\sqrt{\mathrm{2}}{x}+\mathrm{2}}\mid\:+{C} \\ $$

Commented by MJS_new last updated on 11/Dec/20

please search for Ostrogradski′s Method here or on the www. it′s easy to understand. I don′t have the time now to explain it again

$$\mathrm{please}\:\mathrm{search}\:\mathrm{for}\:\mathrm{Ostrogradski}'\mathrm{s}\:\mathrm{Method} \\ $$$$\mathrm{here}\:\mathrm{or}\:\mathrm{on}\:\mathrm{the}\:\mathrm{www}.\:\mathrm{it}'\mathrm{s}\:\mathrm{easy}\:\mathrm{to}\:\mathrm{understand}. \\ $$$$\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{have}\:\mathrm{the}\:\mathrm{time}\:\mathrm{now}\:\mathrm{to}\:\mathrm{explain}\:\mathrm{it}\:\mathrm{again} \\ $$

Commented by joki last updated on 11/Dec/20

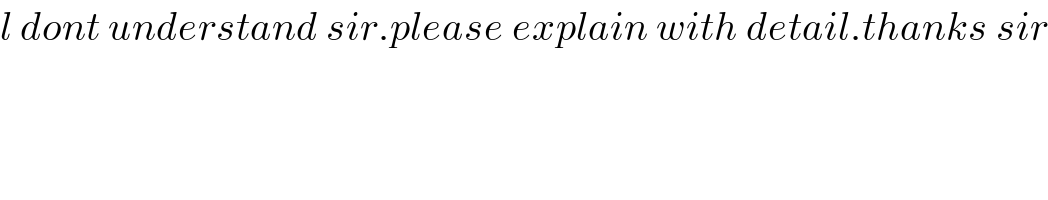
l dont understand sir.please explain with detail.thanks sir

$${l}\:{dont}\:{understand}\:{sir}.{please}\:{explain}\:{with}\:{detail}.{thanks}\:{sir} \\ $$

Commented by MJS_new last updated on 14/Dec/20

∫((P(x))/(Q(x)))dx=((P_1 (x))/(Q_1 (x)))+∫((P_2 (x))/(Q_2 (x)))dx where P(x), Q(x) are polynomes and the degree of P(x) < the degree of Q(x) and the fraction cannot be further simplified. then Q_1 (x)=gcd (Q(x), Q^′ (x)) and Q_2 (x)=((Q(x))/(Q_1 (x))) P_1 (x) and P_2 (x) are polynomes with degrees smaller than degrees of Q_1 (x) resp. Q_2 (x) we find the constants of P_1 (x), P_2 (x) by comparing the constants of both sides of ((P(x))/(Q(x)))=(d/dx)[((P_1 (x))/(Q_1 (x)))]+((P_2 (x))/(Q_2 (x)))

$$\int\frac{{P}\left({x}\right)}{{Q}\left({x}\right)}{dx}=\frac{{P}_{\mathrm{1}} \left({x}\right)}{{Q}_{\mathrm{1}} \left({x}\right)}+\int\frac{{P}_{\mathrm{2}} \left({x}\right)}{{Q}_{\mathrm{2}} \left({x}\right)}{dx} \\ $$$$\mathrm{where}\:{P}\left({x}\right),\:{Q}\left({x}\right)\:\mathrm{are}\:\mathrm{polynomes}\:\mathrm{and}\:\mathrm{the} \\ $$$$\mathrm{degree}\:\mathrm{of}\:{P}\left({x}\right)\:<\:\mathrm{the}\:\mathrm{degree}\:\mathrm{of}\:{Q}\left({x}\right)\:\mathrm{and}\:\mathrm{the} \\ $$$$\mathrm{fraction}\:\mathrm{cannot}\:\mathrm{be}\:\mathrm{further}\:\mathrm{simplified}. \\ $$$$\mathrm{then}\:{Q}_{\mathrm{1}} \left({x}\right)=\mathrm{gcd}\:\left({Q}\left({x}\right),\:{Q}^{'} \left({x}\right)\right) \\ $$$$\mathrm{and}\:{Q}_{\mathrm{2}} \left({x}\right)=\frac{{Q}\left({x}\right)}{{Q}_{\mathrm{1}} \left({x}\right)} \\ $$$${P}_{\mathrm{1}} \left({x}\right)\:\mathrm{and}\:{P}_{\mathrm{2}} \left({x}\right)\:\mathrm{are}\:\mathrm{polynomes}\:\mathrm{with}\:\mathrm{degrees} \\ $$$$\mathrm{smaller}\:\mathrm{than}\:\mathrm{degrees}\:\mathrm{of}\:{Q}_{\mathrm{1}} \left({x}\right)\:\mathrm{resp}.\:{Q}_{\mathrm{2}} \left({x}\right) \\ $$$$\mathrm{we}\:\mathrm{find}\:\mathrm{the}\:\mathrm{constants}\:\mathrm{of}\:{P}_{\mathrm{1}} \left({x}\right),\:{P}_{\mathrm{2}} \left({x}\right)\:\mathrm{by} \\ $$$$\mathrm{comparing}\:\mathrm{the}\:\mathrm{constants}\:\mathrm{of}\:\mathrm{both}\:\mathrm{sides}\:\mathrm{of} \\ $$$$\frac{{P}\left({x}\right)}{{Q}\left({x}\right)}=\frac{{d}}{{dx}}\left[\frac{{P}_{\mathrm{1}} \left({x}\right)}{{Q}_{\mathrm{1}} \left({x}\right)}\right]+\frac{{P}_{\mathrm{2}} \left({x}\right)}{{Q}_{\mathrm{2}} \left({x}\right)} \\ $$

Commented by joki last updated on 14/Dec/20

can you give me formula your answer sir ?thanks sir

$$\mathrm{can}\:\mathrm{you}\:\mathrm{give}\:\mathrm{me}\:\mathrm{formula}\:\mathrm{your}\:\mathrm{answer}\:\mathrm{sir}\:?\mathrm{thanks}\:\mathrm{sir} \\ $$

Commented by joki last updated on 15/Dec/20

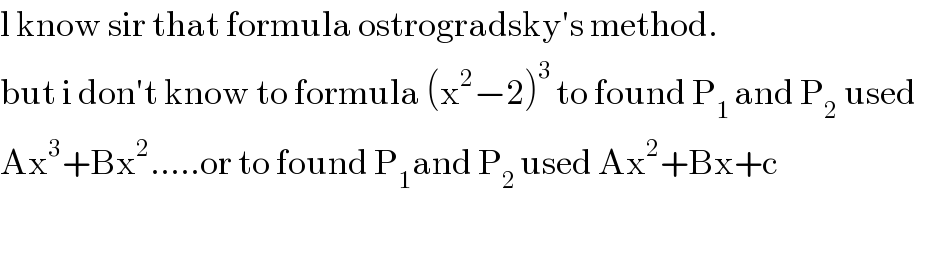
l know sir that formula ostrogradsky′s method. but i don′t know to formula (x^2 −2)^(3 ) to found P_(1 ) and P_2 used Ax^3 +Bx^2 .....or to found P_1 and P_(2 ) used Ax^2 +Bx+c

$$\mathrm{l}\:\mathrm{know}\:\mathrm{sir}\:\mathrm{that}\:\mathrm{formula}\:\mathrm{ostrogradsky}'\mathrm{s}\:\mathrm{method}. \\ $$$$\mathrm{but}\:\mathrm{i}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{to}\:\mathrm{formula}\:\left(\mathrm{x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{3}\:} \mathrm{to}\:\mathrm{found}\:\mathrm{P}_{\mathrm{1}\:} \mathrm{and}\:\mathrm{P}_{\mathrm{2}} \:\mathrm{used}\:\: \\ $$$$\mathrm{Ax}^{\mathrm{3}} +\mathrm{Bx}^{\mathrm{2}} .....\mathrm{or}\:\mathrm{to}\:\mathrm{found}\:\mathrm{P}_{\mathrm{1}} \mathrm{and}\:\mathrm{P}_{\mathrm{2}\:} \mathrm{used}\:\mathrm{Ax}^{\mathrm{2}} +\mathrm{Bx}+\mathrm{c} \\ $$$$ \\ $$

Commented by Tawa11 last updated on 06/Nov/21

Great

$$\mathrm{Great} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com