Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 132000 by bramlexs22 last updated on 10/Feb/21

super nice ∫_0 ^∞ ((1/((x^3 +(1/x^3 ))^2 )) )dx

$$\:\mathrm{super}\:\mathrm{nice}\: \\ $$$$\:\int_{\mathrm{0}} ^{\infty} \left(\frac{\mathrm{1}}{\left(\mathrm{x}^{\mathrm{3}} +\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{3}} }\right)^{\mathrm{2}} }\:\right)\mathrm{dx} \\ $$

Answered by EDWIN88 last updated on 10/Feb/21

E = ∫ (x^6 /((x^6 +1)^2 )) dx ; apply integration by parts { ((u=x⇒du=dx)),((dv=(x^5 /((x^6 +1)^2 ))⇒v=−(1/6).(1/(x^6 +1)))) :} E = −(x/(6(x^6 +1))) ]_0 ^∞ +(1/6)∫_0 ^∞ (dx/(x^6 +1)) E=0+(1/6)∫_0 ^∞ (dx/(x^6 +1)); replacing x by (1/x) E=(1/6)∫_0 ^∞ (x^4 /(x^6 +1)) dx ; adding we get 2E=(1/6)∫_0 ^∞ ((x^4 +1)/(x^6 +1)) dx E=(1/(12))∫_0 ^∞ ((x^2 +x^(−2) )/(x^3 +x^(−3) )) ((dx/x)) =(1/(12))∫_0 ^∞ ((x^2 +x^(−2) )/(x^3 +x^(−3) )) ((d(x−x^(−1) ))/(x+x^(−1) )) E=(1/(12))∫_0 ^∞ ((x^2 +x^(−2) )/(x^2 −1+x^(−2) )) ((d(x−x^(−1) ))/((x+x^(−1) )^2 )) letting r = x−x^(−1) E=(1/(12))∫_(−∞) ^∞ ((r^2 +2)/((r^2 +1)(r^2 +4)))dr E=(1/(36))∫^∞ _(−∞) ((1/(r^2 +1))+(2/(r^2 +4)))dr E=(1/(36)) (arctan r + arctan ((r/2))]_(−∞) ^∞ =(π/(18))

$$\mathrm{E}\:=\:\int\:\frac{\mathrm{x}^{\mathrm{6}} }{\left(\mathrm{x}^{\mathrm{6}} +\mathrm{1}\right)^{\mathrm{2}} }\:\mathrm{dx}\:;\:\mathrm{apply}\:\mathrm{integration}\:\mathrm{by}\:\mathrm{parts} \\ $$$$\:\begin{cases}{\mathrm{u}=\mathrm{x}\Rightarrow\mathrm{du}=\mathrm{dx}}\\{\mathrm{dv}=\frac{\mathrm{x}^{\mathrm{5}} }{\left(\mathrm{x}^{\mathrm{6}} +\mathrm{1}\right)^{\mathrm{2}} }\Rightarrow\mathrm{v}=−\frac{\mathrm{1}}{\mathrm{6}}.\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{6}} +\mathrm{1}}}\end{cases}\: \\ $$$$\left.\mathrm{E}\:=\:−\frac{\mathrm{x}}{\mathrm{6}\left(\mathrm{x}^{\mathrm{6}} +\mathrm{1}\right)}\:\right]_{\mathrm{0}} ^{\infty} +\frac{\mathrm{1}}{\mathrm{6}}\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{6}} +\mathrm{1}} \\ $$$$\mathrm{E}=\mathrm{0}+\frac{\mathrm{1}}{\mathrm{6}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{6}} +\mathrm{1}};\:\mathrm{replacing}\:\mathrm{x}\:\mathrm{by}\:\frac{\mathrm{1}}{\mathrm{x}} \\ $$$$\mathrm{E}=\frac{\mathrm{1}}{\mathrm{6}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{x}^{\mathrm{6}} +\mathrm{1}}\:\mathrm{dx}\:;\:\mathrm{adding}\:\mathrm{we}\:\mathrm{get}\: \\ $$$$\mathrm{2E}=\frac{\mathrm{1}}{\mathrm{6}}\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{x}^{\mathrm{4}} +\mathrm{1}}{\mathrm{x}^{\mathrm{6}} +\mathrm{1}}\:\mathrm{dx}\: \\ $$$$\mathrm{E}=\frac{\mathrm{1}}{\mathrm{12}}\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{x}^{−\mathrm{2}} }{\mathrm{x}^{\mathrm{3}} +\mathrm{x}^{−\mathrm{3}} }\:\left(\frac{\mathrm{dx}}{\mathrm{x}}\right)\:=\frac{\mathrm{1}}{\mathrm{12}}\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{x}^{−\mathrm{2}} }{\mathrm{x}^{\mathrm{3}} +\mathrm{x}^{−\mathrm{3}} }\:\frac{\mathrm{d}\left(\mathrm{x}−\mathrm{x}^{−\mathrm{1}} \right)}{\mathrm{x}+\mathrm{x}^{−\mathrm{1}} } \\ $$$$\mathrm{E}=\frac{\mathrm{1}}{\mathrm{12}}\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{x}^{−\mathrm{2}} }{\mathrm{x}^{\mathrm{2}} −\mathrm{1}+\mathrm{x}^{−\mathrm{2}} }\:\frac{\mathrm{d}\left(\mathrm{x}−\mathrm{x}^{−\mathrm{1}} \right)}{\left(\mathrm{x}+\mathrm{x}^{−\mathrm{1}} \right)^{\mathrm{2}} } \\ $$$$\mathrm{letting}\:\mathrm{r}\:=\:\mathrm{x}−\mathrm{x}^{−\mathrm{1}} \\ $$$$\mathrm{E}=\frac{\mathrm{1}}{\mathrm{12}}\int_{−\infty} ^{\infty} \frac{\mathrm{r}^{\mathrm{2}} +\mathrm{2}}{\left(\mathrm{r}^{\mathrm{2}} +\mathrm{1}\right)\left(\mathrm{r}^{\mathrm{2}} +\mathrm{4}\right)}\mathrm{dr} \\ $$$$\mathrm{E}=\frac{\mathrm{1}}{\mathrm{36}}\underset{−\infty} {\int}^{\infty} \left(\frac{\mathrm{1}}{\mathrm{r}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{2}}{\mathrm{r}^{\mathrm{2}} +\mathrm{4}}\right)\mathrm{dr} \\ $$$$\mathrm{E}=\frac{\mathrm{1}}{\mathrm{36}}\:\left(\mathrm{arctan}\:\mathrm{r}\:+\:\mathrm{arctan}\:\left(\frac{\mathrm{r}}{\mathrm{2}}\right)\right]_{−\infty} ^{\infty} =\frac{\pi}{\mathrm{18}} \\ $$$$ \\ $$

Answered by Ar Brandon last updated on 10/Feb/21

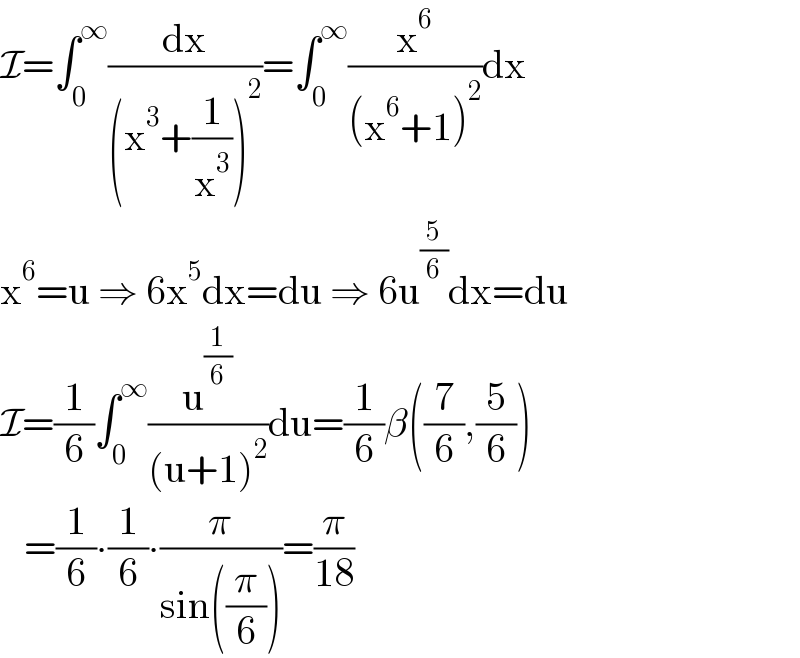
I=∫_0 ^∞ (dx/((x^3 +(1/x^3 ))^2 ))=∫_0 ^∞ (x^6 /((x^6 +1)^2 ))dx x^6 =u ⇒ 6x^5 dx=du ⇒ 6u^(5/6) dx=du I=(1/6)∫_0 ^∞ (u^(1/6) /((u+1)^2 ))du=(1/6)β((7/6),(5/6)) =(1/6)∙(1/6)∙(π/(sin((π/6))))=(π/(18))

$$\mathcal{I}=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{dx}}{\left(\mathrm{x}^{\mathrm{3}} +\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{3}} }\right)^{\mathrm{2}} }=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{x}^{\mathrm{6}} }{\left(\mathrm{x}^{\mathrm{6}} +\mathrm{1}\right)^{\mathrm{2}} }\mathrm{dx} \\ $$$$\mathrm{x}^{\mathrm{6}} =\mathrm{u}\:\Rightarrow\:\mathrm{6x}^{\mathrm{5}} \mathrm{dx}=\mathrm{du}\:\Rightarrow\:\mathrm{6u}^{\frac{\mathrm{5}}{\mathrm{6}}} \mathrm{dx}=\mathrm{du} \\ $$$$\mathcal{I}=\frac{\mathrm{1}}{\mathrm{6}}\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{u}^{\frac{\mathrm{1}}{\mathrm{6}}} }{\left(\mathrm{u}+\mathrm{1}\right)^{\mathrm{2}} }\mathrm{du}=\frac{\mathrm{1}}{\mathrm{6}}\beta\left(\frac{\mathrm{7}}{\mathrm{6}},\frac{\mathrm{5}}{\mathrm{6}}\right) \\ $$$$\:\:\:=\frac{\mathrm{1}}{\mathrm{6}}\centerdot\frac{\mathrm{1}}{\mathrm{6}}\centerdot\frac{\pi}{\mathrm{sin}\left(\frac{\pi}{\mathrm{6}}\right)}=\frac{\pi}{\mathrm{18}} \\ $$

Commented by bramlexs22 last updated on 10/Feb/21

with betha function

$$\mathrm{with}\:\mathrm{betha}\:\mathrm{function} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com