Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 133036 by liberty last updated on 18/Feb/21

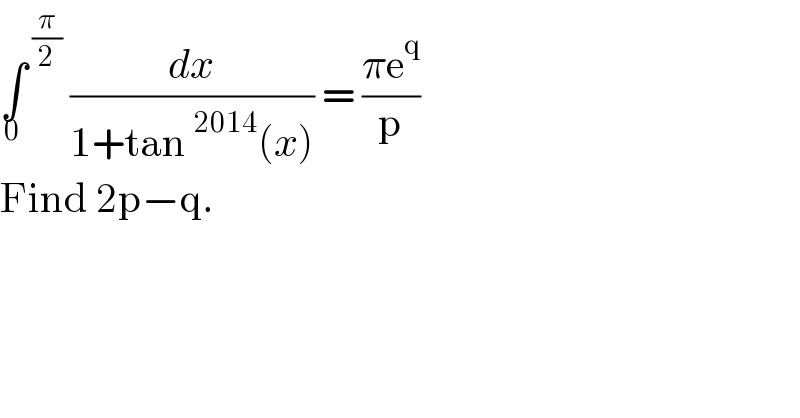
∫^( (π/2)) _0 (dx/(1+tan^(2014) (x))) = ((πe^q )/p) Find 2p−q.

$$\underset{\mathrm{0}} {\int}^{\:\frac{\pi}{\mathrm{2}}} \:\frac{{dx}}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2014}} \left({x}\right)}\:=\:\frac{\pi\mathrm{e}^{\mathrm{q}} }{\mathrm{p}} \\ $$$$\mathrm{Find}\:\mathrm{2p}−\mathrm{q}.\: \\ $$

Answered by liberty last updated on 18/Feb/21

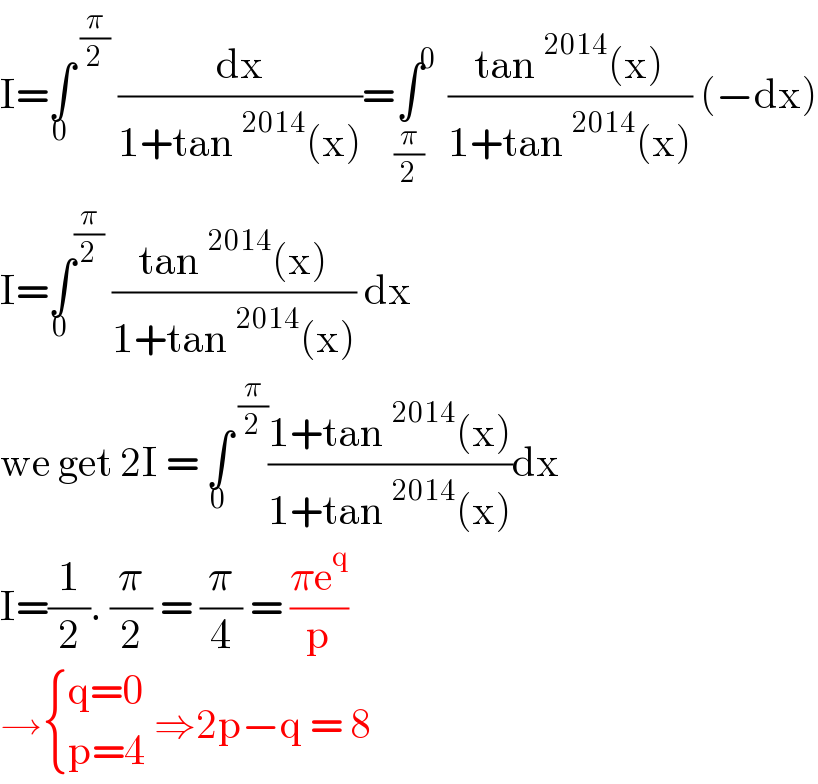
I=∫^( (π/2)) _0 (dx/(1+tan^(2014) (x)))=∫^0 _(π/2) ((tan^(2014) (x))/(1+tan^(2014) (x))) (−dx) I=∫^(π/2) _0 ((tan^(2014) (x))/(1+tan^(2014) (x))) dx we get 2I = ∫^( (π/2)) _0 ((1+tan^(2014) (x))/(1+tan^(2014) (x)))dx I=(1/2). (π/2) = (π/4) = ((πe^q )/p) → { ((q=0)),((p=4)) :} ⇒2p−q = 8

$$\mathrm{I}=\underset{\mathrm{0}} {\int}^{\:\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{dx}}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2014}} \left(\mathrm{x}\right)}=\underset{\frac{\pi}{\mathrm{2}}} {\int}^{\mathrm{0}} \:\frac{\mathrm{tan}\:^{\mathrm{2014}} \left(\mathrm{x}\right)}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2014}} \left(\mathrm{x}\right)}\:\left(−\mathrm{dx}\right) \\ $$$$\mathrm{I}=\underset{\mathrm{0}} {\int}^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{tan}\:^{\mathrm{2014}} \left(\mathrm{x}\right)}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2014}} \left(\mathrm{x}\right)}\:\mathrm{dx}\: \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{2I}\:=\:\underset{\mathrm{0}} {\int}^{\:\frac{\pi}{\mathrm{2}}} \frac{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2014}} \left(\mathrm{x}\right)}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2014}} \left(\mathrm{x}\right)}\mathrm{dx} \\ $$$$\mathrm{I}=\frac{\mathrm{1}}{\mathrm{2}}.\:\frac{\pi}{\mathrm{2}}\:=\:\frac{\pi}{\mathrm{4}}\:=\:\frac{\pi\mathrm{e}^{\mathrm{q}} }{\mathrm{p}} \\ $$$$\rightarrow\begin{cases}{\mathrm{q}=\mathrm{0}}\\{\mathrm{p}=\mathrm{4}}\end{cases}\:\Rightarrow\mathrm{2p}−\mathrm{q}\:=\:\mathrm{8}\: \\ $$

Answered by mathmax by abdo last updated on 20/Feb/21

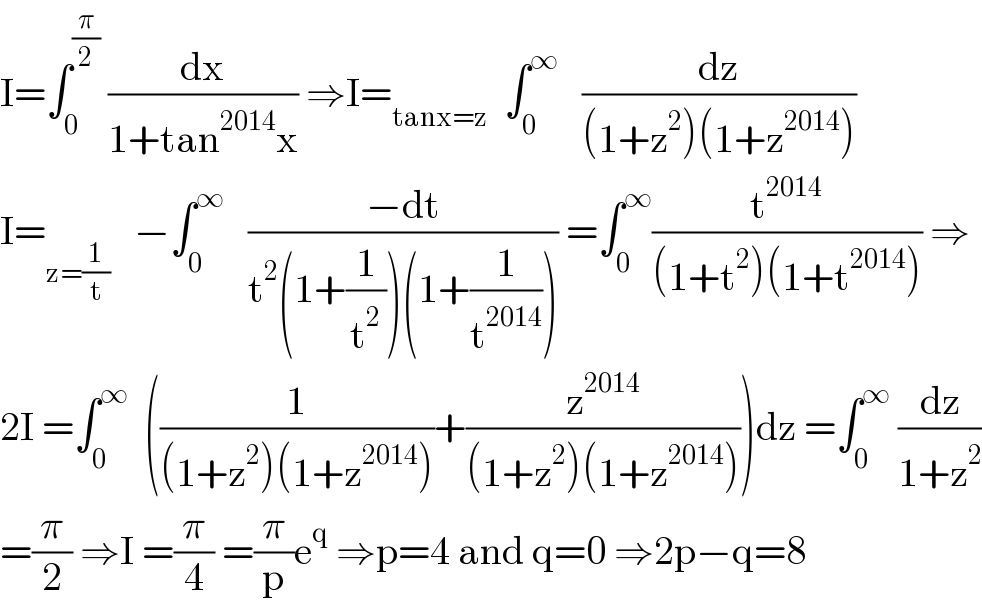
I=∫_0 ^(π/2) (dx/(1+tan^(2014) x)) ⇒I=_(tanx=z) ∫_0 ^∞ (dz/((1+z^2 )(1+z^(2014) ))) I=_(z=(1/t)) −∫_0 ^∞ ((−dt)/(t^2 (1+(1/t^2 ))(1+(1/t^(2014) )))) =∫_0 ^∞ (t^(2014) /((1+t^2 )(1+t^(2014) ))) ⇒ 2I =∫_0 ^∞ ((1/((1+z^2 )(1+z^(2014) )))+(z^(2014) /((1+z^2 )(1+z^(2014) ))))dz =∫_0 ^∞ (dz/(1+z^2 )) =(π/2) ⇒I =(π/4) =(π/p)e^q ⇒p=4 and q=0 ⇒2p−q=8

$$\mathrm{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{dx}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2014}} \mathrm{x}}\:\Rightarrow\mathrm{I}=_{\mathrm{tanx}=\mathrm{z}} \:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{dz}}{\left(\mathrm{1}+\mathrm{z}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{z}^{\mathrm{2014}} \right)} \\ $$$$\mathrm{I}=_{\mathrm{z}=\frac{\mathrm{1}}{\mathrm{t}}} \:\:\:−\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{−\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} \left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} }\right)\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2014}} }\right)}\:=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{t}^{\mathrm{2014}} }{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{t}^{\mathrm{2014}} \right)}\:\Rightarrow \\ $$$$\mathrm{2I}\:=\int_{\mathrm{0}} ^{\infty} \:\:\left(\frac{\mathrm{1}}{\left(\mathrm{1}+\mathrm{z}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{z}^{\mathrm{2014}} \right)}+\frac{\mathrm{z}^{\mathrm{2014}} }{\left(\mathrm{1}+\mathrm{z}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{z}^{\mathrm{2014}} \right)}\right)\mathrm{dz}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{dz}}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} } \\ $$$$=\frac{\pi}{\mathrm{2}}\:\Rightarrow\mathrm{I}\:=\frac{\pi}{\mathrm{4}}\:=\frac{\pi}{\mathrm{p}}\mathrm{e}^{\mathrm{q}} \:\Rightarrow\mathrm{p}=\mathrm{4}\:\mathrm{and}\:\mathrm{q}=\mathrm{0}\:\Rightarrow\mathrm{2p}−\mathrm{q}=\mathrm{8} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com