
Question Number 133730 by mr W last updated on 24/Feb/21

Commented by mr W last updated on 23/Feb/21
$${as}\:{Q}\mathrm{133275},\:{but}\:{with}\:{coefficient}\:{of} \\ $$$${restitution}\:\boldsymbol{{e}}\:{for}\:{the}\:{impacts}. \\ $$
Commented by Abdoulaye last updated on 23/Feb/21
$${how}\:{to}\:{take}\:{it}\:{here}? \\ $$
Answered by mr W last updated on 23/Feb/21
Commented by mr W last updated on 26/Feb/21

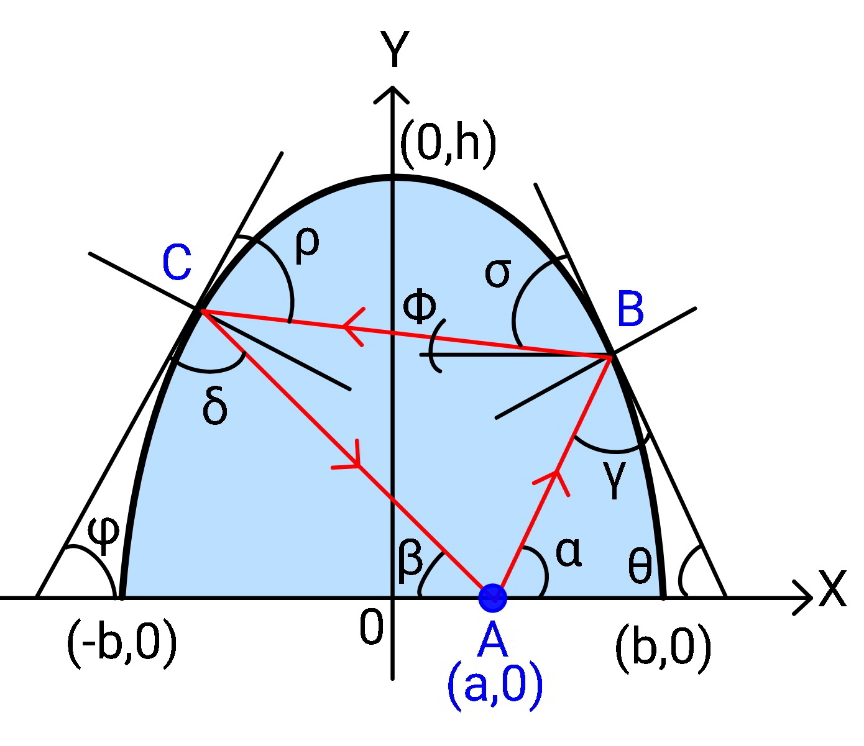
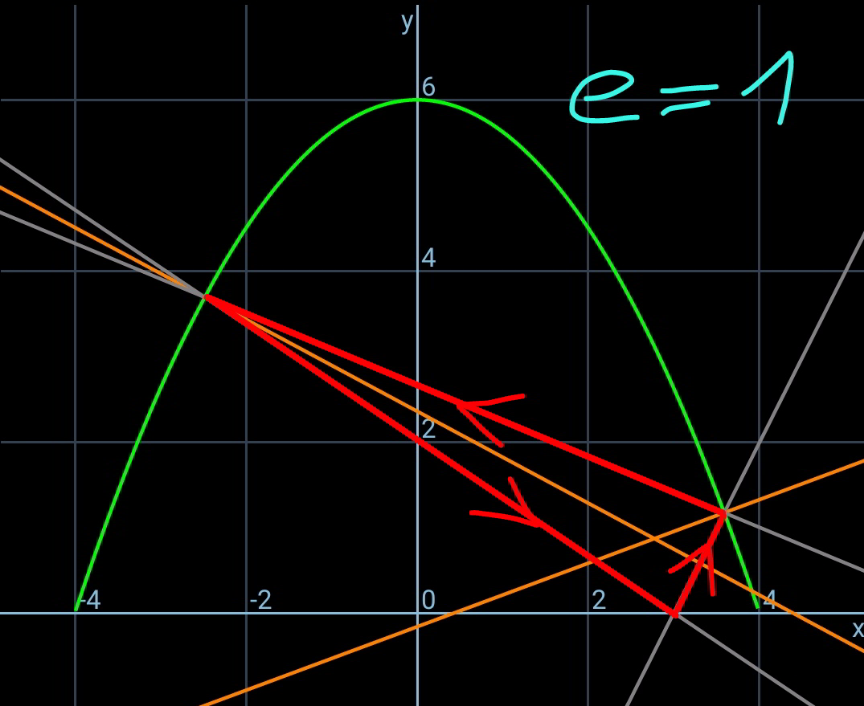
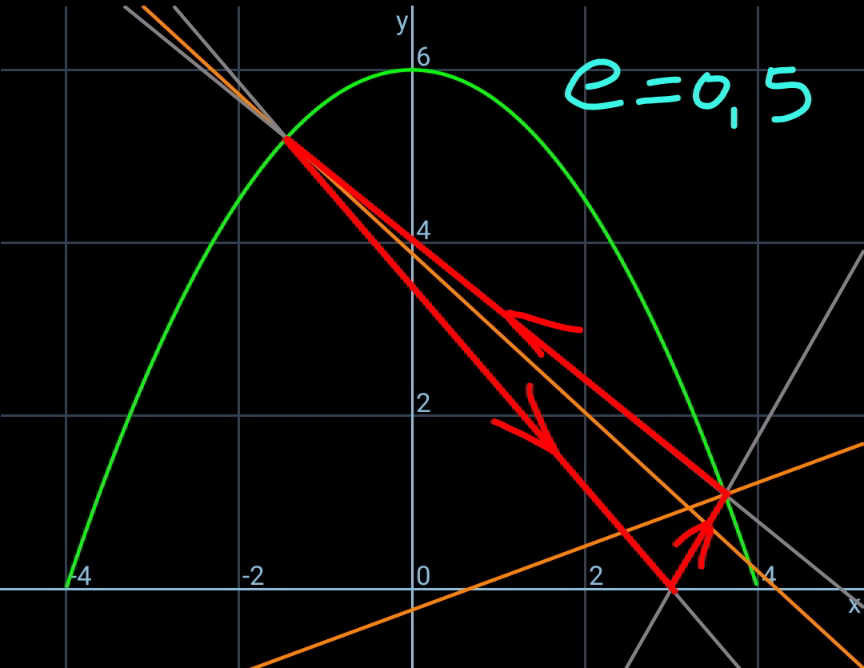
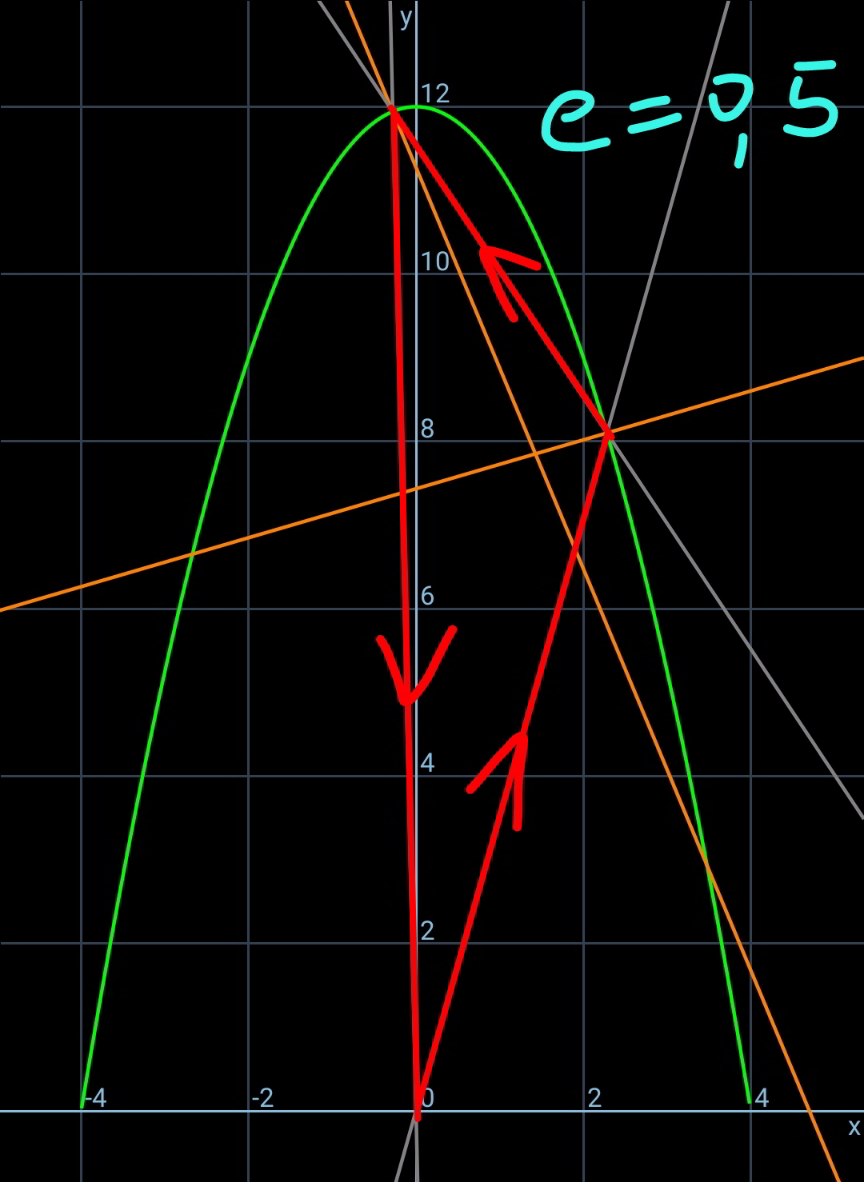
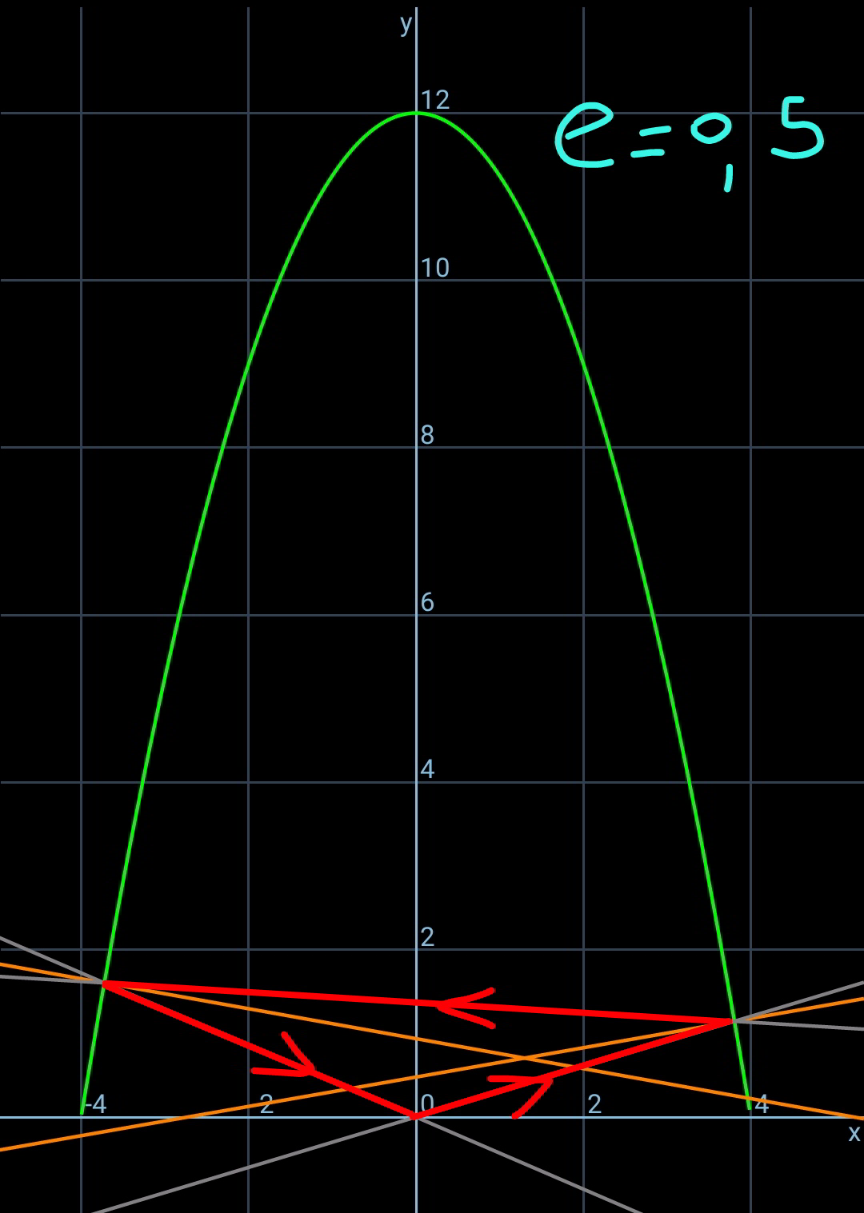
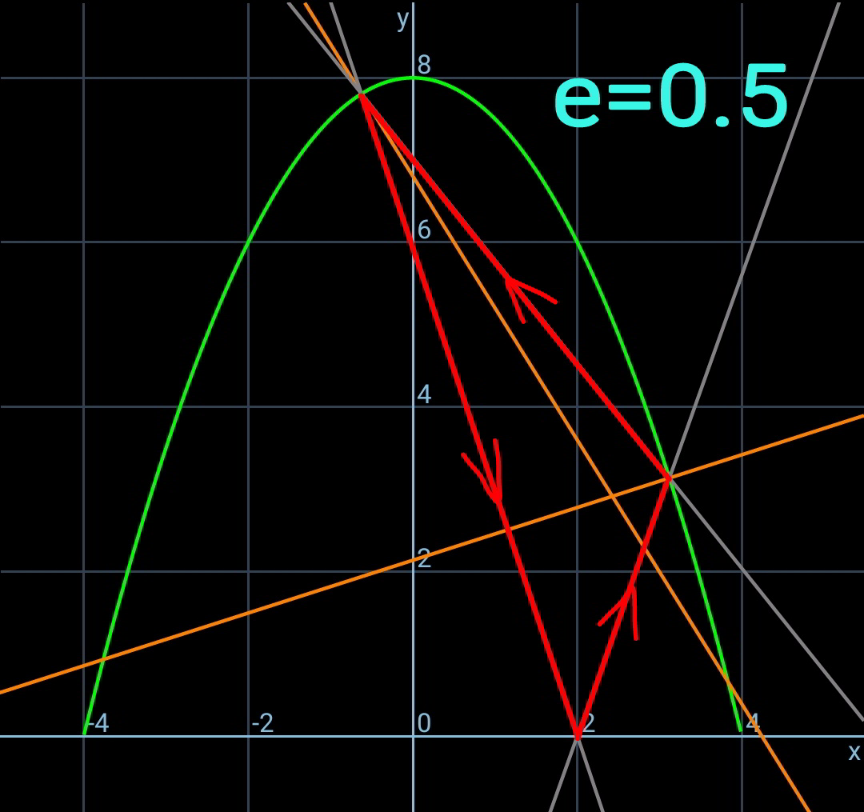
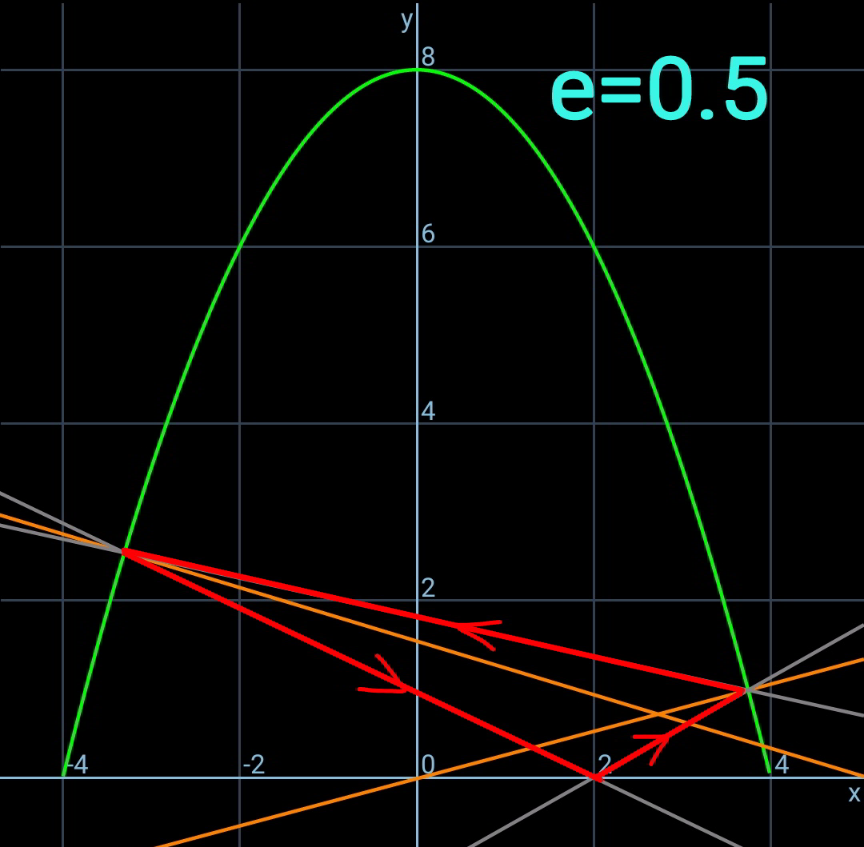
$${let}\:\eta=\frac{{h}}{{b}},\:\xi=\frac{{a}}{{b}},\:\lambda=\frac{{p}}{{b}},\:\mu=\frac{{q}}{{b}} \\ $$$${equation}\:{of}\:{parabola}: \\ $$$${y}={h}−\frac{{hx}^{\mathrm{2}} }{{b}^{\mathrm{2}} } \\ $$$$\frac{{dy}}{{dx}}=−\frac{\mathrm{2}{hx}}{{b}^{\mathrm{2}} } \\ $$$${say}\:{B}\left({p},\:{y}_{{B}} \right)\:{with}\:{y}_{{B}} ={h}−\frac{{hp}^{\mathrm{2}} }{{b}^{\mathrm{2}} } \\ $$$${say}\:{C}\left({q},\:{y}_{{B}} \right)\:{with}\:{y}_{{C}} ={h}−\frac{{hq}^{\mathrm{2}} }{{b}^{\mathrm{2}} } \\ $$$$\mathrm{tan}\:\theta=\frac{\mathrm{2}{hp}}{{b}^{\mathrm{2}} }=\mathrm{2}\eta\lambda \\ $$$$\mathrm{tan}\:\varphi=−\frac{\mathrm{2}{hq}}{{b}^{\mathrm{2}} }=−\mathrm{2}\eta\mu \\ $$$$\mathrm{tan}\:\alpha=\frac{{y}_{{B}} }{{p}−{a}}=\frac{{h}−\frac{{hp}^{\mathrm{2}} }{{b}^{\mathrm{2}} }}{{p}−{a}}=\frac{\eta\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)}{\lambda−\xi} \\ $$$$\mathrm{tan}\:\beta=\frac{{y}_{{C}} }{{a}−{q}}=\frac{{h}−\frac{{hq}^{\mathrm{2}} }{{b}^{\mathrm{2}} }}{{a}−{q}}=\frac{\eta\left(\mathrm{1}−\mu^{\mathrm{2}} \right)}{\xi−\mu} \\ $$$$\mathrm{tan}\:\phi=\frac{{y}_{{C}} −{y}_{{B}} }{{p}−{q}}=\frac{−\frac{{hq}^{\mathrm{2}} }{{b}^{\mathrm{2}} }+\frac{{hp}^{\mathrm{2}} }{{b}^{\mathrm{2}} }}{{p}−{q}}=\eta\left(\lambda+\mu\right) \\ $$$$\gamma=\pi−\alpha−\theta \\ $$$$\delta=\pi−\beta−\varphi \\ $$$$ \\ $$$$\sigma=\theta−\phi \\ $$$$\mathrm{tan}\:\sigma={e}\:\mathrm{tan}\:\gamma \\ $$$$\mathrm{tan}\:\left(\theta−\phi\right)=−{e}\:\mathrm{tan}\:\left(\theta+\alpha\right) \\ $$$$\frac{\mathrm{tan}\:\theta−\mathrm{tan}\:\phi}{\mathrm{1}+\mathrm{tan}\:\theta\:\mathrm{tan}\:\phi}=−{e}\frac{\mathrm{tan}\:\theta+\mathrm{tan}\:\alpha}{\mathrm{1}−\mathrm{tan}\:\theta\:\mathrm{tan}\:\alpha} \\ $$$$\frac{\mathrm{2}\eta\lambda−\eta\left(\lambda+\mu\right)}{\mathrm{1}+\mathrm{2}\eta\lambda\eta\left(\lambda+\mu\right)}=−{e}\frac{\mathrm{2}\eta\lambda+\frac{\eta\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)}{\lambda−\xi}}{\mathrm{1}−\mathrm{2}\eta\lambda\frac{\eta\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)}{\lambda−\xi}} \\ $$$$\frac{\lambda−\mu}{\mathrm{1}+\mathrm{2}\eta^{\mathrm{2}} \lambda\left(\lambda+\mu\right)}=−{e}\frac{\lambda^{\mathrm{2}} −\mathrm{2}\xi\lambda+\mathrm{1}}{\lambda−\xi−\mathrm{2}\eta^{\mathrm{2}} \lambda\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)} \\ $$$$\Rightarrow\frac{\lambda−\mu}{\mathrm{1}+\mathrm{2}\eta^{\mathrm{2}} \lambda\left(\lambda+\mu\right)}+{e}×\frac{\lambda^{\mathrm{2}} −\mathrm{2}\xi\lambda+\mathrm{1}}{\lambda−\xi−\mathrm{2}\eta^{\mathrm{2}} \lambda\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)}=\mathrm{0}\:\:\:...\left({i}\right) \\ $$$$ \\ $$$$\rho=\varphi+\phi \\ $$$${e}\:\mathrm{tan}\:\rho=\mathrm{tan}\:\delta \\ $$$$\mathrm{tan}\:\left(\varphi+\phi\right)=−\frac{\mathrm{1}}{{e}}\mathrm{tan}\:\left(\varphi+\beta\right) \\ $$$$\frac{\mathrm{tan}\:\varphi+\mathrm{tan}\:\phi}{\mathrm{1}−\mathrm{tan}\:\varphi\:\mathrm{tan}\:\phi}=−\frac{\mathrm{1}}{{e}}\frac{\mathrm{tan}\:\varphi+\mathrm{tan}\:\beta}{\mathrm{1}−\mathrm{tan}\:\varphi\:\mathrm{tan}\:\beta} \\ $$$$\frac{−\mathrm{2}\eta\mu+\eta\left(\lambda+\mu\right)}{\mathrm{1}+\mathrm{2}\eta\mu\eta\left(\lambda+\mu\right)}=−\frac{\mathrm{1}}{{e}}\frac{−\mathrm{2}\eta\mu+\frac{\eta\left(\mathrm{1}−\mu^{\mathrm{2}} \right)}{\xi−\mu}}{\mathrm{1}+\mathrm{2}\eta\mu\frac{\eta\left(\mathrm{1}−\mu^{\mathrm{2}} \right)}{\xi−\mu}} \\ $$$$\Rightarrow\frac{\lambda−\mu}{\mathrm{1}+\mathrm{2}\eta^{\mathrm{2}} \mu\left(\lambda+\mu\right)}−\frac{\mathrm{1}}{{e}}×\frac{\mu^{\mathrm{2}} −\mathrm{2}\xi\mu+\mathrm{1}}{\mu−\xi−\mathrm{2}\eta^{\mathrm{2}} \mu\left(\mathrm{1}−\mu^{\mathrm{2}} \right)}=\mathrm{0}\:\:\:...\left({ii}\right) \\ $$$$ \\ $$$${from}\:\left({i}\right)\:{and}\:\left({ii}\right)\:{we}\:{get}\:\lambda\:{and}\:\mu. \\ $$$$ \\ $$$${see}\:{examples}. \\ $$$$ \\ $$$${such}\:{that}\:{the}\:{ball}\:{after}\:{impact}\:{at}\:{A} \\ $$$${continues}\:{to}\:{move}\:{to}\:{B},\:{C}\:{etc}. \\ $$$$\mathrm{tan}\:\alpha={e}\:\mathrm{tan}\:\beta \\ $$$$\frac{\eta\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)}{\lambda−\xi}={e}\:\frac{\eta\left(\mathrm{1}−\mu^{\mathrm{2}} \right)}{\xi−\mu} \\ $$$$\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\left(\xi−\mu\right)={e}\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\left(\lambda−\xi\right) \\ $$$$\xi=\frac{\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\mu+{e}\left(\mathrm{1}−\mu^{\mathrm{2}} \right)\lambda}{\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)+{e}\left(\mathrm{1}−\mu^{\mathrm{2}} \right)}\:\:\:...\left({iii}\right) \\ $$
Commented by mr W last updated on 24/Feb/21

Commented by mr W last updated on 24/Feb/21
Commented by mr W last updated on 24/Feb/21
Commented by mr W last updated on 24/Feb/21

Commented by mr W last updated on 24/Feb/21
Commented by mr W last updated on 24/Feb/21
Commented by mr W last updated on 24/Feb/21
Commented by mr W last updated on 24/Feb/21
Commented by Abdoulaye last updated on 24/Feb/21
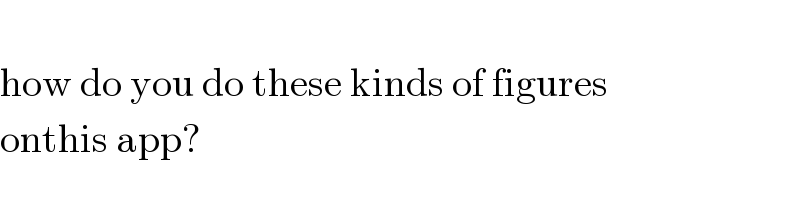
$$ \\ $$$$\mathrm{how}\:\mathrm{do}\:\mathrm{you}\:\mathrm{do}\:\mathrm{these}\:\mathrm{kinds}\:\mathrm{of}\:\mathrm{figures}\: \\ $$$$\mathrm{onthis}\:\mathrm{app}? \\ $$
Commented by mr W last updated on 24/Feb/21
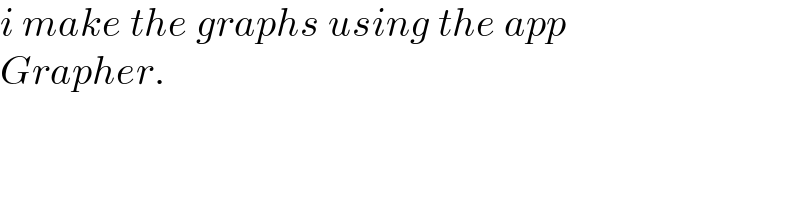
$${i}\:{make}\:{the}\:{graphs}\:{using}\:{the}\:{app} \\ $$$${Grapher}. \\ $$
Commented by Abdoulaye last updated on 24/Feb/21

$${okay}\:{thank}\:{you}! \\ $$
