Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 13929 by ajfour last updated on 25/May/17

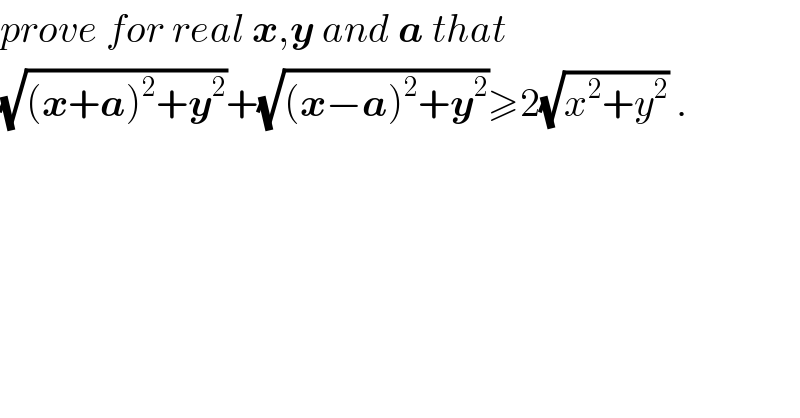
prove for real x,y and a that (√((x+a)^2 +y^2 ))+(√((x−a)^2 +y^2 ))≥2(√(x^2 +y^2 )) .

$${prove}\:{for}\:{real}\:\boldsymbol{{x}},\boldsymbol{{y}}\:{and}\:\boldsymbol{{a}}\:{that} \\ $$$$\sqrt{\left(\boldsymbol{{x}}+\boldsymbol{{a}}\right)^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} }+\sqrt{\left(\boldsymbol{{x}}−\boldsymbol{{a}}\right)^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} }\geqslant\mathrm{2}\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }\:. \\ $$$$ \\ $$

Answered by mrW1 last updated on 25/May/17

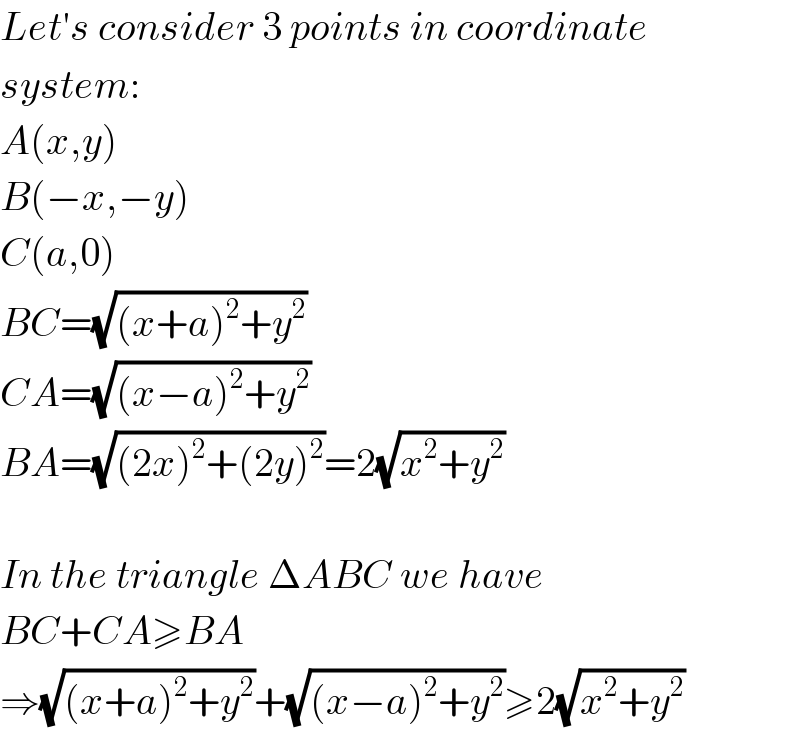
Let′s consider 3 points in coordinate system: A(x,y) B(−x,−y) C(a,0) BC=(√((x+a)^2 +y^2 )) CA=(√((x−a)^2 +y^2 )) BA=(√((2x)^2 +(2y)^2 ))=2(√(x^2 +y^2 )) In the triangle ΔABC we have BC+CA≥BA ⇒(√((x+a)^2 +y^2 ))+(√((x−a)^2 +y^2 ))≥2(√(x^2 +y^2 ))

$${Let}'{s}\:{consider}\:\mathrm{3}\:{points}\:{in}\:{coordinate} \\ $$$${system}: \\ $$$${A}\left({x},{y}\right) \\ $$$${B}\left(−{x},−{y}\right) \\ $$$${C}\left({a},\mathrm{0}\right) \\ $$$${BC}=\sqrt{\left({x}+{a}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$$${CA}=\sqrt{\left({x}−{a}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$$${BA}=\sqrt{\left(\mathrm{2}{x}\right)^{\mathrm{2}} +\left(\mathrm{2}{y}\right)^{\mathrm{2}} }=\mathrm{2}\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$$$ \\ $$$${In}\:{the}\:{triangle}\:\Delta{ABC}\:{we}\:{have} \\ $$$${BC}+{CA}\geqslant{BA} \\ $$$$\Rightarrow\sqrt{\left({x}+{a}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} }+\sqrt{\left({x}−{a}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} }\geqslant\mathrm{2}\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$

Commented by mrW1 last updated on 25/May/17

Commented by ajfour last updated on 25/May/17

Commented by ajfour last updated on 25/May/17

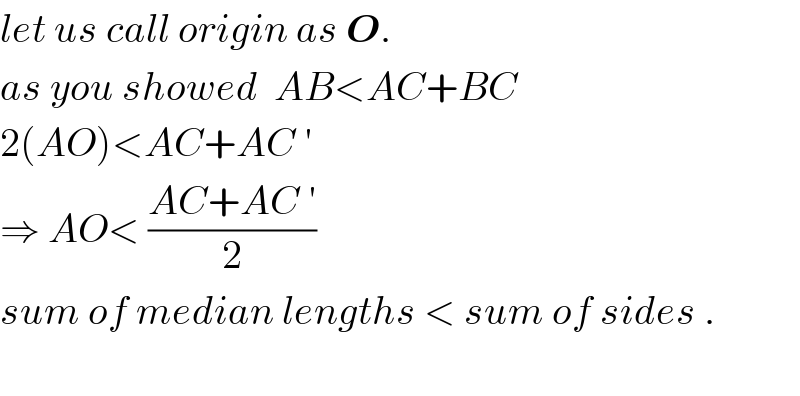
let us call origin as O. as you showed AB<AC+BC 2(AO)<AC+AC ′ ⇒ AO< ((AC+AC ′)/2) sum of median lengths < sum of sides .

$${let}\:{us}\:{call}\:{origin}\:{as}\:\boldsymbol{{O}}. \\ $$$${as}\:{you}\:{showed}\:\:{AB}<{AC}+{BC} \\ $$$$\mathrm{2}\left({AO}\right)<{AC}+{AC}\:' \\ $$$$\Rightarrow\:{AO}<\:\frac{{AC}+{AC}\:'}{\mathrm{2}}\: \\ $$$${sum}\:{of}\:{median}\:{lengths}\:<\:{sum}\:{of}\:{sides}\:. \\ $$$$ \\ $$

Commented by Joel577 last updated on 25/May/17

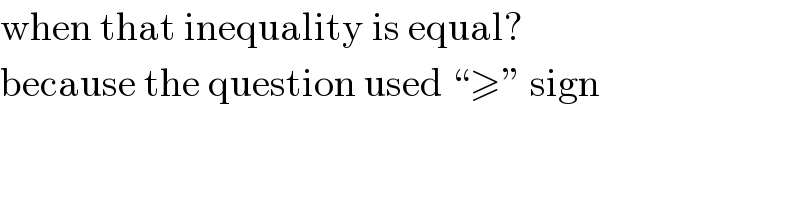
when that inequality is equal? because the question used “≥” sign

$$\mathrm{when}\:\mathrm{that}\:\mathrm{inequality}\:\mathrm{is}\:\mathrm{equal}? \\ $$$$\mathrm{because}\:\mathrm{the}\:\mathrm{question}\:\mathrm{used}\:``\geqslant''\:\mathrm{sign} \\ $$

Commented by ajfour last updated on 25/May/17

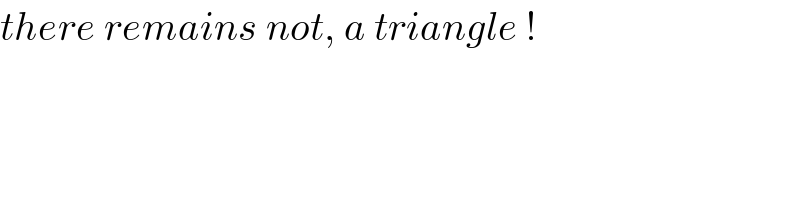
there remains not, a triangle !

$${there}\:{remains}\:{not},\:{a}\:{triangle}\:! \\ $$

Commented by mrW1 last updated on 25/May/17

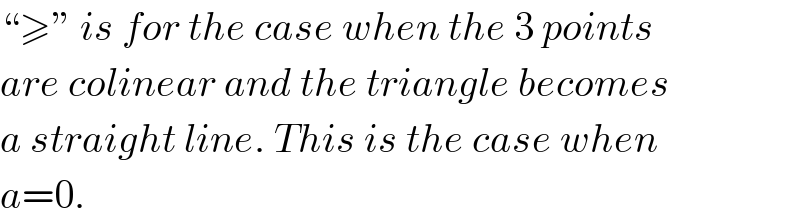
“≥” is for the case when the 3 points are colinear and the triangle becomes a straight line. This is the case when a=0.

$$``\geqslant''\:{is}\:{for}\:{the}\:{case}\:{when}\:{the}\:\mathrm{3}\:{points} \\ $$$${are}\:{colinear}\:{and}\:{the}\:{triangle}\:{becomes} \\ $$$${a}\:{straight}\:{line}.\:{This}\:{is}\:{the}\:{case}\:{when} \\ $$$${a}=\mathrm{0}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com