Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 140896 by bramlexs22 last updated on 14/May/21

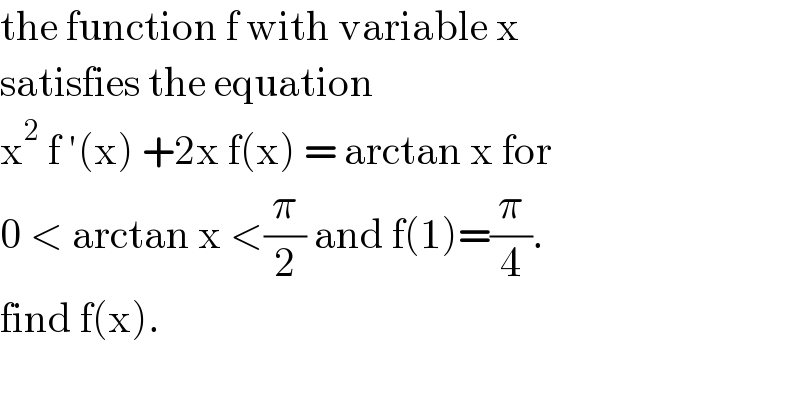
the function f with variable x satisfies the equation x^2 f ′(x) +2x f(x) = arctan x for 0 < arctan x <(π/2) and f(1)=(π/4). find f(x).

$$\mathrm{the}\:\mathrm{function}\:\mathrm{f}\:\mathrm{with}\:\mathrm{variable}\:\mathrm{x} \\ $$ $$\mathrm{satisfies}\:\mathrm{the}\:\mathrm{equation}\: \\ $$ $$\mathrm{x}^{\mathrm{2}} \:\mathrm{f}\:'\left(\mathrm{x}\right)\:+\mathrm{2x}\:\mathrm{f}\left(\mathrm{x}\right)\:=\:\mathrm{arctan}\:\mathrm{x}\:\mathrm{for}\: \\ $$ $$\mathrm{0}\:<\:\mathrm{arctan}\:\mathrm{x}\:<\frac{\pi}{\mathrm{2}}\:\mathrm{and}\:\mathrm{f}\left(\mathrm{1}\right)=\frac{\pi}{\mathrm{4}}. \\ $$ $$\mathrm{find}\:\mathrm{f}\left(\mathrm{x}\right). \\ $$

Answered by EDWIN88 last updated on 15/May/21

let g(x)= x^2 f(x) , diff wrt x give g ′(x)= x^2 f ′(x)+2x f(x) so that g ′(x)= arctan x integration by part g(x)=∫ arctan x dx = x arctan x −(1/2)ln (1+x^2 )+c the condition g(1)= f(1)= (π/4) ⇒(π/4)= arctan 1 −(1/2)ln (2) + c ⇒c = ln (√2) ∴ f(x)= ((g(x))/x^2 ) = ((arctan x )/x)−((ln (√(1+x^2 )))/x^2 ) + ((ln (√2))/x^2 )

$$\mathrm{let}\:\mathrm{g}\left(\mathrm{x}\right)=\:\mathrm{x}^{\mathrm{2}} \mathrm{f}\left(\mathrm{x}\right)\:,\:\mathrm{diff}\:\mathrm{wrt}\:\mathrm{x}\:\mathrm{give} \\ $$ $$\mathrm{g}\:'\left(\mathrm{x}\right)=\:\mathrm{x}^{\mathrm{2}} \:\mathrm{f}\:'\left(\mathrm{x}\right)+\mathrm{2x}\:\mathrm{f}\left(\mathrm{x}\right)\: \\ $$ $$\mathrm{so}\:\mathrm{that}\:\mathrm{g}\:'\left(\mathrm{x}\right)=\:\mathrm{arctan}\:\mathrm{x}\: \\ $$ $$\mathrm{integration}\:\mathrm{by}\:\mathrm{part}\: \\ $$ $$\mathrm{g}\left(\mathrm{x}\right)=\int\:\mathrm{arctan}\:\mathrm{x}\:\mathrm{dx}\:=\:\mathrm{x}\:\mathrm{arctan}\:\mathrm{x}\:−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)+\mathrm{c} \\ $$ $$\mathrm{the}\:\mathrm{condition}\:\mathrm{g}\left(\mathrm{1}\right)=\:\mathrm{f}\left(\mathrm{1}\right)=\:\frac{\pi}{\mathrm{4}} \\ $$ $$\Rightarrow\cancel{\frac{\pi}{\mathrm{4}}}=\:\cancel{\mathrm{arctan}\:\mathrm{1}}\:−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\mathrm{2}\right)\:+\:\mathrm{c} \\ $$ $$\Rightarrow\mathrm{c}\:=\:\mathrm{ln}\:\sqrt{\mathrm{2}}\: \\ $$ $$\therefore\:\mathrm{f}\left(\mathrm{x}\right)=\:\frac{\mathrm{g}\left(\mathrm{x}\right)}{\mathrm{x}^{\mathrm{2}} }\:=\:\frac{\mathrm{arctan}\:\mathrm{x}\:}{\mathrm{x}}−\frac{\mathrm{ln}\:\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }}{\mathrm{x}^{\mathrm{2}} }\:+\:\frac{\mathrm{ln}\:\sqrt{\mathrm{2}}}{\mathrm{x}^{\mathrm{2}} } \\ $$

Answered by mathmax by abdo last updated on 14/May/21

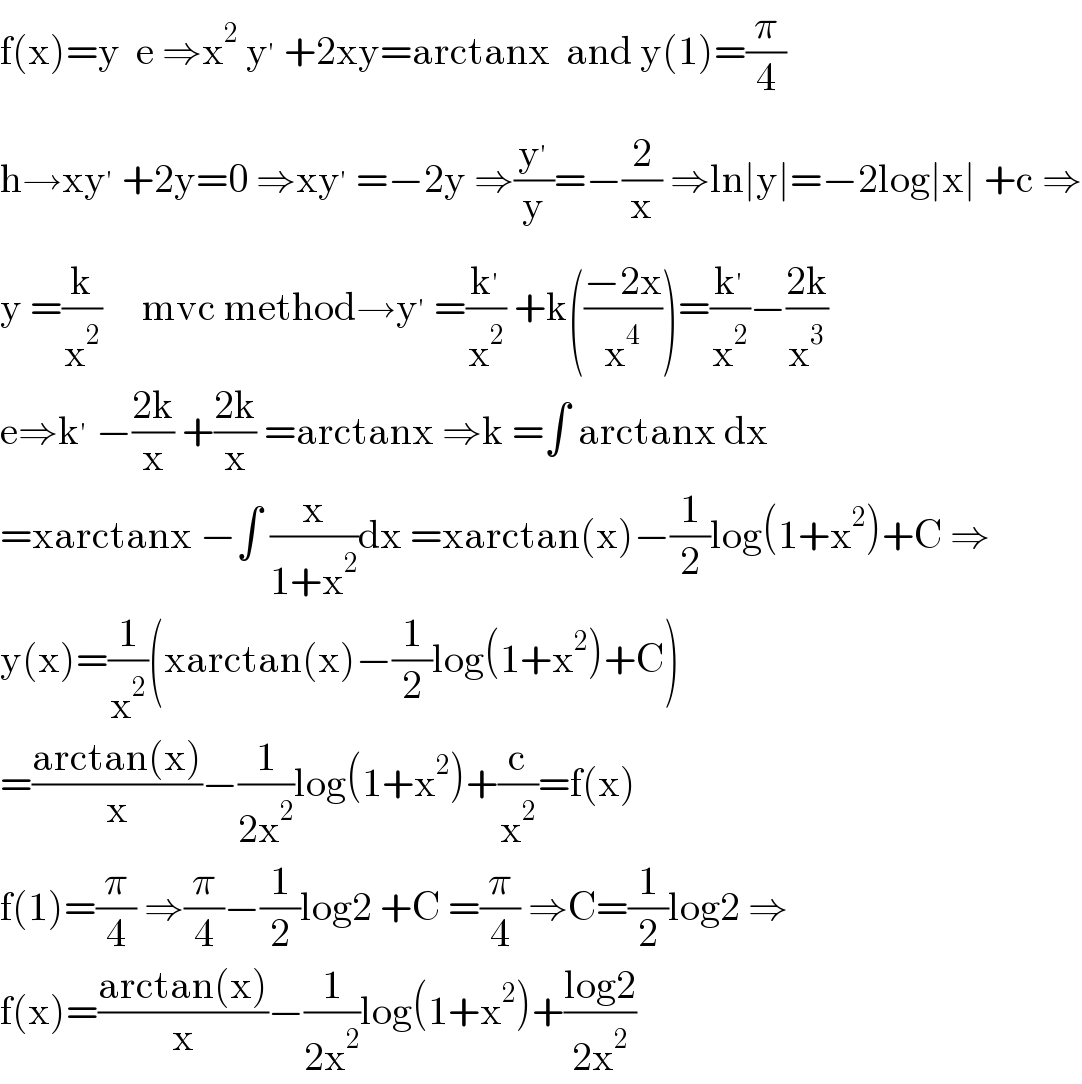
f(x)=y e ⇒x^2 y^′ +2xy=arctanx and y(1)=(π/4) h→xy^′ +2y=0 ⇒xy^′ =−2y ⇒(y^′ /y)=−(2/x) ⇒ln∣y∣=−2log∣x∣ +c ⇒ y =(k/x^2 ) mvc method→y^′ =(k^′ /x^2 ) +k(((−2x)/x^4 ))=(k^′ /x^2 )−((2k)/x^3 ) e⇒k^′ −((2k)/x) +((2k)/x) =arctanx ⇒k =∫ arctanx dx =xarctanx −∫ (x/(1+x^2 ))dx =xarctan(x)−(1/2)log(1+x^2 )+C ⇒ y(x)=(1/x^2 )(xarctan(x)−(1/2)log(1+x^2 )+C) =((arctan(x))/x)−(1/(2x^2 ))log(1+x^2 )+(c/x^2 )=f(x) f(1)=(π/4) ⇒(π/4)−(1/2)log2 +C =(π/4) ⇒C=(1/2)log2 ⇒ f(x)=((arctan(x))/x)−(1/(2x^2 ))log(1+x^2 )+((log2)/(2x^2 ))

$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{y}\:\:\mathrm{e}\:\Rightarrow\mathrm{x}^{\mathrm{2}} \:\mathrm{y}^{'} \:+\mathrm{2xy}=\mathrm{arctanx}\:\:\mathrm{and}\:\mathrm{y}\left(\mathrm{1}\right)=\frac{\pi}{\mathrm{4}} \\ $$ $$\mathrm{h}\rightarrow\mathrm{xy}^{'} \:+\mathrm{2y}=\mathrm{0}\:\Rightarrow\mathrm{xy}^{'} \:=−\mathrm{2y}\:\Rightarrow\frac{\mathrm{y}^{'} }{\mathrm{y}}=−\frac{\mathrm{2}}{\mathrm{x}}\:\Rightarrow\mathrm{ln}\mid\mathrm{y}\mid=−\mathrm{2log}\mid\mathrm{x}\mid\:+\mathrm{c}\:\Rightarrow \\ $$ $$\mathrm{y}\:=\frac{\mathrm{k}}{\mathrm{x}^{\mathrm{2}} }\:\:\:\:\:\mathrm{mvc}\:\mathrm{method}\rightarrow\mathrm{y}^{'} \:=\frac{\mathrm{k}^{'} }{\mathrm{x}^{\mathrm{2}} }\:+\mathrm{k}\left(\frac{−\mathrm{2x}}{\mathrm{x}^{\mathrm{4}} }\right)=\frac{\mathrm{k}^{'} }{\mathrm{x}^{\mathrm{2}} }−\frac{\mathrm{2k}}{\mathrm{x}^{\mathrm{3}} } \\ $$ $$\mathrm{e}\Rightarrow\mathrm{k}^{'} \:−\frac{\mathrm{2k}}{\mathrm{x}}\:+\frac{\mathrm{2k}}{\mathrm{x}}\:=\mathrm{arctanx}\:\Rightarrow\mathrm{k}\:=\int\:\mathrm{arctanx}\:\mathrm{dx} \\ $$ $$=\mathrm{xarctanx}\:−\int\:\frac{\mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:=\mathrm{xarctan}\left(\mathrm{x}\right)−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{log}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)+\mathrm{C}\:\Rightarrow \\ $$ $$\mathrm{y}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }\left(\mathrm{xarctan}\left(\mathrm{x}\right)−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{log}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)+\mathrm{C}\right) \\ $$ $$=\frac{\mathrm{arctan}\left(\mathrm{x}\right)}{\mathrm{x}}−\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} }\mathrm{log}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)+\frac{\mathrm{c}}{\mathrm{x}^{\mathrm{2}} }=\mathrm{f}\left(\mathrm{x}\right) \\ $$ $$\mathrm{f}\left(\mathrm{1}\right)=\frac{\pi}{\mathrm{4}}\:\Rightarrow\frac{\pi}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{log2}\:+\mathrm{C}\:=\frac{\pi}{\mathrm{4}}\:\Rightarrow\mathrm{C}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{log2}\:\Rightarrow \\ $$ $$\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{arctan}\left(\mathrm{x}\right)}{\mathrm{x}}−\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} }\mathrm{log}\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)+\frac{\mathrm{log2}}{\mathrm{2x}^{\mathrm{2}} } \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com