Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 141914 by mnjuly1970 last updated on 24/May/21

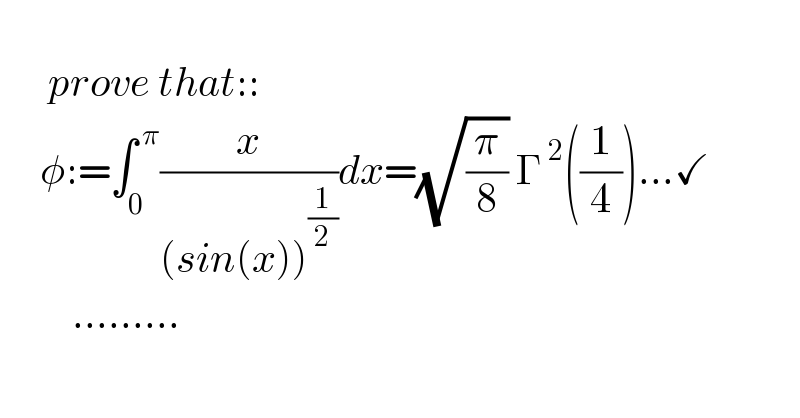
prove that:: φ:=∫_0 ^( π) (x/((sin(x))^(1/2) ))dx=(√(π/8)) Γ^( 2) ((1/4))...✓ .........

$$\:\:\:\: \\ $$$$\:\:\:\:\:\:{prove}\:{that}:: \\ $$$$\:\:\:\:\:\phi:=\int_{\mathrm{0}} ^{\:\pi} \frac{{x}}{\left({sin}\left({x}\right)\right)^{\frac{\mathrm{1}}{\mathrm{2}}} }{dx}=\sqrt{\frac{\pi}{\mathrm{8}}}\:\Gamma^{\:\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)...\checkmark \\ $$$$\:\:\:\:\:\:\:\:\:......... \\ $$

Answered by qaz last updated on 24/May/21

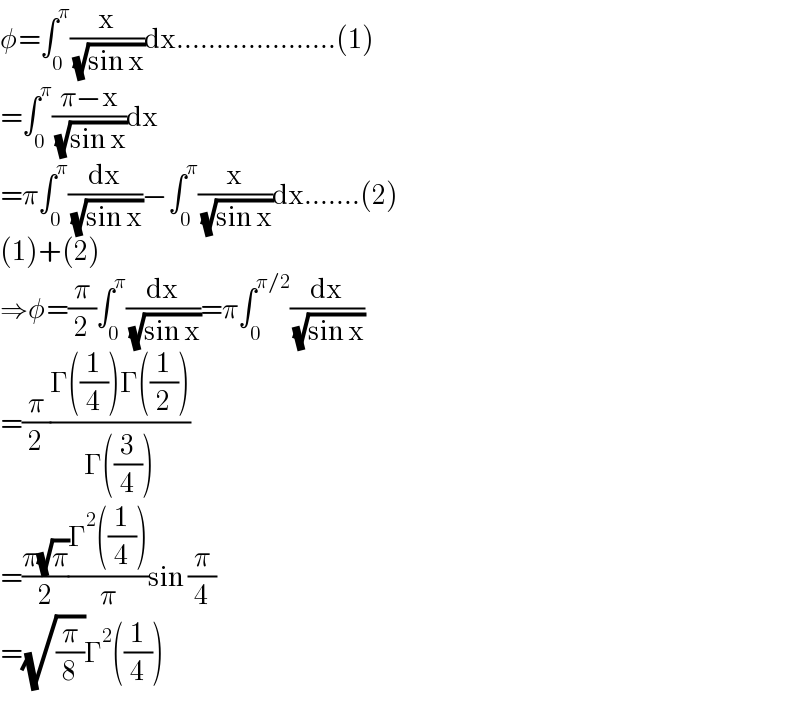
φ=∫_0 ^π (x/( (√(sin x))))dx....................(1) =∫_0 ^π ((π−x)/( (√(sin x))))dx =π∫_0 ^π (dx/( (√(sin x))))−∫_0 ^π (x/( (√(sin x))))dx.......(2) (1)+(2) ⇒φ=(π/2)∫_0 ^π (dx/( (√(sin x))))=π∫_0 ^(π/2) (dx/( (√(sin x)))) =(π/2)((Γ((1/4))Γ((1/2)))/(Γ((3/4)))) =((π(√π))/2)((Γ^2 ((1/4)))/π)sin (π/4) =(√(π/8))Γ^2 ((1/4))

$$\phi=\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{x}}{\:\sqrt{\mathrm{sin}\:\mathrm{x}}}\mathrm{dx}....................\left(\mathrm{1}\right) \\ $$$$=\int_{\mathrm{0}} ^{\pi} \frac{\pi−\mathrm{x}}{\:\sqrt{\mathrm{sin}\:\mathrm{x}}}\mathrm{dx} \\ $$$$=\pi\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{dx}}{\:\sqrt{\mathrm{sin}\:\mathrm{x}}}−\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{x}}{\:\sqrt{\mathrm{sin}\:\mathrm{x}}}\mathrm{dx}.......\left(\mathrm{2}\right) \\ $$$$\left(\mathrm{1}\right)+\left(\mathrm{2}\right) \\ $$$$\Rightarrow\phi=\frac{\pi}{\mathrm{2}}\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{dx}}{\:\sqrt{\mathrm{sin}\:\mathrm{x}}}=\pi\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \frac{\mathrm{dx}}{\:\sqrt{\mathrm{sin}\:\mathrm{x}}} \\ $$$$=\frac{\pi}{\mathrm{2}}\frac{\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)} \\ $$$$=\frac{\pi\sqrt{\pi}}{\mathrm{2}}\frac{\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)}{\pi}\mathrm{sin}\:\frac{\pi}{\mathrm{4}} \\ $$$$=\sqrt{\frac{\pi}{\mathrm{8}}}\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right) \\ $$

Answered by Dwaipayan Shikari last updated on 24/May/21

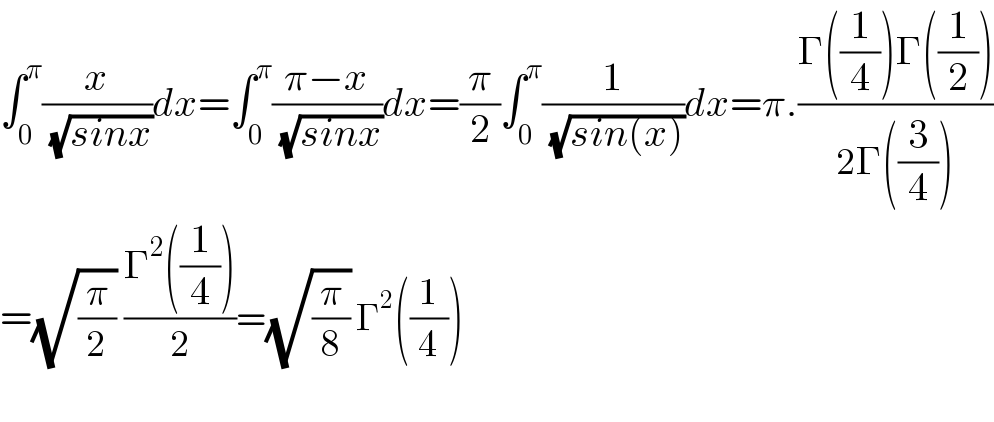
∫_0 ^π (x/( (√(sinx))))dx=∫_0 ^π ((π−x)/( (√(sinx))))dx=(π/2)∫_0 ^π (1/( (√(sin(x)))))dx=π.((Γ((1/4))Γ((1/2)))/(2Γ((3/4)))) =(√(π/2)) ((Γ^2 ((1/4)))/2)=(√(π/8)) Γ^2 ((1/4))

$$\int_{\mathrm{0}} ^{\pi} \frac{{x}}{\:\sqrt{{sinx}}}{dx}=\int_{\mathrm{0}} ^{\pi} \frac{\pi−{x}}{\:\sqrt{{sinx}}}{dx}=\frac{\pi}{\mathrm{2}}\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{1}}{\:\sqrt{{sin}\left({x}\right)}}{dx}=\pi.\frac{\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\mathrm{2}\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)} \\ $$$$=\sqrt{\frac{\pi}{\mathrm{2}}}\:\frac{\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)}{\mathrm{2}}=\sqrt{\frac{\pi}{\mathrm{8}}}\:\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right) \\ $$$$ \\ $$

Answered by mathmax by abdo last updated on 24/May/21

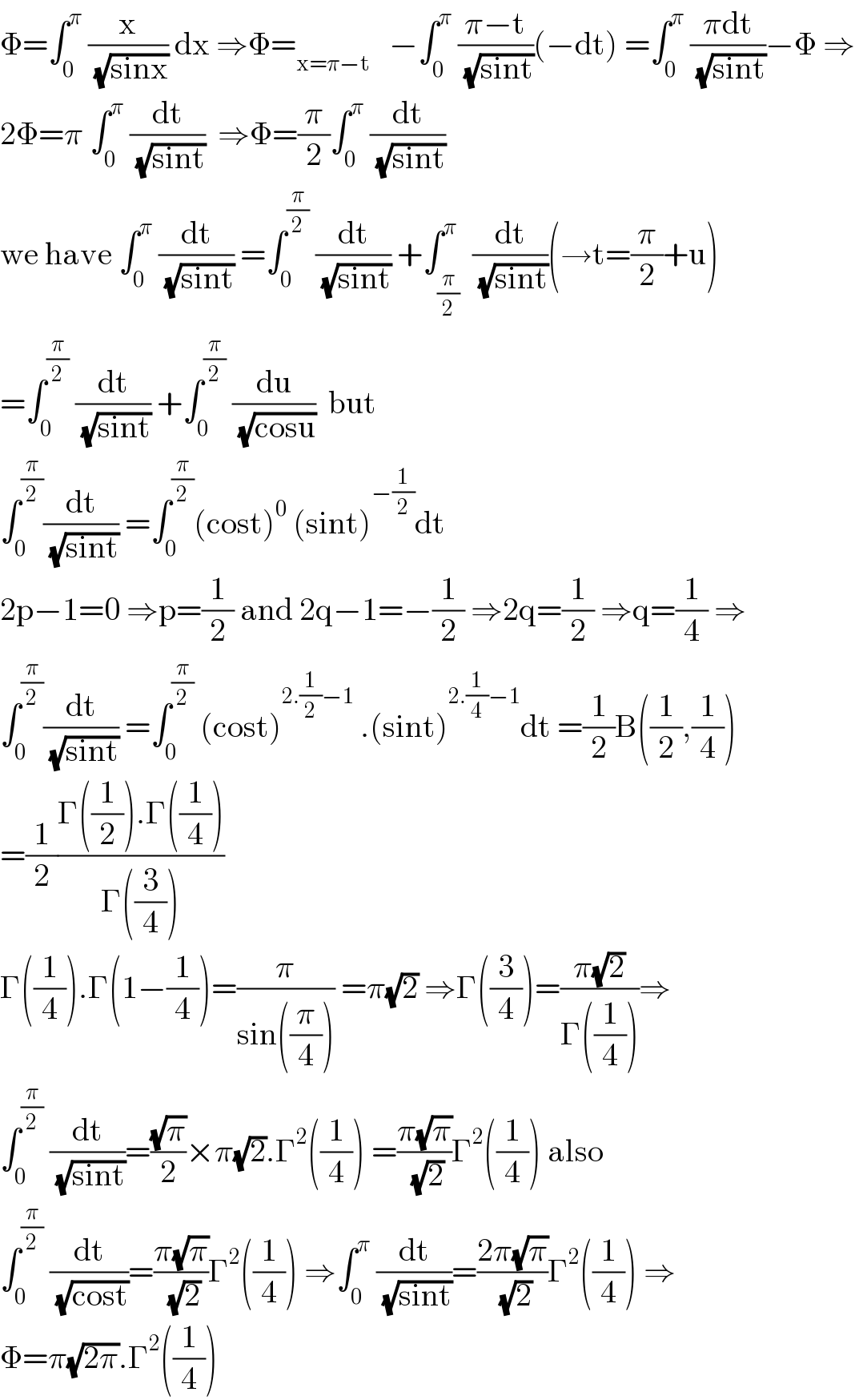
Φ=∫_0 ^π (x/( (√(sinx)))) dx ⇒Φ=_(x=π−t) −∫_0 ^π ((π−t)/( (√(sint))))(−dt) =∫_0 ^π ((πdt)/( (√(sint))))−Φ ⇒ 2Φ=π ∫_0 ^π (dt/( (√(sint)))) ⇒Φ=(π/2)∫_0 ^π (dt/( (√(sint)))) we have ∫_0 ^π (dt/( (√(sint)))) =∫_0 ^(π/2) (dt/( (√(sint)))) +∫_(π/2) ^π (dt/( (√(sint))))(→t=(π/2)+u) =∫_0 ^(π/2) (dt/( (√(sint)))) +∫_0 ^(π/2) (du/( (√(cosu)))) but ∫_0 ^(π/2) (dt/( (√(sint)))) =∫_0 ^(π/2) (cost)^0 (sint)^(−(1/2)) dt 2p−1=0 ⇒p=(1/2) and 2q−1=−(1/2) ⇒2q=(1/2) ⇒q=(1/4) ⇒ ∫_0 ^(π/2) (dt/( (√(sint)))) =∫_0 ^(π/2) (cost)^(2.(1/2)−1) .(sint)^(2.(1/4)−1) dt =(1/2)B((1/2),(1/4)) =(1/2)((Γ((1/2)).Γ((1/4)))/(Γ((3/4)))) Γ((1/4)).Γ(1−(1/4))=(π/(sin((π/4)))) =π(√2) ⇒Γ((3/4))=((π(√2))/(Γ((1/4))))⇒ ∫_0 ^(π/2) (dt/( (√(sint))))=((√π)/2)×π(√2).Γ^2 ((1/4)) =((π(√π))/( (√2)))Γ^2 ((1/4)) also ∫_0 ^(π/2) (dt/( (√(cost))))=((π(√π))/( (√2)))Γ^2 ((1/4)) ⇒∫_0 ^π (dt/( (√(sint))))=((2π(√π))/( (√2)))Γ^2 ((1/4)) ⇒ Φ=π(√(2π)).Γ^2 ((1/4))

$$\Phi=\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{x}}{\:\sqrt{\mathrm{sinx}}}\:\mathrm{dx}\:\Rightarrow\Phi=_{\mathrm{x}=\pi−\mathrm{t}} \:\:\:−\int_{\mathrm{0}} ^{\pi} \:\frac{\pi−\mathrm{t}}{\:\sqrt{\mathrm{sint}}}\left(−\mathrm{dt}\right)\:=\int_{\mathrm{0}} ^{\pi} \:\frac{\pi\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}−\Phi\:\Rightarrow \\ $$$$\mathrm{2}\Phi=\pi\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}\:\:\Rightarrow\Phi=\frac{\pi}{\mathrm{2}}\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}} \\ $$$$\mathrm{we}\:\mathrm{have}\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}\:+\int_{\frac{\pi}{\mathrm{2}}} ^{\pi} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}\left(\rightarrow\mathrm{t}=\frac{\pi}{\mathrm{2}}+\mathrm{u}\right) \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}\:+\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{du}}{\:\sqrt{\mathrm{cosu}}}\:\:\mathrm{but} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{cost}\right)^{\mathrm{0}} \:\left(\mathrm{sint}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \mathrm{dt} \\ $$$$\mathrm{2p}−\mathrm{1}=\mathrm{0}\:\Rightarrow\mathrm{p}=\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{and}\:\mathrm{2q}−\mathrm{1}=−\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\mathrm{2q}=\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\mathrm{q}=\frac{\mathrm{1}}{\mathrm{4}}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\left(\mathrm{cost}\right)^{\mathrm{2}.\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} \:.\left(\mathrm{sint}\right)^{\mathrm{2}.\frac{\mathrm{1}}{\mathrm{4}}−\mathrm{1}} \mathrm{dt}\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{B}\left(\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{4}}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\frac{\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right).\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)}{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)} \\ $$$$\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right).\Gamma\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4}}\right)=\frac{\pi}{\mathrm{sin}\left(\frac{\pi}{\mathrm{4}}\right)}\:=\pi\sqrt{\mathrm{2}}\:\Rightarrow\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)=\frac{\pi\sqrt{\mathrm{2}}}{\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)}\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}=\frac{\sqrt{\pi}}{\mathrm{2}}×\pi\sqrt{\mathrm{2}}.\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)\:=\frac{\pi\sqrt{\pi}}{\:\sqrt{\mathrm{2}}}\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)\:\mathrm{also} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{cost}}}=\frac{\pi\sqrt{\pi}}{\:\sqrt{\mathrm{2}}}\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)\:\Rightarrow\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{sint}}}=\frac{\mathrm{2}\pi\sqrt{\pi}}{\:\sqrt{\mathrm{2}}}\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)\:\Rightarrow \\ $$$$\Phi=\pi\sqrt{\mathrm{2}\pi}.\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com