Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 143339 by bramlexs22 last updated on 13/Jun/21

Commented by justtry last updated on 13/Jun/21

maybe 0

$${maybe}\:\mathrm{0} \\ $$

Answered by mathmax by abdo last updated on 13/Jun/21

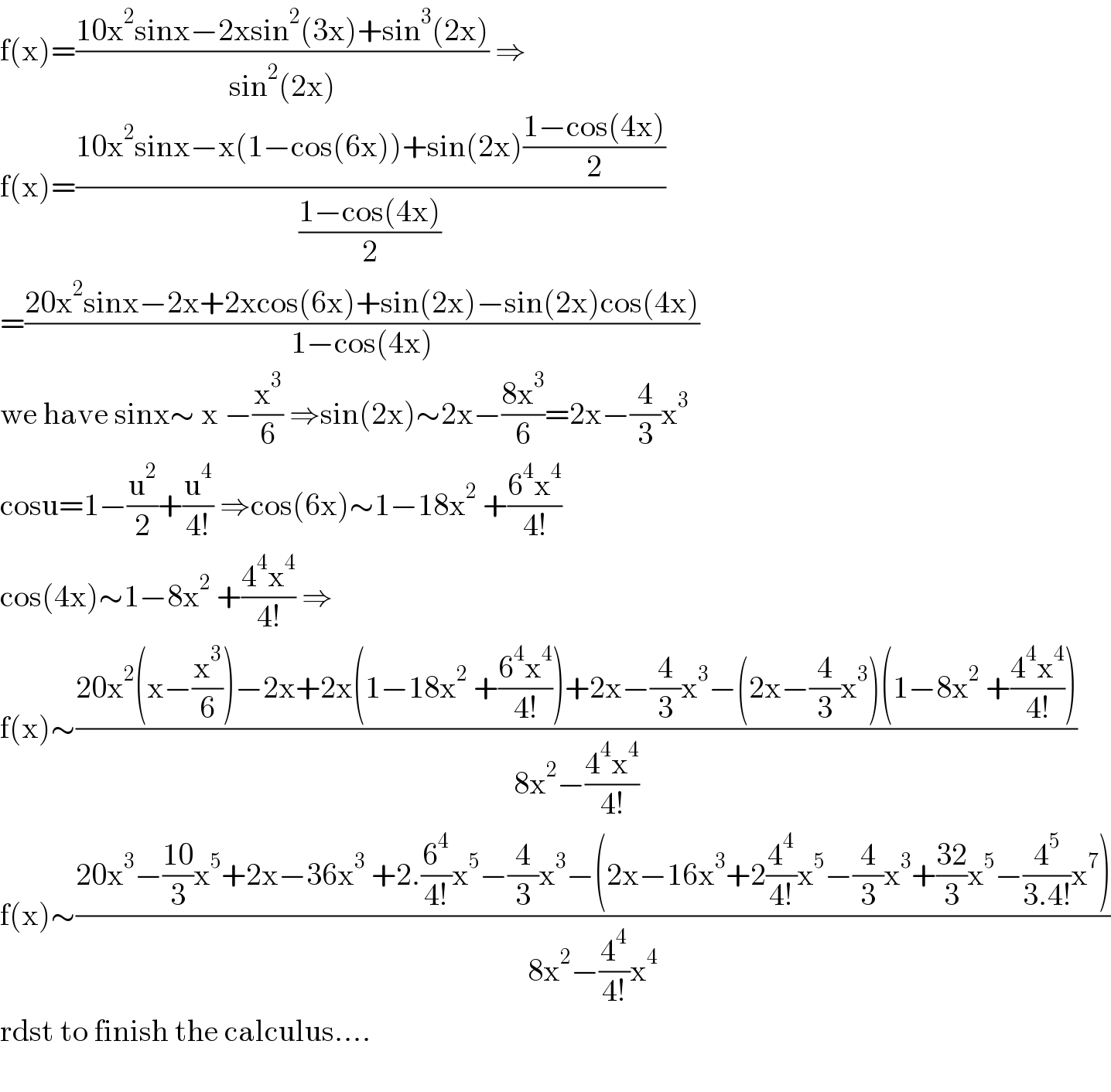
f(x)=((10x^2 sinx−2xsin^2 (3x)+sin^3 (2x))/(sin^2 (2x))) ⇒ f(x)=((10x^2 sinx−x(1−cos(6x))+sin(2x)((1−cos(4x))/2))/((1−cos(4x))/2)) =((20x^2 sinx−2x+2xcos(6x)+sin(2x)−sin(2x)cos(4x))/(1−cos(4x))) we have sinx∼ x −(x^3 /6) ⇒sin(2x)∼2x−((8x^3 )/6)=2x−(4/3)x^3 cosu=1−(u^2 /2)+(u^4 /(4!)) ⇒cos(6x)∼1−18x^2 +((6^4 x^4 )/(4!)) cos(4x)∼1−8x^2 +((4^4 x^4 )/(4!)) ⇒ f(x)∼((20x^2 (x−(x^3 /6))−2x+2x(1−18x^2 +((6^4 x^4 )/(4!)))+2x−(4/3)x^3 −(2x−(4/3)x^3 )(1−8x^2 +((4^4 x^4 )/(4!))))/(8x^2 −((4^4 x^4 )/(4!)))) f(x)∼((20x^3 −((10)/3)x^5 +2x−36x^3 +2.(6^4 /(4!))x^5 −(4/3)x^3 −(2x−16x^3 +2(4^4 /(4!))x^5 −(4/3)x^3 +((32)/3)x^5 −(4^5 /(3.4!))x^7 ))/(8x^2 −(4^4 /(4!))x^4 )) rdst to finish the calculus....

$$\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{10x}^{\mathrm{2}} \mathrm{sinx}−\mathrm{2xsin}^{\mathrm{2}} \left(\mathrm{3x}\right)+\mathrm{sin}^{\mathrm{3}} \left(\mathrm{2x}\right)}{\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2x}\right)}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{10x}^{\mathrm{2}} \mathrm{sinx}−\mathrm{x}\left(\mathrm{1}−\mathrm{cos}\left(\mathrm{6x}\right)\right)+\mathrm{sin}\left(\mathrm{2x}\right)\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{2}}}{\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{2}}} \\ $$$$=\frac{\mathrm{20x}^{\mathrm{2}} \mathrm{sinx}−\mathrm{2x}+\mathrm{2xcos}\left(\mathrm{6x}\right)+\mathrm{sin}\left(\mathrm{2x}\right)−\mathrm{sin}\left(\mathrm{2x}\right)\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{1}−\mathrm{cos}\left(\mathrm{4x}\right)} \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{sinx}\sim\:\mathrm{x}\:−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}\:\Rightarrow\mathrm{sin}\left(\mathrm{2x}\right)\sim\mathrm{2x}−\frac{\mathrm{8x}^{\mathrm{3}} }{\mathrm{6}}=\mathrm{2x}−\frac{\mathrm{4}}{\mathrm{3}}\mathrm{x}^{\mathrm{3}} \\ $$$$\mathrm{cosu}=\mathrm{1}−\frac{\mathrm{u}^{\mathrm{2}} }{\mathrm{2}}+\frac{\mathrm{u}^{\mathrm{4}} }{\mathrm{4}!}\:\Rightarrow\mathrm{cos}\left(\mathrm{6x}\right)\sim\mathrm{1}−\mathrm{18x}^{\mathrm{2}} \:+\frac{\mathrm{6}^{\mathrm{4}} \mathrm{x}^{\mathrm{4}} }{\mathrm{4}!} \\ $$$$\mathrm{cos}\left(\mathrm{4x}\right)\sim\mathrm{1}−\mathrm{8x}^{\mathrm{2}} \:+\frac{\mathrm{4}^{\mathrm{4}} \mathrm{x}^{\mathrm{4}} }{\mathrm{4}!}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\sim\frac{\mathrm{20x}^{\mathrm{2}} \left(\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}\right)−\mathrm{2x}+\mathrm{2x}\left(\mathrm{1}−\mathrm{18x}^{\mathrm{2}} \:+\frac{\mathrm{6}^{\mathrm{4}} \mathrm{x}^{\mathrm{4}} }{\mathrm{4}!}\right)+\mathrm{2x}−\frac{\mathrm{4}}{\mathrm{3}}\mathrm{x}^{\mathrm{3}} −\left(\mathrm{2x}−\frac{\mathrm{4}}{\mathrm{3}}\mathrm{x}^{\mathrm{3}} \right)\left(\mathrm{1}−\mathrm{8x}^{\mathrm{2}} \:+\frac{\mathrm{4}^{\mathrm{4}} \mathrm{x}^{\mathrm{4}} }{\mathrm{4}!}\right)}{\mathrm{8x}^{\mathrm{2}} −\frac{\mathrm{4}^{\mathrm{4}} \mathrm{x}^{\mathrm{4}} }{\mathrm{4}!}} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\sim\frac{\mathrm{20x}^{\mathrm{3}} −\frac{\mathrm{10}}{\mathrm{3}}\mathrm{x}^{\mathrm{5}} +\mathrm{2x}−\mathrm{36x}^{\mathrm{3}} \:+\mathrm{2}.\frac{\mathrm{6}^{\mathrm{4}} }{\mathrm{4}!}\mathrm{x}^{\mathrm{5}} −\frac{\mathrm{4}}{\mathrm{3}}\mathrm{x}^{\mathrm{3}} −\left(\mathrm{2x}−\mathrm{16x}^{\mathrm{3}} +\mathrm{2}\frac{\mathrm{4}^{\mathrm{4}} }{\mathrm{4}!}\mathrm{x}^{\mathrm{5}} −\frac{\mathrm{4}}{\mathrm{3}}\mathrm{x}^{\mathrm{3}} +\frac{\mathrm{32}}{\mathrm{3}}\mathrm{x}^{\mathrm{5}} −\frac{\mathrm{4}^{\mathrm{5}} }{\mathrm{3}.\mathrm{4}!}\mathrm{x}^{\mathrm{7}} \right)}{\mathrm{8x}^{\mathrm{2}} −\frac{\mathrm{4}^{\mathrm{4}} }{\mathrm{4}!}\mathrm{x}^{\mathrm{4}} } \\ $$$$\mathrm{rdst}\:\mathrm{to}\:\mathrm{finish}\:\mathrm{the}\:\mathrm{calculus}.... \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com