Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 144439 by mathdanisur last updated on 25/Jun/21

Commented by Rasheed.Sindhi last updated on 25/Jun/21

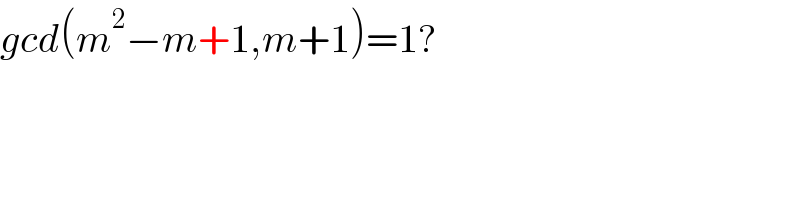
gcd(m^2 −m+1,m+1)=1?

$${gcd}\left({m}^{\mathrm{2}} −{m}+\mathrm{1},{m}+\mathrm{1}\right)=\mathrm{1}? \\ $$

Commented by mathdanisur last updated on 25/Jun/21

sorry Sir, yes +1

$${sorry}\:{Sir},\:{yes}\:+\mathrm{1} \\ $$

Commented by mathmax by abdo last updated on 25/Jun/21

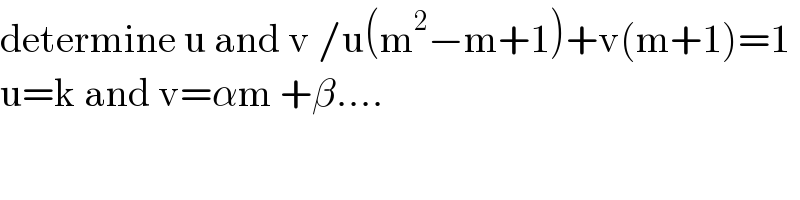
determine u and v /u(m^2 −m+1)+v(m+1)=1 u=k and v=αm +β....

$$\mathrm{determine}\:\mathrm{u}\:\mathrm{and}\:\mathrm{v}\:/\mathrm{u}\left(\mathrm{m}^{\mathrm{2}} −\mathrm{m}+\mathrm{1}\right)+\mathrm{v}\left(\mathrm{m}+\mathrm{1}\right)=\mathrm{1} \\ $$$$\mathrm{u}=\mathrm{k}\:\mathrm{and}\:\mathrm{v}=\alpha\mathrm{m}\:+\beta.... \\ $$

Commented by Rasheed.Sindhi last updated on 26/Jun/21

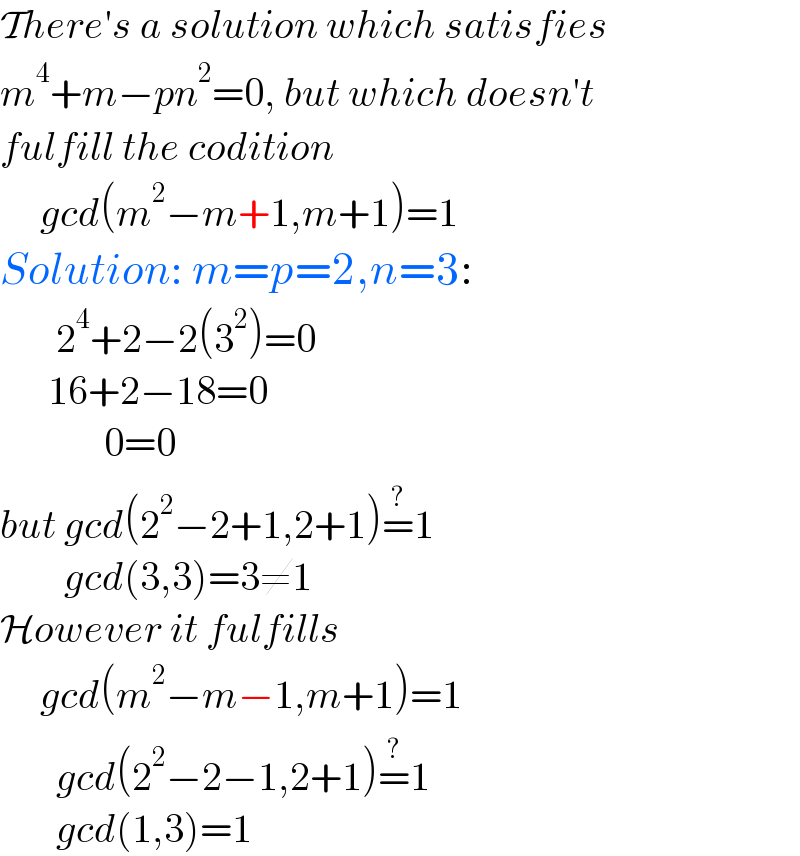
There′s a solution which satisfies m^4 +m−pn^2 =0, but which doesn′t fulfill the codition gcd(m^2 −m+1,m+1)=1 Solution: m=p=2,n=3: 2^4 +2−2(3^2 )=0 16+2−18=0 0=0 but gcd(2^2 −2+1,2+1)=^(?) 1 gcd(3,3)=3≠1 However it fulfills gcd(m^2 −m−1,m+1)=1 gcd(2^2 −2−1,2+1)=^(?) 1 gcd(1,3)=1

$$\mathcal{T}{here}'{s}\:{a}\:{solution}\:{which}\:{satisfies} \\ $$$${m}^{\mathrm{4}} +{m}−{pn}^{\mathrm{2}} =\mathrm{0},\:{but}\:{which}\:{doesn}'{t} \\ $$$${fulfill}\:{the}\:{codition} \\ $$$$\:\:\:\:\:{gcd}\left({m}^{\mathrm{2}} −{m}+\mathrm{1},{m}+\mathrm{1}\right)=\mathrm{1} \\ $$$${Solution}:\:{m}={p}=\mathrm{2},{n}=\mathrm{3}: \\ $$$$\:\:\:\:\:\:\:\mathrm{2}^{\mathrm{4}} +\mathrm{2}−\mathrm{2}\left(\mathrm{3}^{\mathrm{2}} \right)=\mathrm{0} \\ $$$$\:\:\:\:\:\:\mathrm{16}+\mathrm{2}−\mathrm{18}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{0}=\mathrm{0} \\ $$$${but}\:{gcd}\left(\mathrm{2}^{\mathrm{2}} −\mathrm{2}+\mathrm{1},\mathrm{2}+\mathrm{1}\right)\overset{?} {=}\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:{gcd}\left(\mathrm{3},\mathrm{3}\right)=\mathrm{3}\neq\mathrm{1} \\ $$$$\mathcal{H}{owever}\:{it}\:{fulfills} \\ $$$$\:\:\:\:\:{gcd}\left({m}^{\mathrm{2}} −{m}−\mathrm{1},{m}+\mathrm{1}\right)=\mathrm{1} \\ $$$$\:\:\:\:\:\:\:{gcd}\left(\mathrm{2}^{\mathrm{2}} −\mathrm{2}−\mathrm{1},\mathrm{2}+\mathrm{1}\right)\overset{?} {=}\mathrm{1} \\ $$$$\:\:\:\:\:\:\:{gcd}\left(\mathrm{1},\mathrm{3}\right)=\mathrm{1} \\ $$

Answered by Rasheed.Sindhi last updated on 26/Jun/21

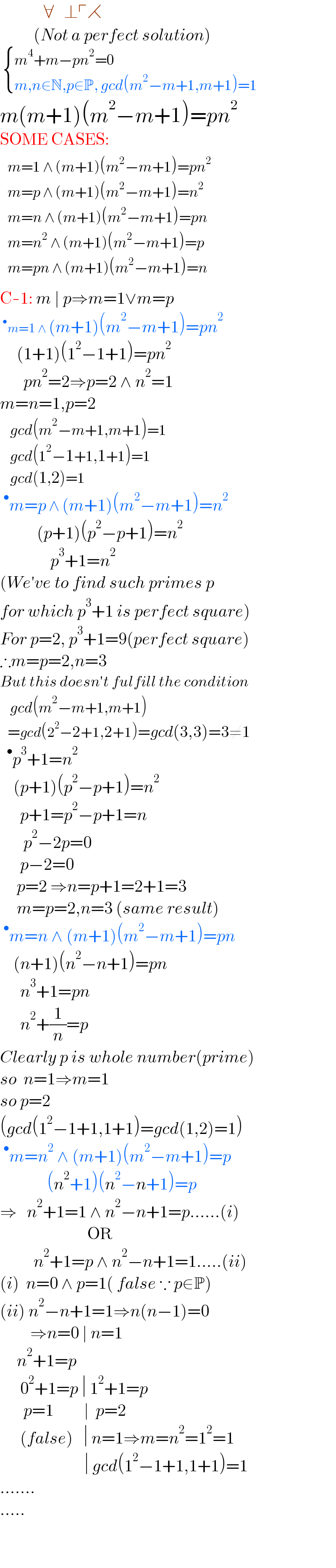
∀ ⊥┌⋌ (Not a perfect solution) { ((m^4 +m−pn^2 =0)),((m,n∈N,p∈P, gcd(m^2 −m+1,m+1)=1)) :} m(m+1)(m^2 −m+1)=pn^2 SOME CASES: determinant (((m=1 ∧ (m+1)(m^2 −m+1)=pn^2 )),((m=p ∧ (m+1)(m^2 −m+1)=n^2 )),((m=n ∧ (m+1)(m^2 −m+1)=pn)),((m=n^2 ∧ (m+1)(m^2 −m+1)=p)),((m=pn ∧ (m+1)(m^2 −m+1)=n))) C-1: m ∣ p⇒m=1∨m=p ^• m=1 ∧ (m+1)(m^2 −m+1)=pn^2 (1+1)(1^2 −1+1)=pn^2 pn^2 =2⇒p=2 ∧ n^2 =1 m=n=1,p=2 gcd(m^2 −m+1,m+1)=1 gcd(1^2 −1+1,1+1)=1 gcd(1,2)=1 ^• m=p ∧ (m+1)(m^2 −m+1)=n^2 (p+1)(p^2 −p+1)=n^2 p^3 +1=n^2 (We′ve to find such primes p for which p^3 +1 is perfect square) For p=2, p^3 +1=9(perfect square) ∴m=p=2,n=3 But this doesn′t fulfill the condition gcd(m^2 −m+1,m+1) =gcd(2^2 −2+1,2+1)=gcd(3,3)=3≠1 ^• p^3 +1=n^2 (p+1)(p^2 −p+1)=n^2 p+1=p^2 −p+1=n p^2 −2p=0 p−2=0 p=2 ⇒n=p+1=2+1=3 m=p=2,n=3 (same result) ^• m=n ∧ (m+1)(m^2 −m+1)=pn (n+1)(n^2 −n+1)=pn n^3 +1=pn n^2 +(1/n)=p Clearly p is whole number(prime) so n=1⇒m=1 so p=2 (gcd(1^2 −1+1,1+1)=gcd(1,2)=1) ^• m=n^2 ∧ (m+1)(m^2 −m+1)=p (n^2 +1)(n^2 −n+1)=p ⇒ n^2 +1=1 ∧ n^2 −n+1=p......(i) OR n^2 +1=p ∧ n^2 −n+1=1.....(ii) (i) n=0 ∧ p=1( false ∵ p∈P) (ii) n^2 −n+1=1⇒n(n−1)=0 ⇒n=0 ∣ n=1 n^2 +1=p 0^2 +1=p ∣ 1^2 +1=p p=1 ∣ p=2 (false) ∣ n=1⇒m=n^2 =1^2 =1 ∣ gcd(1^2 −1+1,1+1)=1 ....... .....

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\forall\:\:\:\bot\ulcorner\rightthreetimes \\ $$$$\:\:\:\:\:\:\:\:\:\:\left({Not}\:{a}\:{perfect}\:{solution}\right) \\ $$$$\:\begin{cases}{{m}^{\mathrm{4}} +{m}−{pn}^{\mathrm{2}} =\mathrm{0}}\\{{m},{n}\in\mathbb{N},{p}\in\mathbb{P},\:{gcd}\left({m}^{\mathrm{2}} −{m}+\mathrm{1},{m}+\mathrm{1}\right)=\mathrm{1}}\end{cases} \\ $$$${m}\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={pn}^{\mathrm{2}} \\ $$$$\mathrm{SOME}\:\mathrm{CASES}: \\ $$$$\begin{matrix}{{m}=\mathrm{1}\:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={pn}^{\mathrm{2}} }\\{{m}={p}\:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={n}^{\mathrm{2}} }\\{{m}={n}\:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={pn}}\\{{m}={n}^{\mathrm{2}} \:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={p}}\\{{m}={pn}\:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={n}}\end{matrix} \\ $$$$\mathrm{C}-\mathrm{1}:\:{m}\:\mid\:{p}\Rightarrow{m}=\mathrm{1}\vee{m}={p} \\ $$$$\:^{\bullet} {m}=\mathrm{1}\:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={pn}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}^{\mathrm{2}} −\mathrm{1}+\mathrm{1}\right)={pn}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:{pn}^{\mathrm{2}} =\mathrm{2}\Rightarrow{p}=\mathrm{2}\:\wedge\:{n}^{\mathrm{2}} =\mathrm{1} \\ $$$${m}={n}=\mathrm{1},{p}=\mathrm{2} \\ $$$$\:\:\:{gcd}\left({m}^{\mathrm{2}} −{m}+\mathrm{1},{m}+\mathrm{1}\right)=\mathrm{1} \\ $$$$\:\:\:{gcd}\left(\mathrm{1}^{\mathrm{2}} −\mathrm{1}+\mathrm{1},\mathrm{1}+\mathrm{1}\right)=\mathrm{1} \\ $$$$\:\:\:{gcd}\left(\mathrm{1},\mathrm{2}\right)=\mathrm{1} \\ $$$$\:^{\bullet} {m}={p}\:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={n}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\left({p}+\mathrm{1}\right)\left({p}^{\mathrm{2}} −{p}+\mathrm{1}\right)={n}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{p}^{\mathrm{3}} +\mathrm{1}={n}^{\mathrm{2}} \\ $$$$\left({We}'{ve}\:{to}\:{find}\:{such}\:{primes}\:{p}\right. \\ $$$$\left.{for}\:{which}\:{p}^{\mathrm{3}} +\mathrm{1}\:{is}\:{perfect}\:{square}\right) \\ $$$${For}\:{p}=\mathrm{2},\:{p}^{\mathrm{3}} +\mathrm{1}=\mathrm{9}\left({perfect}\:{square}\right) \\ $$$$\therefore{m}={p}=\mathrm{2},{n}=\mathrm{3} \\ $$$${But}\:{this}\:{doesn}'{t}\:{fulfill}\:{the}\:{condition} \\ $$$$\:\:\:{gcd}\left({m}^{\mathrm{2}} −{m}+\mathrm{1},{m}+\mathrm{1}\right) \\ $$$$\:\:\:={gcd}\left(\mathrm{2}^{\mathrm{2}} −\mathrm{2}+\mathrm{1},\mathrm{2}+\mathrm{1}\right)={gcd}\left(\mathrm{3},\mathrm{3}\right)=\mathrm{3}\neq\mathrm{1} \\ $$$$\:\:^{\bullet} {p}^{\mathrm{3}} +\mathrm{1}={n}^{\mathrm{2}} \\ $$$$\:\:\:\:\left({p}+\mathrm{1}\right)\left({p}^{\mathrm{2}} −{p}+\mathrm{1}\right)={n}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:{p}+\mathrm{1}={p}^{\mathrm{2}} −{p}+\mathrm{1}={n} \\ $$$$\:\:\:\:\:\:\:{p}^{\mathrm{2}} −\mathrm{2}{p}=\mathrm{0} \\ $$$$\:\:\:\:\:\:{p}−\mathrm{2}=\mathrm{0} \\ $$$$\:\:\:\:\:{p}=\mathrm{2}\:\Rightarrow{n}={p}+\mathrm{1}=\mathrm{2}+\mathrm{1}=\mathrm{3} \\ $$$$\:\:\:\:\:{m}={p}=\mathrm{2},{n}=\mathrm{3}\:\left({same}\:{result}\right) \\ $$$$\:^{\bullet} {m}={n}\:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={pn} \\ $$$$\:\:\:\:\left({n}+\mathrm{1}\right)\left({n}^{\mathrm{2}} −{n}+\mathrm{1}\right)={pn} \\ $$$$\:\:\:\:\:\:{n}^{\mathrm{3}} +\mathrm{1}={pn} \\ $$$$\:\:\:\:\:\:{n}^{\mathrm{2}} +\frac{\mathrm{1}}{{n}}={p} \\ $$$${Clearly}\:{p}\:{is}\:{whole}\:{number}\left({prime}\right) \\ $$$${so}\:\:{n}=\mathrm{1}\Rightarrow{m}=\mathrm{1} \\ $$$${so}\:{p}=\mathrm{2}\: \\ $$$$\left({gcd}\left(\mathrm{1}^{\mathrm{2}} −\mathrm{1}+\mathrm{1},\mathrm{1}+\mathrm{1}\right)={gcd}\left(\mathrm{1},\mathrm{2}\right)=\mathrm{1}\right) \\ $$$$\:^{\bullet} {m}={n}^{\mathrm{2}} \:\wedge\:\left({m}+\mathrm{1}\right)\left({m}^{\mathrm{2}} −{m}+\mathrm{1}\right)={p} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({n}^{\mathrm{2}} +\mathrm{1}\right)\left({n}^{\mathrm{2}} −{n}+\mathrm{1}\right)={p} \\ $$$$\Rightarrow\:\:\:{n}^{\mathrm{2}} +\mathrm{1}=\mathrm{1}\:\wedge\:{n}^{\mathrm{2}} −{n}+\mathrm{1}={p}......\left({i}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{OR} \\ $$$$\:\:\:\:\:\:\:\:\:\:{n}^{\mathrm{2}} +\mathrm{1}={p}\:\wedge\:{n}^{\mathrm{2}} −{n}+\mathrm{1}=\mathrm{1}.....\left({ii}\right) \\ $$$$\left({i}\right)\:\:{n}=\mathrm{0}\:\wedge\:{p}=\mathrm{1}\left(\:{false}\:\because\:{p}\in\mathbb{P}\right) \\ $$$$\left({ii}\right)\:{n}^{\mathrm{2}} −{n}+\mathrm{1}=\mathrm{1}\Rightarrow{n}\left({n}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\Rightarrow{n}=\mathrm{0}\:\mid\:{n}=\mathrm{1} \\ $$$$\:\:\:\:\:{n}^{\mathrm{2}} +\mathrm{1}={p}\:\: \\ $$$$\:\:\:\:\:\:\mathrm{0}^{\mathrm{2}} +\mathrm{1}={p}\:\mid\:\mathrm{1}^{\mathrm{2}} +\mathrm{1}={p} \\ $$$$\:\:\:\:\:\:\:{p}=\mathrm{1}\:\:\:\:\:\:\:\:\:\mid\:\:{p}=\mathrm{2} \\ $$$$\:\:\:\:\:\:\left({false}\right)\:\:\:\mid\:{n}=\mathrm{1}\Rightarrow{m}={n}^{\mathrm{2}} =\mathrm{1}^{\mathrm{2}} =\mathrm{1}\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mid\:{gcd}\left(\mathrm{1}^{\mathrm{2}} −\mathrm{1}+\mathrm{1},\mathrm{1}+\mathrm{1}\right)=\mathrm{1}\: \\ $$$$....... \\ $$$$..... \\ $$$$ \\ $$$$\:\:\:\: \\ $$

Commented by mathdanisur last updated on 26/Jun/21

How Sir..

$${How}\:{Sir}.. \\ $$

Commented by Rasheed.Sindhi last updated on 26/Jun/21

On editing, a great part of my answer has been disappeared!!! Don′t know why? Perhaps because my answer was too lengthy!

$${On}\:{editing},\:{a}\:{great}\:{part}\:{of}\:{my}\:\:{answer}\:{has} \\ $$$${been}\:{disappeared}!!!\:{Don}'{t}\:{know} \\ $$$${why}?\:{Perhaps}\:{because}\:{my}\:{answer} \\ $$$${was}\:{too}\:{lengthy}! \\ $$

Commented by mathdanisur last updated on 26/Jun/21

It′s a pity sometimes, thank you Sir

$${It}'{s}\:{a}\:{pity}\:{sometimes},\:{thank}\:{you}\:{Sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com