Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 145316 by imjagoll last updated on 04/Jul/21

Commented by imjagoll last updated on 04/Jul/21

interesting problem ≠geometry

$$\mathrm{interesting}\:\mathrm{problem} \\ $$$$\neq\mathrm{geometry} \\ $$

Commented by imjagoll last updated on 04/Jul/21

can anyone solve this?

$$\mathrm{can}\:\mathrm{anyone}\:\mathrm{solve}\:\mathrm{this}? \\ $$

Answered by ajfour last updated on 04/Jul/21

Commented by ajfour last updated on 04/Jul/21

let B be the origin and x-axis leftwards. A(0,d) eq. of first circles x^2 +y^2 =r^2 (x−d)^2 +y^2 =1 subtracting the two for x coordinate of point of intersection. d(2p−d)=r^2 −1 p=((r^2 +d^2 −1)/(2d)) q=±(√(r^2 −p^2 )) m=((dy/dx))_r =−(p/q) s=((dy/dx))_2 =((d−p)/q) ((m−s)/(1+ms))=(√3) (=tan 60°) m−s=(√3)+(√3)ms −(p/q)−((d−p)/q)=(√3)−(√3)((p/q))(((d−p)/q)) dq=(√3)(dp−p^2 −q^2 ) d(√(r^2 −p^2 ))=(√3)(dp−p^2 −r^2 +p^2 ) ⇒ d(√(r^2 −p^2 ))=(√3)(dp−r^2 ) d^2 {r^2 −(((r^2 +d^2 −1)/(2d)))^2 } =3(((r^2 +d^2 −1)/2)−r^2 )^2 ⇒ r^2 d^2 −(((r^2 +d^2 −1)^2 )/4) =(3/4)(r^2 +d^2 −1)^2 +3r^4 −3r^2 (r^2 +d^2 −1) ⇒ (r^2 +d^2 −1)^2 +3r^4 =3r^2 (r^2 +d^2 −1)+r^2 d^2 let d^2 −1=kr^2 ⇒ (1+k)^2 +3=3(1+k)+(1/r^2 )+k ⇒ k^2 −2k+1=(1/r^2 ) or r=(1/(k−1)) d^2 =1+(1+(1/r))r^2 d^2 =1+r^2 +r d^2 =(r+(1/2))^2 +(3/4) d is minimum for r=−(1/2) d_(min) =((√3)/2)

$${let}\:{B}\:{be}\:{the}\:{origin}\:{and}\:{x}-{axis} \\ $$$${leftwards}.\:{A}\left(\mathrm{0},{d}\right) \\ $$$${eq}.\:{of}\:{first}\:{circles} \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={r}^{\mathrm{2}} \:\:\: \\ $$$$\left({x}−{d}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{1} \\ $$$${subtracting}\:{the}\:{two}\:{for}\: \\ $$$${x}\:{coordinate}\:{of}\:{point}\:{of} \\ $$$${intersection}. \\ $$$${d}\left(\mathrm{2}{p}−{d}\right)={r}^{\mathrm{2}} −\mathrm{1} \\ $$$${p}=\frac{{r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}{d}} \\ $$$${q}=\pm\sqrt{{r}^{\mathrm{2}} −{p}^{\mathrm{2}} } \\ $$$${m}=\left(\frac{{dy}}{{dx}}\right)_{{r}} =−\frac{{p}}{{q}}\: \\ $$$${s}=\left(\frac{{dy}}{{dx}}\right)_{\mathrm{2}} =\frac{{d}−{p}}{{q}} \\ $$$$\frac{{m}−{s}}{\mathrm{1}+{ms}}=\sqrt{\mathrm{3}}\:\:\:\:\:\:\left(=\mathrm{tan}\:\mathrm{60}°\right) \\ $$$${m}−{s}=\sqrt{\mathrm{3}}+\sqrt{\mathrm{3}}{ms} \\ $$$$−\frac{{p}}{{q}}−\frac{{d}−{p}}{{q}}=\sqrt{\mathrm{3}}−\sqrt{\mathrm{3}}\left(\frac{{p}}{{q}}\right)\left(\frac{{d}−{p}}{{q}}\right) \\ $$$${dq}=\sqrt{\mathrm{3}}\left({dp}−{p}^{\mathrm{2}} −{q}^{\mathrm{2}} \right) \\ $$$${d}\sqrt{{r}^{\mathrm{2}} −{p}^{\mathrm{2}} }=\sqrt{\mathrm{3}}\left({dp}−{p}^{\mathrm{2}} −{r}^{\mathrm{2}} +{p}^{\mathrm{2}} \right) \\ $$$$\Rightarrow\:{d}\sqrt{{r}^{\mathrm{2}} −{p}^{\mathrm{2}} }=\sqrt{\mathrm{3}}\left({dp}−{r}^{\mathrm{2}} \right) \\ $$$$\:\:{d}^{\mathrm{2}} \left\{{r}^{\mathrm{2}} −\left(\frac{{r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}{d}}\right)^{\mathrm{2}} \right\} \\ $$$$\:\:\:\:=\mathrm{3}\left(\frac{{r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}}−{r}^{\mathrm{2}} \right)^{\mathrm{2}} \\ $$$$\Rightarrow\:\:{r}^{\mathrm{2}} {d}^{\mathrm{2}} −\frac{\left({r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\:\:=\frac{\mathrm{3}}{\mathrm{4}}\left({r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{3}{r}^{\mathrm{4}} \\ $$$$\:\:\:\:\:\:\:\:−\mathrm{3}{r}^{\mathrm{2}} \left({r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\Rightarrow \\ $$$$\left({r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{3}{r}^{\mathrm{4}} \\ $$$$\:\:\:\:\:=\mathrm{3}{r}^{\mathrm{2}} \left({r}^{\mathrm{2}} +{d}^{\mathrm{2}} −\mathrm{1}\right)+{r}^{\mathrm{2}} {d}^{\mathrm{2}} \\ $$$${let}\:\:\:{d}^{\mathrm{2}} −\mathrm{1}={kr}^{\mathrm{2}} \:\:\Rightarrow \\ $$$$\left(\mathrm{1}+{k}\right)^{\mathrm{2}} +\mathrm{3}=\mathrm{3}\left(\mathrm{1}+{k}\right)+\frac{\mathrm{1}}{{r}^{\mathrm{2}} }+{k} \\ $$$$\Rightarrow\:\:{k}^{\mathrm{2}} −\mathrm{2}{k}+\mathrm{1}=\frac{\mathrm{1}}{{r}^{\mathrm{2}} }\:\:\:\:{or} \\ $$$${r}=\frac{\mathrm{1}}{{k}−\mathrm{1}} \\ $$$${d}^{\mathrm{2}} =\mathrm{1}+\left(\mathrm{1}+\frac{\mathrm{1}}{{r}}\right){r}^{\mathrm{2}} \\ $$$${d}^{\mathrm{2}} =\mathrm{1}+{r}^{\mathrm{2}} +{r} \\ $$$$\:{d}^{\mathrm{2}} =\left({r}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}} \\ $$$${d}\:{is}\:{minimum}\:{for}\:{r}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\:\:{d}_{{min}} =\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$

Answered by mr W last updated on 04/Jul/21

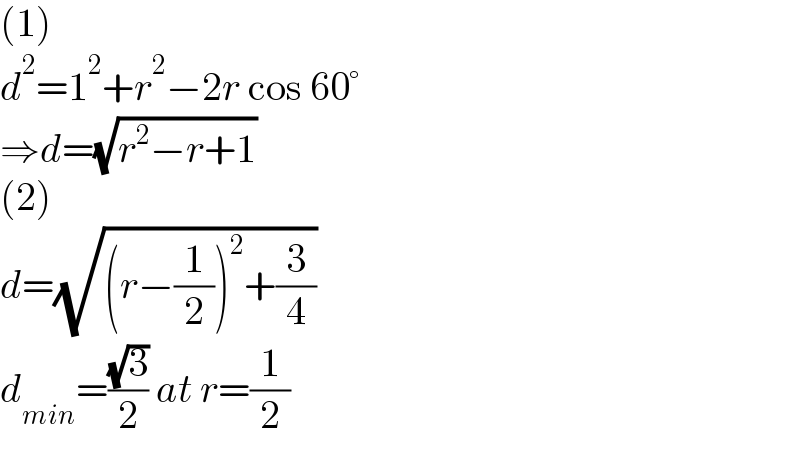
(1) d^2 =1^2 +r^2 −2r cos 60° ⇒d=(√(r^2 −r+1)) (2) d=(√((r−(1/2))^2 +(3/4))) d_(min) =((√3)/2) at r=(1/2)

$$\left(\mathrm{1}\right) \\ $$$${d}^{\mathrm{2}} =\mathrm{1}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{2}{r}\:\mathrm{cos}\:\mathrm{60}° \\ $$$$\Rightarrow{d}=\sqrt{{r}^{\mathrm{2}} −{r}+\mathrm{1}} \\ $$$$\left(\mathrm{2}\right) \\ $$$${d}=\sqrt{\left({r}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}} \\ $$$${d}_{{min}} =\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{at}\:{r}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Commented by mr W last updated on 04/Jul/21

Commented by mr W last updated on 04/Jul/21

(3) area of intersection: (π/2)×((1/2))^2 +(1^2 /2)((π/3)−sin (π/3)) =((7π)/(24))−((√3)/4)

$$\left(\mathrm{3}\right) \\ $$$${area}\:{of}\:{intersection}: \\ $$$$\frac{\pi}{\mathrm{2}}×\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{\mathrm{1}^{\mathrm{2}} }{\mathrm{2}}\left(\frac{\pi}{\mathrm{3}}−\mathrm{sin}\:\frac{\pi}{\mathrm{3}}\right) \\ $$$$=\frac{\mathrm{7}\pi}{\mathrm{24}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{4}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com