Question and Answers Forum

All Questions      Topic List

Probability and Statistics Questions

Previous in All Question      Next in All Question      

Previous in Probability and Statistics      Next in Probability and Statistics      

Question Number 147906 by Odhiambojr last updated on 24/Jul/21

4 bad apples are mixed accidentally with 20 good apples. Obtain the probability distribution of the numbers of bad apples in a draw of 2 apples at random

$$\mathrm{4}\:{bad}\:{apples}\:{are}\:{mixed}\:{accidentally} \\ $$$${with}\:\mathrm{20}\:{good}\:{apples}.\:{Obtain}\:{the} \\ $$$$\:{probability}\:{distribution}\:{of}\:{the}\: \\ $$$${numbers}\:{of}\:{bad}\:{apples}\:{in}\:{a}\:{draw}\:{of}\: \\ $$$$\mathrm{2}\:{apples}\:{at}\:{random} \\ $$

Answered by Olaf_Thorendsen last updated on 24/Jul/21

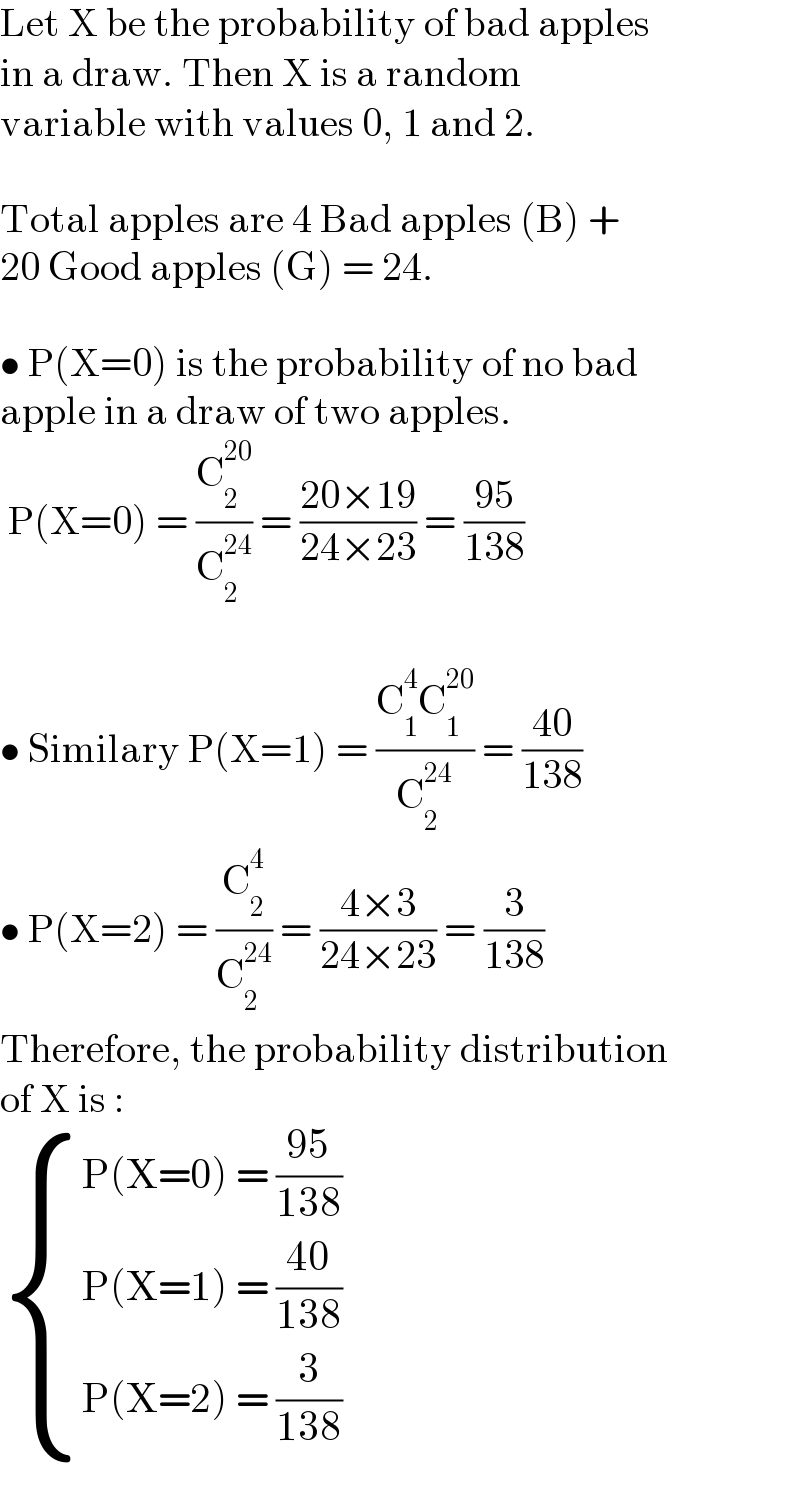
Let X be the probability of bad apples in a draw. Then X is a random variable with values 0, 1 and 2. Total apples are 4 Bad apples (B) + 20 Good apples (G) = 24. • P(X=0) is the probability of no bad apple in a draw of two apples. P(X=0) = (C_2 ^(20) /C_2 ^(24) ) = ((20×19)/(24×23)) = ((95)/(138)) • Similary P(X=1) = ((C_1 ^4 C_1 ^(20) )/C_2 ^(24) ) = ((40)/(138)) • P(X=2) = (C_2 ^4 /C_2 ^(24) ) = ((4×3)/(24×23)) = (3/(138)) Therefore, the probability distribution of X is : { ((P(X=0) = ((95)/(138)))),((P(X=1) = ((40)/(138)))),((P(X=2) = (3/(138)))) :}

$$\mathrm{Let}\:\mathrm{X}\:\mathrm{be}\:\mathrm{the}\:\mathrm{probability}\:\mathrm{of}\:\mathrm{bad}\:\mathrm{apples} \\ $$$$\mathrm{in}\:\mathrm{a}\:\mathrm{draw}.\:\mathrm{Then}\:\mathrm{X}\:\mathrm{is}\:\mathrm{a}\:\mathrm{random} \\ $$$$\mathrm{variable}\:\mathrm{with}\:\mathrm{values}\:\mathrm{0},\:\mathrm{1}\:\mathrm{and}\:\mathrm{2}. \\ $$$$ \\ $$$$\mathrm{Total}\:\mathrm{apples}\:\mathrm{are}\:\mathrm{4}\:\mathrm{Bad}\:\mathrm{apples}\:\left(\mathrm{B}\right)\:+ \\ $$$$\mathrm{20}\:\mathrm{Good}\:\mathrm{apples}\:\left(\mathrm{G}\right)\:=\:\mathrm{24}. \\ $$$$ \\ $$$$\bullet\:\mathrm{P}\left(\mathrm{X}=\mathrm{0}\right)\:\mathrm{is}\:\mathrm{the}\:\mathrm{probability}\:\mathrm{of}\:\mathrm{no}\:\mathrm{bad} \\ $$$$\mathrm{apple}\:\mathrm{in}\:\mathrm{a}\:\mathrm{draw}\:\mathrm{of}\:\mathrm{two}\:\mathrm{apples}. \\ $$$$\:\mathrm{P}\left(\mathrm{X}=\mathrm{0}\right)\:=\:\frac{\mathrm{C}_{\mathrm{2}} ^{\mathrm{20}} }{\mathrm{C}_{\mathrm{2}} ^{\mathrm{24}} }\:=\:\frac{\mathrm{20}×\mathrm{19}}{\mathrm{24}×\mathrm{23}}\:=\:\frac{\mathrm{95}}{\mathrm{138}} \\ $$$$ \\ $$$$\bullet\:\mathrm{Similary}\:\mathrm{P}\left(\mathrm{X}=\mathrm{1}\right)\:=\:\frac{\mathrm{C}_{\mathrm{1}} ^{\mathrm{4}} \mathrm{C}_{\mathrm{1}} ^{\mathrm{20}} }{\mathrm{C}_{\mathrm{2}} ^{\mathrm{24}} }\:=\:\frac{\mathrm{40}}{\mathrm{138}} \\ $$$$\bullet\:\mathrm{P}\left(\mathrm{X}=\mathrm{2}\right)\:=\:\frac{\mathrm{C}_{\mathrm{2}} ^{\mathrm{4}} }{\mathrm{C}_{\mathrm{2}} ^{\mathrm{24}} }\:=\:\frac{\mathrm{4}×\mathrm{3}}{\mathrm{24}×\mathrm{23}}\:=\:\frac{\mathrm{3}}{\mathrm{138}} \\ $$$$\mathrm{Therefore},\:\mathrm{the}\:\mathrm{probability}\:\mathrm{distribution} \\ $$$$\mathrm{of}\:\mathrm{X}\:\mathrm{is}\:: \\ $$$$\begin{cases}{\mathrm{P}\left(\mathrm{X}=\mathrm{0}\right)\:=\:\frac{\mathrm{95}}{\mathrm{138}}}\\{\mathrm{P}\left(\mathrm{X}=\mathrm{1}\right)\:=\:\frac{\mathrm{40}}{\mathrm{138}}}\\{\mathrm{P}\left(\mathrm{X}=\mathrm{2}\right)\:=\:\frac{\mathrm{3}}{\mathrm{138}}}\end{cases} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com