Question Number 148454 by mathdanisur last updated on 28/Jul/21

sin^6 𝛂 + co^6 𝛂 = (3/4) ⇒ 6cos4𝛂=?

Answered by Ar Brandon last updated on 28/Jul/21

sin^6 α+cos^6 α=(3/4) (sin^2 α+cos^2 α)[(sin^2 α+cos^2 α)^2 −3sin^2 αcos^2 α]=(3/4) 1−3sin^2 αcos^2 α=(3/4)⇒sin^2 αcos^2 α=(1/(12)) 6cos4α=6(cos^2 2α−sin^2 2α) 6cos4α=6[(cos^4 α−2sin^2 αcos^2 α+sin^4 α)−4sin^2 αcos^2 α] =6[(cos^2 α+sin^2 α)^2 −8sin^2 αcos^2 α] =6(1−8sin^2 αcos^2 α)=6(1−(8/(12)))=2

Commented bymathdanisur last updated on 28/Jul/21

Thank you Ser cool

Answered by Olaf_Thorendsen last updated on 28/Jul/21

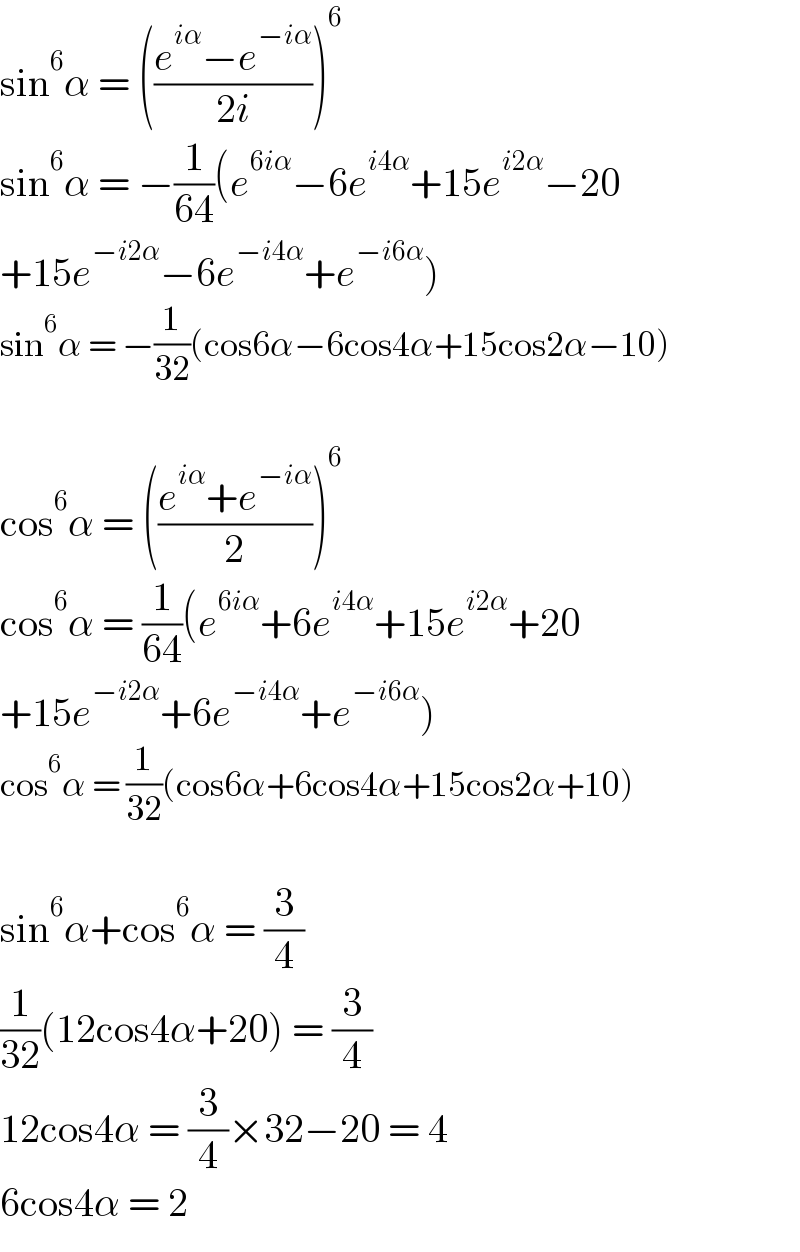
sin^6 α = (((e^(iα) −e^(−iα) )/(2i)))^6 sin^6 α = −(1/(64))(e^(6iα) −6e^(i4α) +15e^(i2α) −20 +15e^(−i2α) −6e^(−i4α) +e^(−i6α) ) sin^6 α = −(1/(32))(cos6α−6cos4α+15cos2α−10) cos^6 α = (((e^(iα) +e^(−iα) )/2))^6 cos^6 α = (1/(64))(e^(6iα) +6e^(i4α) +15e^(i2α) +20 +15e^(−i2α) +6e^(−i4α) +e^(−i6α) ) cos^6 α = (1/(32))(cos6α+6cos4α+15cos2α+10) sin^6 α+cos^6 α = (3/4) (1/(32))(12cos4α+20) = (3/4) 12cos4α = (3/4)×32−20 = 4 6cos4α = 2

Commented bymathdanisur last updated on 28/Jul/21

Thank you Ser cool