Question Number 149339 by mnjuly1970 last updated on 04/Aug/21

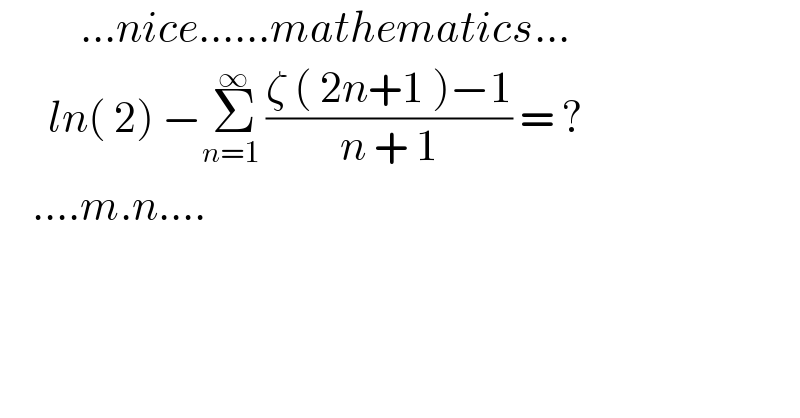
...nice......mathematics... ln( 2) −Σ_(n=1 ) ^∞ ((ζ ( 2n+1 )−1)/(n + 1)) = ? ....m.n....

Answered by Kamel last updated on 04/Aug/21

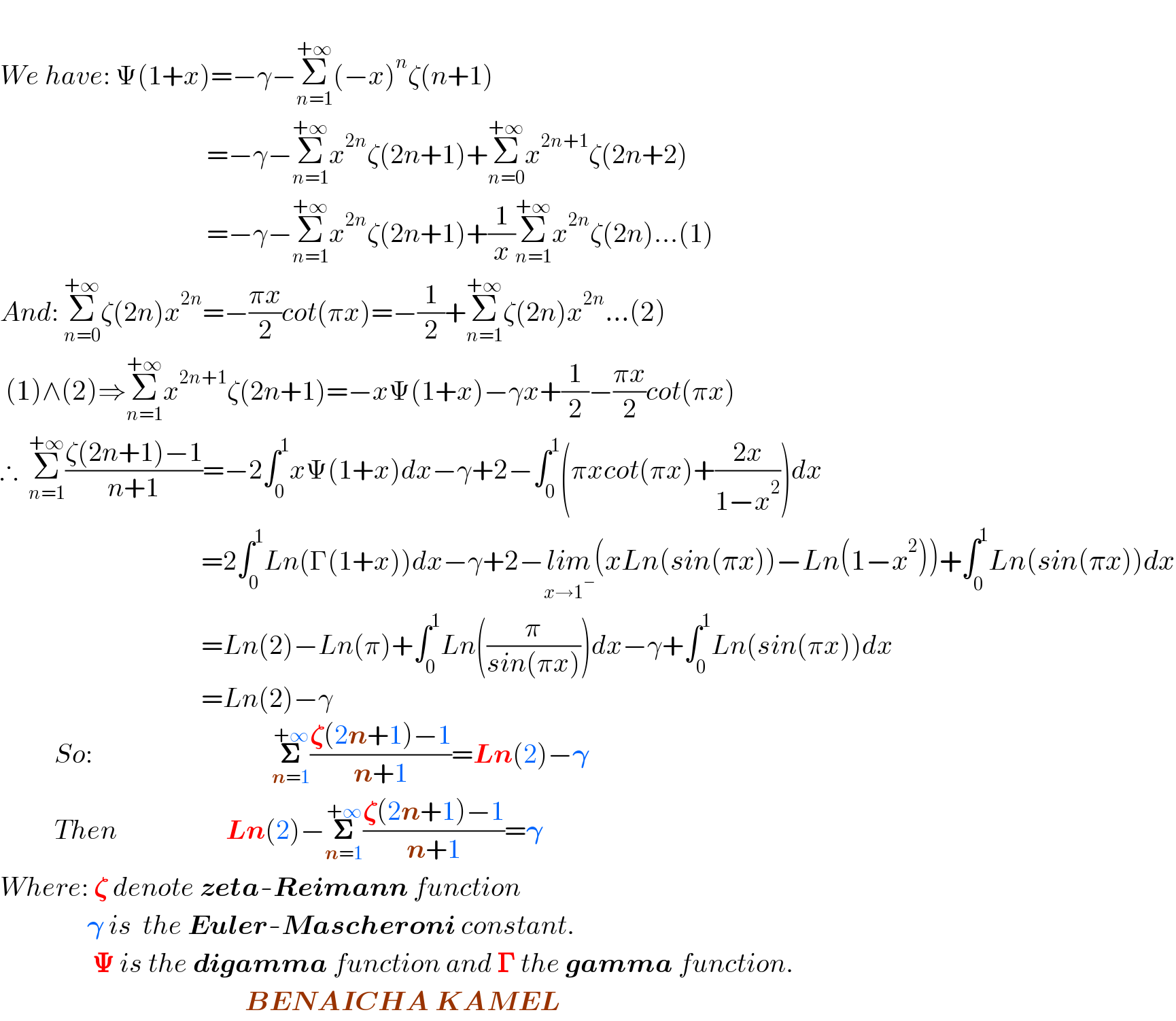
We have: Ψ(1+x)=−γ−Σ_(n=1) ^(+∞) (−x)^n ζ(n+1) =−γ−Σ_(n=1) ^(+∞) x^(2n) ζ(2n+1)+Σ_(n=0) ^(+∞) x^(2n+1) ζ(2n+2) =−γ−Σ_(n=1) ^(+∞) x^(2n) ζ(2n+1)+(1/x)Σ_(n=1) ^(+∞) x^(2n) ζ(2n)...(1) And: Σ_(n=0) ^(+∞) ζ(2n)x^(2n) =−((πx)/2)cot(πx)=−(1/2)+Σ_(n=1) ^(+∞) ζ(2n)x^(2n) ...(2) (1)∧(2)⇒Σ_(n=1) ^(+∞) x^(2n+1) ζ(2n+1)=−xΨ(1+x)−γx+(1/2)−((πx)/2)cot(πx) ∴ Σ_(n=1) ^(+∞) ((ζ(2n+1)−1)/(n+1))=−2∫_0 ^1 xΨ(1+x)dx−γ+2−∫_0 ^1 (πxcot(πx)+((2x)/(1−x^2 )))dx =2∫_0 ^1 Ln(Γ(1+x))dx−γ+2−lim_(x→1^− ) (xLn(sin(πx))−Ln(1−x^2 ))+∫_0 ^1 Ln(sin(πx))dx =Ln(2)−Ln(π)+∫_0 ^1 Ln((π/(sin(πx))))dx−γ+∫_0 ^1 Ln(sin(πx))dx =Ln(2)−γ So: 𝚺_(n=1) ^(+∞) ((𝛇(2n+1)−1)/(n+1))=Ln(2)−𝛄 Then Ln(2)−𝚺_(n=1) ^(+∞) ((𝛇(2n+1)−1)/(n+1))=𝛄 Where: 𝛇 denote zeta-Reimann function 𝛄 is the Euler-Mascheroni constant. 𝚿 is the digamma function and 𝚪 the gamma function. BENAICHA KAMEL

Commented bymnjuly1970 last updated on 04/Aug/21

very nice master , Mr .Kamel..

Commented byKamel last updated on 04/Aug/21

Thank you.

Commented byTawa11 last updated on 04/Aug/21

Weldone sir.