Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 149598 by naka3546 last updated on 06/Aug/21

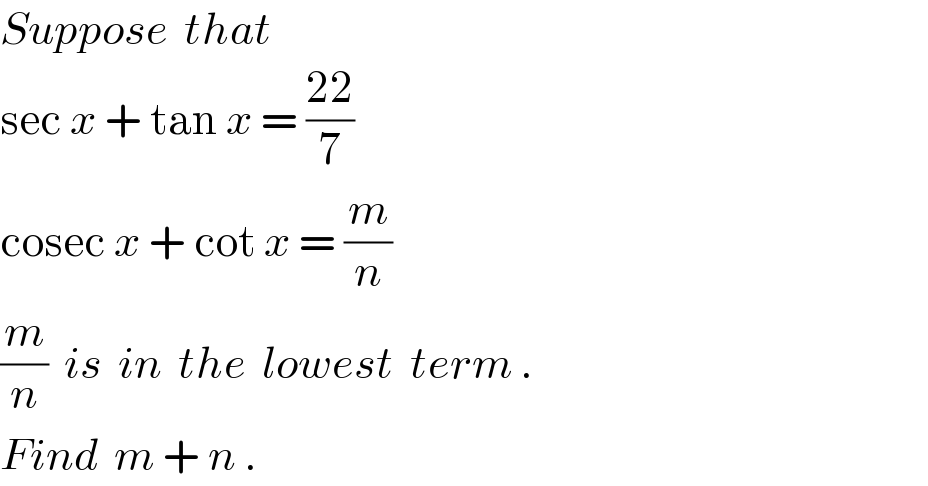
Suppose that sec x + tan x = ((22)/7) cosec x + cot x = (m/n) (m/n) is in the lowest term . Find m + n .

$${Suppose}\:\:{that}\:\: \\ $$$$\mathrm{sec}\:{x}\:+\:\mathrm{tan}\:{x}\:=\:\frac{\mathrm{22}}{\mathrm{7}} \\ $$$$\mathrm{cosec}\:{x}\:+\:\mathrm{cot}\:{x}\:=\:\frac{{m}}{{n}} \\ $$$$\frac{{m}}{{n}}\:\:{is}\:\:{in}\:\:{the}\:\:{lowest}\:\:{term}\:. \\ $$$${Find}\:\:{m}\:+\:{n}\:. \\ $$

Answered by iloveisrael last updated on 06/Aug/21

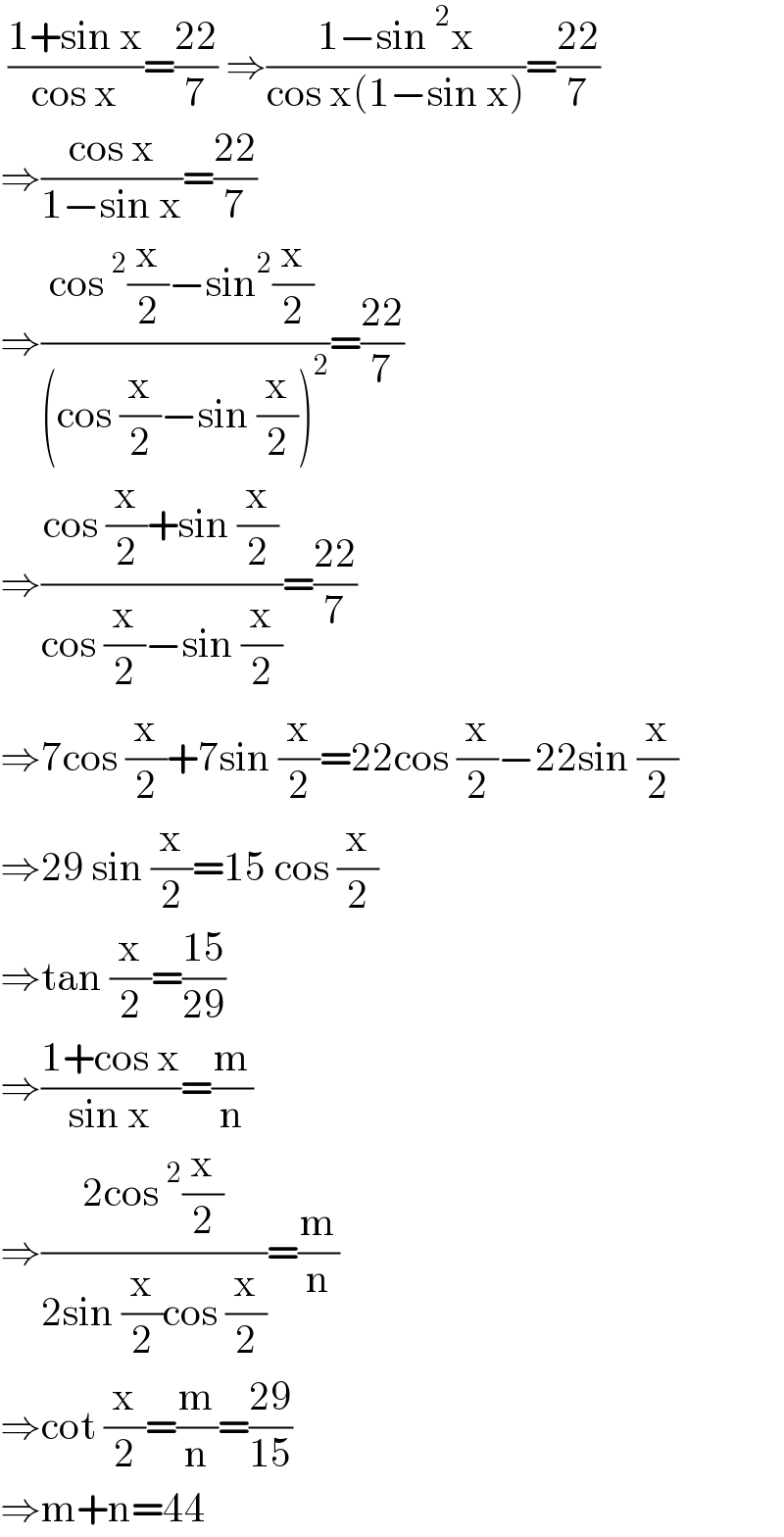
((1+sin x)/(cos x))=((22)/7) ⇒((1−sin^2 x)/(cos x(1−sin x)))=((22)/7) ⇒((cos x)/(1−sin x))=((22)/7) ⇒((cos^2 (x/2)−sin^2 (x/2) )/((cos (x/2)−sin (x/2))^2 ))=((22)/7) ⇒((cos (x/2)+sin (x/2))/(cos (x/2)−sin (x/2)))=((22)/7) ⇒7cos (x/2)+7sin (x/2)=22cos (x/2)−22sin (x/2) ⇒29 sin (x/2)=15 cos (x/2) ⇒tan (x/2)=((15)/(29)) ⇒((1+cos x)/(sin x))=(m/n) ⇒((2cos^2 (x/2))/(2sin (x/2)cos (x/2)))=(m/n) ⇒cot (x/2)=(m/n)=((29)/(15)) ⇒m+n=44

$$\:\frac{\mathrm{1}+\mathrm{sin}\:\mathrm{x}}{\mathrm{cos}\:\mathrm{x}}=\frac{\mathrm{22}}{\mathrm{7}}\:\Rightarrow\frac{\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} \mathrm{x}}{\mathrm{cos}\:\mathrm{x}\left(\mathrm{1}−\mathrm{sin}\:\mathrm{x}\right)}=\frac{\mathrm{22}}{\mathrm{7}} \\ $$$$\Rightarrow\frac{\mathrm{cos}\:\mathrm{x}}{\mathrm{1}−\mathrm{sin}\:\mathrm{x}}=\frac{\mathrm{22}}{\mathrm{7}} \\ $$$$\Rightarrow\frac{\mathrm{cos}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}−\mathrm{sin}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\:}{\left(\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}−\mathrm{sin}\:\frac{\mathrm{x}}{\mathrm{2}}\right)^{\mathrm{2}} }=\frac{\mathrm{22}}{\mathrm{7}} \\ $$$$\Rightarrow\frac{\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}+\mathrm{sin}\:\frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}−\mathrm{sin}\:\frac{\mathrm{x}}{\mathrm{2}}}=\frac{\mathrm{22}}{\mathrm{7}} \\ $$$$\Rightarrow\mathrm{7cos}\:\frac{\mathrm{x}}{\mathrm{2}}+\mathrm{7sin}\:\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{22cos}\:\frac{\mathrm{x}}{\mathrm{2}}−\mathrm{22sin}\:\frac{\mathrm{x}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{29}\:\mathrm{sin}\:\frac{\mathrm{x}}{\mathrm{2}}=\mathrm{15}\:\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}=\frac{\mathrm{15}}{\mathrm{29}} \\ $$$$\Rightarrow\frac{\mathrm{1}+\mathrm{cos}\:\mathrm{x}}{\mathrm{sin}\:\mathrm{x}}=\frac{\mathrm{m}}{\mathrm{n}} \\ $$$$\Rightarrow\frac{\mathrm{2cos}\:^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{2sin}\:\frac{\mathrm{x}}{\mathrm{2}}\mathrm{cos}\:\frac{\mathrm{x}}{\mathrm{2}}}=\frac{\mathrm{m}}{\mathrm{n}} \\ $$$$\Rightarrow\mathrm{cot}\:\frac{\mathrm{x}}{\mathrm{2}}=\frac{\mathrm{m}}{\mathrm{n}}=\frac{\mathrm{29}}{\mathrm{15}} \\ $$$$\Rightarrow\mathrm{m}+\mathrm{n}=\mathrm{44} \\ $$

Answered by nimnim last updated on 06/Aug/21

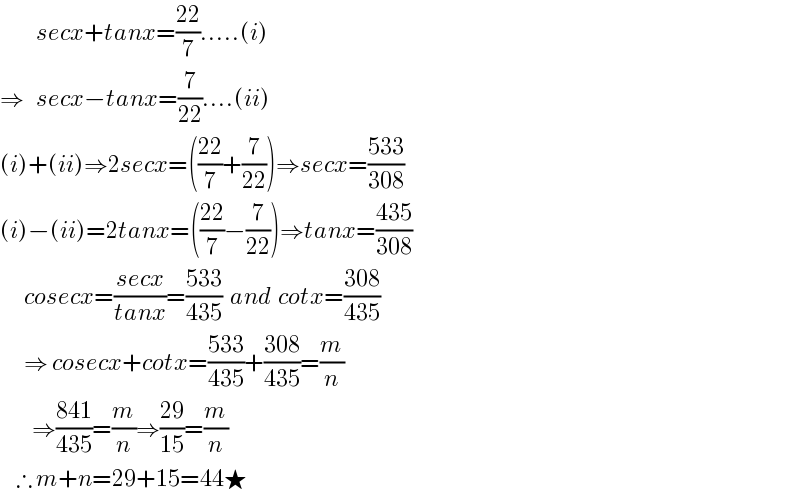
secx+tanx=((22)/7).....(i) ⇒ secx−tanx=(7/(22))....(ii) (i)+(ii)⇒2secx=(((22)/7)+(7/(22)))⇒secx=((533)/(308)) (i)−(ii)=2tanx=(((22)/7)−(7/(22)))⇒tanx=((435)/(308)) cosecx=((secx)/(tanx))=((533)/(435)) and cotx=((308)/(435)) ⇒ cosecx+cotx=((533)/(435))+((308)/(435))=(m/n) ⇒((841)/(435))=(m/n)⇒((29)/(15))=(m/n) ∴ m+n=29+15=44★

$$\:\:\:\:\:\:\:\:\:{secx}+{tanx}=\frac{\mathrm{22}}{\mathrm{7}}.....\left({i}\right) \\ $$$$\Rightarrow\:\:\:{secx}−{tanx}=\frac{\mathrm{7}}{\mathrm{22}}....\left({ii}\right) \\ $$$$\left({i}\right)+\left({ii}\right)\Rightarrow\mathrm{2}{secx}=\left(\frac{\mathrm{22}}{\mathrm{7}}+\frac{\mathrm{7}}{\mathrm{22}}\right)\Rightarrow{secx}=\frac{\mathrm{533}}{\mathrm{308}} \\ $$$$\left({i}\right)−\left({ii}\right)=\mathrm{2}{tanx}=\left(\frac{\mathrm{22}}{\mathrm{7}}−\frac{\mathrm{7}}{\mathrm{22}}\right)\Rightarrow{tanx}=\frac{\mathrm{435}}{\mathrm{308}}\: \\ $$$$\:\:\:\:\:\:{cosecx}=\frac{{secx}}{{tanx}}=\frac{\mathrm{533}}{\mathrm{435}}\:\:{and}\:\:{cotx}=\frac{\mathrm{308}}{\mathrm{435}} \\ $$$$\:\:\:\:\:\:\Rightarrow\:{cosecx}+{cotx}=\frac{\mathrm{533}}{\mathrm{435}}+\frac{\mathrm{308}}{\mathrm{435}}=\frac{{m}}{{n}} \\ $$$$\:\:\:\:\:\:\:\:\Rightarrow\frac{\mathrm{841}}{\mathrm{435}}=\frac{{m}}{{n}}\Rightarrow\frac{\mathrm{29}}{\mathrm{15}}=\frac{{m}}{{n}} \\ $$$$\:\:\:\:\therefore\:{m}+{n}=\mathrm{29}+\mathrm{15}=\mathrm{44}\bigstar \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com