Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 149621 by mathdanisur last updated on 06/Aug/21

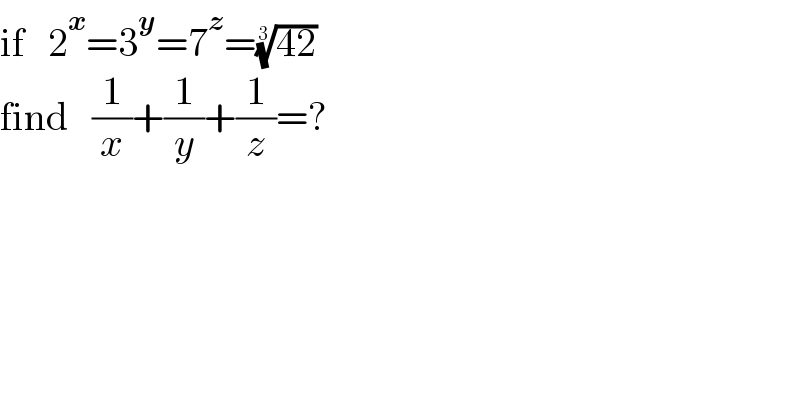
if 2^x =3^y =7^z =((42))^(1/3) find (1/x)+(1/y)+(1/z)=?

$$\mathrm{if}\:\:\:\mathrm{2}^{\boldsymbol{{x}}} =\mathrm{3}^{\boldsymbol{{y}}} =\mathrm{7}^{\boldsymbol{{z}}} =\sqrt[{\mathrm{3}}]{\mathrm{42}} \\ $$$$\mathrm{find}\:\:\:\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}+\frac{\mathrm{1}}{{z}}=? \\ $$

Answered by iloveisrael last updated on 06/Aug/21

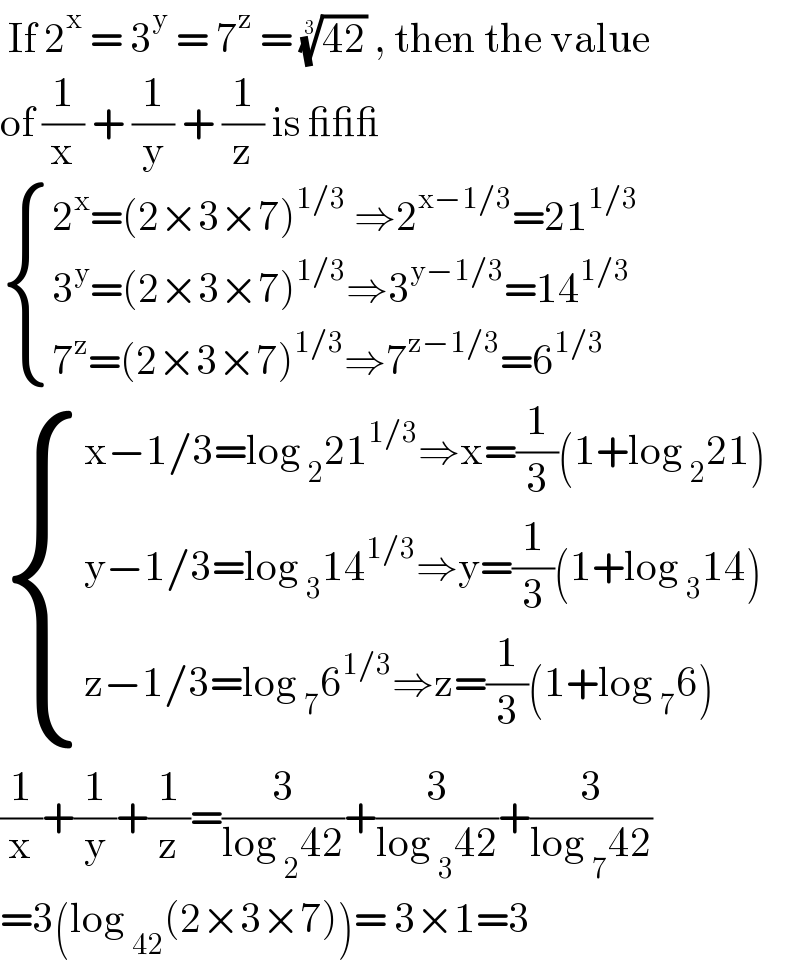
If 2^x = 3^y = 7^z = ((42))^(1/3) , then the value of (1/x) + (1/y) + (1/z) is ___ { ((2^x =(2×3×7)^(1/3) ⇒2^(x−1/3) =21^(1/3) )),((3^y =(2×3×7)^(1/3) ⇒3^(y−1/3) =14^(1/3) )),((7^z =(2×3×7)^(1/3) ⇒7^(z−1/3) =6^(1/3) )) :} { ((x−1/3=log _2 21^(1/3) ⇒x=(1/3)(1+log _2 21))),((y−1/3=log _3 14^(1/3) ⇒y=(1/3)(1+log _3 14))),((z−1/3=log _7 6^(1/3) ⇒z=(1/3)(1+log _7 6))) :} (1/x)+(1/y)+(1/z)=(3/(log _2 42))+(3/(log _3 42))+(3/(log _7 42)) =3(log _(42) (2×3×7))= 3×1=3

$$\:\mathrm{If}\:\mathrm{2}^{\mathrm{x}} \:=\:\mathrm{3}^{\mathrm{y}} \:=\:\mathrm{7}^{\mathrm{z}} \:=\:\sqrt[{\mathrm{3}}]{\mathrm{42}}\:,\:\mathrm{then}\:\mathrm{the}\:\mathrm{value} \\ $$$$\mathrm{of}\:\frac{\mathrm{1}}{\mathrm{x}}\:+\:\frac{\mathrm{1}}{\mathrm{y}}\:+\:\frac{\mathrm{1}}{\mathrm{z}}\:\mathrm{is}\:\_\_\_ \\ $$$$\begin{cases}{\mathrm{2}^{\mathrm{x}} =\left(\mathrm{2}×\mathrm{3}×\mathrm{7}\right)^{\mathrm{1}/\mathrm{3}} \:\Rightarrow\mathrm{2}^{\mathrm{x}−\mathrm{1}/\mathrm{3}} =\mathrm{21}^{\mathrm{1}/\mathrm{3}} }\\{\mathrm{3}^{\mathrm{y}} =\left(\mathrm{2}×\mathrm{3}×\mathrm{7}\right)^{\mathrm{1}/\mathrm{3}} \Rightarrow\mathrm{3}^{\mathrm{y}−\mathrm{1}/\mathrm{3}} =\mathrm{14}^{\mathrm{1}/\mathrm{3}} }\\{\mathrm{7}^{\mathrm{z}} =\left(\mathrm{2}×\mathrm{3}×\mathrm{7}\right)^{\mathrm{1}/\mathrm{3}} \Rightarrow\mathrm{7}^{\mathrm{z}−\mathrm{1}/\mathrm{3}} =\mathrm{6}^{\mathrm{1}/\mathrm{3}} }\end{cases} \\ $$$$\begin{cases}{\mathrm{x}−\mathrm{1}/\mathrm{3}=\mathrm{log}\:_{\mathrm{2}} \mathrm{21}^{\mathrm{1}/\mathrm{3}} \Rightarrow\mathrm{x}=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}+\mathrm{log}\:_{\mathrm{2}} \mathrm{21}\right)}\\{\mathrm{y}−\mathrm{1}/\mathrm{3}=\mathrm{log}\:_{\mathrm{3}} \mathrm{14}^{\mathrm{1}/\mathrm{3}} \Rightarrow\mathrm{y}=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}+\mathrm{log}\:_{\mathrm{3}} \mathrm{14}\right)}\\{\mathrm{z}−\mathrm{1}/\mathrm{3}=\mathrm{log}\:_{\mathrm{7}} \mathrm{6}^{\mathrm{1}/\mathrm{3}} \Rightarrow\mathrm{z}=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}+\mathrm{log}\:_{\mathrm{7}} \mathrm{6}\right)}\end{cases} \\ $$$$\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{1}}{\mathrm{y}}+\frac{\mathrm{1}}{\mathrm{z}}=\frac{\mathrm{3}}{\mathrm{log}\:_{\mathrm{2}} \mathrm{42}}+\frac{\mathrm{3}}{\mathrm{log}\:_{\mathrm{3}} \mathrm{42}}+\frac{\mathrm{3}}{\mathrm{log}\:_{\mathrm{7}} \mathrm{42}} \\ $$$$=\mathrm{3}\left(\mathrm{log}\:_{\mathrm{42}} \left(\mathrm{2}×\mathrm{3}×\mathrm{7}\right)\right)=\:\mathrm{3}×\mathrm{1}=\mathrm{3} \\ $$

Commented by mathdanisur last updated on 06/Aug/21

Thankyou Ser

$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{S}}\mathrm{er} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com