Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 149796 by mr W last updated on 07/Aug/21

Commented by mr W last updated on 07/Aug/21

solution to Q148806

$${solution}\:{to}\:{Q}\mathrm{148806} \\ $$

Commented by mr W last updated on 07/Aug/21

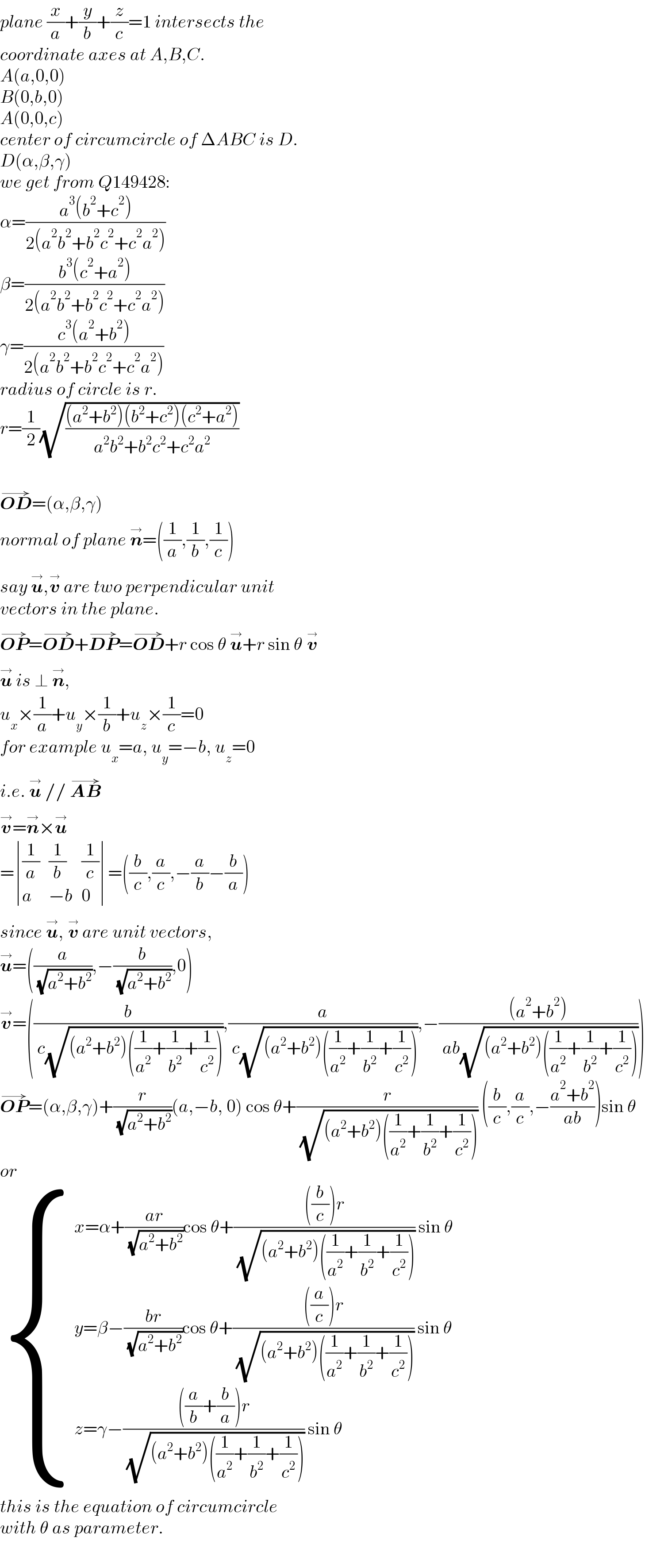
plane (x/a)+(y/b)+(z/c)=1 intersects the coordinate axes at A,B,C. A(a,0,0) B(0,b,0) A(0,0,c) center of circumcircle of ΔABC is D. D(α,β,γ) we get from Q149428: α=((a^3 (b^2 +c^2 ))/(2(a^2 b^2 +b^2 c^2 +c^2 a^2 ))) β=((b^3 (c^2 +a^2 ))/(2(a^2 b^2 +b^2 c^2 +c^2 a^2 ))) γ=((c^3 (a^2 +b^2 ))/(2(a^2 b^2 +b^2 c^2 +c^2 a^2 ))) radius of circle is r. r=(1/2)(√(((a^2 +b^2 )(b^2 +c^2 )(c^2 +a^2 ))/(a^2 b^2 +b^2 c^2 +c^2 a^2 ))) OD^(→) =(α,β,γ) normal of plane n^(→) =((1/a),(1/b),(1/c)) say u^(→) ,v^(→) are two perpendicular unit vectors in the plane. OP^(→) =OD^(→) +DP^(→) =OD^(→) +r cos θ u^(→) +r sin θ v^(→) u^→ is ⊥ n^(→) , u_x ×(1/a)+u_y ×(1/b)+u_z ×(1/c)=0 for example u_x =a, u_y =−b, u_z =0 i.e. u^(→) // AB^(→) v^→ =n^(→) ×u^(→) = determinant (((1/a),((1/b) ),(1/c)),(a,(−b),0))=((b/c),(a/c),−(a/b)−(b/a)) since u^(→) , v^(→) are unit vectors, u^→ =((a/( (√(a^2 +b^2 )))),−(b/( (√(a^2 +b^2 )))),0) v^→ =((b/( c(√((a^2 +b^2 )((1/a^2 )+(1/b^2 )+(1/c^2 )))))),(a/( c(√((a^2 +b^2 )((1/a^2 )+(1/b^2 )+(1/c^2 )))))),−(((a^2 +b^2 ))/( ab(√((a^2 +b^2 )((1/a^2 )+(1/b^2 )+(1/c^2 ))))))) OP^(→) =(α,β,γ)+(r/( (√(a^2 +b^2 ))))(a,−b, 0) cos θ+(r/( (√((a^2 +b^2 )((1/a^2 )+(1/b^2 )+(1/c^2 )))))) ((b/c),(a/c),−((a^2 +b^2 )/(ab)))sin θ or { ((x=α+((ar)/( (√(a^2 +b^2 ))))cos θ+((((b/c))r)/( (√((a^2 +b^2 )((1/a^2 )+(1/b^2 )+(1/c^2 )))))) sin θ)),((y=β−((br)/( (√(a^2 +b^2 ))))cos θ+((((a/c))r)/( (√((a^2 +b^2 )((1/a^2 )+(1/b^2 )+(1/c^2 )))))) sin θ)),((z=γ−((((a/b)+(b/a))r)/( (√((a^2 +b^2 )((1/a^2 )+(1/b^2 )+(1/c^2 )))))) sin θ)) :} this is the equation of circumcircle with θ as parameter.

$${plane}\:\frac{{x}}{{a}}+\frac{{y}}{{b}}+\frac{{z}}{{c}}=\mathrm{1}\:{intersects}\:{the} \\ $$$${coordinate}\:{axes}\:{at}\:{A},{B},{C}. \\ $$$${A}\left({a},\mathrm{0},\mathrm{0}\right) \\ $$$${B}\left(\mathrm{0},{b},\mathrm{0}\right) \\ $$$${A}\left(\mathrm{0},\mathrm{0},{c}\right) \\ $$$${center}\:{of}\:{circumcircle}\:{of}\:\Delta{ABC}\:{is}\:{D}. \\ $$$${D}\left(\alpha,\beta,\gamma\right) \\ $$$${we}\:{get}\:{from}\:{Q}\mathrm{149428}: \\ $$$$\alpha=\frac{{a}^{\mathrm{3}} \left({b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)}{\mathrm{2}\left({a}^{\mathrm{2}} {b}^{\mathrm{2}} +{b}^{\mathrm{2}} {c}^{\mathrm{2}} +{c}^{\mathrm{2}} {a}^{\mathrm{2}} \right)} \\ $$$$\beta=\frac{{b}^{\mathrm{3}} \left({c}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)}{\mathrm{2}\left({a}^{\mathrm{2}} {b}^{\mathrm{2}} +{b}^{\mathrm{2}} {c}^{\mathrm{2}} +{c}^{\mathrm{2}} {a}^{\mathrm{2}} \right)} \\ $$$$\gamma=\frac{{c}^{\mathrm{3}} \left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)}{\mathrm{2}\left({a}^{\mathrm{2}} {b}^{\mathrm{2}} +{b}^{\mathrm{2}} {c}^{\mathrm{2}} +{c}^{\mathrm{2}} {a}^{\mathrm{2}} \right)} \\ $$$${radius}\:{of}\:{circle}\:{is}\:{r}. \\ $$$${r}=\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left({b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)\left({c}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)}{{a}^{\mathrm{2}} {b}^{\mathrm{2}} +{b}^{\mathrm{2}} {c}^{\mathrm{2}} +{c}^{\mathrm{2}} {a}^{\mathrm{2}} }} \\ $$$$ \\ $$$$\overset{\rightarrow} {\boldsymbol{{OD}}}=\left(\alpha,\beta,\gamma\right) \\ $$$${normal}\:{of}\:{plane}\:\overset{\rightarrow} {\boldsymbol{{n}}}=\left(\frac{\mathrm{1}}{{a}},\frac{\mathrm{1}}{{b}},\frac{\mathrm{1}}{{c}}\right) \\ $$$${say}\:\overset{\rightarrow} {\boldsymbol{{u}}},\overset{\rightarrow} {\boldsymbol{{v}}}\:{are}\:{two}\:{perpendicular}\:{unit}\: \\ $$$${vectors}\:{in}\:{the}\:{plane}. \\ $$$$\overset{\rightarrow} {\boldsymbol{{OP}}}=\overset{\rightarrow} {\boldsymbol{{OD}}}+\overset{\rightarrow} {\boldsymbol{{DP}}}=\overset{\rightarrow} {\boldsymbol{{OD}}}+{r}\:\mathrm{cos}\:\theta\:\overset{\rightarrow} {\boldsymbol{{u}}}+{r}\:\mathrm{sin}\:\theta\:\overset{\rightarrow} {\boldsymbol{{v}}} \\ $$$$\overset{\rightarrow} {\boldsymbol{{u}}}\:{is}\:\bot\:\overset{\rightarrow} {\boldsymbol{{n}}}, \\ $$$${u}_{{x}} ×\frac{\mathrm{1}}{{a}}+{u}_{{y}} ×\frac{\mathrm{1}}{{b}}+{u}_{{z}} ×\frac{\mathrm{1}}{{c}}=\mathrm{0} \\ $$$${for}\:{example}\:{u}_{{x}} ={a},\:{u}_{{y}} =−{b},\:{u}_{{z}} =\mathrm{0} \\ $$$${i}.{e}.\:\overset{\rightarrow} {\boldsymbol{{u}}}\://\:\overset{\rightarrow} {\boldsymbol{{AB}}} \\ $$$$\overset{\rightarrow} {\boldsymbol{{v}}}=\overset{\rightarrow} {\boldsymbol{{n}}}×\overset{\rightarrow} {\boldsymbol{{u}}} \\ $$$$=\begin{vmatrix}{\frac{\mathrm{1}}{{a}}}&{\frac{\mathrm{1}}{{b}}\:}&{\frac{\mathrm{1}}{{c}}}\\{{a}}&{−{b}}&{\mathrm{0}}\end{vmatrix}=\left(\frac{{b}}{{c}},\frac{{a}}{{c}},−\frac{{a}}{{b}}−\frac{{b}}{{a}}\right) \\ $$$${since}\:\overset{\rightarrow} {\boldsymbol{{u}}},\:\overset{\rightarrow} {\boldsymbol{{v}}}\:{are}\:{unit}\:{vectors}, \\ $$$$\overset{\rightarrow} {\boldsymbol{{u}}}=\left(\frac{{a}}{\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }},−\frac{{b}}{\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }},\mathrm{0}\right) \\ $$$$\overset{\rightarrow} {\boldsymbol{{v}}}=\left(\frac{{b}}{\:{c}\sqrt{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\right)}},\frac{{a}}{\:{c}\sqrt{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\right)}},−\frac{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)}{\:{ab}\sqrt{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\right)}}\right) \\ $$$$\overset{\rightarrow} {\boldsymbol{{OP}}}=\left(\alpha,\beta,\gamma\right)+\frac{{r}}{\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\left({a},−{b},\:\mathrm{0}\right)\:\mathrm{cos}\:\theta+\frac{{r}}{\:\sqrt{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\right)}}\:\left(\frac{{b}}{{c}},\frac{{a}}{{c}},−\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{{ab}}\right)\mathrm{sin}\:\theta \\ $$$${or} \\ $$$$\begin{cases}{{x}=\alpha+\frac{{ar}}{\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\mathrm{cos}\:\theta+\frac{\left(\frac{{b}}{{c}}\right){r}}{\:\sqrt{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\right)}}\:\mathrm{sin}\:\theta}\\{{y}=\beta−\frac{{br}}{\:\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\mathrm{cos}\:\theta+\frac{\left(\frac{{a}}{{c}}\right){r}}{\:\sqrt{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\right)}}\:\mathrm{sin}\:\theta}\\{{z}=\gamma−\frac{\left(\frac{{a}}{{b}}+\frac{{b}}{{a}}\right){r}}{\:\sqrt{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{b}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\right)}}\:\mathrm{sin}\:\theta}\end{cases} \\ $$$${this}\:{is}\:{the}\:{equation}\:{of}\:{circumcircle} \\ $$$${with}\:\theta\:{as}\:{parameter}. \\ $$

Commented by Tawa11 last updated on 07/Aug/21

Kudox sir

$$\mathrm{Kudox}\:\mathrm{sir} \\ $$

Commented by mr W last updated on 07/Aug/21

Terms of Service

Privacy Policy

Contact: info@tinkutara.com