Question Number 149894 by ajfour last updated on 08/Aug/21

Commented byajfour last updated on 08/Aug/21

Find minimum and maximum values for the side of equilateral triangle shown.

Answered by mr W last updated on 08/Aug/21

Commented bymr W last updated on 08/Aug/21

Commented bymr W last updated on 08/Aug/21

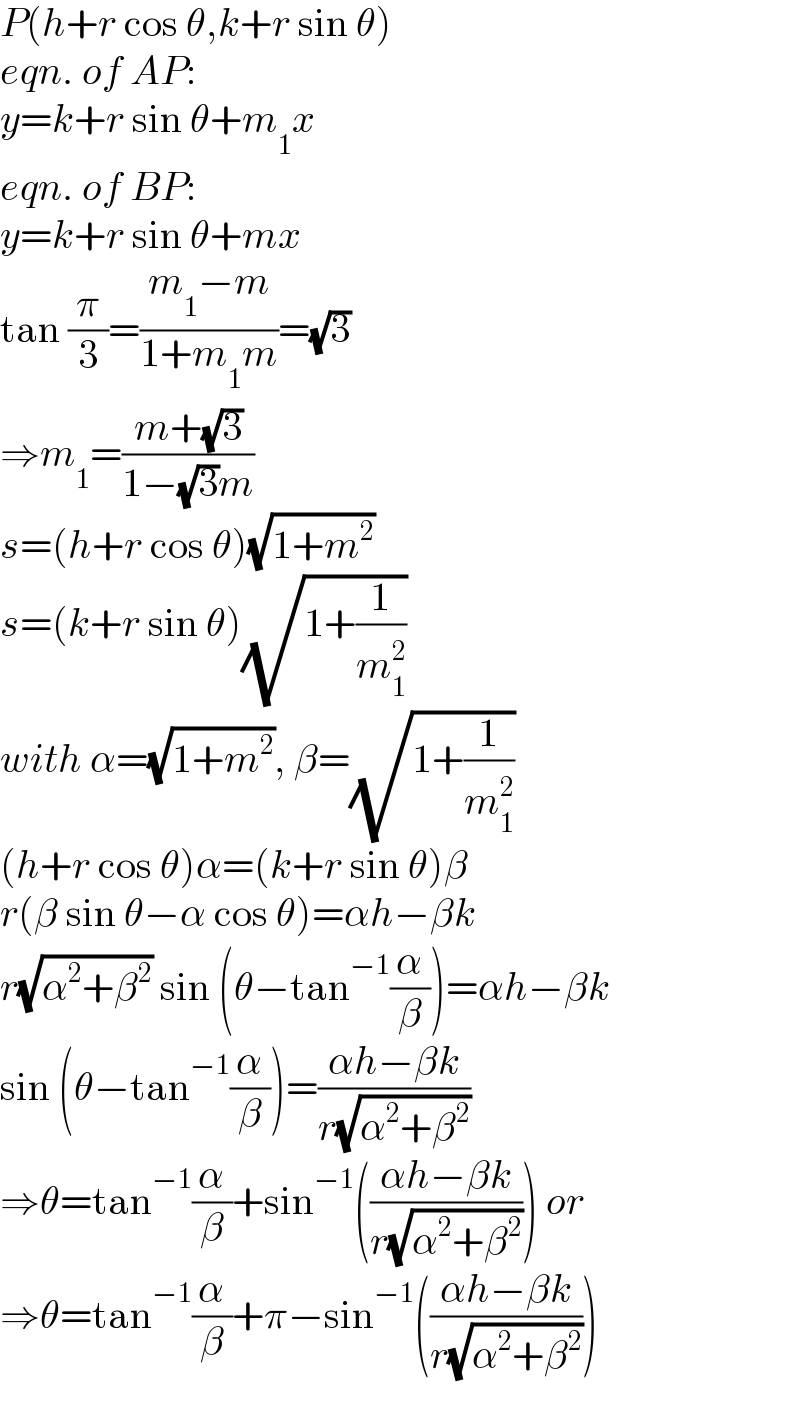
P(h+r cos θ,k+r sin θ) eqn. of AP: y=k+r sin θ+m_1 x eqn. of BP: y=k+r sin θ+mx tan (π/3)=((m_1 −m)/(1+m_1 m))=(√3) ⇒m_1 =((m+(√3))/(1−(√3)m)) s=(h+r cos θ)(√(1+m^2 )) s=(k+r sin θ)(√(1+(1/m_1 ^2 ))) with α=(√(1+m^2 )), β=(√(1+(1/m_1 ^2 ))) (h+r cos θ)α=(k+r sin θ)β r(β sin θ−α cos θ)=αh−βk r(√(α^2 +β^2 )) sin (θ−tan^(−1) (α/β))=αh−βk sin (θ−tan^(−1) (α/β))=((αh−βk)/(r(√(α^2 +β^2 )))) ⇒θ=tan^(−1) (α/β)+sin^(−1) (((αh−βk)/(r(√(α^2 +β^2 ))))) or ⇒θ=tan^(−1) (α/β)+π−sin^(−1) (((αh−βk)/(r(√(α^2 +β^2 )))))

Commented byajfour last updated on 08/Aug/21

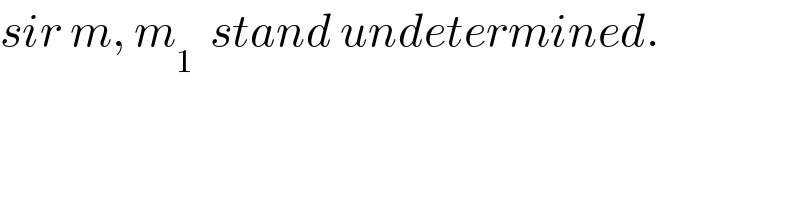
sir m, m_1 stand undetermined.

Commented byajfour last updated on 08/Aug/21

A(scos θ,0) ; B(0,ssin θ) P(((scos θ)/2)+((s(√3)sin θ)/2), ((ssin θ)/2)+((s(√3)cos θ)/2)) s^2 (cos θ+(√3)sin θ−2h)^2 +s^2 (sin θ+(√3)cos θ−2k)^2 =4r^2 s=((2r)/( (√((cos θ+(√3)sin θ−2h)^2 +(sin θ+(√3)cos θ−2k)^2 )))) s=((2r)/( (√(D(θ))))) D(θ)=4+4(h^2 +k^2 ) +4(√3)sin θcos θ −2(√3)(hsin θ+kcos θ) −2(hcos θ+ksin θ) =4(h^2 +k^2 +1)+2(√3)sin 2θ −2(√(h^2 +k^2 )){(√3)sin (θ+ϕ)+cos (θ−ϕ)} (dD/dθ)=0 ⇒ 2(√3)cos 2θ= (√(h^2 +k^2 )){(√3)cos (θ+ϕ)−sin (θ−ϕ)} where tan ϕ=(k/h) s=((2r)/( (√((cos θ+(√3)sin θ−2h)^2 +(sin θ+(√3)cos θ−2k)^2 ))))

Commented bymr W last updated on 08/Aug/21

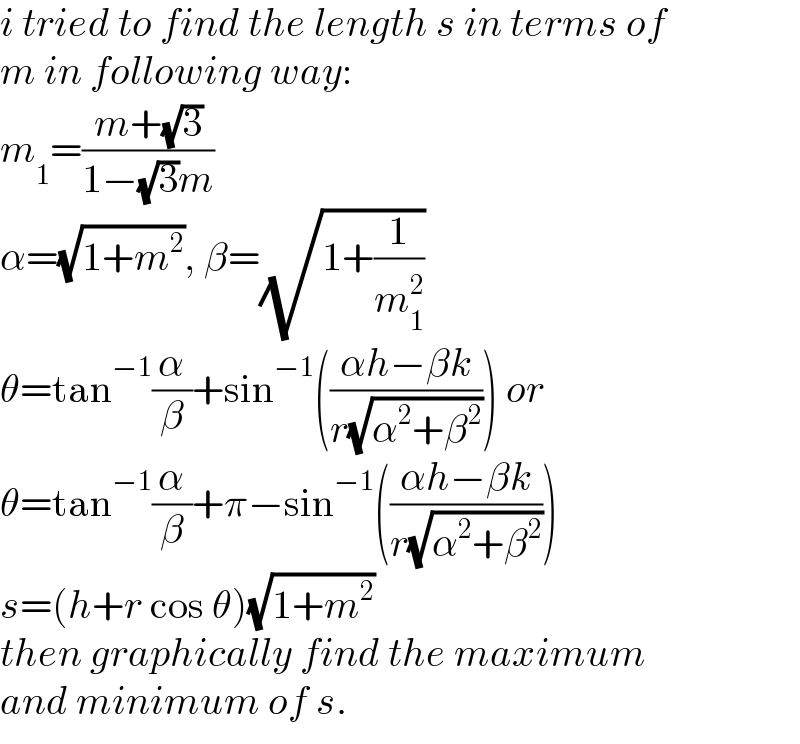
i tried to find the length s in terms of m in following way: m_1 =((m+(√3))/(1−(√3)m)) α=(√(1+m^2 )), β=(√(1+(1/m_1 ^2 ))) θ=tan^(−1) (α/β)+sin^(−1) (((αh−βk)/(r(√(α^2 +β^2 ))))) or θ=tan^(−1) (α/β)+π−sin^(−1) (((αh−βk)/(r(√(α^2 +β^2 ))))) s=(h+r cos θ)(√(1+m^2 )) then graphically find the maximum and minimum of s.

Commented bymr W last updated on 08/Aug/21

Commented bymr W last updated on 10/Aug/21

sir, please check: s^2 (cos θ+(√3)sin θ−2h/s)^2 +s^2 (sin θ+(√3)cos θ−2k/s)^2 =4r^2