etudier la continuite, et derivabilite: x^2 sin((1/x)) si≠0 et f(0)=0


Question and Answers Forum

All Questions Topic List
None Questions
Previous in All Question Next in All Question
Previous in None Next in None
Question Number 154337 by SANOGO last updated on 17/Sep/21

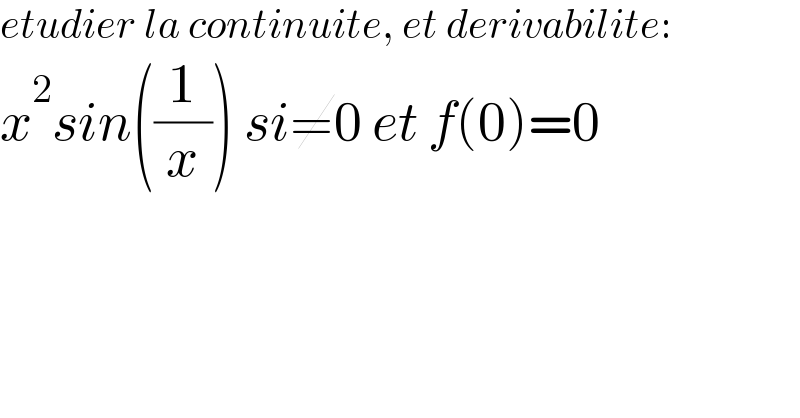
$${etudier}\:{la}\:{continuite},\:{et}\:{derivabilite}: \\ $$$${x}^{\mathrm{2}} {sin}\left(\frac{\mathrm{1}}{{x}}\right)\:{si}\neq\mathrm{0}\:{et}\:{f}\left(\mathrm{0}\right)=\mathrm{0} \\ $$
	Answered by puissant last updated on 17/Sep/21
	$posons: { ((f(0)=0 si x=0 )),((f(x)=x^2 sin((1/x)) , sinon..)) :} ∗ Continuite^� : Premiere methode: lim_(x→0^+ ) f(x)= lim_(x→0^− ) f(x)=0 et f(0)=0 alors, f(x) est continu sur R.. Deuxieme methode: les fonctons carre^� (x^2 ), sin et inverse ((1/x)) sont continus sur R donc leur compose^� f(x) est continu sur R.. ∗ Derivabilite^� : on a : lim_(x→0) ((f(x)−f(0))/(x−0))=lim_(x→0) ((x^2 sin((1/x)))/x) =lim_(x→0) xsin((1/x)).. on a: −1≤sin((1/x))≤1 →−x≤xsin((1/x))≤x ⇒ lim_(x→0) xsin((1/x))=0 d′apres le theoreme des gendarmes.. D′ou f est derivable sur R^∗ ..$
	$${posons}:\begin{cases}{{f}\left(\mathrm{0}\right)=\mathrm{0}\:{si}\:{x}=\mathrm{0}\:}\\{{f}\left({x}\right)={x}^{\mathrm{2}} {sin}\left(\frac{\mathrm{1}}{{x}}\right)\:,\:{sinon}..}\end{cases} \\ $$$$\ast\:{Continuit}\acute {{e}}\::\: \\ $$$$ \\ $$$${Premiere}\:{methode}: \\ $$$$ \\ $$$$\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}{f}\left({x}\right)=\:\underset{{x}\rightarrow\mathrm{0}^{−} } {\mathrm{lim}}{f}\left({x}\right)=\mathrm{0}\:{et}\:{f}\left(\mathrm{0}\right)=\mathrm{0} \\ $$$${alors},\:{f}\left({x}\right)\:{est}\:{continu}\:{sur}\:\mathbb{R}.. \\ $$$$ \\ $$$${Deuxieme}\:{methode}: \\ $$$$ \\ $$$${les}\:{fonctons}\:{carr}\acute {{e}}\left({x}^{\mathrm{2}} \right),\:{sin}\:{et}\:{inverse}\:\left(\frac{\mathrm{1}}{{x}}\right) \\ $$$${sont}\:{continus}\:{sur}\:\mathbb{R}\:{donc}\:{leur}\:{compos}\acute {{e}} \\ $$$${f}\left({x}\right)\:{est}\:{continu}\:{sur}\:\mathbb{R}.. \\ $$$$ \\ $$$$\ast\:{Derivabilit}\acute {{e}}\::\: \\ $$$$ \\ $$$${on}\:{a}\::\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{f}\left({x}\right)−{f}\left(\mathrm{0}\right)}{{x}−\mathrm{0}}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{x}^{\mathrm{2}} {sin}\left(\frac{\mathrm{1}}{{x}}\right)}{{x}} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}{xsin}\left(\frac{\mathrm{1}}{{x}}\right).. \\ $$$${on}\:{a}:\:\:−\mathrm{1}\leqslant{sin}\left(\frac{\mathrm{1}}{{x}}\right)\leqslant\mathrm{1}\:\rightarrow−{x}\leqslant{xsin}\left(\frac{\mathrm{1}}{{x}}\right)\leqslant{x} \\ $$$$\Rightarrow\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}{xsin}\left(\frac{\mathrm{1}}{{x}}\right)=\mathrm{0}\:{d}'{apres}\:{le}\:{theoreme} \\ $$$${des}\:{gendarmes}.. \\ $$$${D}'{ou}\:{f}\:{est}\:{derivable}\:{sur}\:\mathbb{R}^{\ast} ..\: \\ $$
	Commented by SANOGO last updated on 17/Sep/21

	$${le}\:{puissant}\:{a}\:{parler}\:{merci}\:{bien} \\ $$
	Commented by puissant last updated on 17/Sep/21

	$${De}\:{rien}\:.. \\ $$
	Answered by Jonathanwaweh last updated on 17/Sep/21
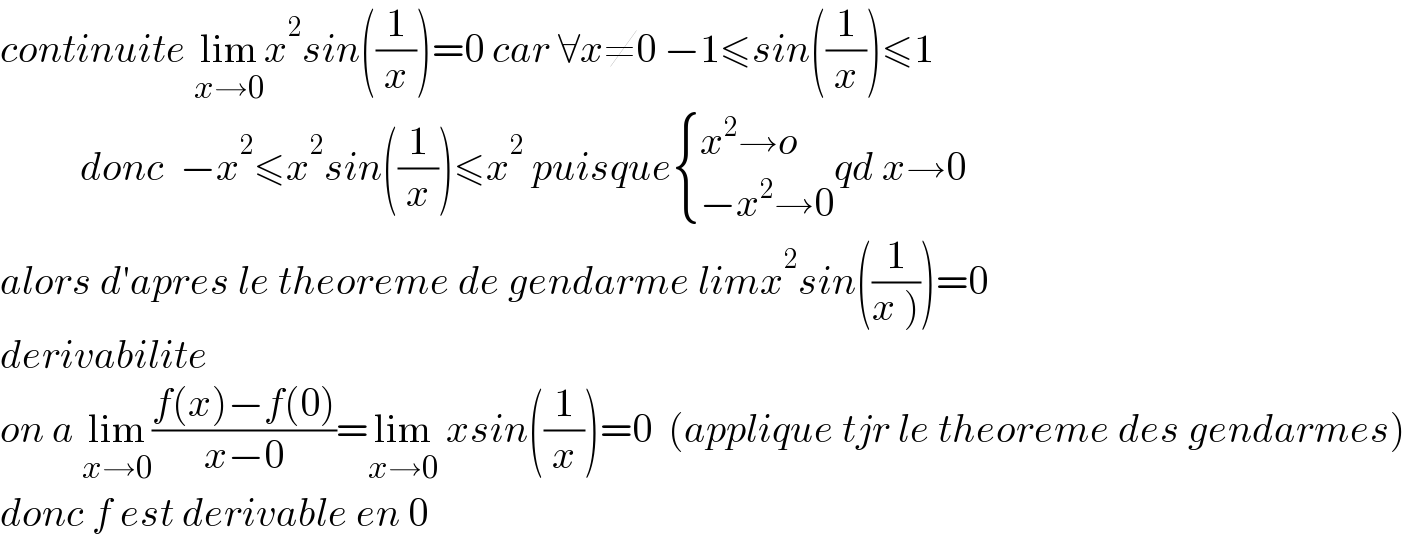
	$continuite lim_(x→0) x^2 sin((1/x))=0 car ∀x≠0 −1≤sin((1/x))≤1 donc −x^2 ≤x^2 sin((1/x))≤x^2 puisque { ((x^2 →o)),((−x^2 →0)) :}qd x→0 alors d′apres le theoreme de gendarme limx^2 sin((1/(x ))))=0 derivabilite on a lim_(x→0) ((f(x)−f(0))/(x−0))=lim_(x→0) xsin((1/x))=0 (applique tjr le theoreme des gendarmes) donc f est derivable en 0$
	$${continuite}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}{x}^{\mathrm{2}} {sin}\left(\frac{\mathrm{1}}{{x}}\right)=\mathrm{0}\:{car}\:\forall{x}\neq\mathrm{0}\:−\mathrm{1}\leqslant{sin}\left(\frac{\mathrm{1}}{{x}}\right)\leqslant\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:{donc}\:\:−{x}^{\mathrm{2}} \leqslant{x}^{\mathrm{2}} {sin}\left(\frac{\mathrm{1}}{{x}}\right)\leqslant{x}^{\mathrm{2}} \:{puisque\begin{cases}{{x}^{\mathrm{2}} \rightarrow{o}}\\{−{x}^{\mathrm{2}} \rightarrow\mathrm{0}}\end{cases}qd}\:{x}\rightarrow\mathrm{0} \\ $$$${alors}\:{d}'{apres}\:{le}\:{theoreme}\:{de}\:{gendarme}\:{limx}^{\mathrm{2}} {sin}\left(\frac{\mathrm{1}}{\left.{x}\:\right)}\right)=\mathrm{0} \\ $$$${derivabilite}\: \\ $$$${on}\:{a}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{f}\left({x}\right)−{f}\left(\mathrm{0}\right)}{{x}−\mathrm{0}}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:{xsin}\left(\frac{\mathrm{1}}{{x}}\right)=\mathrm{0}\:\:\left({applique}\:{tjr}\:{le}\:{theoreme}\:{des}\:{gendarmes}\right) \\ $$$${donc}\:{f}\:{est}\:{derivable}\:{en}\:\mathrm{0} \\ $$
	Commented by SANOGO last updated on 17/Sep/21

	$${merci}\:{bien} \\ $$
	Commented by puissant last updated on 17/Sep/21

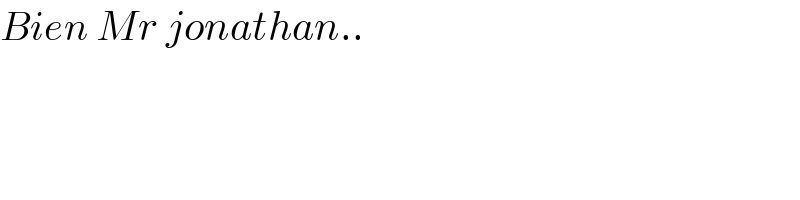
	$${Bien}\:{Mr}\:{jonathan}.. \\ $$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum