Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 157712 by ajfour last updated on 26/Oct/21

Commented by ajfour last updated on 26/Oct/21

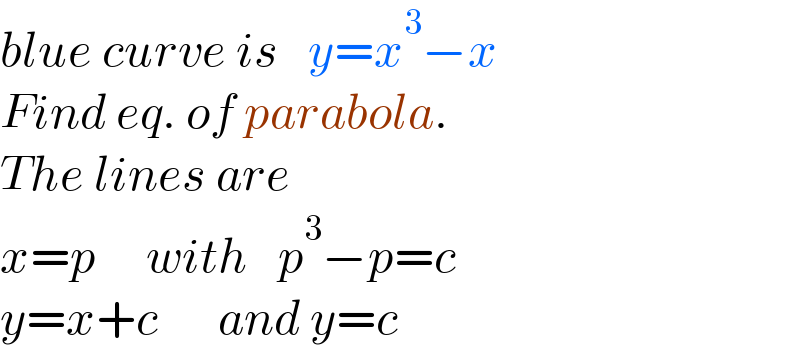
blue curve is y=x^3 −x Find eq. of parabola. The lines are x=p with p^3 −p=c y=x+c and y=c

$${blue}\:{curve}\:{is}\:\:\:{y}={x}^{\mathrm{3}} −{x} \\ $$$${Find}\:{eq}.\:{of}\:{parabola}. \\ $$$${The}\:{lines}\:{are} \\ $$$${x}={p}\:\:\:\:\:{with}\:\:\:{p}^{\mathrm{3}} −{p}={c} \\ $$$${y}={x}+{c}\:\:\:\:\:\:{and}\:{y}={c} \\ $$

Commented by ajfour last updated on 26/Oct/21

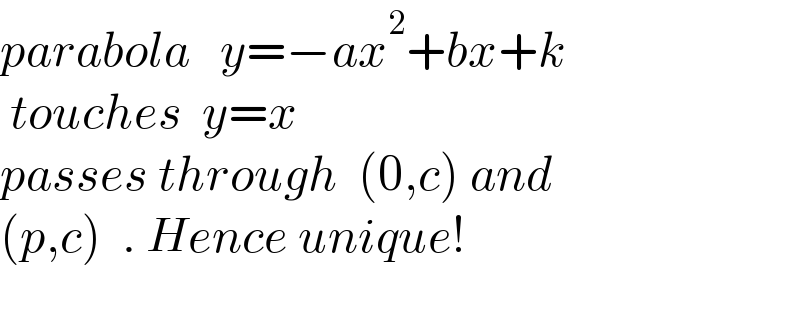
parabola y=−ax^2 +bx+k touches y=x passes through (0,c) and (p,c) . Hence unique!

$${parabola}\:\:\:{y}=−{ax}^{\mathrm{2}} +{bx}+{k} \\ $$$$\:{touches}\:\:{y}={x} \\ $$$${passes}\:{through}\:\:\left(\mathrm{0},{c}\right)\:{and} \\ $$$$\left({p},{c}\right)\:\:.\:{Hence}\:{unique}! \\ $$$$ \\ $$

Answered by ajfour last updated on 26/Oct/21

y=c+q^2 −A(x−q)^2 and p=2q so 8q^3 =2q+c x+c=c−Ax^2 +2Aqx+(1−A)q^2 Ax^2 +(2Aq−1)x+(1−A)q^2 =0 touches y=x+c hence (2Aq−1)^2 =4(1−A)q^2 ...(i) & 8q^3 =2q+c ...(ii) let q=s+t (2As+2At−1)^2 =4(1−A)(s+t)^2 if 2At+2As−1=m(s+t) ⇒ s+t=(1/(2A−m)) & m^2 =4(1−A) ⇒ A=1−(m^2 /4) (8/((2A−m)^3 ))=(2/((2A−m)))+c (8/((2−(m^2 /2)−m)^3 ))=(2/((2−(m^2 /2)−m)))+c 8=(2−(m^2 /2)−m)^2 +c(2−(m^2 /2)−m)^3 let m=2k 2=(1−k^2 −k)^2 +2c(1−k^2 −k)^3 2={(5/4)−(k−(1/2))^2 }^2 +2c{(5/4)−(k−(1/2))^2 }^3 let (k−(1/2))^2 =λ 2=((25)/(16))−((5λ)/2)+λ^2 +2c(((125)/(64))−λ^3 −((15λ^2 )/4)+((75λ)/(16))) 2cλ^3 +(((15c)/2)−1)λ^2 +((5/2)−((75c)/8))λ +((28−250c)/(64))=0

$${y}={c}+{q}^{\mathrm{2}} −{A}\left({x}−{q}\right)^{\mathrm{2}} \\ $$$${and}\:\:{p}=\mathrm{2}{q} \\ $$$${so}\:\:\mathrm{8}{q}^{\mathrm{3}} =\mathrm{2}{q}+{c} \\ $$$${x}+{c}={c}−{Ax}^{\mathrm{2}} +\mathrm{2}{Aqx}+\left(\mathrm{1}−{A}\right){q}^{\mathrm{2}} \\ $$$${Ax}^{\mathrm{2}} +\left(\mathrm{2}{Aq}−\mathrm{1}\right){x}+\left(\mathrm{1}−{A}\right){q}^{\mathrm{2}} =\mathrm{0} \\ $$$${touches}\:{y}={x}+{c}\:{hence} \\ $$$$\left(\mathrm{2}{Aq}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{4}\left(\mathrm{1}−{A}\right){q}^{\mathrm{2}} \:\:...\left({i}\right) \\ $$$$\& \\ $$$$\:\:\:\mathrm{8}{q}^{\mathrm{3}} =\mathrm{2}{q}+{c}\:\:\:\:...\left({ii}\right) \\ $$$${let}\:\:\:{q}={s}+{t} \\ $$$$\left(\mathrm{2}{As}+\mathrm{2}{At}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{4}\left(\mathrm{1}−{A}\right)\left({s}+{t}\right)^{\mathrm{2}} \\ $$$${if}\:\:\:\mathrm{2}{At}+\mathrm{2}{As}−\mathrm{1}={m}\left({s}+{t}\right) \\ $$$$\Rightarrow\:\:\:{s}+{t}=\frac{\mathrm{1}}{\mathrm{2}{A}−{m}} \\ $$$$\&\:\:\:\:{m}^{\mathrm{2}} =\mathrm{4}\left(\mathrm{1}−{A}\right) \\ $$$$\Rightarrow\:\:{A}=\mathrm{1}−\frac{{m}^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\frac{\mathrm{8}}{\left(\mathrm{2}{A}−{m}\right)^{\mathrm{3}} }=\frac{\mathrm{2}}{\left(\mathrm{2}{A}−{m}\right)}+{c} \\ $$$$\frac{\mathrm{8}}{\left(\mathrm{2}−\frac{{m}^{\mathrm{2}} }{\mathrm{2}}−{m}\right)^{\mathrm{3}} }=\frac{\mathrm{2}}{\left(\mathrm{2}−\frac{{m}^{\mathrm{2}} }{\mathrm{2}}−{m}\right)}+{c} \\ $$$$\mathrm{8}=\left(\mathrm{2}−\frac{{m}^{\mathrm{2}} }{\mathrm{2}}−{m}\right)^{\mathrm{2}} +{c}\left(\mathrm{2}−\frac{{m}^{\mathrm{2}} }{\mathrm{2}}−{m}\right)^{\mathrm{3}} \\ $$$${let}\:\:\:{m}=\mathrm{2}{k} \\ $$$$\mathrm{2}=\left(\mathrm{1}−{k}^{\mathrm{2}} −{k}\right)^{\mathrm{2}} +\mathrm{2}{c}\left(\mathrm{1}−{k}^{\mathrm{2}} −{k}\right)^{\mathrm{3}} \\ $$$$\mathrm{2}=\left\{\frac{\mathrm{5}}{\mathrm{4}}−\left({k}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \right\}^{\mathrm{2}} +\mathrm{2}{c}\left\{\frac{\mathrm{5}}{\mathrm{4}}−\left({k}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \right\}^{\mathrm{3}} \\ $$$${let}\:\:\left({k}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} =\lambda \\ $$$$\mathrm{2}=\frac{\mathrm{25}}{\mathrm{16}}−\frac{\mathrm{5}\lambda}{\mathrm{2}}+\lambda^{\mathrm{2}} +\mathrm{2}{c}\left(\frac{\mathrm{125}}{\mathrm{64}}−\lambda^{\mathrm{3}} −\frac{\mathrm{15}\lambda^{\mathrm{2}} }{\mathrm{4}}+\frac{\mathrm{75}\lambda}{\mathrm{16}}\right) \\ $$$$\mathrm{2}{c}\lambda^{\mathrm{3}} +\left(\frac{\mathrm{15}{c}}{\mathrm{2}}−\mathrm{1}\right)\lambda^{\mathrm{2}} +\left(\frac{\mathrm{5}}{\mathrm{2}}−\frac{\mathrm{75}{c}}{\mathrm{8}}\right)\lambda \\ $$$$+\frac{\mathrm{28}−\mathrm{250}{c}}{\mathrm{64}}=\mathrm{0} \\ $$

Answered by mr W last updated on 27/Oct/21

Commented by mr W last updated on 27/Oct/21

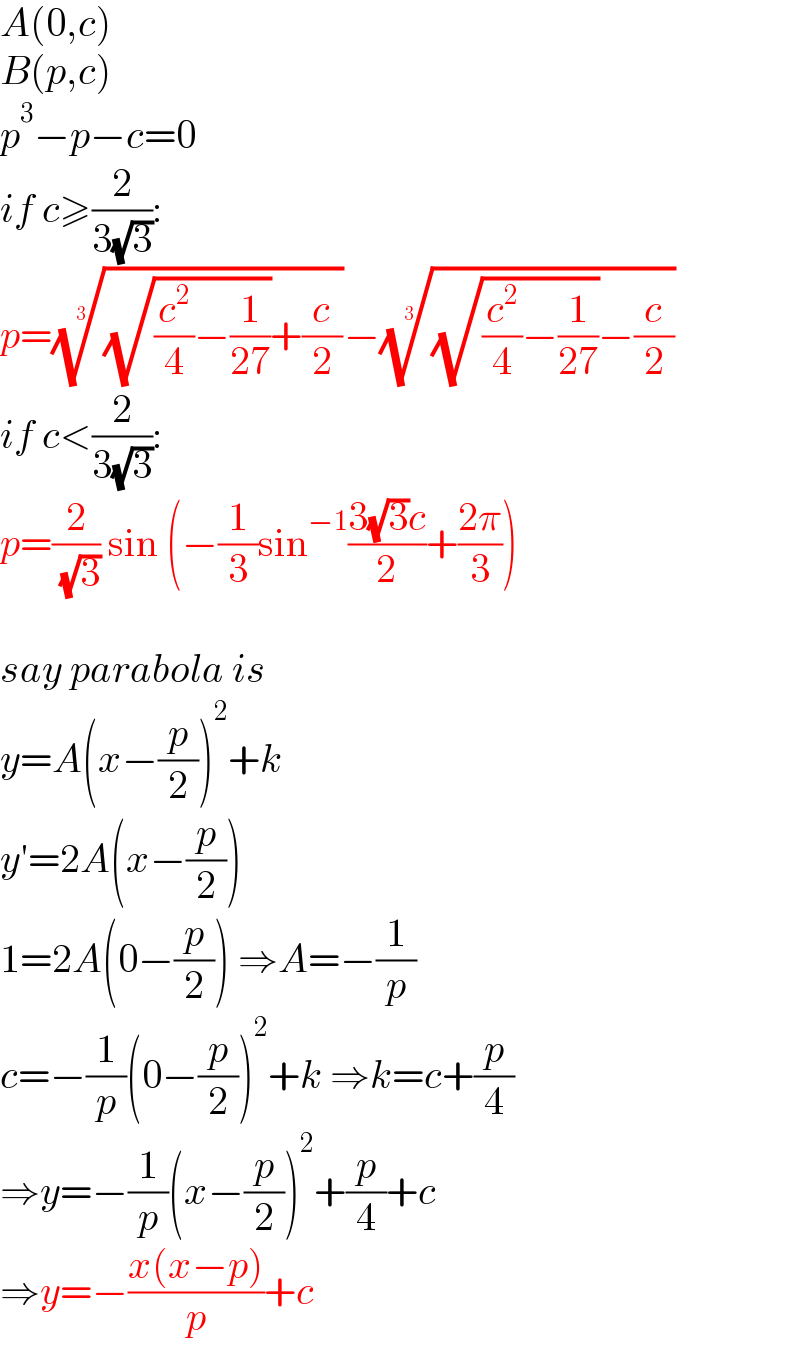
A(0,c) B(p,c) p^3 −p−c=0 if c≥(2/(3(√3))): p=(((√((c^2 /4)−(1/(27))))+(c/2)))^(1/3) −(((√((c^2 /4)−(1/(27))))−(c/2)))^(1/3) if c<(2/(3(√3))): p=(2/( (√3))) sin (−(1/3)sin^(−1) ((3(√3)c)/2)+((2π)/3)) say parabola is y=A(x−(p/2))^2 +k y′=2A(x−(p/2)) 1=2A(0−(p/2)) ⇒A=−(1/p) c=−(1/p)(0−(p/2))^2 +k ⇒k=c+(p/4) ⇒y=−(1/p)(x−(p/2))^2 +(p/4)+c ⇒y=−((x(x−p))/p)+c

$${A}\left(\mathrm{0},{c}\right) \\ $$$${B}\left({p},{c}\right) \\ $$$${p}^{\mathrm{3}} −{p}−{c}=\mathrm{0} \\ $$$${if}\:{c}\geqslant\frac{\mathrm{2}}{\mathrm{3}\sqrt{\mathrm{3}}}: \\ $$$${p}=\sqrt[{\mathrm{3}}]{\sqrt{\frac{{c}^{\mathrm{2}} }{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{27}}}+\frac{{c}}{\mathrm{2}}}−\sqrt[{\mathrm{3}}]{\sqrt{\frac{{c}^{\mathrm{2}} }{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{27}}}−\frac{{c}}{\mathrm{2}}} \\ $$$${if}\:{c}<\frac{\mathrm{2}}{\mathrm{3}\sqrt{\mathrm{3}}}: \\ $$$${p}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\:\mathrm{sin}\:\left(−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{sin}^{−\mathrm{1}} \frac{\mathrm{3}\sqrt{\mathrm{3}}{c}}{\mathrm{2}}+\frac{\mathrm{2}\pi}{\mathrm{3}}\right) \\ $$$$ \\ $$$${say}\:{parabola}\:{is} \\ $$$${y}={A}\left({x}−\frac{{p}}{\mathrm{2}}\right)^{\mathrm{2}} +{k} \\ $$$${y}'=\mathrm{2}{A}\left({x}−\frac{{p}}{\mathrm{2}}\right) \\ $$$$\mathrm{1}=\mathrm{2}{A}\left(\mathrm{0}−\frac{{p}}{\mathrm{2}}\right)\:\Rightarrow{A}=−\frac{\mathrm{1}}{{p}} \\ $$$${c}=−\frac{\mathrm{1}}{{p}}\left(\mathrm{0}−\frac{{p}}{\mathrm{2}}\right)^{\mathrm{2}} +{k}\:\Rightarrow{k}={c}+\frac{{p}}{\mathrm{4}} \\ $$$$\Rightarrow{y}=−\frac{\mathrm{1}}{{p}}\left({x}−\frac{{p}}{\mathrm{2}}\right)^{\mathrm{2}} +\frac{{p}}{\mathrm{4}}+{c} \\ $$$$\Rightarrow{y}=−\frac{{x}\left({x}−{p}\right)}{{p}}+{c} \\ $$

Commented by mr W last updated on 27/Oct/21

Commented by mr W last updated on 27/Oct/21

Commented by ajfour last updated on 27/Oct/21

thank you sir!

$${thank}\:{you}\:{sir}!\: \\ $$

Commented by Ahmed1hamouda last updated on 29/Oct/21

What applications do you use in thee enginering solution

$$\mathrm{What}\:\mathrm{applications}\:\mathrm{do}\:\mathrm{you}\:\mathrm{use}\:\mathrm{in}\:\mathrm{thee} \\ $$$$\mathrm{enginering}\:\mathrm{solution} \\ $$

Commented by mr W last updated on 29/Oct/21

Grapher

$${Grapher} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com