Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 161443 by smallEinstein last updated on 18/Dec/21

Answered by mathmax by abdo last updated on 18/Dec/21

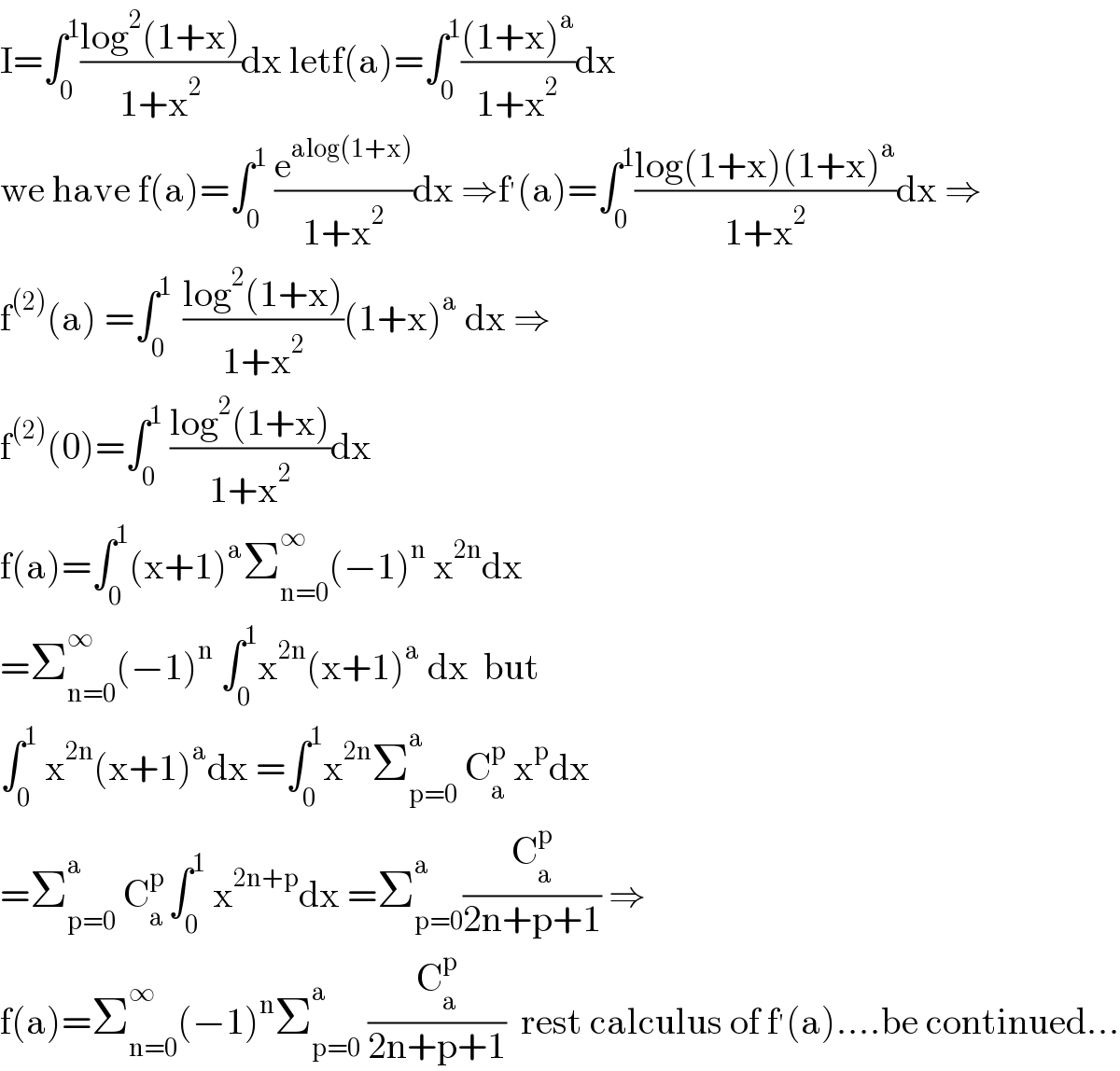
I=∫_0 ^1 ((log^2 (1+x))/(1+x^2 ))dx letf(a)=∫_0 ^1 (((1+x)^a )/(1+x^2 ))dx we have f(a)=∫_0 ^1 (e^(alog(1+x)) /(1+x^2 ))dx ⇒f^′ (a)=∫_0 ^1 ((log(1+x)(1+x)^a )/(1+x^2 ))dx ⇒ f^((2)) (a) =∫_0 ^(1 ) ((log^2 (1+x))/(1+x^2 ))(1+x)^a dx ⇒ f^((2)) (0)=∫_0 ^1 ((log^2 (1+x))/(1+x^2 ))dx f(a)=∫_0 ^1 (x+1)^a Σ_(n=0) ^∞ (−1)^n x^(2n) dx =Σ_(n=0) ^∞ (−1)^n ∫_0 ^1 x^(2n) (x+1)^a dx but ∫_0 ^1 x^(2n) (x+1)^a dx =∫_0 ^1 x^(2n) Σ_(p=0) ^(a ) C_a ^p x^p dx =Σ_(p=0) ^a C_a ^(p ) ∫_0 ^1 x^(2n+p) dx =Σ_(p=0) ^a (C_a ^p /(2n+p+1)) ⇒ f(a)=Σ_(n=0) ^∞ (−1)^n Σ_(p=0) ^a (C_a ^p /(2n+p+1)) rest calculus of f^′ (a)....be continued...

$$\mathrm{I}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{log}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{x}\right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\mathrm{letf}\left(\mathrm{a}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{a}} }{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\:\:\:\:\: \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{f}\left(\mathrm{a}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{e}^{\mathrm{alog}\left(\mathrm{1}+\mathrm{x}\right)} }{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\Rightarrow\mathrm{f}^{'} \left(\mathrm{a}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{log}\left(\mathrm{1}+\mathrm{x}\right)\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{a}} }{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{f}^{\left(\mathrm{2}\right)} \left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}\:} \:\frac{\mathrm{log}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{x}\right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{a}} \:\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{f}^{\left(\mathrm{2}\right)} \left(\mathrm{0}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{log}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{x}\right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$$$\mathrm{f}\left(\mathrm{a}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{x}+\mathrm{1}\right)^{\mathrm{a}} \sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{x}^{\mathrm{2n}} \mathrm{dx} \\ $$$$=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{\mathrm{n}} \:\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}^{\mathrm{2n}} \left(\mathrm{x}+\mathrm{1}\right)^{\mathrm{a}} \:\mathrm{dx}\:\:\mathrm{but} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{2n}} \left(\mathrm{x}+\mathrm{1}\right)^{\mathrm{a}} \mathrm{dx}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{x}^{\mathrm{2n}} \sum_{\mathrm{p}=\mathrm{0}} ^{\mathrm{a}\:} \:\mathrm{C}_{\mathrm{a}} ^{\mathrm{p}} \:\mathrm{x}^{\mathrm{p}} \mathrm{dx} \\ $$$$=\sum_{\mathrm{p}=\mathrm{0}} ^{\mathrm{a}} \:\mathrm{C}_{\mathrm{a}} ^{\mathrm{p}\:} \int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{2n}+\mathrm{p}} \mathrm{dx}\:=\sum_{\mathrm{p}=\mathrm{0}} ^{\mathrm{a}} \frac{\mathrm{C}_{\mathrm{a}} ^{\mathrm{p}} }{\mathrm{2n}+\mathrm{p}+\mathrm{1}}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{a}\right)=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{\mathrm{n}} \sum_{\mathrm{p}=\mathrm{0}} ^{\mathrm{a}} \:\frac{\mathrm{C}_{\mathrm{a}} ^{\mathrm{p}} }{\mathrm{2n}+\mathrm{p}+\mathrm{1}}\:\:\mathrm{rest}\:\mathrm{calculus}\:\mathrm{of}\:\mathrm{f}^{'} \left(\mathrm{a}\right)....\mathrm{be}\:\mathrm{continued}... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com