Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 17260 by Umar math last updated on 03/Jul/17

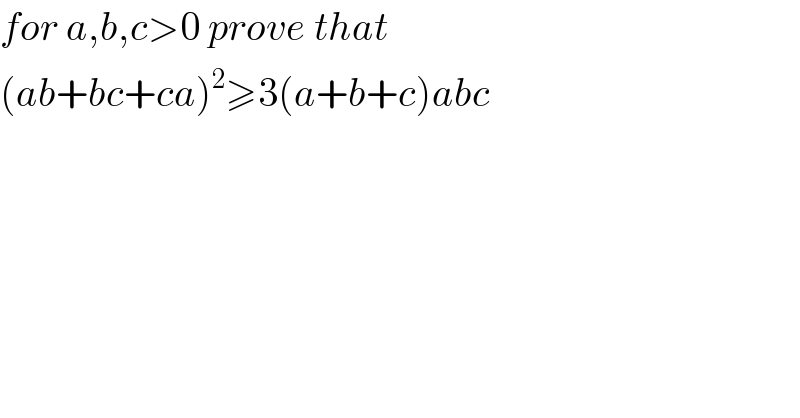
for a,b,c>0 prove that (ab+bc+ca)^2 ≥3(a+b+c)abc

$${for}\:{a},{b},{c}>\mathrm{0}\:{prove}\:{that} \\ $$ $$\left({ab}+{bc}+{ca}\right)^{\mathrm{2}} \geqslant\mathrm{3}\left({a}+{b}+{c}\right){abc} \\ $$

Commented byprakash jain last updated on 03/Jul/17

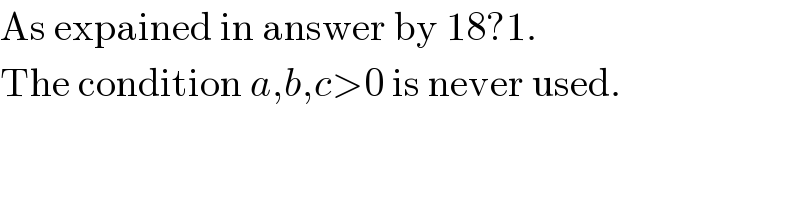
As expained in answer by 18?1. The condition a,b,c>0 is never used.

$$\mathrm{As}\:\mathrm{expained}\:\mathrm{in}\:\mathrm{answer}\:\mathrm{by}\:\mathrm{18}?\mathrm{1}. \\ $$ $$\mathrm{The}\:\mathrm{condition}\:{a},{b},{c}>\mathrm{0}\:\mathrm{is}\:\mathrm{never}\:\mathrm{used}. \\ $$

Answered by 18±1 last updated on 05/Jul/17

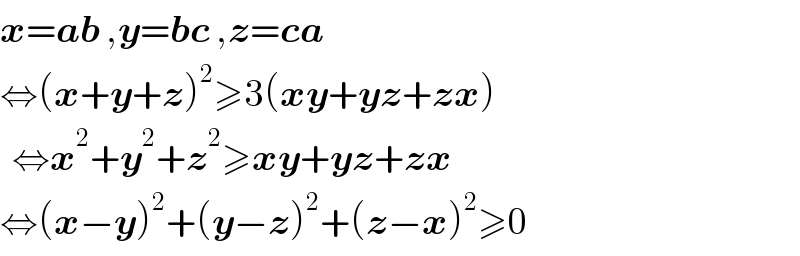
x=ab ,y=bc ,z=ca ⇔(x+y+z)^2 ≥3(xy+yz+zx) ⇔x^2 +y^2 +z^2 ≥xy+yz+zx ⇔(x−y)^2 +(y−z)^2 +(z−x)^2 ≥0

$$\boldsymbol{{x}}=\boldsymbol{{ab}}\:,\boldsymbol{{y}}=\boldsymbol{{bc}}\:,\boldsymbol{{z}}=\boldsymbol{{ca}} \\ $$ $$\Leftrightarrow\left(\boldsymbol{{x}}+\boldsymbol{{y}}+\boldsymbol{{z}}\right)^{\mathrm{2}} \geqslant\mathrm{3}\left(\boldsymbol{{xy}}+\boldsymbol{{yz}}+\boldsymbol{{zx}}\right) \\ $$ $$\:\:\Leftrightarrow\boldsymbol{{x}}^{\mathrm{2}} +\boldsymbol{{y}}^{\mathrm{2}} +\boldsymbol{{z}}^{\mathrm{2}} \geqslant\boldsymbol{{xy}}+\boldsymbol{{yz}}+\boldsymbol{{zx}} \\ $$ $$\Leftrightarrow\left(\boldsymbol{{x}}−\boldsymbol{{y}}\right)^{\mathrm{2}} +\left(\boldsymbol{{y}}−\boldsymbol{{z}}\right)^{\mathrm{2}} +\left(\boldsymbol{{z}}−\boldsymbol{{x}}\right)^{\mathrm{2}} \geqslant\mathrm{0} \\ $$

Commented by18±1 last updated on 03/Jul/17

sorry 3(xy+yz+zx) not a,b,c

$$\boldsymbol{{sorry}}\:\mathrm{3}\left(\boldsymbol{{xy}}+\boldsymbol{{yz}}+\boldsymbol{{zx}}\right)\:\boldsymbol{{not}}\:\boldsymbol{{a}},\boldsymbol{{b}},\boldsymbol{{c}} \\ $$

Commented byUmar math last updated on 03/Jul/17

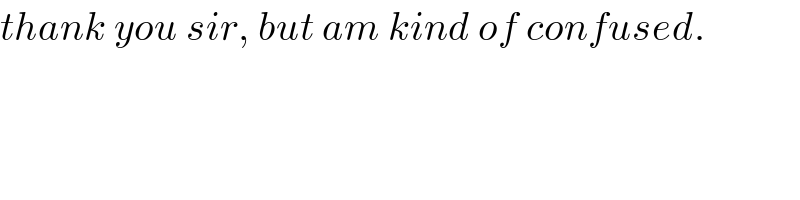
thank you sir, but am kind of confused.

$${thank}\:{you}\:{sir},\:{but}\:{am}\:{kind}\:{of}\:{confused}. \\ $$

Commented by1234Hello last updated on 03/Jul/17

I am in doubt that the result is proved or not?!

$$\mathrm{I}\:\mathrm{am}\:\mathrm{in}\:\mathrm{doubt}\:\mathrm{that}\:\mathrm{the}\:\mathrm{result}\:\mathrm{is}\:\mathrm{proved} \\ $$ $$\mathrm{or}\:\mathrm{not}?! \\ $$

Commented byprakash jain last updated on 03/Jul/17

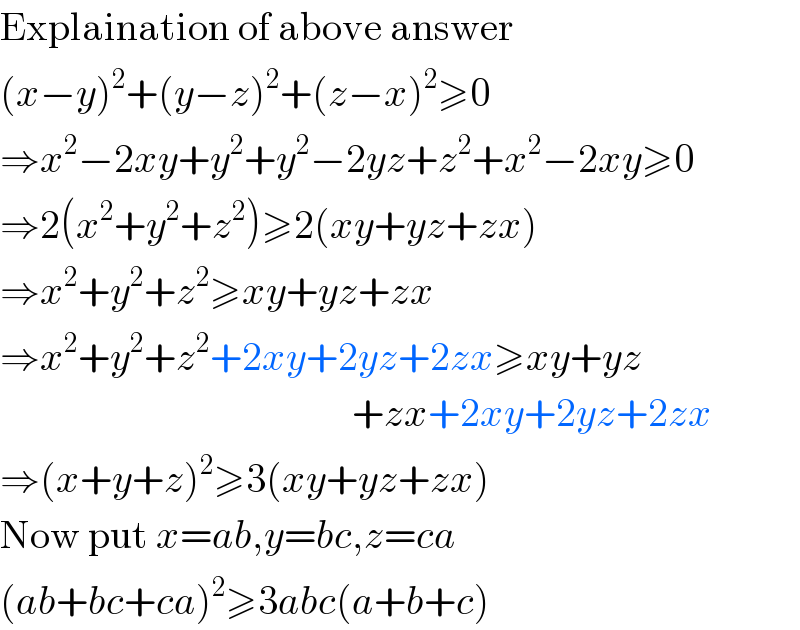
Explaination of above answer (x−y)^2 +(y−z)^2 +(z−x)^2 ≥0 ⇒x^2 −2xy+y^2 +y^2 −2yz+z^2 +x^2 −2xy≥0 ⇒2(x^2 +y^2 +z^2 )≥2(xy+yz+zx) ⇒x^2 +y^2 +z^2 ≥xy+yz+zx ⇒x^2 +y^2 +z^2 +2xy+2yz+2zx≥xy+yz +zx+2xy+2yz+2zx ⇒(x+y+z)^2 ≥3(xy+yz+zx) Now put x=ab,y=bc,z=ca (ab+bc+ca)^2 ≥3abc(a+b+c)

$$\mathrm{Explaination}\:\mathrm{of}\:\mathrm{above}\:\mathrm{answer} \\ $$ $$\left({x}−{y}\right)^{\mathrm{2}} +\left({y}−{z}\right)^{\mathrm{2}} +\left({z}−{x}\right)^{\mathrm{2}} \geqslant\mathrm{0} \\ $$ $$\Rightarrow{x}^{\mathrm{2}} −\mathrm{2}{xy}+{y}^{\mathrm{2}} +{y}^{\mathrm{2}} −\mathrm{2}{yz}+{z}^{\mathrm{2}} +{x}^{\mathrm{2}} −\mathrm{2}{xy}\geqslant\mathrm{0} \\ $$ $$\Rightarrow\mathrm{2}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)\geqslant\mathrm{2}\left({xy}+{yz}+{zx}\right) \\ $$ $$\Rightarrow{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \geqslant{xy}+{yz}+{zx} \\ $$ $$\Rightarrow{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} +\mathrm{2}{xy}+\mathrm{2}{yz}+\mathrm{2}{zx}\geqslant{xy}+{yz} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+{zx}+\mathrm{2}{xy}+\mathrm{2}{yz}+\mathrm{2}{zx} \\ $$ $$\Rightarrow\left({x}+{y}+{z}\right)^{\mathrm{2}} \geqslant\mathrm{3}\left({xy}+{yz}+{zx}\right) \\ $$ $$\mathrm{Now}\:\mathrm{put}\:{x}={ab},{y}={bc},{z}={ca} \\ $$ $$\left({ab}+{bc}+{ca}\right)^{\mathrm{2}} \geqslant\mathrm{3}{abc}\left({a}+{b}+{c}\right) \\ $$

Commented byUmar math last updated on 03/Jul/17

thanks a bunch sir, am clear know

$${thanks}\:{a}\:{bunch}\:{sir},\:{am}\:{clear}\:{know} \\ $$

Commented byTinkutara last updated on 04/Jul/17

Thanks Sir!

$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com