Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 184048 by cortano1 last updated on 02/Jan/23

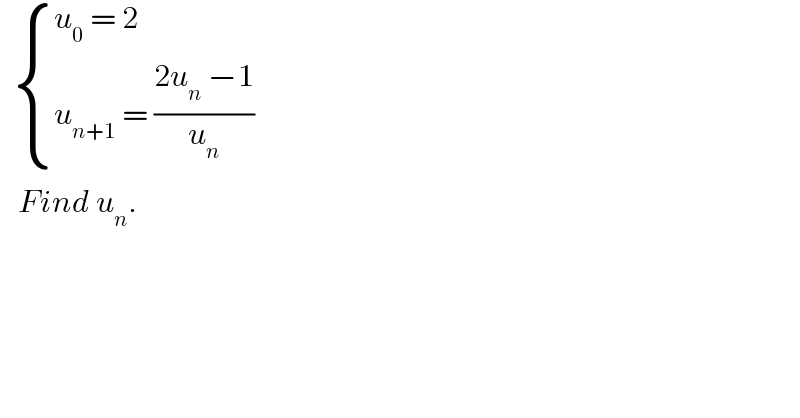
{ ((u_0 = 2)),((u_(n+1) = ((2u_n −1)/u_n ))) :} Find u_n .

$$\:\:\begin{cases}{{u}_{\mathrm{0}} \:=\:\mathrm{2}}\\{{u}_{{n}+\mathrm{1}} \:=\:\frac{\mathrm{2}{u}_{{n}} \:−\mathrm{1}}{{u}_{{n}} }}\end{cases} \\ $$$$\:\:\:{Find}\:{u}_{{n}} . \\ $$

Answered by SEKRET last updated on 02/Jan/23

n=0 u_1 =(3/2) n=1 u_2 = (4/3) n=2 u_3 = (5/4) n = 3 u_4 = (6/5) n=4 u_5 = (7/6) (2/1); (3/2);(4/3) ;(5/4);(6/5);(7/6) U_n = ((n+2)/(n+1)) ABDULAZIZ ABDUVALIYEV

$$\:\:\boldsymbol{\mathrm{n}}=\mathrm{0}\:\:\:\:\:\boldsymbol{\mathrm{u}}_{\mathrm{1}} =\frac{\mathrm{3}}{\mathrm{2}}\:\:\:\:\:\boldsymbol{\mathrm{n}}=\mathrm{1}\:\:\:\:\:\:\boldsymbol{\mathrm{u}}_{\mathrm{2}} \:=\:\:\frac{\mathrm{4}}{\mathrm{3}} \\ $$$$\boldsymbol{\mathrm{n}}=\mathrm{2}\:\:\:\:\:\:\boldsymbol{\mathrm{u}}_{\mathrm{3}} =\:\frac{\mathrm{5}}{\mathrm{4}}\:\:\:\:\:\:\boldsymbol{\mathrm{n}}\:=\:\mathrm{3}\:\:\:\:\boldsymbol{\mathrm{u}}_{\mathrm{4}} =\:\:\frac{\mathrm{6}}{\mathrm{5}} \\ $$$$\:\boldsymbol{\mathrm{n}}=\mathrm{4}\:\:\:\boldsymbol{\mathrm{u}}_{\mathrm{5}} =\:\frac{\mathrm{7}}{\mathrm{6}} \\ $$$$\:\:\:\:\:\frac{\mathrm{2}}{\mathrm{1}};\:\frac{\mathrm{3}}{\mathrm{2}};\frac{\mathrm{4}}{\mathrm{3}}\:;\frac{\mathrm{5}}{\mathrm{4}};\frac{\mathrm{6}}{\mathrm{5}};\frac{\mathrm{7}}{\mathrm{6}} \\ $$$$\:\:\boldsymbol{\mathrm{U}}_{\boldsymbol{\mathrm{n}}} =\:\frac{\boldsymbol{\mathrm{n}}+\mathrm{2}}{\boldsymbol{\mathrm{n}}+\mathrm{1}}\:\: \\ $$$$\:\boldsymbol{{ABDULAZIZ}}\:\:\:\boldsymbol{{ABDUVALIYEV}} \\ $$

Commented by mr W last updated on 02/Jan/23

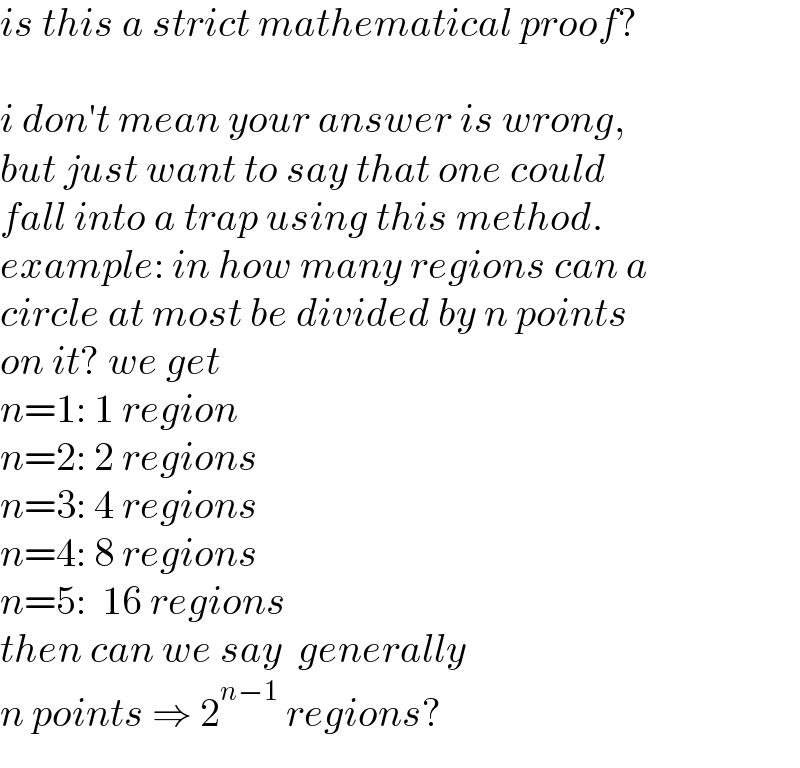
is this a strict mathematical proof? i don′t mean your answer is wrong, but just want to say that one could fall into a trap using this method. example: in how many regions can a circle at most be divided by n points on it? we get n=1: 1 region n=2: 2 regions n=3: 4 regions n=4: 8 regions n=5: 16 regions then can we say generally n points ⇒ 2^(n−1) regions?

$${is}\:{this}\:{a}\:{strict}\:{mathematical}\:{proof}? \\ $$$$ \\ $$$${i}\:{don}'{t}\:{mean}\:{your}\:{answer}\:{is}\:{wrong}, \\ $$$${but}\:{just}\:{want}\:{to}\:{say}\:{that}\:{one}\:{could}\: \\ $$$${fall}\:{into}\:{a}\:{trap}\:{using}\:{this}\:{method}.\: \\ $$$${example}:\:{in}\:{how}\:{many}\:{regions}\:{can}\:{a}\: \\ $$$${circle}\:{at}\:{most}\:{be}\:{divided}\:{by}\:{n}\:{points}\: \\ $$$${on}\:{it}?\:{we}\:{get} \\ $$$${n}=\mathrm{1}:\:\mathrm{1}\:{region} \\ $$$${n}=\mathrm{2}:\:\mathrm{2}\:{regions} \\ $$$${n}=\mathrm{3}:\:\mathrm{4}\:{regions} \\ $$$${n}=\mathrm{4}:\:\mathrm{8}\:{regions} \\ $$$${n}=\mathrm{5}:\:\:\mathrm{16}\:{regions} \\ $$$${then}\:{can}\:{we}\:{say}\:\:{generally}\: \\ $$$${n}\:{points}\:\Rightarrow\:\mathrm{2}^{{n}−\mathrm{1}} \:{regions}? \\ $$

Commented by Acem last updated on 02/Jan/23

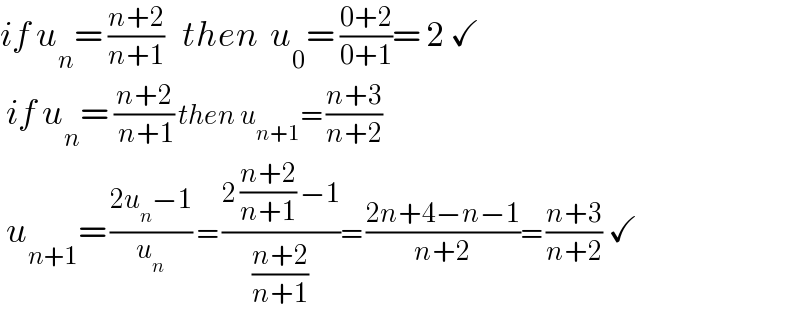
if u_n = ((n+2)/(n+1)) then u_0 = ((0+2)/(0+1))= 2 ✓ if u_n = ((n+2)/( n+1)) then u_(n+1) = ((n+3)/(n+2)) u_(n+1) = ((2u_n −1)/u_n ) = ((2 ((n+2)/(n+1)) −1)/((n+2)/(n+1)))= ((2n+4−n−1)/(n+2))= ((n+3)/(n+2)) ✓

$${if}\:{u}_{{n}} =\:\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}\:\:\:{then}\:\:{u}_{\mathrm{0}} =\:\frac{\mathrm{0}+\mathrm{2}}{\mathrm{0}+\mathrm{1}}=\:\mathrm{2}\:\checkmark \\ $$$$\:{if}\:{u}_{{n}} =\:\frac{{n}+\mathrm{2}}{\:{n}+\mathrm{1}}\:{then}\:{u}_{{n}+\mathrm{1}} =\:\frac{{n}+\mathrm{3}}{{n}+\mathrm{2}}\: \\ $$$$\:{u}_{{n}+\mathrm{1}} =\:\frac{\mathrm{2}{u}_{{n}} −\mathrm{1}}{{u}_{{n}} }\:=\:\frac{\mathrm{2}\:\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}\:−\mathrm{1}}{\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}}=\:\frac{\mathrm{2}{n}+\mathrm{4}−{n}−\mathrm{1}}{{n}+\mathrm{2}}=\:\frac{{n}+\mathrm{3}}{{n}+\mathrm{2}}\:\checkmark \\ $$

Commented by mr W last updated on 02/Jan/23

nice sir! this is a strict proof for u_n =((n+2)/(n+1)) when we already know u_n =((n+2)/(n+1)). but how to find u_n generally? let′s say u_0 =c. c could be any real value.

$${nice}\:{sir}! \\ $$$${this}\:{is}\:{a}\:{strict}\:{proof}\:{for}\:{u}_{{n}} =\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}} \\ $$$${when}\:{we}\:{already}\:{know}\:{u}_{{n}} =\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}. \\ $$$${but}\:{how}\:{to}\:{find}\:{u}_{{n}} \:{generally}?\:{let}'{s} \\ $$$${say}\:{u}_{\mathrm{0}} ={c}.\:{c}\:{could}\:{be}\:{any}\:{real}\:{value}. \\ $$

Commented by SEKRET last updated on 02/Jan/23

thanks sir.

$$\boldsymbol{\mathrm{thanks}}\:\:\boldsymbol{\mathrm{sir}}.\:\: \\ $$

Commented by Acem last updated on 02/Jan/23

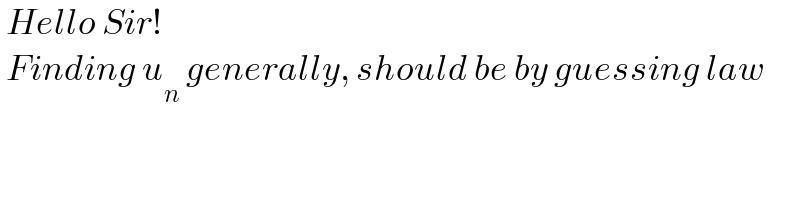
Hello Sir! Finding u_n generally, should be by guessing law

$$\:{Hello}\:{Sir}! \\ $$$$\:{Finding}\:{u}_{{n}} \:{generally},\:{should}\:{be}\:{by}\:{guessing}\:{law} \\ $$

Commented by mr W last updated on 02/Jan/23

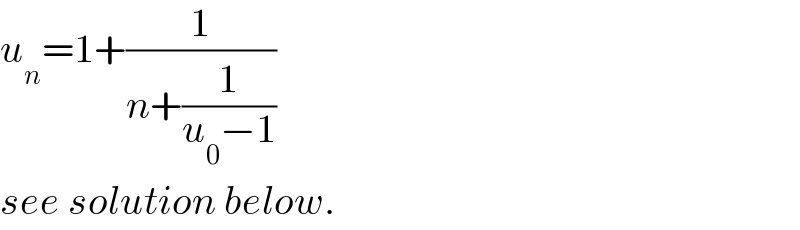
u_n =1+(1/(n+(1/(u_0 −1)))) see solution below.

$${u}_{{n}} =\mathrm{1}+\frac{\mathrm{1}}{{n}+\frac{\mathrm{1}}{{u}_{\mathrm{0}} −\mathrm{1}}} \\ $$$${see}\:{solution}\:{below}. \\ $$

Answered by cortano2 last updated on 02/Jan/23

u_0 =2 u_1 =3/2 u_2 =4/3 u_3 =5/4 u_n =((n+2)/(n+1))

$${u}_{\mathrm{0}} =\mathrm{2} \\ $$$${u}_{\mathrm{1}} =\mathrm{3}/\mathrm{2} \\ $$$${u}_{\mathrm{2}} =\mathrm{4}/\mathrm{3} \\ $$$${u}_{\mathrm{3}} =\mathrm{5}/\mathrm{4} \\ $$$${u}_{{n}} =\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}} \\ $$

Commented by mr W last updated on 02/Jan/23

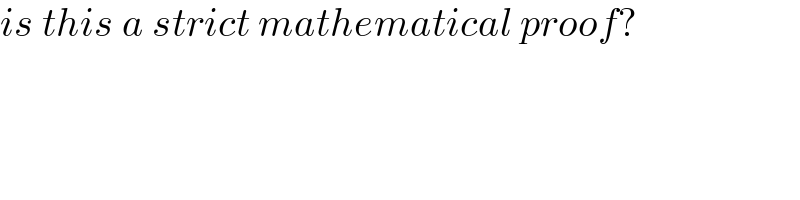
is this a strict mathematical proof?

$${is}\:{this}\:{a}\:{strict}\:{mathematical}\:{proof}? \\ $$

Commented by Acem last updated on 02/Jan/23

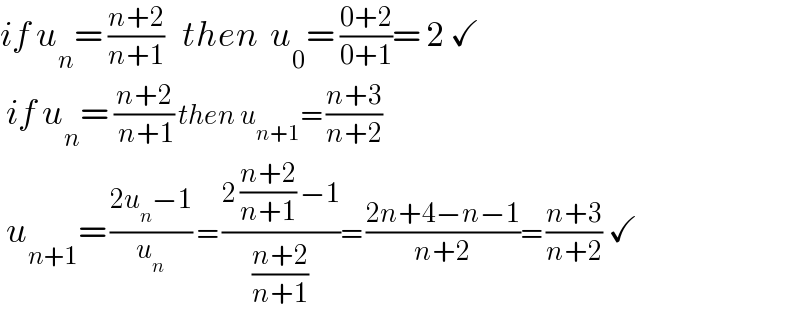
if u_n = ((n+2)/(n+1)) then u_0 = ((0+2)/(0+1))= 2 ✓ if u_n = ((n+2)/( n+1)) then u_(n+1) = ((n+3)/(n+2)) u_(n+1) = ((2u_n −1)/u_n ) = ((2 ((n+2)/(n+1)) −1)/((n+2)/(n+1)))= ((2n+4−n−1)/(n+2))= ((n+3)/(n+2)) ✓

$${if}\:{u}_{{n}} =\:\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}\:\:\:{then}\:\:{u}_{\mathrm{0}} =\:\frac{\mathrm{0}+\mathrm{2}}{\mathrm{0}+\mathrm{1}}=\:\mathrm{2}\:\checkmark \\ $$$$\:{if}\:{u}_{{n}} =\:\frac{{n}+\mathrm{2}}{\:{n}+\mathrm{1}}\:{then}\:{u}_{{n}+\mathrm{1}} =\:\frac{{n}+\mathrm{3}}{{n}+\mathrm{2}}\: \\ $$$$\:{u}_{{n}+\mathrm{1}} =\:\frac{\mathrm{2}{u}_{{n}} −\mathrm{1}}{{u}_{{n}} }\:=\:\frac{\mathrm{2}\:\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}\:−\mathrm{1}}{\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}}=\:\frac{\mathrm{2}{n}+\mathrm{4}−{n}−\mathrm{1}}{{n}+\mathrm{2}}=\:\frac{{n}+\mathrm{3}}{{n}+\mathrm{2}}\:\checkmark \\ $$

Answered by a.lgnaoui last updated on 02/Jan/23

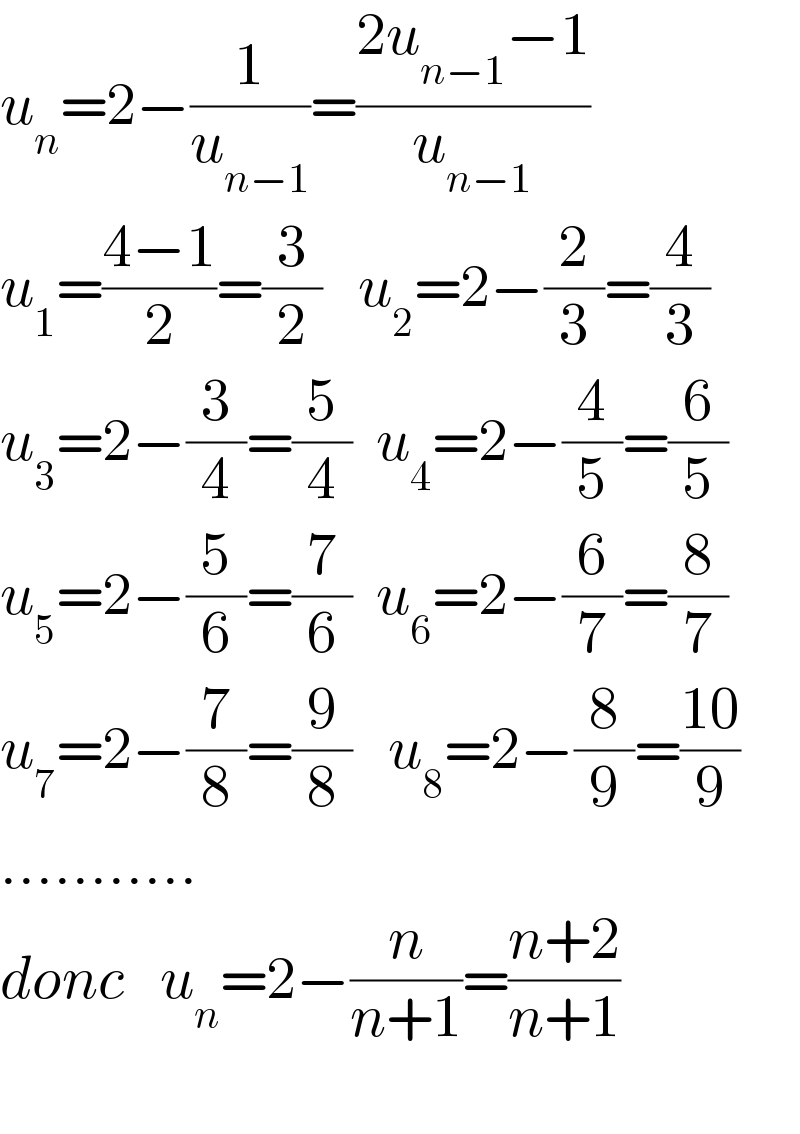
u_n =2−(1/u_(n−1) )=((2u_(n−1) −1)/u_(n−1) ) u_1 =((4−1)/2)=(3/2) u_2 =2−(2/3)=(4/3) u_3 =2−(3/4)=(5/4) u_4 =2−(4/5)=(6/5) u_5 =2−(5/6)=(7/6) u_6 =2−(6/7)=(8/7) u_7 =2−(7/8)=(9/8) u_8 =2−(8/9)=((10)/9) ........... donc u_n =2−(n/(n+1))=((n+2)/(n+1))

$${u}_{{n}} =\mathrm{2}−\frac{\mathrm{1}}{{u}_{{n}−\mathrm{1}} }=\frac{\mathrm{2}{u}_{{n}−\mathrm{1}} −\mathrm{1}}{{u}_{{n}−\mathrm{1}} } \\ $$$${u}_{\mathrm{1}} =\frac{\mathrm{4}−\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{3}}{\mathrm{2}}\:\:\:{u}_{\mathrm{2}} =\mathrm{2}−\frac{\mathrm{2}}{\mathrm{3}}=\frac{\mathrm{4}}{\mathrm{3}} \\ $$$${u}_{\mathrm{3}} =\mathrm{2}−\frac{\mathrm{3}}{\mathrm{4}}=\frac{\mathrm{5}}{\mathrm{4}}\:\:{u}_{\mathrm{4}} =\mathrm{2}−\frac{\mathrm{4}}{\mathrm{5}}=\frac{\mathrm{6}}{\mathrm{5}} \\ $$$${u}_{\mathrm{5}} =\mathrm{2}−\frac{\mathrm{5}}{\mathrm{6}}=\frac{\mathrm{7}}{\mathrm{6}}\:\:{u}_{\mathrm{6}} =\mathrm{2}−\frac{\mathrm{6}}{\mathrm{7}}=\frac{\mathrm{8}}{\mathrm{7}} \\ $$$${u}_{\mathrm{7}} =\mathrm{2}−\frac{\mathrm{7}}{\mathrm{8}}=\frac{\mathrm{9}}{\mathrm{8}}\:\:\:{u}_{\mathrm{8}} =\mathrm{2}−\frac{\mathrm{8}}{\mathrm{9}}=\frac{\mathrm{10}}{\mathrm{9}} \\ $$$$........... \\ $$$${donc}\:\:\:{u}_{{n}} =\mathrm{2}−\frac{{n}}{{n}+\mathrm{1}}=\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}} \\ $$$$ \\ $$

Answered by CrispyXYZ last updated on 02/Jan/23

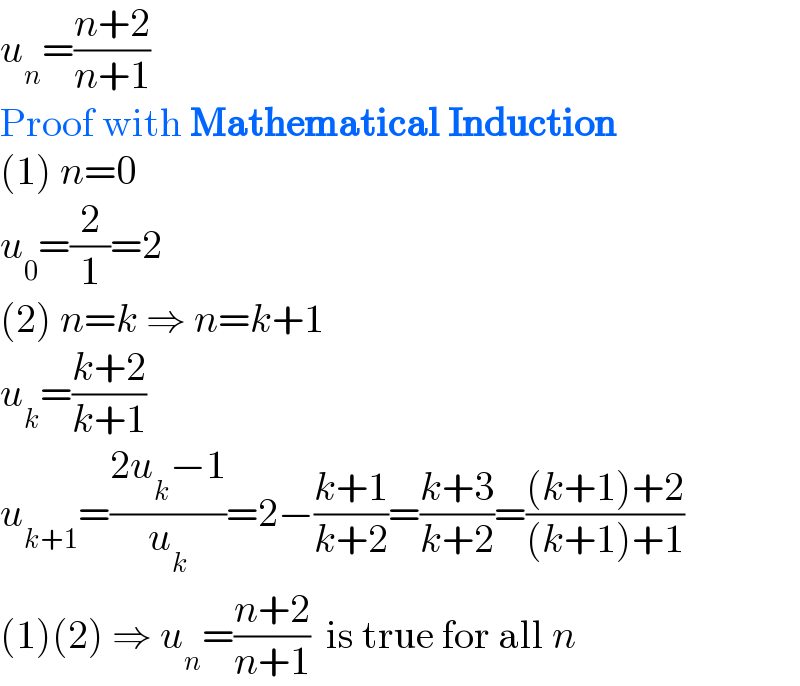
u_n =((n+2)/(n+1)) Proof with Mathematical Induction (1) n=0 u_0 =(2/1)=2 (2) n=k ⇒ n=k+1 u_k =((k+2)/(k+1)) u_(k+1) =((2u_k −1)/u_k )=2−((k+1)/(k+2))=((k+3)/(k+2))=(((k+1)+2)/((k+1)+1)) (1)(2) ⇒ u_n =((n+2)/(n+1)) is true for all n

$${u}_{{n}} =\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}} \\ $$$$\mathrm{Proof}\:\mathrm{with}\:\boldsymbol{\mathrm{Mathematical}}\:\boldsymbol{\mathrm{Induction}} \\ $$$$\left(\mathrm{1}\right)\:{n}=\mathrm{0} \\ $$$${u}_{\mathrm{0}} =\frac{\mathrm{2}}{\mathrm{1}}=\mathrm{2} \\ $$$$\left(\mathrm{2}\right)\:{n}={k}\:\Rightarrow\:{n}={k}+\mathrm{1} \\ $$$${u}_{{k}} =\frac{{k}+\mathrm{2}}{{k}+\mathrm{1}} \\ $$$${u}_{{k}+\mathrm{1}} =\frac{\mathrm{2}{u}_{{k}} −\mathrm{1}}{{u}_{{k}} }=\mathrm{2}−\frac{{k}+\mathrm{1}}{{k}+\mathrm{2}}=\frac{{k}+\mathrm{3}}{{k}+\mathrm{2}}=\frac{\left({k}+\mathrm{1}\right)+\mathrm{2}}{\left({k}+\mathrm{1}\right)+\mathrm{1}} \\ $$$$\left(\mathrm{1}\right)\left(\mathrm{2}\right)\:\Rightarrow\:{u}_{{n}} =\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}}\:\:\mathrm{is}\:\mathrm{true}\:\mathrm{for}\:\mathrm{all}\:{n} \\ $$

Answered by mr W last updated on 02/Jan/23

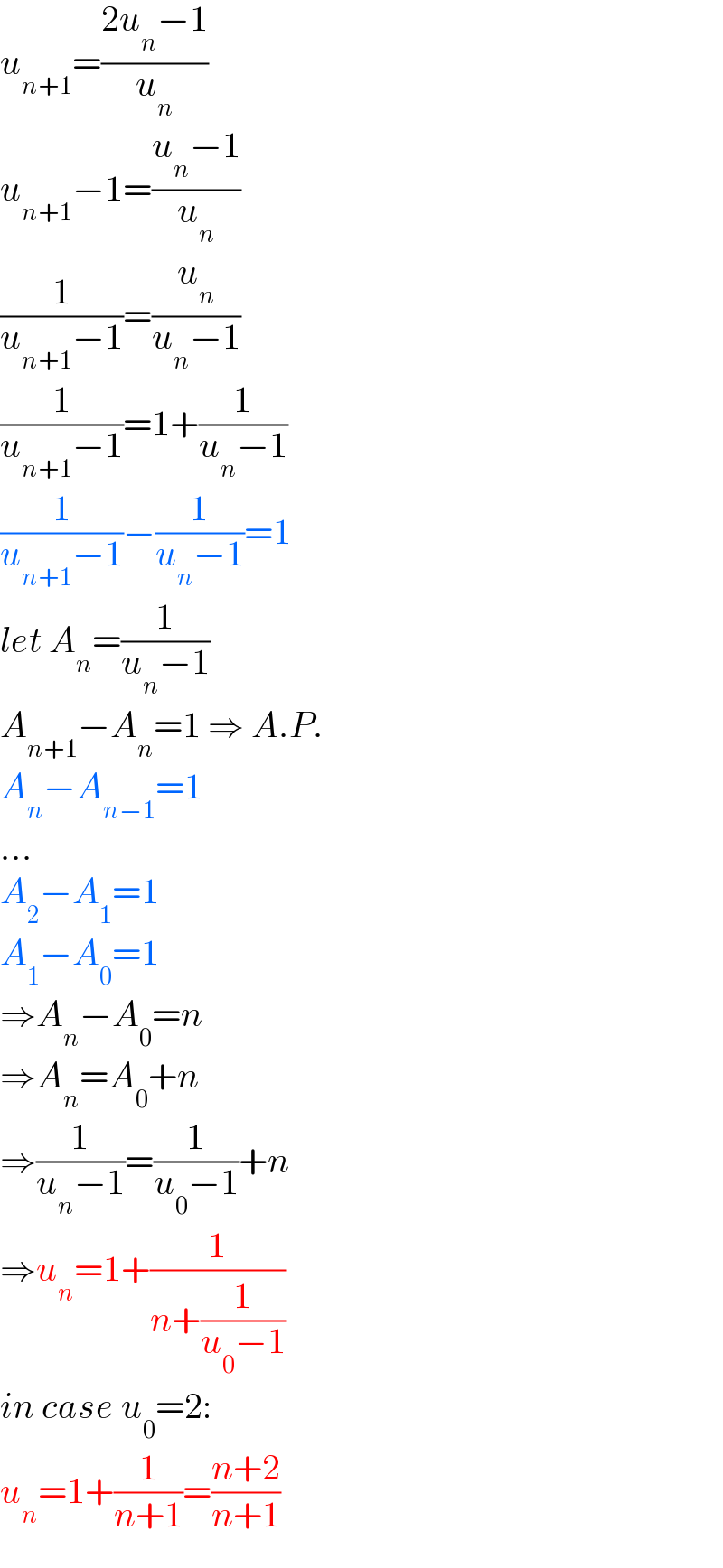
u_(n+1) =((2u_n −1)/u_n ) u_(n+1) −1=((u_n −1)/u_n ) (1/(u_(n+1) −1))=(u_n /(u_n −1)) (1/(u_(n+1) −1))=1+(1/(u_n −1)) (1/(u_(n+1) −1))−(1/(u_n −1))=1 let A_n =(1/(u_n −1)) A_(n+1) −A_n =1 ⇒ A.P. A_n −A_(n−1) =1 ... A_2 −A_1 =1 A_1 −A_0 =1 ⇒A_n −A_0 =n ⇒A_n =A_0 +n ⇒(1/(u_n −1))=(1/(u_0 −1))+n ⇒u_n =1+(1/(n+(1/(u_0 −1)))) in case u_0 =2: u_n =1+(1/(n+1))=((n+2)/(n+1))

$${u}_{{n}+\mathrm{1}} =\frac{\mathrm{2}{u}_{{n}} −\mathrm{1}}{{u}_{{n}} } \\ $$$${u}_{{n}+\mathrm{1}} −\mathrm{1}=\frac{{u}_{{n}} −\mathrm{1}}{{u}_{{n}} } \\ $$$$\frac{\mathrm{1}}{{u}_{{n}+\mathrm{1}} −\mathrm{1}}=\frac{{u}_{{n}} }{{u}_{{n}} −\mathrm{1}} \\ $$$$\frac{\mathrm{1}}{{u}_{{n}+\mathrm{1}} −\mathrm{1}}=\mathrm{1}+\frac{\mathrm{1}}{{u}_{{n}} −\mathrm{1}} \\ $$$$\frac{\mathrm{1}}{{u}_{{n}+\mathrm{1}} −\mathrm{1}}−\frac{\mathrm{1}}{{u}_{{n}} −\mathrm{1}}=\mathrm{1} \\ $$$${let}\:{A}_{{n}} =\frac{\mathrm{1}}{{u}_{{n}} −\mathrm{1}} \\ $$$${A}_{{n}+\mathrm{1}} −{A}_{{n}} =\mathrm{1}\:\Rightarrow\:{A}.{P}. \\ $$$${A}_{{n}} −{A}_{{n}−\mathrm{1}} =\mathrm{1} \\ $$$$... \\ $$$${A}_{\mathrm{2}} −{A}_{\mathrm{1}} =\mathrm{1} \\ $$$${A}_{\mathrm{1}} −{A}_{\mathrm{0}} =\mathrm{1} \\ $$$$\Rightarrow{A}_{{n}} −{A}_{\mathrm{0}} ={n} \\ $$$$\Rightarrow{A}_{{n}} ={A}_{\mathrm{0}} +{n} \\ $$$$\Rightarrow\frac{\mathrm{1}}{{u}_{{n}} −\mathrm{1}}=\frac{\mathrm{1}}{{u}_{\mathrm{0}} −\mathrm{1}}+{n} \\ $$$$\Rightarrow{u}_{{n}} =\mathrm{1}+\frac{\mathrm{1}}{{n}+\frac{\mathrm{1}}{{u}_{\mathrm{0}} −\mathrm{1}}} \\ $$$${in}\:{case}\:{u}_{\mathrm{0}} =\mathrm{2}: \\ $$$${u}_{{n}} =\mathrm{1}+\frac{\mathrm{1}}{{n}+\mathrm{1}}=\frac{{n}+\mathrm{2}}{{n}+\mathrm{1}} \\ $$

Commented by Acem last updated on 02/Jan/23

Nice Sir! thanks question, bec my Eng. what′s A.P.

$${Nice}\:{Sir}!\:{thanks} \\ $$$$\:{question},\:{bec}\:{my}\:{Eng}.\:{what}'{s}\:{A}.{P}. \\ $$

Commented by cortano1 last updated on 03/Jan/23

aritmetic progression

$${aritmetic}\:{progression} \\ $$

Commented by mr W last updated on 03/Jan/23

A.P.=arithmethic progression G.P.=geometric progression

$${A}.{P}.={arithmethic}\:{progression} \\ $$$${G}.{P}.={geometric}\:{progression} \\ $$

Commented by Acem last updated on 04/Jan/23

Thx friends!

$${Thx}\:{friends}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com