
Question Number 27600 by abdo imad last updated on 10/Jan/18
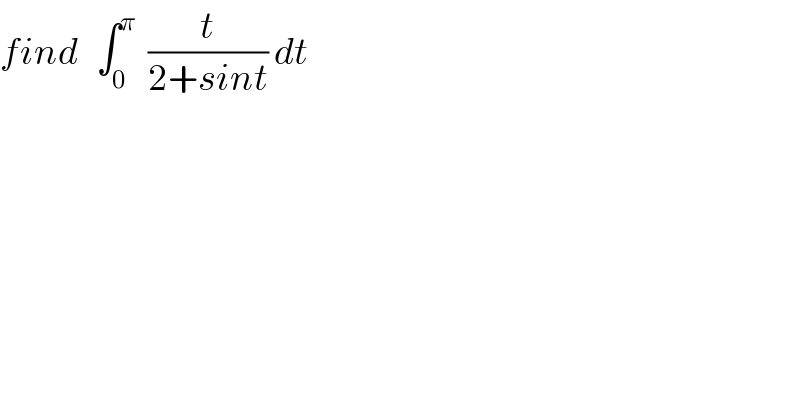
$${find}\:\:\:\int_{\mathrm{0}} ^{\pi} \:\:\frac{{t}}{\mathrm{2}+{sint}}\:{dt} \\ $$
Commented by abdo imad last updated on 11/Jan/18
![let put I=∫_0 ^π (t/(2+sint))dt and the ch. tan((t/2))=x⇔ t=2arctanx I= ∫_0 ^∞ ((2arctanx)/(2+((2x)/(1+x^2 )))) ((2dx)/(1+x^2 ))= 2 ∫_0 ^∞ ((arctanx)/(1+x^2 +2x))dx =2∫_0 ^∞ ((arctanx)/((x+1)^2 ))dx then the integration per parts give ∫_0 ^∞ ((arctanx)/((x+1)^2 ))dx= [−(1/(x+1)) arctanx]_0 ^∝ − ∫_0 ^∞ −(1/(x+1)) (dx/(1+x^2 )) = ∫_0 ^∞ (dx/((x+1)(1+x^2 ))) let decompose rational fraction F(x)= (1/((x+1)(1+x^2 )))⇒F(x)= (a/(x+1)) + ((bx+c)/(1+x^2 )) a= lim_(x−>−1^ ) (x+1)F(x)= (1/2) lim_(x−>∝) xF(x)=0=a+b⇒b=−(1/2) so F(x)= (1/(2(x+1))) + ((((−x)/2)+c)/(1+x^2 )) ,F(0)=1= (1/2)+c⇒c=(1/2) F(x)= (1/(2(x+1))) −(1/2) ((x−1)/(1+x^2 )) I= ∫_0 ^∞ (dx/(x+1)) −∫_0 ^∞ ((x−1)/(1+x^2 ))dx = ∫_0 ^∞ (dx/(x+1)) −(1/2) ∫_0 ^∞ ((2x−2)/(1+x^2 ))dx I= ∫_0 ^∞ ( (1/(x+1)) −(1/2)((2x)/(1+x^2 )))dx + ∫_0 ^∞ (dx/(1+x^2 )) I=[ln/x+1/−(1/2)ln/1+x^2 /]_0 ^∝ + (π/2) I=[ln/((x+1)/(√(1+x^2 )))/ ]_0 ^∝ +(π/2)=0+(π/2) ∫_0 ^π (t/(2+sint))dt = (π/2) .](Q27644.png)
$${let}\:{put}\:{I}=\int_{\mathrm{0}} ^{\pi} \:\:\frac{{t}}{\mathrm{2}+{sint}}{dt}\:{and}\:{the}\:{ch}.\:{tan}\left(\frac{{t}}{\mathrm{2}}\right)={x}\Leftrightarrow\: \\ $$$${t}=\mathrm{2}{arctanx} \\ $$$${I}=\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{\mathrm{2}{arctanx}}{\mathrm{2}+\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }}\:\frac{\mathrm{2}{dx}}{\mathrm{1}+{x}^{\mathrm{2}} }=\:\mathrm{2}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctanx}}{\mathrm{1}+{x}^{\mathrm{2}} +\mathrm{2}{x}}{dx} \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{arctanx}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }{dx}\:\:{then}\:{the}\:{integration}\:{per}\:{parts}\:{give} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{{arctanx}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }{dx}=\:\left[−\frac{\mathrm{1}}{{x}+\mathrm{1}}\:{arctanx}\right]_{\mathrm{0}} ^{\propto} −\:\int_{\mathrm{0}} ^{\infty} −\frac{\mathrm{1}}{{x}+\mathrm{1}}\:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$=\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\:{let}\:{decompose}\:{rational}\:{fraction} \\ $$$${F}\left({x}\right)=\:\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\Rightarrow{F}\left({x}\right)=\:\frac{{a}}{{x}+\mathrm{1}}\:+\:\frac{{bx}+{c}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${a}=\:{lim}_{{x}−>−\mathrm{1}^{} } \:\left({x}+\mathrm{1}\right){F}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${lim}_{{x}−>\propto} {xF}\left({x}\right)=\mathrm{0}={a}+{b}\Rightarrow{b}=−\frac{\mathrm{1}}{\mathrm{2}}\:{so} \\ $$$${F}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{2}\left({x}+\mathrm{1}\right)}\:+\:\:\frac{\frac{−{x}}{\mathrm{2}}+{c}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\:,{F}\left(\mathrm{0}\right)=\mathrm{1}=\:\frac{\mathrm{1}}{\mathrm{2}}+{c}\Rightarrow{c}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${F}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{2}\left({x}+\mathrm{1}\right)}\:−\frac{\mathrm{1}}{\mathrm{2}}\:\frac{{x}−\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${I}=\:\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{{x}+\mathrm{1}}\:−\int_{\mathrm{0}} ^{\infty} \:\:\frac{{x}−\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$$=\:\int_{\mathrm{0}} ^{\infty} \:\frac{{dx}}{{x}+\mathrm{1}}\:\:−\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{2}{x}−\mathrm{2}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$${I}=\:\int_{\mathrm{0}} ^{\infty} \left(\:\:\:\frac{\mathrm{1}}{{x}+\mathrm{1}}\:−\frac{\mathrm{1}}{\mathrm{2}}\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right){dx}\:+\:\int_{\mathrm{0}} ^{\infty} \:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${I}=\left[{ln}/{x}+\mathrm{1}/−\frac{\mathrm{1}}{\mathrm{2}}{ln}/\mathrm{1}+{x}^{\mathrm{2}} /\right]_{\mathrm{0}} ^{\propto} \:+\:\frac{\pi}{\mathrm{2}} \\ $$$${I}=\left[{ln}/\frac{{x}+\mathrm{1}}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}/\:\right]_{\mathrm{0}} ^{\propto} +\frac{\pi}{\mathrm{2}}=\mathrm{0}+\frac{\pi}{\mathrm{2}} \\ $$$$\int_{\mathrm{0}} ^{\pi} \:\frac{{t}}{\mathrm{2}+{sint}}{dt}\:=\:\frac{\pi}{\mathrm{2}}\:. \\ $$
