
Question Number 38465 by maxmathsup by imad last updated on 25/Jun/18
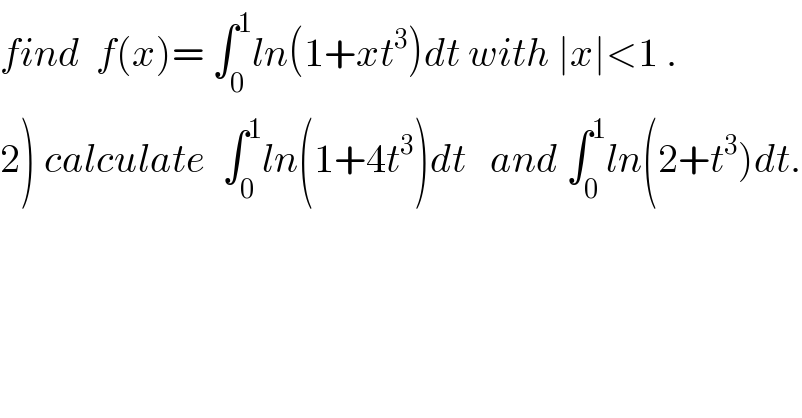
$${find}\:\:{f}\left({x}\right)=\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+{xt}^{\mathrm{3}} \right){dt}\:{with}\:\mid{x}\mid<\mathrm{1}\:. \\ $$ $$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+\mathrm{4}{t}^{\mathrm{3}} \right){dt}\:\:\:{and}\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{2}+{t}^{\mathrm{3}} \right){dt}. \\ $$
Commented bymaxmathsup by imad last updated on 30/Jun/18
![1) we have f(x)= ∫_0 ^1 (Σ_(n=1) ^∞ (((−1)^(n−1) )/n) x^n t^(3n) )dt = Σ_(n=1) ^∞ (((−1)^(n−1) )/n) x^n (1/(3n+1)) = Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(3n+1)))x^n ((f(x))/3) =Σ_(n=1) ^∞ (((−1)^(n−1) )/(3n(3n+1))) x^n = Σ_(n=1) ^∞ (−1)^(n−1) { (1/(3n)) −(1/(3n+1))}x^n =(1/3) Σ_(n=1) ^∞ (((−1)^(n−1) )/n)x^n −Σ_(n=1) ^∞ (((−1)^(n−1) )/(3n+1)) x^n but Σ_(n=1) ^∞ (((−1)^n )/n) x^n =ln(1+x) let w(x)=Σ_(n=1) ^∞ (((−1)^(n−1) )/(3n+1)) x^n if 0<x<1 w(x)= (1/x^(1/3) ) Σ_(n=1) ^∞ (((−1)^(n−1) )/(3n+1)) (^3 (√x))^(3n+1) =^3 ((√x))^(−1) ϕ(^3 (√x)) with ϕ(x)=Σ_(n=1) ^∞ (((−1)^(n−1) )/(3n+1)) x^(3n+1) ⇒ϕ^′ (x)=Σ_(n=1) ^∞ (−1)^(n−1) x^(3n) =Σ_(n=0) ^∞ (−1)^n x^(3n+3) =x^3 (1/(1+x^3 )) =(x^3 /(1+x^3 )) ⇒ϕ(x)=∫_0 ^x (t^3 /(1+t^3 ))dt +c c=ϕ(o)=0 ⇒ ϕ(x)= ∫_0 ^x (t^3 /(1+t^3 ))dt =∫_0 ^x ((1+t^3 −1)/(1+t^3 ))dt = x −∫_0 ^x (dt/(1+t^3 )) let decompose F(t)= (1/(1+t^3 )) F(t)=(1/((t+1)(t^2 −t+1))) = (a/(t+1)) +((bt +c)/(t^2 −t +1)) a=lim_(t→−1) (t+1)ϕ(t)=(1/3) lim_(t→+∞) t ϕ(t) = 0 =a+b ⇒b=−(1/3) ⇒F(t)= (1/(3(t+1))) +((−(1/3)t +c)/(t^2 −t+1)) F(0) =1 = (1/3) +c ⇒c=(2/3) ⇒F(x)= (1/(3(t+1))) +(1/3) ((−t +1)/(t^2 −t +1)) ∫_0 ^x F(t)dt =(1/3) ∫_0 ^x (dt/(t+1)) −(1/6) ∫_0 ^x ((2t−1−1)/(t^2 −t +1))dt = [ (1/3)ln∣t+1∣−(1/6)ln(∣t^2 −t+1∣]_0 ^x +(1/6) ∫_0 ^x (dt/(t^2 −t +1)) =[ (1/6)ln((((t+1)^2 )/(t^2 −t+1)))]_0 ^x +(1/6) ∫_0 ^x (dt/(t^2 −t +1)) =(1/6)ln(((x^2 +2x+1)/(x^2 −x+1))) +I I =(1/6) ∫_0 ^x (dt/(t^2 −2 (t/2) +(1/4) +(3/4))) ⇒6I = ∫_0 ^x (dt/((t−(1/2))^2 +(3/4))) =_(t−(1/2) =((√3)/2)u) (4/3)∫_(−(1/(√3))) ^((2x−1)/(√3)) (1/(1+u^2 )) ((√3)/2) du = (2/(√3)) [arctan (((2x−1)/(√3))) +arctan((1/(√3)))} ⇒ ∫_0 ^x F(t)dt = (1/6)ln(((x^2 +2x+1)/(x^2 −x+1))) +(1/(3(√3))){ arctan(((2x−1)/(√3))) +arctan((1/(√3)))} ϕ(x) =x −(1/6)ln(((x^2 +2x+1)/(x^2 −x+1))) −(1/(3(√3))){ arctan(((2x−1)/(√3)))+(π/6)} w(x)= (1/((^3 (√x)))) ϕ(^3 (√x)) ⇒ ((f(x))/3) =(1/3)ln(1+x) −w(x) ⇒ f(x) =ln(1+x)−3w(x) .](Q38884.png)
$$\left.\mathrm{1}\right)\:{we}\:{have}\:{f}\left({x}\right)=\:\int_{\mathrm{0}} ^{\mathrm{1}} \left(\sum_{{n}=\mathrm{1}} ^{\infty} \frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{{n}}\:{x}^{{n}} \:{t}^{\mathrm{3}{n}} \right){dt} \\ $$ $$=\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{{n}}\:{x}^{{n}} \:\:\frac{\mathrm{1}}{\mathrm{3}{n}+\mathrm{1}}\:=\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{{n}\left(\mathrm{3}{n}+\mathrm{1}\right)}{x}^{{n}} \\ $$ $$\frac{{f}\left({x}\right)}{\mathrm{3}}\:=\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{\mathrm{3}{n}\left(\mathrm{3}{n}+\mathrm{1}\right)}\:{x}^{{n}} \:=\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} \left\{\:\frac{\mathrm{1}}{\mathrm{3}{n}}\:−\frac{\mathrm{1}}{\mathrm{3}{n}+\mathrm{1}}\right\}{x}^{{n}} \\ $$ $$=\frac{\mathrm{1}}{\mathrm{3}}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{{n}}\boldsymbol{{x}}^{\boldsymbol{{n}}} \:−\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{\boldsymbol{{n}}−\mathrm{1}} }{\mathrm{3}\boldsymbol{{n}}+\mathrm{1}}\:\boldsymbol{{x}}^{\boldsymbol{{n}}} \:\:\boldsymbol{{but}} \\ $$ $$\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}}\:{x}^{{n}} \:={ln}\left(\mathrm{1}+{x}\right)\:\:{let}\:{w}\left({x}\right)=\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{\mathrm{3}{n}+\mathrm{1}}\:{x}^{{n}} \\ $$ $${if}\:\mathrm{0}<{x}<\mathrm{1}\:{w}\left({x}\right)=\:\frac{\mathrm{1}}{{x}^{\frac{\mathrm{1}}{\mathrm{3}}} }\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{\mathrm{3}{n}+\mathrm{1}}\:\left(^{\mathrm{3}} \sqrt{{x}}\right)^{\mathrm{3}{n}+\mathrm{1}} =\:^{\mathrm{3}} \left(\sqrt{{x}}\right)^{−\mathrm{1}} \:\varphi\left(\:^{\mathrm{3}} \sqrt{{x}}\right)\:{with} \\ $$ $$\varphi\left({x}\right)=\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{\mathrm{3}{n}+\mathrm{1}}\:{x}^{\mathrm{3}{n}+\mathrm{1}} \:\:\:\Rightarrow\varphi^{'} \left({x}\right)=\sum_{{n}=\mathrm{1}} ^{\infty} \:\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} \:{x}^{\mathrm{3}{n}} \\ $$ $$=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{3}{n}+\mathrm{3}} ={x}^{\mathrm{3}} \:\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{3}} }\:=\frac{{x}^{\mathrm{3}} }{\mathrm{1}+{x}^{\mathrm{3}} }\:\Rightarrow\varphi\left({x}\right)=\int_{\mathrm{0}} ^{{x}} \:\frac{{t}^{\mathrm{3}} }{\mathrm{1}+{t}^{\mathrm{3}} }{dt}\:+{c} \\ $$ $${c}=\varphi\left({o}\right)=\mathrm{0}\:\Rightarrow\:\varphi\left({x}\right)=\:\int_{\mathrm{0}} ^{{x}} \:\:\frac{{t}^{\mathrm{3}} }{\mathrm{1}+{t}^{\mathrm{3}} }{dt}\:=\int_{\mathrm{0}} ^{{x}} \:\frac{\mathrm{1}+{t}^{\mathrm{3}} −\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{3}} }{dt} \\ $$ $$=\:{x}\:−\int_{\mathrm{0}} ^{{x}} \:\:\:\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{3}} }\:\:{let}\:{decompose}\:{F}\left({t}\right)=\:\frac{\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{3}} } \\ $$ $${F}\left({t}\right)=\frac{\mathrm{1}}{\left({t}+\mathrm{1}\right)\left({t}^{\mathrm{2}} −{t}+\mathrm{1}\right)}\:=\:\frac{{a}}{{t}+\mathrm{1}}\:+\frac{{bt}\:+{c}}{{t}^{\mathrm{2}} −{t}\:+\mathrm{1}} \\ $$ $${a}={lim}_{{t}\rightarrow−\mathrm{1}} \left({t}+\mathrm{1}\right)\varphi\left({t}\right)=\frac{\mathrm{1}}{\mathrm{3}} \\ $$ $${lim}_{{t}\rightarrow+\infty} {t}\:\varphi\left({t}\right)\:=\:\mathrm{0}\:={a}+{b}\:\Rightarrow{b}=−\frac{\mathrm{1}}{\mathrm{3}}\:\Rightarrow{F}\left({t}\right)=\:\frac{\mathrm{1}}{\mathrm{3}\left({t}+\mathrm{1}\right)}\:+\frac{−\frac{\mathrm{1}}{\mathrm{3}}{t}\:+{c}}{{t}^{\mathrm{2}} \:−{t}+\mathrm{1}} \\ $$ $${F}\left(\mathrm{0}\right)\:=\mathrm{1}\:=\:\frac{\mathrm{1}}{\mathrm{3}}\:+{c}\:\Rightarrow{c}=\frac{\mathrm{2}}{\mathrm{3}}\:\Rightarrow{F}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{3}\left({t}+\mathrm{1}\right)}\:+\frac{\mathrm{1}}{\mathrm{3}}\:\frac{−{t}\:+\mathrm{1}}{{t}^{\mathrm{2}} \:−{t}\:+\mathrm{1}} \\ $$ $$\int_{\mathrm{0}} ^{{x}} \:{F}\left({t}\right){dt}\:=\frac{\mathrm{1}}{\mathrm{3}}\:\int_{\mathrm{0}} ^{{x}} \:\frac{{dt}}{{t}+\mathrm{1}}\:−\frac{\mathrm{1}}{\mathrm{6}}\:\int_{\mathrm{0}} ^{{x}} \:\:\frac{\mathrm{2}{t}−\mathrm{1}−\mathrm{1}}{{t}^{\mathrm{2}} −{t}\:+\mathrm{1}}{dt} \\ $$ $$=\:\left[\:\frac{\mathrm{1}}{\mathrm{3}}{ln}\mid{t}+\mathrm{1}\mid−\frac{\mathrm{1}}{\mathrm{6}}{ln}\left(\mid{t}^{\mathrm{2}} −{t}+\mathrm{1}\mid\right]_{\mathrm{0}} ^{{x}} \:\:\:+\frac{\mathrm{1}}{\mathrm{6}}\:\int_{\mathrm{0}} ^{{x}} \:\:\:\frac{{dt}}{{t}^{\mathrm{2}} −{t}\:+\mathrm{1}}\right. \\ $$ $$=\left[\:\frac{\mathrm{1}}{\mathrm{6}}{ln}\left(\frac{\left({t}+\mathrm{1}\right)^{\mathrm{2}} }{{t}^{\mathrm{2}} −{t}+\mathrm{1}}\right)\right]_{\mathrm{0}} ^{{x}} \:+\frac{\mathrm{1}}{\mathrm{6}}\:\int_{\mathrm{0}} ^{{x}} \:\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:−{t}\:+\mathrm{1}}\:=\frac{\mathrm{1}}{\mathrm{6}}{ln}\left(\frac{{x}^{\mathrm{2}} \:+\mathrm{2}{x}+\mathrm{1}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}}\right)\:+{I} \\ $$ $${I}\:\:=\frac{\mathrm{1}}{\mathrm{6}}\:\int_{\mathrm{0}} ^{{x}} \:\:\:\frac{{dt}}{{t}^{\mathrm{2}} −\mathrm{2}\:\frac{{t}}{\mathrm{2}}\:+\frac{\mathrm{1}}{\mathrm{4}}\:+\frac{\mathrm{3}}{\mathrm{4}}}\:\Rightarrow\mathrm{6}{I}\:=\:\int_{\mathrm{0}} ^{{x}} \:\:\frac{{dt}}{\left({t}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}} \\ $$ $$=_{{t}−\frac{\mathrm{1}}{\mathrm{2}}\:=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{u}} \:\:\:\:\:\:\:\frac{\mathrm{4}}{\mathrm{3}}\int_{−\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\frac{\mathrm{2}{x}−\mathrm{1}}{\sqrt{\mathrm{3}}}} \:\:\:\:\:\:\:\frac{\mathrm{1}}{\mathrm{1}+{u}^{\mathrm{2}} }\:\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:{du} \\ $$ $$=\:\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\:\left[{arctan}\:\left(\frac{\mathrm{2}{x}−\mathrm{1}}{\sqrt{\mathrm{3}}}\right)\:+{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\right)\right\}\:\Rightarrow \\ $$ $$\int_{\mathrm{0}} ^{{x}} \:{F}\left({t}\right){dt}\:=\:\frac{\mathrm{1}}{\mathrm{6}}{ln}\left(\frac{{x}^{\mathrm{2}} \:+\mathrm{2}{x}+\mathrm{1}}{{x}^{\mathrm{2}} \:−{x}+\mathrm{1}}\right)\:+\frac{\mathrm{1}}{\mathrm{3}\sqrt{\mathrm{3}}}\left\{\:{arctan}\left(\frac{\mathrm{2}{x}−\mathrm{1}}{\sqrt{\mathrm{3}}}\right)\:+{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\right)\right\} \\ $$ $$\varphi\left({x}\right)\:={x}\:−\frac{\mathrm{1}}{\mathrm{6}}{ln}\left(\frac{{x}^{\mathrm{2}} \:+\mathrm{2}{x}+\mathrm{1}}{{x}^{\mathrm{2}} \:−{x}+\mathrm{1}}\right)\:−\frac{\mathrm{1}}{\mathrm{3}\sqrt{\mathrm{3}}}\left\{\:{arctan}\left(\frac{\mathrm{2}{x}−\mathrm{1}}{\sqrt{\mathrm{3}}}\right)+\frac{\pi}{\mathrm{6}}\right\} \\ $$ $${w}\left({x}\right)=\:\frac{\mathrm{1}}{\left(^{\mathrm{3}} \sqrt{{x}}\right)}\:\varphi\left(^{\mathrm{3}} \sqrt{{x}}\right)\:\Rightarrow\:\frac{{f}\left({x}\right)}{\mathrm{3}}\:=\frac{\mathrm{1}}{\mathrm{3}}{ln}\left(\mathrm{1}+{x}\right)\:−{w}\left({x}\right)\:\Rightarrow \\ $$ $${f}\left({x}\right)\:={ln}\left(\mathrm{1}+{x}\right)−\mathrm{3}{w}\left({x}\right)\:. \\ $$ $$ \\ $$
Commented bymaxmathsup by imad last updated on 30/Jun/18
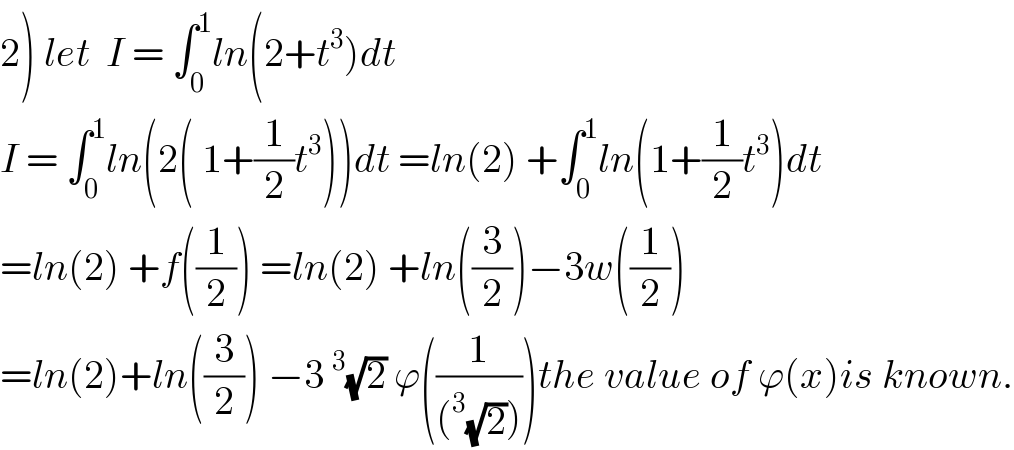
$$\left.\mathrm{2}\right)\:{let}\:\:{I}\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{2}+{t}^{\mathrm{3}} \right){dt}\: \\ $$ $${I}\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{2}\left(\:\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}{t}^{\mathrm{3}} \right)\right){dt}\:={ln}\left(\mathrm{2}\right)\:+\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}{t}^{\mathrm{3}} \right){dt} \\ $$ $$={ln}\left(\mathrm{2}\right)\:+{f}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\:={ln}\left(\mathrm{2}\right)\:+{ln}\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\mathrm{3}{w}\left(\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$ $$={ln}\left(\mathrm{2}\right)+{ln}\left(\frac{\mathrm{3}}{\mathrm{2}}\right)\:−\mathrm{3}\:^{\mathrm{3}} \sqrt{\mathrm{2}}\:\varphi\left(\frac{\mathrm{1}}{\left(^{\mathrm{3}} \sqrt{\mathrm{2}}\right)}\right){the}\:{value}\:{of}\:\varphi\left({x}\right){is}\:{known}. \\ $$
