Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 42579 by Raj Singh last updated on 28/Aug/18

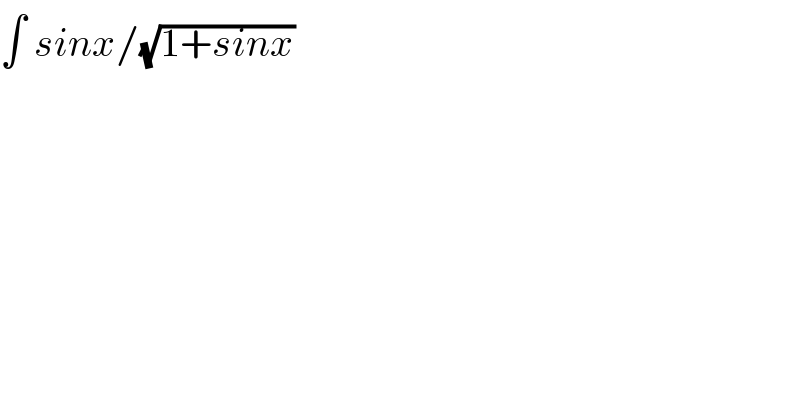
∫ sinx/(√(1+sinx))

$$\int\:{sinx}/\sqrt{\mathrm{1}+{sinx}} \\ $$

Commented by maxmathsup by imad last updated on 28/Aug/18

let A = ∫ ((sinx)/(√(1+sinx))) dx we have 1+sinx =1+2sin((x/2))cos((x/2)) =(sin((x/2)) +cos((x/2)))^2 ⇒(√(1+sinx)) =sin((x/2))+cos((x/2)) A = ∫ ((1+sinx −1)/(√(1+sinx)))dx = ∫ (√(1+sinx))dx −∫ (dx/(√(1+sinx))) =∫ (sin((x/2)) +cos((x/2)))dx −∫ (dx/(sin((x/2))+cos((x/2)))) =−2cos((x/2)) +2sin((x/2)) − ∫ (dx/((√2)cos((x/2)−(π/4)))) changement (x/2)−(π/4) =t give ∫ (dx/((√2)cos((x/2)−(π/4)))) = ∫ ((2dt)/((√2)cos(t))) =(√2) ∫ (dt/(cos(t))) =_(tan((t/2)) =u) (√2) ∫ (1/((1−u^2 )/(1+u^2 ))) ((2du)/(1+u^2 )) = 2(√2) ∫ (du/(1−u^2 )) =(√2)∫ ((1/(1+u)) +(1/(1−u)))du =(√2)ln∣((1+u)/(1−u))∣ =(√2)ln∣((1+tan((t/2)))/(1−tan((t/2))))∣ =(√2)ln∣tan((t/2) +(π/4))∣ =(√2)ln∣tan((x/4) −(π/8) +(π/4))∣=(√2)ln∣tan((x/4)+(π/8))∣ ⇒ A = 2sin((x/2))−2cos((x/2))−(√2)ln∣tan((x/4)+(π/8))∣ +c .

$${let}\:{A}\:=\:\int\:\:\:\frac{{sinx}}{\sqrt{\mathrm{1}+{sinx}}}\:{dx}\:\:{we}\:{have}\:\:\mathrm{1}+{sinx}\:=\mathrm{1}+\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right){cos}\left(\frac{{x}}{\mathrm{2}}\right) \\ $$$$=\left({sin}\left(\frac{{x}}{\mathrm{2}}\right)\:+{cos}\left(\frac{{x}}{\mathrm{2}}\right)\right)^{\mathrm{2}} \:\Rightarrow\sqrt{\mathrm{1}+{sinx}}\:={sin}\left(\frac{{x}}{\mathrm{2}}\right)+{cos}\left(\frac{{x}}{\mathrm{2}}\right) \\ $$$$\:{A}\:=\:\int\:\frac{\mathrm{1}+{sinx}\:−\mathrm{1}}{\sqrt{\mathrm{1}+{sinx}}}{dx}\:=\:\int\:\sqrt{\mathrm{1}+{sinx}}{dx}\:−\int\:\:\:\frac{{dx}}{\sqrt{\mathrm{1}+{sinx}}} \\ $$$$=\int\:\:\left({sin}\left(\frac{{x}}{\mathrm{2}}\right)\:+{cos}\left(\frac{{x}}{\mathrm{2}}\right)\right){dx}\:−\int\:\:\:\:\frac{{dx}}{{sin}\left(\frac{{x}}{\mathrm{2}}\right)+{cos}\left(\frac{{x}}{\mathrm{2}}\right)} \\ $$$$=−\mathrm{2}{cos}\left(\frac{{x}}{\mathrm{2}}\right)\:+\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right)\:−\:\int\:\:\:\:\:\frac{{dx}}{\sqrt{\mathrm{2}}{cos}\left(\frac{{x}}{\mathrm{2}}−\frac{\pi}{\mathrm{4}}\right)}\:{changement}\:\frac{{x}}{\mathrm{2}}−\frac{\pi}{\mathrm{4}}\:={t}\:{give} \\ $$$$\int\:\:\:\:\frac{{dx}}{\sqrt{\mathrm{2}}{cos}\left(\frac{{x}}{\mathrm{2}}−\frac{\pi}{\mathrm{4}}\right)}\:=\:\int\:\:\:\:\:\frac{\mathrm{2}{dt}}{\sqrt{\mathrm{2}}{cos}\left({t}\right)}\:=\sqrt{\mathrm{2}}\:\int\:\:\:\:\frac{{dt}}{{cos}\left({t}\right)} \\ $$$$=_{{tan}\left(\frac{{t}}{\mathrm{2}}\right)\:={u}} \:\:\:\:\sqrt{\mathrm{2}}\:\int\:\:\:\:\:\:\frac{\mathrm{1}}{\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }}\:\:\frac{\mathrm{2}{du}}{\mathrm{1}+{u}^{\mathrm{2}} }\:=\:\mathrm{2}\sqrt{\mathrm{2}}\:\:\int\:\:\:\frac{{du}}{\mathrm{1}−{u}^{\mathrm{2}} } \\ $$$$=\sqrt{\mathrm{2}}\int\:\:\left(\frac{\mathrm{1}}{\mathrm{1}+{u}}\:+\frac{\mathrm{1}}{\mathrm{1}−{u}}\right){du}\:\:=\sqrt{\mathrm{2}}{ln}\mid\frac{\mathrm{1}+{u}}{\mathrm{1}−{u}}\mid\:=\sqrt{\mathrm{2}}{ln}\mid\frac{\mathrm{1}+{tan}\left(\frac{{t}}{\mathrm{2}}\right)}{\mathrm{1}−{tan}\left(\frac{{t}}{\mathrm{2}}\right)}\mid \\ $$$$=\sqrt{\mathrm{2}}{ln}\mid{tan}\left(\frac{{t}}{\mathrm{2}}\:+\frac{\pi}{\mathrm{4}}\right)\mid\:=\sqrt{\mathrm{2}}{ln}\mid{tan}\left(\frac{{x}}{\mathrm{4}}\:−\frac{\pi}{\mathrm{8}}\:+\frac{\pi}{\mathrm{4}}\right)\mid=\sqrt{\mathrm{2}}{ln}\mid{tan}\left(\frac{{x}}{\mathrm{4}}+\frac{\pi}{\mathrm{8}}\right)\mid\:\Rightarrow \\ $$$${A}\:=\:\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right)−\mathrm{2}{cos}\left(\frac{{x}}{\mathrm{2}}\right)−\sqrt{\mathrm{2}}{ln}\mid{tan}\left(\frac{{x}}{\mathrm{4}}+\frac{\pi}{\mathrm{8}}\right)\mid\:+{c}\:. \\ $$$$ \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 28/Aug/18

∫((sinx)/(√(1+sinx)))dx ∫((1+sinx−1)/(√(1+sinx)))dx ∫(√(1+sinx)) dx−∫(dx/(√(1+sinx)))dx ∫(sin(x/2)+cos(x/2))−∫(dx/(sin(x/2)+cos(x/2))) ∫sin(x/2)+cos(x/2)dx−(1/(√2))∫(dx/(((1/(√2))sin(x/2)+(1/(√2))cos(x/2)))) ∫(sin(x/2)+cos(x/2))dx−(1/(√2))∫cosec((Π/4)+(x/2))dx =−((cos(x/2))/(1/2))+((sin(x/2))/(1/2))−(1/(√2))lntan((((Π/4)+(x/2))/2))+c =2(−cos(x/2)+sin(x/2))−(1/(√2))lntan((Π/8)+(x/4))+c

$$\int\frac{{sinx}}{\sqrt{\mathrm{1}+{sinx}}}{dx} \\ $$$$\int\frac{\mathrm{1}+{sinx}−\mathrm{1}}{\sqrt{\mathrm{1}+{sinx}}}{dx} \\ $$$$\int\sqrt{\mathrm{1}+{sinx}}\:{dx}−\int\frac{{dx}}{\sqrt{\mathrm{1}+{sinx}}}{dx} \\ $$$$\int\left({sin}\frac{{x}}{\mathrm{2}}+{cos}\frac{{x}}{\mathrm{2}}\right)−\int\frac{{dx}}{{sin}\frac{{x}}{\mathrm{2}}+{cos}\frac{{x}}{\mathrm{2}}} \\ $$$$\int{sin}\frac{{x}}{\mathrm{2}}+{cos}\frac{{x}}{\mathrm{2}}{dx}−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int\frac{{dx}}{\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{sin}\frac{{x}}{\mathrm{2}}+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{cos}\frac{{x}}{\mathrm{2}}\right)} \\ $$$$\int\left({sin}\frac{{x}}{\mathrm{2}}+{cos}\frac{{x}}{\mathrm{2}}\right){dx}−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int{cosec}\left(\frac{\Pi}{\mathrm{4}}+\frac{{x}}{\mathrm{2}}\right){dx} \\ $$$$=−\frac{{cos}\frac{{x}}{\mathrm{2}}}{\frac{\mathrm{1}}{\mathrm{2}}}+\frac{{sin}\frac{{x}}{\mathrm{2}}}{\frac{\mathrm{1}}{\mathrm{2}}}−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{lntan}\left(\frac{\frac{\Pi}{\mathrm{4}}+\frac{{x}}{\mathrm{2}}}{\mathrm{2}}\right)+{c} \\ $$$$=\mathrm{2}\left(−{cos}\frac{{x}}{\mathrm{2}}+{sin}\frac{{x}}{\mathrm{2}}\right)−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{lntan}\left(\frac{\Pi}{\mathrm{8}}+\frac{{x}}{\mathrm{4}}\right)+{c} \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 28/Aug/18

your answer is correct sir Tanmay.

$${your}\:{answer}\:{is}\:{correct}\:{sir}\:{Tanmay}. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 28/Aug/18

thank you sir...

$${thank}\:{you}\:{sir}... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com