Question and Answers Forum

All Questions      Topic List

Mensuration Questions

Previous in All Question      Next in All Question      

Previous in Mensuration      Next in Mensuration      

Question Number 48338 by ajfour last updated on 22/Nov/18

Commented by mr W last updated on 23/Nov/18

$$niceworking!$$

Commented by ajfour last updated on 22/Nov/18

$$Forexamplep=2,q=4,r=3.$$$$Findx=?.$$

Commented by ajfour last updated on 22/Nov/18

$$WillthisdoSir?$$

Commented by ajfour last updated on 22/Nov/18

Commented by ajfour last updated on 22/Nov/18

$$R^2{\cos }^{-1}\frac{h}{R}-h\sqrt{R^2-h^2}=p+q$$$$R^2{\cos }^{-1}\frac{h}{R}-k\sqrt{R^2-k^2}=r+p$$$$x=\pi R^2-p-q-r$$$$And\frac{\pi R^2}{4}=\frac{q+r}{2}+hk$$$$\Rightarrow \frac{\pi R^2}{4}=\frac{q+r}{2}+h^{\prime }{k}^{\prime }{R}^2$$$$R^2=\frac{(q+r)/2}{\frac{\pi }{4}-h^{\prime }{k}^{\prime }}$$$$\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}\mathrm{\_}$$$$let\frac{h}{R}=h^{\prime }\mathrm{\&}\frac{p}{R^2}=p^{\prime }andsoon$$$${\cos }^{-1}{h}^{\prime }-h^{\prime }\sqrt{1-h^{\prime 2}}=\left(\frac{p+q}{q+r}\right)(\frac{\pi }{2}-2h^{\prime }{k}^{\prime })$$$${\cos }^{-1}{k}^{\prime }-k^{\prime }\sqrt{1-k^{\prime 2}}=\left(\frac{r+p}{q+r}\right)(\frac{\pi }{2}-2h^{\prime }{k}^{\prime })$$$$\mathit{x}=\frac{\mathit{\pi }(\mathit{q}+\mathit{r})}{(\frac{\mathit{\pi }}{2}-2{\mathit{h}}^{\prime }{\mathit{k}}^{\prime })}-(\mathit{p}+\mathit{q}+\mathit{r}).$$

Commented by MJS last updated on 22/Nov/18

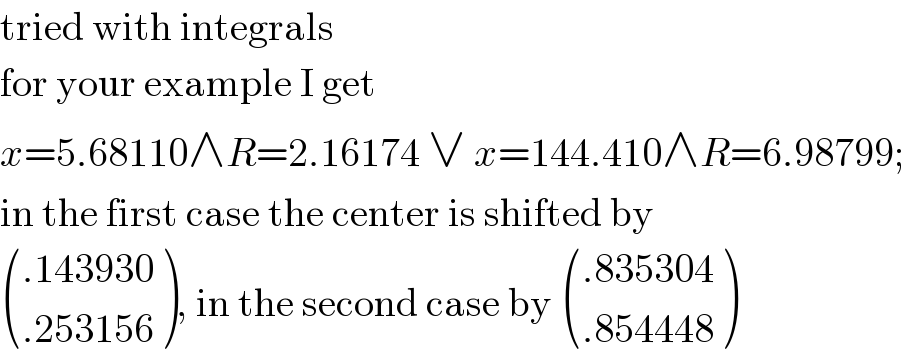
$$\mathrm{tried}\mathrm{with}\mathrm{integrals}$$$$\mathrm{for}\mathrm{your}\mathrm{example}\mathrm{I}\mathrm{get}$$$$x=5.68110\wedge R=2.16174\vee x=144.410\wedge R=6.98799;$$$$\mathrm{in}\mathrm{the}\mathrm{first}\mathrm{case}\mathrm{the}\mathrm{center}\mathrm{is}\mathrm{shifted}\mathrm{by}$$$$\left(\begin{array}{c}.143930\\ .253156\end{array}\right),\mathrm{in}\mathrm{the}\mathrm{second}\mathrm{case}\mathrm{by}\left(\begin{array}{c}.835304\\ .854448\end{array}\right)$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com