
Question Number 53212 by Joel578 last updated on 19/Jan/19
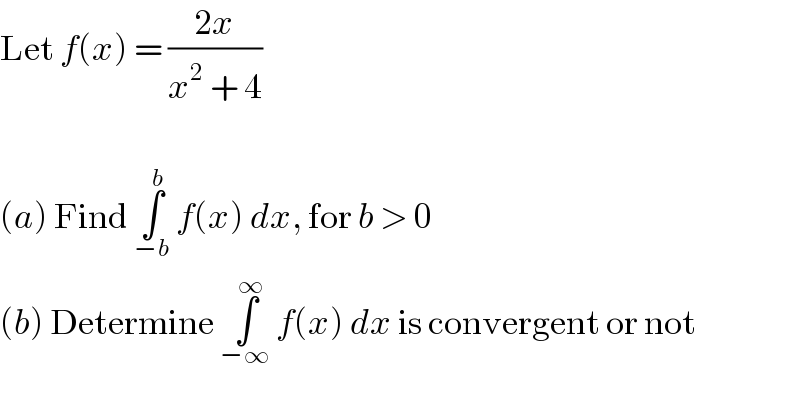
$$\mathrm{Let}\:{f}\left({x}\right)\:=\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} \:+\:\mathrm{4}} \\ $$ $$ \\ $$ $$\left({a}\right)\:\mathrm{Find}\:\underset{−{b}} {\overset{{b}} {\int}}\:{f}\left({x}\right)\:{dx},\:\mathrm{for}\:{b}\:>\:\mathrm{0} \\ $$ $$\left({b}\right)\:\mathrm{Determine}\:\underset{−\infty} {\overset{\infty} {\int}}\:{f}\left({x}\right)\:{dx}\:\mathrm{is}\:\mathrm{convergent}\:\mathrm{or}\:\mathrm{not} \\ $$
Commented byJoel578 last updated on 19/Jan/19
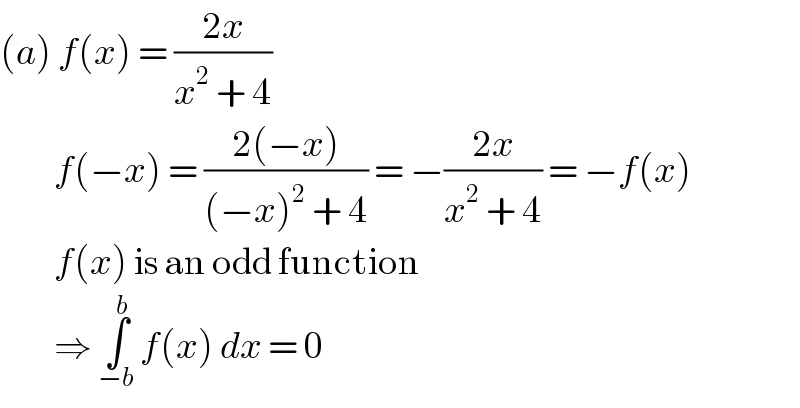
$$\left({a}\right)\:{f}\left({x}\right)\:=\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} \:+\:\mathrm{4}} \\ $$ $$\:\:\:\:\:\:\:\:\:{f}\left(−{x}\right)\:=\:\frac{\mathrm{2}\left(−{x}\right)}{\left(−{x}\right)^{\mathrm{2}} \:+\:\mathrm{4}}\:=\:−\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} \:+\:\mathrm{4}}\:=\:−{f}\left({x}\right) \\ $$ $$\:\:\:\:\:\:\:\:\:{f}\left({x}\right)\:\mathrm{is}\:\mathrm{an}\:\mathrm{odd}\:\mathrm{function} \\ $$ $$\:\:\:\:\:\:\:\:\:\Rightarrow\:\underset{−{b}} {\overset{{b}} {\int}}\:{f}\left({x}\right)\:{dx}\:=\:\mathrm{0} \\ $$
Commented byJoel578 last updated on 19/Jan/19
$$\mathrm{Please}\:\mathrm{help}\:\mathrm{with}\:\mathrm{part}\:\left({b}\right) \\ $$
Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19

$$\mathrm{1}.\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{4}}\:{is}\:{odd}\:{function}\:\:{because}\:{f}\left(−{x}\right)=−{f}\left({x}\right) \\ $$ $$\mathrm{2}.{the}\:{intregal}\:\int_{−{b}} ^{{b}} \frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{4}}{dx}=\int_{−{b}} ^{\mathrm{0}} \frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{4}}{dx}+\int_{\mathrm{0}} ^{{b}} \frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{4}}{dx} \\ $$ $${I}={I}_{\mathrm{1}} +{I}_{\mathrm{2}} \\ $$ $${though}\:{I}_{\mathrm{1}} ={I}_{\mathrm{2}} \:{but}\:{I}_{\mathrm{1}} {represent}\:{area}\:{bound}\:{by} \\ $$ $${curve}\:\frac{\mathrm{2}{x}}{\mathrm{4}+{x}^{\mathrm{2}} }\:\:{from}\:\:{x}=−{b}\:\:{to}\:{x}=\mathrm{0}\:\:{but}\:{that}\:{area} \\ $$ $${is}\:{below}\:{x}\:{axis}\:{so}\:\:{negetive}\:{area}\:. \\ $$ $${I}_{\mathrm{2}} \:{represent}\:{the}\:{area}\:{bound}\:{by}\:{the}\:{curve}\:\frac{\mathrm{2}{x}}{\mathrm{4}+{x}^{\mathrm{2}} } \\ $$ $${from}\:{x}=\mathrm{0}\:\:{to}\:{x}={b}\:\:{above}\:{x}\:{axis}\:{so}\:+{ve}\:{area} \\ $$ $${hence}\:{I}_{\mathrm{1}} +{I}_{\mathrm{2}} =\mathrm{0} \\ $$ $${this}\:{is}\:{my}\:{opinion}\:{let}\:{others}\:{comment}... \\ $$ $$ \\ $$ $$ \\ $$
Commented byafachri last updated on 19/Jan/19
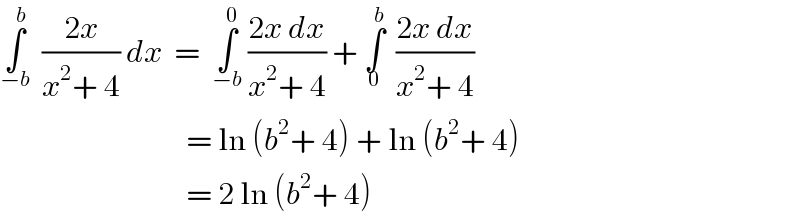
$$\underset{−{b}} {\overset{{b}} {\int}}\:\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\:\mathrm{4}}\:{dx}\:\:=\:\:\underset{−{b}} {\overset{\mathrm{0}} {\int}}\:\frac{\mathrm{2}{x}\:{dx}}{{x}^{\mathrm{2}} +\:\mathrm{4}}\:+\:\underset{\mathrm{0}} {\overset{{b}} {\int}}\:\:\frac{\mathrm{2}{x}\:{dx}}{{x}^{\mathrm{2}} +\:\mathrm{4}} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{ln}\:\left({b}^{\mathrm{2}} +\:\mathrm{4}\right)\:+\:\mathrm{ln}\:\left({b}^{\mathrm{2}} +\:\mathrm{4}\right) \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{2}\:\mathrm{ln}\:\left({b}^{\mathrm{2}} +\:\mathrm{4}\right) \\ $$
Commented byafachri last updated on 19/Jan/19
Commented byafachri last updated on 19/Jan/19
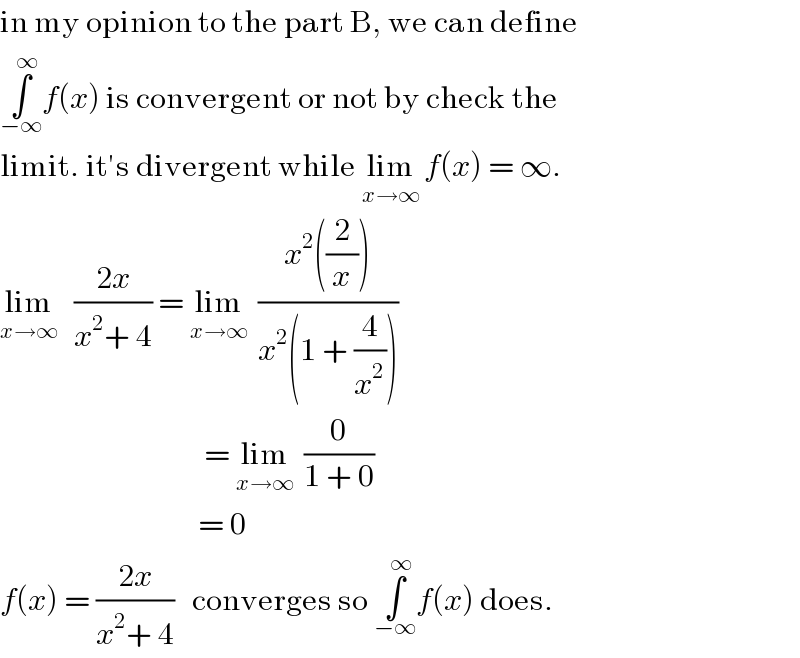
$$\mathrm{in}\:\mathrm{my}\:\mathrm{opinion}\:\mathrm{to}\:\mathrm{the}\:\mathrm{part}\:\mathrm{B},\:\mathrm{we}\:\mathrm{can}\:\mathrm{define} \\ $$ $$\underset{−\infty} {\overset{\infty} {\int}}{f}\left({x}\right)\:\mathrm{is}\:\mathrm{convergent}\:\mathrm{or}\:\mathrm{not}\:\mathrm{by}\:\mathrm{check}\:\mathrm{the} \\ $$ $$\mathrm{limit}.\:\mathrm{it}'\mathrm{s}\:\mathrm{divergent}\:\mathrm{while}\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:{f}\left({x}\right)\:=\:\infty. \\ $$ $$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\:\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\:\mathrm{4}}\:=\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\:\frac{{x}^{\mathrm{2}} \left(\frac{\mathrm{2}}{{x}}\right)}{{x}^{\mathrm{2}} \left(\mathrm{1}\:+\:\frac{\mathrm{4}}{{x}^{\mathrm{2}} }\right)} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\:\frac{\mathrm{0}}{\mathrm{1}\:+\:\mathrm{0}} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{0} \\ $$ $${f}\left({x}\right)\:=\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\:\mathrm{4}}\:\:\:\mathrm{converges}\:\mathrm{so}\:\underset{−\infty} {\overset{\infty} {\int}}{f}\left({x}\right)\:\mathrm{does}. \\ $$
Commented byafachri last updated on 19/Jan/19
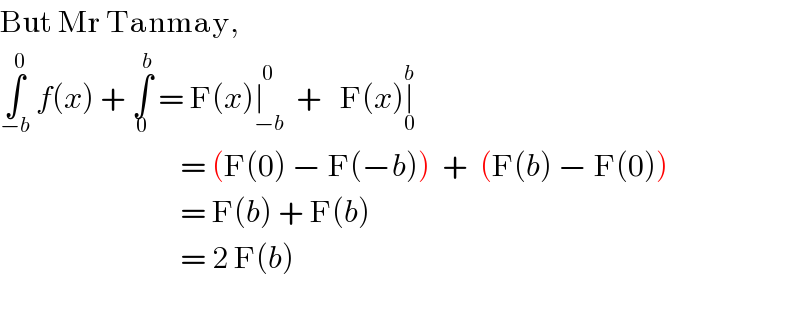
$$\mathrm{But}\:\mathrm{Mr}\:\mathrm{Tanmay},\: \\ $$ $$\underset{−{b}} {\overset{\mathrm{0}} {\int}}\:{f}\left({x}\right)\:+\:\underset{\mathrm{0}} {\overset{{b}} {\int}}\:=\:\mathrm{F}\left({x}\right)\underset{−{b}} {\overset{\mathrm{0}} {\mid}}\:\:+\:\:\:\mathrm{F}\left({x}\right)\underset{\mathrm{0}} {\overset{{b}} {\mid}} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\left(\mathrm{F}\left(\mathrm{0}\right)\:−\:\mathrm{F}\left(−{b}\right)\right)\:\:+\:\:\left(\mathrm{F}\left({b}\right)\:−\:\mathrm{F}\left(\mathrm{0}\right)\right) \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{F}\left({b}\right)\:+\:\mathrm{F}\left({b}\right) \\ $$ $$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{2}\:\mathrm{F}\left({b}\right) \\ $$ $$ \\ $$
Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19
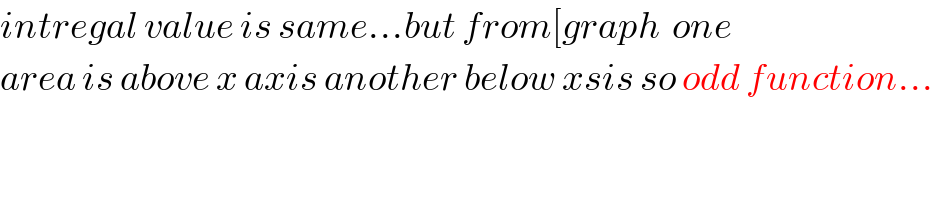
$${intregal}\:{value}\:{is}\:{same}...{but}\:{from}\left[{graph}\:\:{one}\right. \\ $$ $${area}\:{is}\:{above}\:{x}\:{axis}\:{another}\:{below}\:{xsis}\:{so}\:{odd}\:{function}... \\ $$
Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19
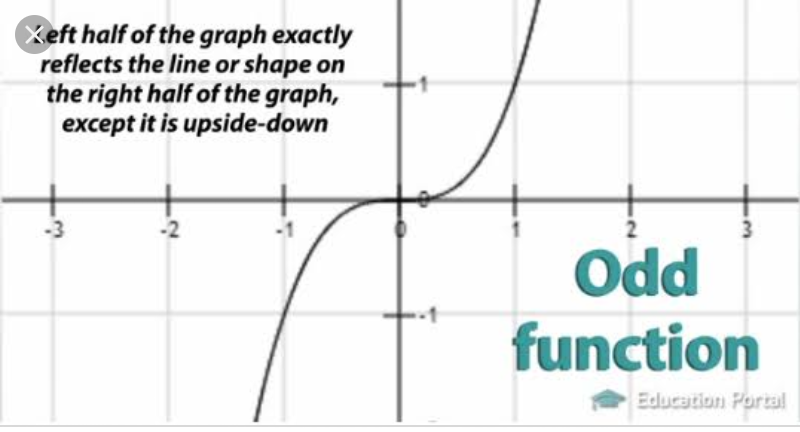
Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented byafachri last updated on 19/Jan/19

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{Mr}\:\mathrm{Damian}\:\mathrm{and}\:\mathrm{all}\:\mathrm{of}\:\mathrm{you}\:\mathrm{Sir} \\ $$ $$\mathrm{who}\:\mathrm{have}\:\mathrm{corrected}\:\mathrm{me}\:\mathrm{for}\:\mathrm{my}\:\mathrm{mistake}.\: \\ $$ $$\mathrm{what}\:\mathrm{an}\:\mathrm{interesting}\:\mathrm{forum}\:\mathrm{here}.\:\mathrm{now}\:\mathrm{i}'\mathrm{m} \\ $$ $$\mathrm{understand}\:\mathrm{it}. \\ $$
Commented byafachri last updated on 19/Jan/19
$$\mathrm{Yes}\:\mathrm{Mr}\:\mathrm{Tanmay}.\:\mathrm{but}\:\mathrm{what}\:\mathrm{i}'\mathrm{m}\:\mathrm{questioning} \\ $$ $$\mathrm{is}\:\mathrm{why}\:\underset{−{b}} {\overset{{b}} {\int}}\:\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\:\mathrm{4}}\:\:=\:\mathrm{0}\:?\:\mathrm{Well},\:\underset{−{b}} {\overset{{b}} {\int}}\:\:\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\:\mathrm{4}}\:\mathrm{states} \\ $$ $$\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{function}\:\mathrm{through}\:\mathrm{absis}.\: \\ $$ $$\mathrm{i}\:\mathrm{hope}\:\mathrm{you}\:\mathrm{don}'\mathrm{t}\:\mathrm{mind}\:\mathrm{for}\:\mathrm{my}\:\mathrm{situation}\: \\ $$ $$\mathrm{Mr}\:\mathrm{Tanmay}. \\ $$
Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19

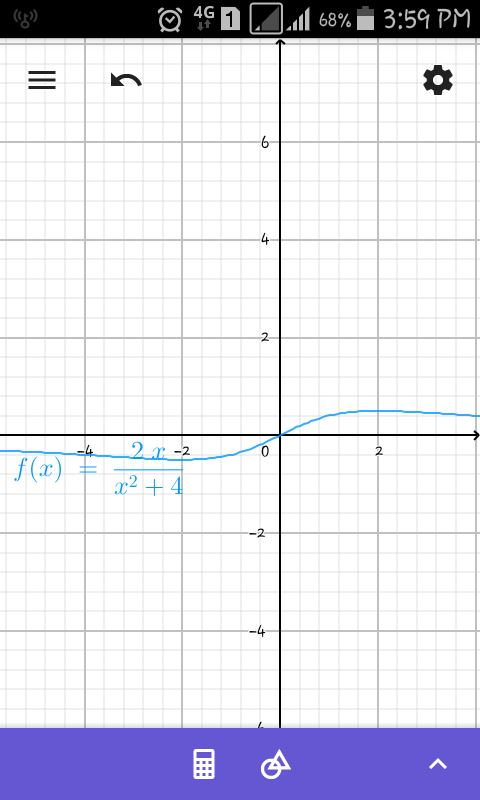
$${wait}...{let}\:{assume}\:{b}=\mathrm{1000} \\ $$ $${then}\:{find}\:\int_{−\mathrm{1000}} ^{\mathrm{1000}} \frac{\mathrm{2}{x}}{\mathrm{4}+{x}^{\mathrm{2}} }{dx}\:{using}\:{graph}\:{app}.. \\ $$
Commented bytanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented byafachri last updated on 19/Jan/19

$$\mathrm{Yes}\:\mathrm{Mr}\:\mathrm{Tanmay}.\:\mathrm{Done}.\:\mathrm{Thank}\:\mathrm{You} \\ $$ $$\mathrm{so}\:\mathrm{much}.\:\mathrm{Really}\:\mathrm{appreciate}\:\mathrm{your}\:\mathrm{time} \\ $$ $$\mathrm{Mr}\:\mathrm{Tanmay} \\ $$
Commented byafachri last updated on 19/Jan/19

Commented byJDamian last updated on 19/Jan/19
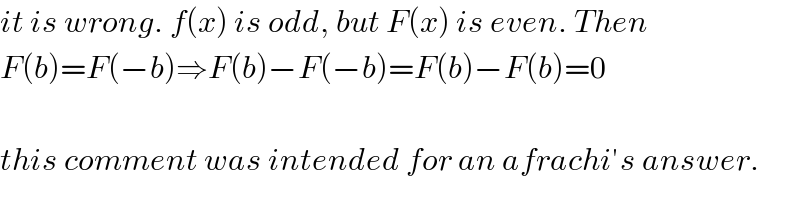
$${it}\:{is}\:{wrong}.\:{f}\left({x}\right)\:{is}\:{odd},\:{but}\:{F}\left({x}\right)\:{is}\:{even}.\:{Then} \\ $$ $${F}\left({b}\right)={F}\left(−{b}\right)\Rightarrow{F}\left({b}\right)−{F}\left(−{b}\right)={F}\left({b}\right)−{F}\left({b}\right)=\mathrm{0} \\ $$ $$ \\ $$ $${this}\:{comment}\:{was}\:{intended}\:{for}\:{an}\:{afrachi}'{s}\:{answer}. \\ $$
Commented byJoel578 last updated on 20/Jan/19
$$\mathrm{Thanks}\:\mathrm{you}\:\mathrm{Sir}\:\mathrm{afachri},\:\mathrm{tanmay},\:\mathrm{JDamian}\:\mathrm{for}\:\mathrm{the}\:\mathrm{answers}. \\ $$ $$\mathrm{Actually}\:\mathrm{I}'\mathrm{m}\:\mathrm{agree}\:\mathrm{with}\:\mathrm{Sir}\:\mathrm{tanmay}.\:\mathrm{That}'\mathrm{s}\: \\ $$ $$\mathrm{what}\:\mathrm{I}\:\mathrm{have}\:\mathrm{been}\:\mathrm{taught}\:\mathrm{in}\:\mathrm{Calculus}\:\mathrm{1}. \\ $$ $$\mathrm{There}\:\mathrm{is}\:\mathrm{a}\:\mathrm{difference}\:\mathrm{between}\:\mathrm{a}\:\mathrm{definite}\:\mathrm{integral} \\ $$ $$\mathrm{and}\:\mathrm{a}\:\mathrm{definite}\:\mathrm{integral}\:\mathrm{as}\:\mathrm{an}\:\mathrm{area} \\ $$
Commented bymaxmathsup by imad last updated on 23/Jan/19
![for all odd function integrable on ]−a,a[ a∈R^− we have ∫_(−a) ^a f(x)dx =0.](Q53609.png)
$$\left.{for}\:{all}\:\:{odd}\:{function}\:\:{integrable}\:{on}\:\right]−{a},{a}\left[\:{a}\in\overset{−} {{R}}\:\:\:{we}\:{have}\:\right. \\ $$ $$\int_{−{a}} ^{{a}} {f}\left({x}\right){dx}\:=\mathrm{0}. \\ $$
Answered by MJS last updated on 20/Jan/19
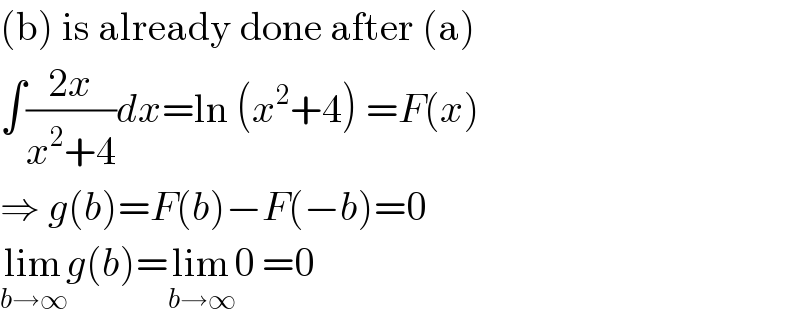
$$\left(\mathrm{b}\right)\:\mathrm{is}\:\mathrm{already}\:\mathrm{done}\:\mathrm{after}\:\left(\mathrm{a}\right) \\ $$ $$\int\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{4}}{dx}=\mathrm{ln}\:\left({x}^{\mathrm{2}} +\mathrm{4}\right)\:={F}\left({x}\right) \\ $$ $$\Rightarrow\:{g}\left({b}\right)={F}\left({b}\right)−{F}\left(−{b}\right)=\mathrm{0} \\ $$ $$\underset{{b}\rightarrow\infty} {\mathrm{lim}}{g}\left({b}\right)=\underset{{b}\rightarrow\infty} {\mathrm{lim}0}\:=\mathrm{0} \\ $$
Commented byJoel578 last updated on 20/Jan/19
$${thank}\:{you}\:{very}\:{much} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19
![b) it is my opinion... ∫_(−∞) ^(+∞) f(x)dx lim_(h→−∞) ∫_h ^0 f(x) dx=−ve A lim_(k→+∞) ∫_0 ^k f(x)dx=+ve A so ∫_(−∞) ^(+∞) ((2x)/(4+x^2 ))dx=0 anoother way... x=2tanθ dx=2sec^2 θdθ ∫_(−(π/2)) ^(π/2) ((2×2tanθ×2sec^2 θdθ)/(4+4tan^2 θ)) =∫_(−(π/2)) ^(π/2) ((8tanθsec^2 θ)/(4sec^2 θ))dθ =2∣lnsecθ∣_(−(π/2)) ^(π/2) =−2∣lncosθ∣_(−(π/2)) ^(π/2) =−2[lncos((π/2))−lncos(−(π/2))] =0 it is my opinion...let others comment...](Q53231.png)
$$\left.{b}\right)\:{it}\:{is}\:{my}\:{opinion}... \\ $$ $$\int_{−\infty} ^{+\infty} {f}\left({x}\right){dx} \\ $$ $$\underset{{h}\rightarrow−\infty} {\mathrm{lim}}\int_{{h}} ^{\mathrm{0}} \:{f}\left({x}\right)\:{dx}=−{ve}\:{A} \\ $$ $$\underset{{k}\rightarrow+\infty} {\mathrm{lim}}\:\int_{\mathrm{0}} ^{{k}} {f}\left({x}\right){dx}=+{ve}\:{A} \\ $$ $${so}\:\int_{−\infty} ^{+\infty} \frac{\mathrm{2}{x}}{\mathrm{4}+{x}^{\mathrm{2}} }{dx}=\mathrm{0} \\ $$ $${anoother}\:{way}... \\ $$ $${x}=\mathrm{2}{tan}\theta\:\:{dx}=\mathrm{2}{sec}^{\mathrm{2}} \theta{d}\theta \\ $$ $$\int_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{2}×\mathrm{2}{tan}\theta×\mathrm{2}{sec}^{\mathrm{2}} \theta{d}\theta}{\mathrm{4}+\mathrm{4}{tan}^{\mathrm{2}} \theta} \\ $$ $$=\int_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{8}{tan}\theta{sec}^{\mathrm{2}} \theta}{\mathrm{4}{sec}^{\mathrm{2}} \theta}{d}\theta \\ $$ $$=\mathrm{2}\mid{lnsec}\theta\mid_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \\ $$ $$=−\mathrm{2}\mid{lncos}\theta\mid_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \\ $$ $$=−\mathrm{2}\left[{lncos}\left(\frac{\pi}{\mathrm{2}}\right)−{lncos}\left(−\frac{\pi}{\mathrm{2}}\right)\right] \\ $$ $$=\mathrm{0} \\ $$ $${it}\:{is}\:{my}\:{opinion}...{let}\:{others}\:{comment}... \\ $$
Commented byJoel578 last updated on 20/Jan/19

$${thank}\:{you}\:{very}\:{much} \\ $$
