Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 60981 by necx1 last updated on 28/May/19

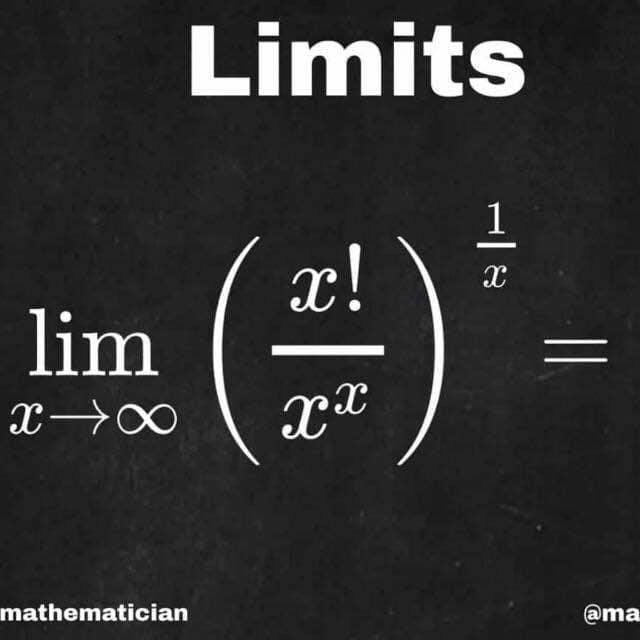
Commented by Prithwish sen last updated on 28/May/19

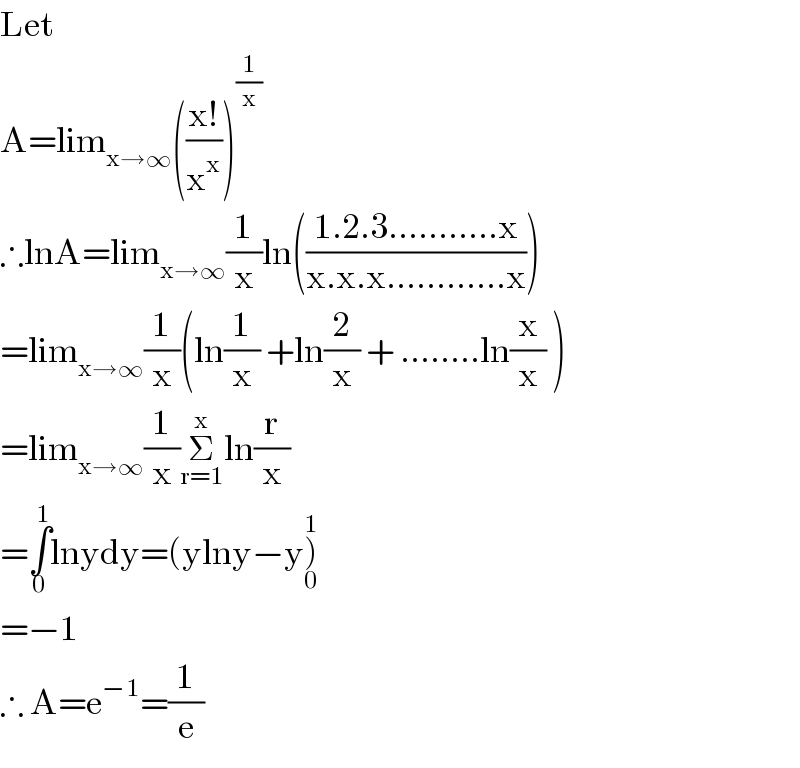
Let A=lim_(x→∞) (((x!)/x^x ))^(1/x) ∴lnA=lim_(x→∞) (1/x)ln(((1.2.3...........x)/(x.x.x............x))) =lim_(x→∞) (1/x)(ln(1/x) +ln(2/x) + ........ln(x/x) ) =lim_(x→∞) (1/x)Σ_(r=1) ^x ln(r/x) =∫_0 ^1 lnydy=(ylny−y)_0 ^1 =−1 ∴ A=e^(−1) =(1/e)

$$\mathrm{Let}\: \\ $$$$\mathrm{A}=\mathrm{lim}_{\mathrm{x}\rightarrow\infty} \left(\frac{\mathrm{x}!}{\mathrm{x}^{\mathrm{x}} }\right)^{\frac{\mathrm{1}}{\mathrm{x}}} \\ $$$$\therefore\mathrm{lnA}=\mathrm{lim}_{\mathrm{x}\rightarrow\infty} \frac{\mathrm{1}}{\mathrm{x}}\mathrm{ln}\left(\frac{\mathrm{1}.\mathrm{2}.\mathrm{3}...........\mathrm{x}}{\mathrm{x}.\mathrm{x}.\mathrm{x}............\mathrm{x}}\right) \\ $$$$=\mathrm{lim}_{\mathrm{x}\rightarrow\infty} \frac{\mathrm{1}}{\mathrm{x}}\left(\mathrm{ln}\frac{\mathrm{1}}{\mathrm{x}}\:+\mathrm{ln}\frac{\mathrm{2}}{\mathrm{x}}\:+\:........\mathrm{ln}\frac{\mathrm{x}}{\mathrm{x}}\:\right) \\ $$$$=\mathrm{lim}_{\mathrm{x}\rightarrow\infty} \frac{\mathrm{1}}{\mathrm{x}}\underset{\mathrm{r}=\mathrm{1}} {\overset{\mathrm{x}} {\sum}}\mathrm{ln}\frac{\mathrm{r}}{\mathrm{x}} \\ $$$$=\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\mathrm{lnydy}=\left(\mathrm{ylny}−\mathrm{y}\underset{\mathrm{0}} {\overset{\mathrm{1}} {\right)}} \\ $$$$=−\mathrm{1} \\ $$$$\therefore\:\mathrm{A}=\mathrm{e}^{−\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{e}} \\ $$

Commented by tanmay last updated on 28/May/19

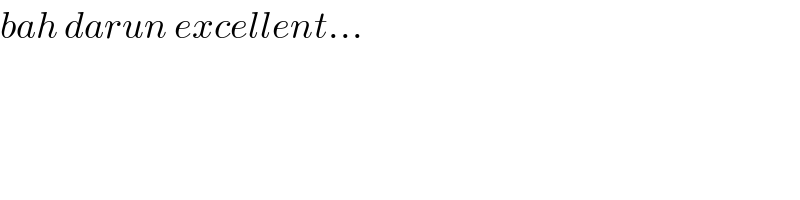
bah darun excellent...

$${bah}\:{darun}\:{excellent}... \\ $$

Commented by Prithwish sen last updated on 28/May/19

thank you sir.

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Commented by ajfour last updated on 28/May/19

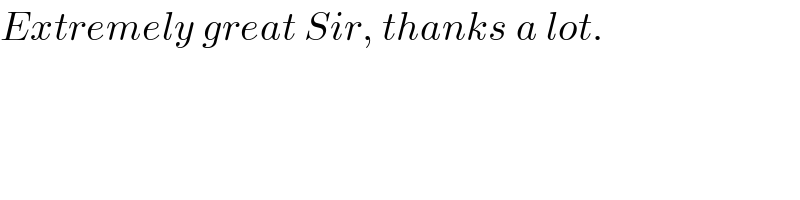
Extremely great Sir, thanks a lot.

$${Extremely}\:{great}\:{Sir},\:{thanks}\:{a}\:{lot}. \\ $$

Commented by Prithwish sen last updated on 28/May/19

welcome.

$$\mathrm{welcome}.\: \\ $$

Commented by Mr X pcx last updated on 28/May/19

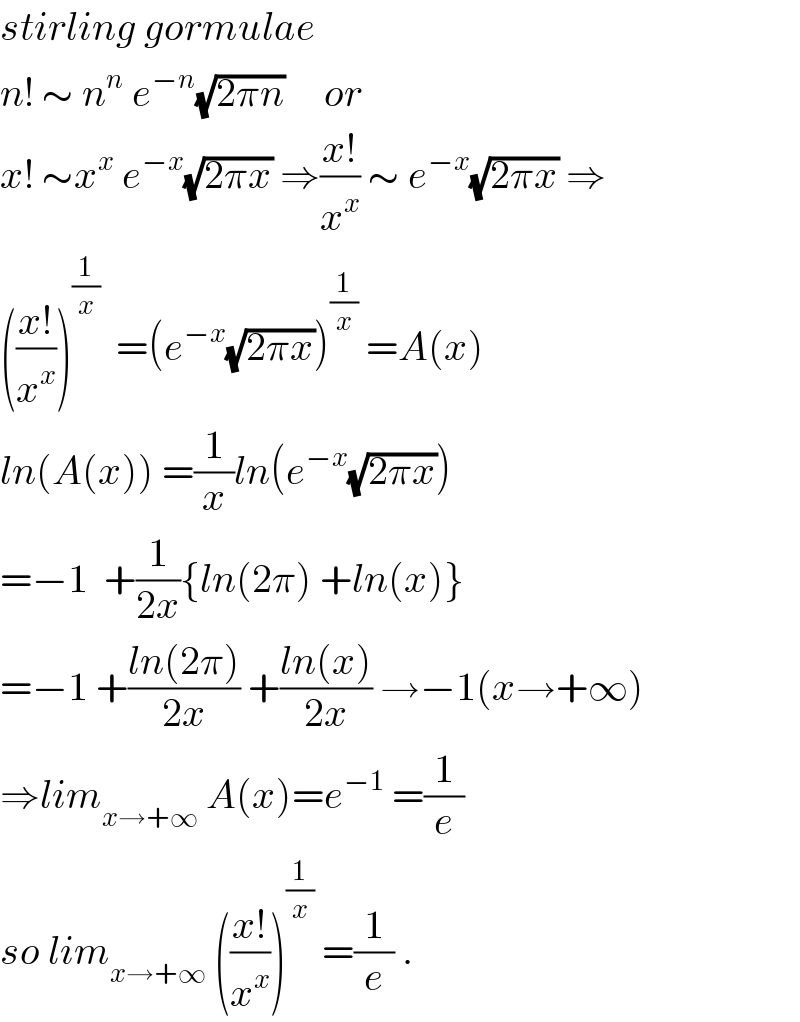
stirling gormulae n! ∼ n^n e^(−n) (√(2πn)) or x! ∼x^x e^(−x) (√(2πx)) ⇒((x!)/x^x ) ∼ e^(−x) (√(2πx)) ⇒ (((x!)/x^x ))^(1/x) =(e^(−x) (√(2πx)))^(1/x) =A(x) ln(A(x)) =(1/x)ln(e^(−x) (√(2πx))) =−1 +(1/(2x)){ln(2π) +ln(x)} =−1 +((ln(2π))/(2x)) +((ln(x))/(2x)) →−1(x→+∞) ⇒lim_(x→+∞) A(x)=e^(−1) =(1/e) so lim_(x→+∞) (((x!)/x^x ))^(1/x) =(1/e) .

$${stirling}\:{gormulae} \\ $$$${n}!\:\sim\:{n}^{{n}} \:{e}^{−{n}} \sqrt{\mathrm{2}\pi{n}}\:\:\:\:\:{or}\: \\ $$$${x}!\:\sim{x}^{{x}} \:{e}^{−{x}} \sqrt{\mathrm{2}\pi{x}}\:\Rightarrow\frac{{x}!}{{x}^{{x}} }\:\sim\:{e}^{−{x}} \sqrt{\mathrm{2}\pi{x}}\:\Rightarrow \\ $$$$\left(\frac{{x}!}{{x}^{{x}} }\right)^{\frac{\mathrm{1}}{{x}}} \:\:=\left({e}^{−{x}} \sqrt{\mathrm{2}\pi{x}}\right)^{\frac{\mathrm{1}}{{x}}} \:={A}\left({x}\right) \\ $$$${ln}\left({A}\left({x}\right)\right)\:=\frac{\mathrm{1}}{{x}}{ln}\left({e}^{−{x}} \sqrt{\mathrm{2}\pi{x}}\right) \\ $$$$=−\mathrm{1}\:\:+\frac{\mathrm{1}}{\mathrm{2}{x}}\left\{{ln}\left(\mathrm{2}\pi\right)\:+{ln}\left({x}\right)\right\} \\ $$$$=−\mathrm{1}\:+\frac{{ln}\left(\mathrm{2}\pi\right)}{\mathrm{2}{x}}\:+\frac{{ln}\left({x}\right)}{\mathrm{2}{x}}\:\rightarrow−\mathrm{1}\left({x}\rightarrow+\infty\right) \\ $$$$\Rightarrow{lim}_{{x}\rightarrow+\infty} \:{A}\left({x}\right)={e}^{−\mathrm{1}} \:=\frac{\mathrm{1}}{{e}} \\ $$$${so}\:{lim}_{{x}\rightarrow+\infty} \:\left(\frac{{x}!}{{x}^{{x}} }\right)^{\frac{\mathrm{1}}{{x}}} \:=\frac{\mathrm{1}}{{e}}\:. \\ $$

Answered by MJS last updated on 28/May/19

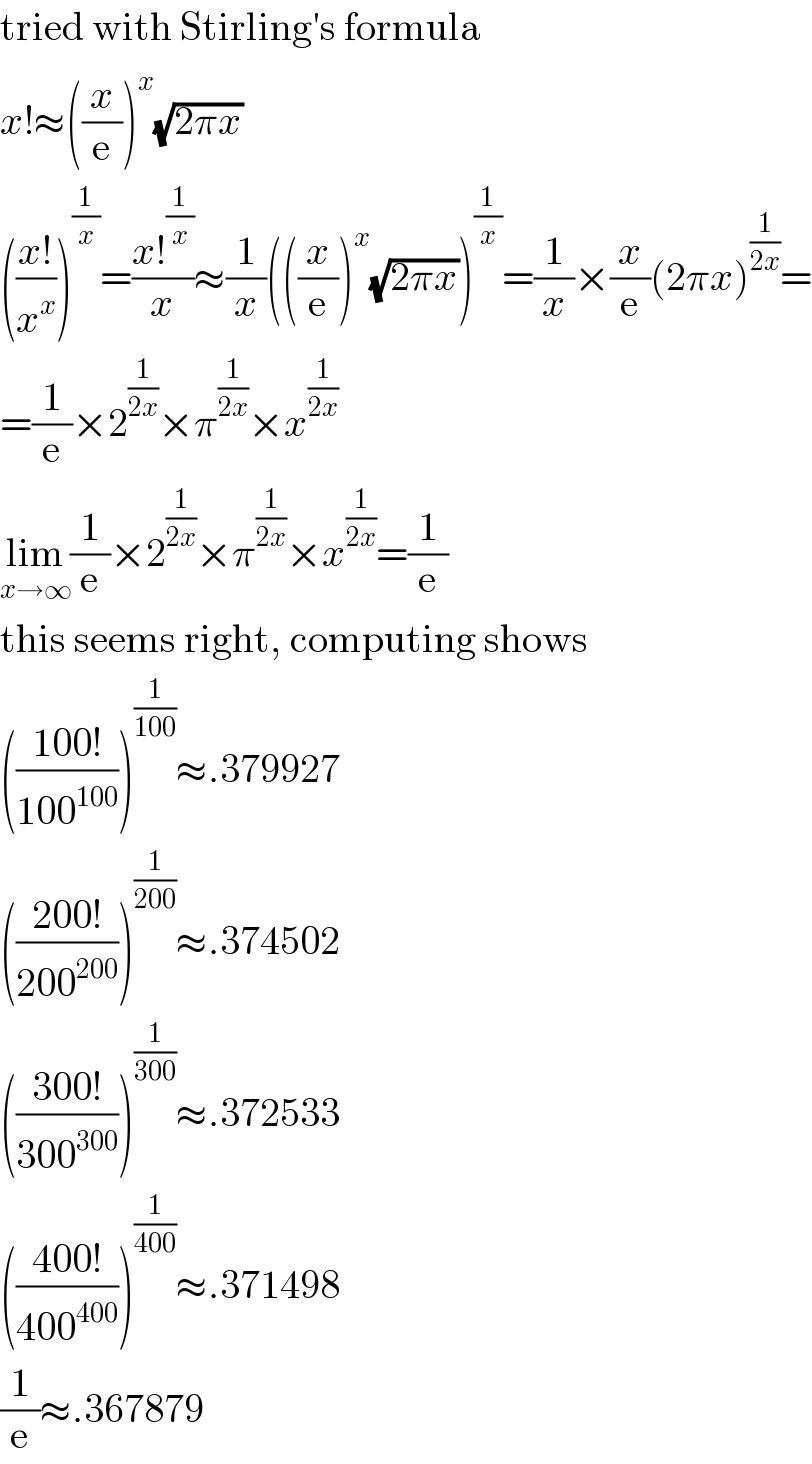
tried with Stirling′s formula x!≈((x/e))^x (√(2πx)) (((x!)/x^x ))^(1/x) =((x!^(1/x) )/x)≈(1/x)(((x/e))^x (√(2πx)))^(1/x) =(1/x)×(x/e)(2πx)^(1/(2x)) = =(1/e)×2^(1/(2x)) ×π^(1/(2x)) ×x^(1/(2x)) lim_(x→∞) (1/e)×2^(1/(2x)) ×π^(1/(2x)) ×x^(1/(2x)) =(1/e) this seems right, computing shows (((100!)/(100^(100) )))^(1/(100)) ≈.379927 (((200!)/(200^(200) )))^(1/(200)) ≈.374502 (((300!)/(300^(300) )))^(1/(300)) ≈.372533 (((400!)/(400^(400) )))^(1/(400)) ≈.371498 (1/e)≈.367879

$$\mathrm{tried}\:\mathrm{with}\:\mathrm{Stirling}'\mathrm{s}\:\mathrm{formula} \\ $$$${x}!\approx\left(\frac{{x}}{\mathrm{e}}\right)^{{x}} \sqrt{\mathrm{2}\pi{x}} \\ $$$$\left(\frac{{x}!}{{x}^{{x}} }\right)^{\frac{\mathrm{1}}{{x}}} =\frac{{x}!^{\frac{\mathrm{1}}{{x}}} }{{x}}\approx\frac{\mathrm{1}}{{x}}\left(\left(\frac{{x}}{\mathrm{e}}\right)^{{x}} \sqrt{\mathrm{2}\pi{x}}\right)^{\frac{\mathrm{1}}{{x}}} =\frac{\mathrm{1}}{{x}}×\frac{{x}}{\mathrm{e}}\left(\mathrm{2}\pi{x}\right)^{\frac{\mathrm{1}}{\mathrm{2}{x}}} = \\ $$$$=\frac{\mathrm{1}}{\mathrm{e}}×\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}{x}}} ×\pi^{\frac{\mathrm{1}}{\mathrm{2}{x}}} ×{x}^{\frac{\mathrm{1}}{\mathrm{2}{x}}} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{e}}×\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}{x}}} ×\pi^{\frac{\mathrm{1}}{\mathrm{2}{x}}} ×{x}^{\frac{\mathrm{1}}{\mathrm{2}{x}}} =\frac{\mathrm{1}}{\mathrm{e}} \\ $$$$\mathrm{this}\:\mathrm{seems}\:\mathrm{right},\:\mathrm{computing}\:\mathrm{shows} \\ $$$$\left(\frac{\mathrm{100}!}{\mathrm{100}^{\mathrm{100}} }\right)^{\frac{\mathrm{1}}{\mathrm{100}}} \approx.\mathrm{379927} \\ $$$$\left(\frac{\mathrm{200}!}{\mathrm{200}^{\mathrm{200}} }\right)^{\frac{\mathrm{1}}{\mathrm{200}}} \approx.\mathrm{374502} \\ $$$$\left(\frac{\mathrm{300}!}{\mathrm{300}^{\mathrm{300}} }\right)^{\frac{\mathrm{1}}{\mathrm{300}}} \approx.\mathrm{372533} \\ $$$$\left(\frac{\mathrm{400}!}{\mathrm{400}^{\mathrm{400}} }\right)^{\frac{\mathrm{1}}{\mathrm{400}}} \approx.\mathrm{371498} \\ $$$$\frac{\mathrm{1}}{\mathrm{e}}\approx.\mathrm{367879} \\ $$

Commented by tanmay last updated on 28/May/19

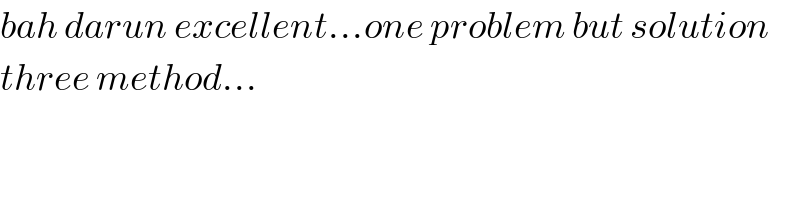
bah darun excellent...one problem but solution three method...

$${bah}\:{darun}\:{excellent}...{one}\:{problem}\:{but}\:{solution} \\ $$$${three}\:{method}... \\ $$

Answered by tanmay last updated on 28/May/19

lim_(x→∞) (a_(x+1) /a_x ) =lim_(x→∞) (({(((x+1)!)/((x+1)^(x+1) ))})/((((x!)/x^x )))) =lim_(x→∞) (((x+1)!)/(x!))×(1/(((x+1)^(x+1) )/x^x )) =lim_(x→∞) (x+1)×(1/((x+1)(((x+1)/x))^x )) =lim_(x→∞) (1/((1+(1/x))^x )) now t=(1/x) y=lim_(t→0) (1/((1+t)^(1/t) )) lny=lim_(t→0) [((−ln(1+t))/t)] lny=−1 y=e^(−1) so lim_(x→∞) (((x!)/x^x ))^(1/x) =e^(−1) →it is the answer using cauchy second theorem on limit lim_(n→∞) (a_n )^(1/n) =l if lim_(n→∞) (a_(n+1) /a_n )=l

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{{a}_{{x}+\mathrm{1}} }{{a}_{{x}} } \\ $$$$=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\:\frac{\left\{\frac{\left({x}+\mathrm{1}\right)!}{\left({x}+\mathrm{1}\right)^{{x}+\mathrm{1}} }\right\}}{\left(\frac{{x}!}{{x}^{{x}} }\right)} \\ $$$$=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\left({x}+\mathrm{1}\right)!}{{x}!}×\frac{\mathrm{1}}{\frac{\left({x}+\mathrm{1}\right)^{{x}+\mathrm{1}} }{{x}^{{x}} }} \\ $$$$=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\left({x}+\mathrm{1}\right)×\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right)\left(\frac{{x}+\mathrm{1}}{{x}}\right)^{{x}} } \\ $$$$=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{1}}{\left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)^{{x}} } \\ $$$${now}\:{t}=\frac{\mathrm{1}}{{x}} \\ $$$${y}=\underset{{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}}{\left(\mathrm{1}+{t}\right)^{\frac{\mathrm{1}}{{t}}} } \\ $$$${lny}=\underset{{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\left[\frac{−{ln}\left(\mathrm{1}+{t}\right)}{{t}}\right] \\ $$$${lny}=−\mathrm{1} \\ $$$${y}={e}^{−\mathrm{1}} \\ $$$${so}\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{x}!}{{x}^{{x}} }\right)^{\frac{\mathrm{1}}{{x}}} ={e}^{−\mathrm{1}} \rightarrow{it}\:{is}\:{the}\:{answer} \\ $$$${using}\:{cauchy}\:{second}\:{theorem}\:{on}\:{limit} \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left({a}_{{n}} \right)^{\frac{\mathrm{1}}{{n}}} ={l} \\ $$$${if}\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:\frac{{a}_{{n}+\mathrm{1}} }{{a}_{{n}} }={l} \\ $$

Commented by necx1 last updated on 28/May/19

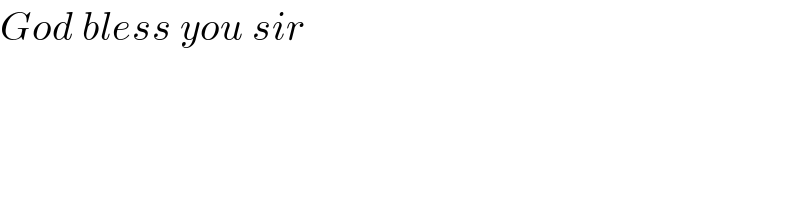
God bless you sir

$${God}\:{bless}\:{you}\:{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com