
Question Number 63976 by Scientist0000001 last updated on 11/Jul/19
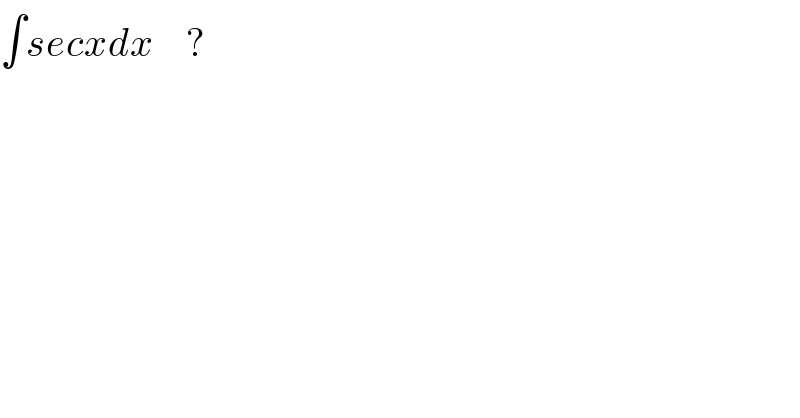
$$\int{secxdx}\:\:\:\:? \\ $$
Commented by Prithwish sen last updated on 12/Jul/19
![∫((sec^2 (x/2))/(1−tan^2 (x/2)))dx putting tan(x/2) = t sec^2 (x/2)dx = 2dt ∴2∫(dt/(1−t^2 )) = ∫[(1/(1−t))+(1/(1+t))]dt =ln∣((1+t)/(1−t))∣ + C](Q63978.png)
$$\int\frac{\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}\mathrm{dx}\:\:\:\:\mathrm{putting}\:\mathrm{tan}\frac{\mathrm{x}}{\mathrm{2}}\:=\:\mathrm{t} \\ $$$$\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\mathrm{dx}\:=\:\mathrm{2dt}\:\:\:\:\therefore\mathrm{2}\int\frac{\mathrm{dt}}{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }\:=\:\int\left[\frac{\mathrm{1}}{\mathrm{1}−\mathrm{t}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{t}}\right]\mathrm{dt} \\ $$$$=\mathrm{ln}\mid\frac{\mathrm{1}+\mathrm{t}}{\mathrm{1}−\mathrm{t}}\mid\:+\:\mathrm{C} \\ $$
Commented by hknkrc46 last updated on 11/Jul/19
![∫secxdx=∫(dx/(cosx))=∫((cosx)/(cos^2 x))dx=∫((cosx)/(1−sin^2 x))dx sinx=u⇒cosxdx=du ∫(du/(1−u^2 ))=(1/2)∫(((1−u)+(1+u))/((1−u)(1+u)))du =(1/2)[∫((1−u)/((1−u)(1+u)))du+∫((1+u)/((1−u)(1+u)))du] =(1/2)[∫(du/(1+u))+∫(du/(1−u))]=(1/2)[ln (1+u)−ln (1−u)]+c =(1/2)ln (((1+u)/(1−u)))+c=(1/2)ln (((1+sinx)/(1−sinx)))+c ★ =(1/2)ln ((((1+sinx)^2 )/((1+sinx)(1−sinx))))+c =(1/2)ln ((((1+sinx)^2 )/(1−sin^2 x)))+c =(1/2)ln ((((1+sinx)^2 )/(cos^2 x)))+c=ln (((1+sinx)/(cosx)))+c =ln ((1/(cosx))+((sinx)/(cosx)))+c=ln (secx+tanx)+c ★★ =ln (secx+tanx)+ln e^c =ln (e^c (secx+tanx)) =ln (c_1 (secx+tanx)) ★★★ {c , c_1 ∈R }](Q63988.png)
$$\int{secxdx}=\int\frac{{dx}}{{cosx}}=\int\frac{{cosx}}{{cos}^{\mathrm{2}} {x}}{dx}=\int\frac{{cosx}}{\mathrm{1}−{sin}^{\mathrm{2}} {x}}{dx} \\ $$$${sinx}={u}\Rightarrow{cosxdx}={du} \\ $$$$\int\frac{{du}}{\mathrm{1}−{u}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\left(\mathrm{1}−{u}\right)+\left(\mathrm{1}+{u}\right)}{\left(\mathrm{1}−{u}\right)\left(\mathrm{1}+{u}\right)}{du} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left[\int\frac{\mathrm{1}−{u}}{\left(\mathrm{1}−{u}\right)\left(\mathrm{1}+{u}\right)}{du}+\int\frac{\mathrm{1}+{u}}{\left(\mathrm{1}−{u}\right)\left(\mathrm{1}+{u}\right)}{du}\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left[\int\frac{{du}}{\mathrm{1}+{u}}+\int\frac{{du}}{\mathrm{1}−{u}}\right]=\frac{\mathrm{1}}{\mathrm{2}}\left[\mathrm{ln}\:\left(\mathrm{1}+{u}\right)−\mathrm{ln}\:\left(\mathrm{1}−{u}\right)\right]+{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\frac{\mathrm{1}+{u}}{\mathrm{1}−{u}}\right)+{c}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\frac{\mathrm{1}+{sinx}}{\mathrm{1}−{sinx}}\right)+{c}\:\bigstar \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\frac{\left(\mathrm{1}+{sinx}\right)^{\mathrm{2}} }{\left(\mathrm{1}+{sinx}\right)\left(\mathrm{1}−{sinx}\right)}\right)+{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\frac{\left(\mathrm{1}+{sinx}\right)^{\mathrm{2}} }{\mathrm{1}−{sin}^{\mathrm{2}} {x}}\right)+{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\frac{\left(\mathrm{1}+{sinx}\right)^{\mathrm{2}} }{{cos}^{\mathrm{2}} {x}}\right)+{c}=\mathrm{ln}\:\left(\frac{\mathrm{1}+{sinx}}{{cosx}}\right)+{c} \\ $$$$=\mathrm{ln}\:\left(\frac{\mathrm{1}}{{cosx}}+\frac{{sinx}}{{cosx}}\right)+{c}=\mathrm{ln}\:\left({secx}+{tanx}\right)+{c}\:\bigstar\bigstar \\ $$$$=\mathrm{ln}\:\left({secx}+{tanx}\right)+\mathrm{ln}\:{e}^{{c}} =\mathrm{ln}\:\left({e}^{{c}} \left({secx}+{tanx}\right)\right) \\ $$$$=\mathrm{ln}\:\left({c}_{\mathrm{1}} \left({secx}+{tanx}\right)\right)\:\bigstar\bigstar\bigstar \\ $$$$\left\{{c}\:,\:{c}_{\mathrm{1}} \:\in\mathbb{R}\:\right\} \\ $$$$ \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 11/Jul/19
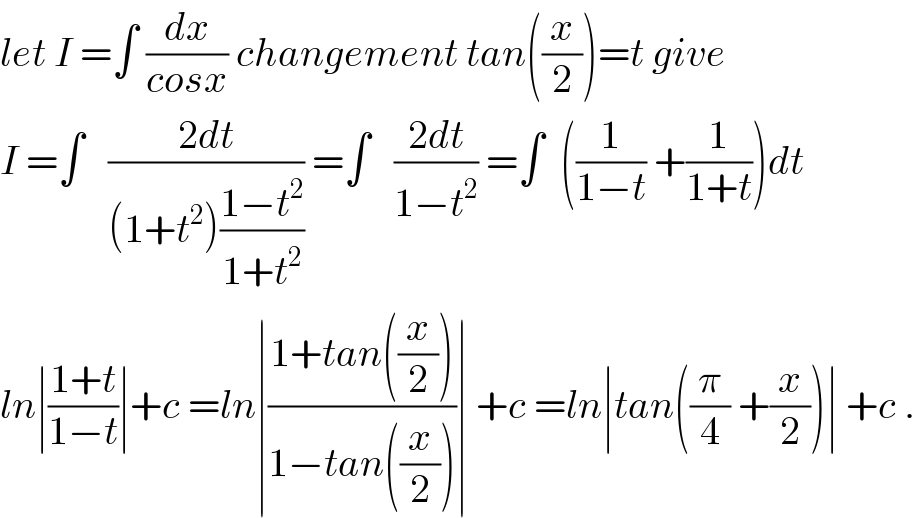
$${let}\:{I}\:=\int\:\frac{{dx}}{{cosx}}\:{changement}\:{tan}\left(\frac{{x}}{\mathrm{2}}\right)={t}\:{give} \\ $$$${I}\:=\int\:\:\:\frac{\mathrm{2}{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }}\:=\int\:\:\:\frac{\mathrm{2}{dt}}{\mathrm{1}−{t}^{\mathrm{2}} }\:=\int\:\:\left(\frac{\mathrm{1}}{\mathrm{1}−{t}}\:+\frac{\mathrm{1}}{\mathrm{1}+{t}}\right){dt} \\ $$$${ln}\mid\frac{\mathrm{1}+{t}}{\mathrm{1}−{t}}\mid+{c}\:={ln}\mid\frac{\mathrm{1}+{tan}\left(\frac{{x}}{\mathrm{2}}\right)}{\mathrm{1}−{tan}\left(\frac{{x}}{\mathrm{2}}\right)}\mid\:+{c}\:={ln}\mid{tan}\left(\frac{\pi}{\mathrm{4}}\:+\frac{{x}}{\mathrm{2}}\right)\mid\:+{c}\:. \\ $$
