Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 65569 by MJS last updated on 31/Jul/19

reposting this: x^4 +(1−2a)x^2 −2ax+1=0 solve for a>0∧x>0 a^5 −((23)/8)x^4 −((49)/4)x^3 +((27)/8)x^2 +3x+(9/8)=0 ⇒ 3 real solutions a_1 <a_2 <a_3 we cannot solve exactly (?) so it′s not possible to get all the below solutions exactly, only rough approximations for the following α,β,γ,δ ∈R the possible natures of the zeros: (1) a<a_1 ∨a_2 <a<a_3 ⇒ x=α∨x=β∨x=γ±δi (2) a>a_3 ⇒ x=α∨x=β∨x=γ∨x=δ (3) a_1 <a<a_2 ⇒ x=α±βi∨x=γ±δi (4) a=a_3 ⇒ x=α=β∨x=γ∨x=δ (5) a=a_1 ∨a=a_2 ⇒ x=α=β∨x=γ±δi

$$\mathrm{reposting}\:\mathrm{this}: \\ $$ $${x}^{\mathrm{4}} +\left(\mathrm{1}−\mathrm{2}{a}\right){x}^{\mathrm{2}} −\mathrm{2}{ax}+\mathrm{1}=\mathrm{0} \\ $$ $$\mathrm{solve}\:\mathrm{for}\:{a}>\mathrm{0}\wedge{x}>\mathrm{0} \\ $$ $$ \\ $$ $$\:\:\:\:\:\:\:\:\:\:{a}^{\mathrm{5}} −\frac{\mathrm{23}}{\mathrm{8}}{x}^{\mathrm{4}} −\frac{\mathrm{49}}{\mathrm{4}}{x}^{\mathrm{3}} +\frac{\mathrm{27}}{\mathrm{8}}{x}^{\mathrm{2}} +\mathrm{3}{x}+\frac{\mathrm{9}}{\mathrm{8}}=\mathrm{0} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\Rightarrow\:\mathrm{3}\:\mathrm{real}\:\mathrm{solutions}\:{a}_{\mathrm{1}} <{a}_{\mathrm{2}} <{a}_{\mathrm{3}} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\mathrm{we}\:\mathrm{cannot}\:\mathrm{solve}\:\mathrm{exactly}\:\left(?\right)\:\mathrm{so}\:\mathrm{it}'\mathrm{s}\:\mathrm{not} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\mathrm{possible}\:\mathrm{to}\:\mathrm{get}\:\mathrm{all}\:\mathrm{the}\:\mathrm{below}\:\mathrm{solutions} \\ $$ $$\:\:\:\:\:\:\:\:\:\:\mathrm{exactly},\:\mathrm{only}\:\mathrm{rough}\:\mathrm{approximations} \\ $$ $$ \\ $$ $$\mathrm{for}\:\mathrm{the}\:\mathrm{following}\:\alpha,\beta,\gamma,\delta\:\in\mathbb{R} \\ $$ $$\mathrm{the}\:\mathrm{possible}\:\mathrm{natures}\:\mathrm{of}\:\mathrm{the}\:\mathrm{zeros}: \\ $$ $$\left(\mathrm{1}\right)\:\:{a}<{a}_{\mathrm{1}} \vee{a}_{\mathrm{2}} <{a}<{a}_{\mathrm{3}} \:\Rightarrow\:{x}=\alpha\vee{x}=\beta\vee{x}=\gamma\pm\delta\mathrm{i} \\ $$ $$\left(\mathrm{2}\right)\:\:{a}>{a}_{\mathrm{3}} \:\Rightarrow\:{x}=\alpha\vee{x}=\beta\vee{x}=\gamma\vee{x}=\delta \\ $$ $$\left(\mathrm{3}\right)\:\:{a}_{\mathrm{1}} <{a}<{a}_{\mathrm{2}} \:\Rightarrow\:{x}=\alpha\pm\beta\mathrm{i}\vee{x}=\gamma\pm\delta\mathrm{i} \\ $$ $$\left(\mathrm{4}\right)\:\:{a}={a}_{\mathrm{3}} \:\Rightarrow\:{x}=\alpha=\beta\vee{x}=\gamma\vee{x}=\delta \\ $$ $$\left(\mathrm{5}\right)\:\:{a}={a}_{\mathrm{1}} \vee{a}={a}_{\mathrm{2}} \:\Rightarrow\:{x}=\alpha=\beta\vee{x}=\gamma\pm\delta\mathrm{i} \\ $$

Commented byMJS last updated on 31/Jul/19

please someone check

$$\mathrm{please}\:\mathrm{someone}\:\mathrm{check} \\ $$

Answered by Prithwish sen last updated on 31/Jul/19

Commented byPrithwish sen last updated on 31/Jul/19

Commented byPrithwish sen last updated on 31/Jul/19

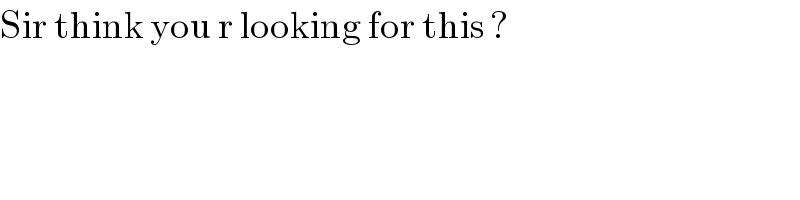
Sir think you r looking for this ?

$$\mathrm{Sir}\:\mathrm{think}\:\mathrm{you}\:\mathrm{r}\:\mathrm{looking}\:\mathrm{for}\:\mathrm{this}\:? \\ $$

Commented byMJS last updated on 31/Jul/19

yes thank you but I had made 2 typos. it must be q not r in two of the cases...

$$\mathrm{yes}\:\mathrm{thank}\:\mathrm{you} \\ $$ $$\mathrm{but}\:\mathrm{I}\:\mathrm{had}\:\mathrm{made}\:\mathrm{2}\:\mathrm{typos}.\:\mathrm{it}\:\mathrm{must}\:\mathrm{be}\:{q}\:\mathrm{not}\:{r} \\ $$ $$\mathrm{in}\:\mathrm{two}\:\mathrm{of}\:\mathrm{the}\:\mathrm{cases}... \\ $$

Commented byMJS last updated on 31/Jul/19

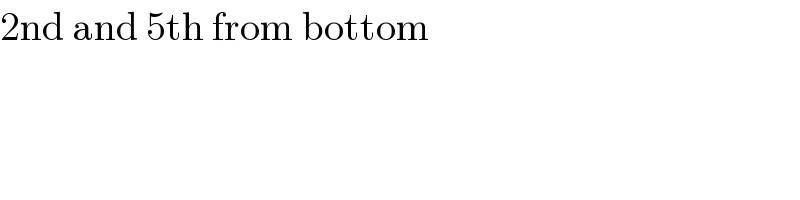
2nd and 5th from bottom

$$\mathrm{2nd}\:\mathrm{and}\:\mathrm{5th}\:\mathrm{from}\:\mathrm{bottom} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com