Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 72145 by Maclaurin Stickker last updated on 25/Oct/19

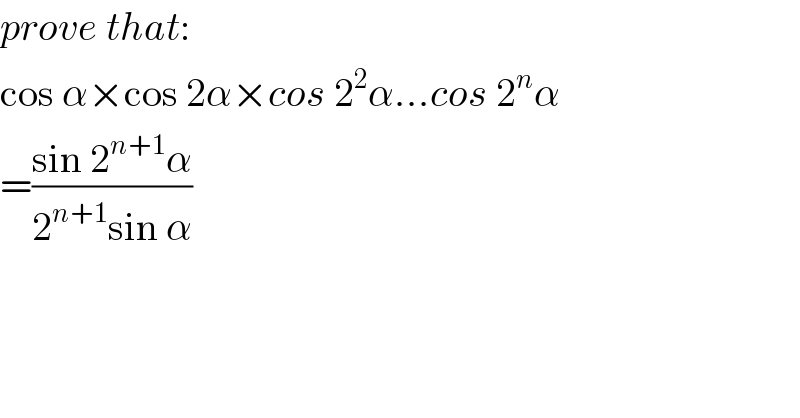
prove that: cos α×cos 2α×cos 2^2 α...cos 2^n α =((sin 2^(n+1) α)/(2^(n+1) sin α))

$${prove}\:{that}: \\ $$$$\mathrm{cos}\:\alpha×\mathrm{cos}\:\mathrm{2}\alpha×{cos}\:\mathrm{2}^{\mathrm{2}} \alpha...{cos}\:\mathrm{2}^{{n}} \alpha \\ $$$$=\frac{\mathrm{sin}\:\mathrm{2}^{{n}+\mathrm{1}} \alpha}{\mathrm{2}^{{n}+\mathrm{1}} \mathrm{sin}\:\alpha} \\ $$$$ \\ $$

Commented by kaivan.ahmadi last updated on 25/Oct/19

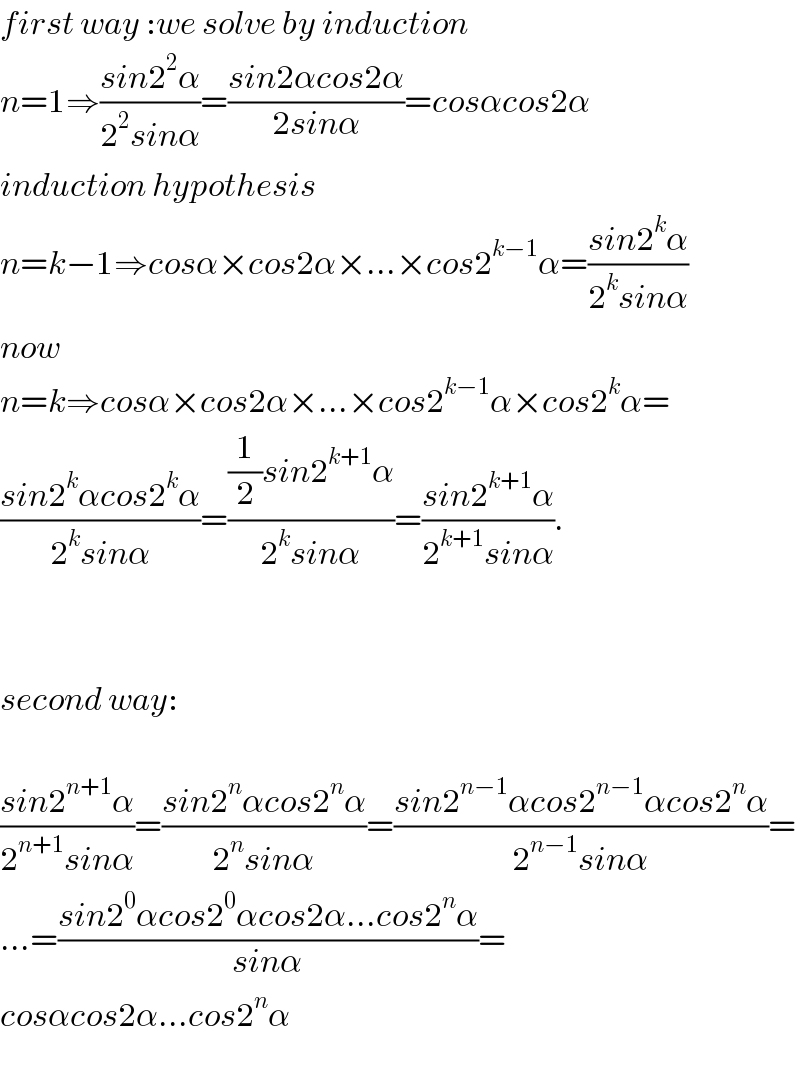
first way :we solve by induction n=1⇒((sin2^2 α)/(2^2 sinα))=((sin2αcos2α)/(2sinα))=cosαcos2α induction hypothesis n=k−1⇒cosα×cos2α×...×cos2^(k−1) α=((sin2^k α)/(2^k sinα)) now n=k⇒cosα×cos2α×...×cos2^(k−1) α×cos2^k α= ((sin2^k αcos2^k α)/(2^k sinα))=(((1/2)sin2^(k+1) α)/(2^k sinα))=((sin2^(k+1) α)/(2^(k+1) sinα)). second way: ((sin2^(n+1) α)/(2^(n+1) sinα))=((sin2^n αcos2^n α)/(2^n sinα))=((sin2^(n−1) αcos2^(n−1) αcos2^n α)/(2^(n−1) sinα))= ...=((sin2^0 αcos2^0 αcos2α...cos2^n α)/(sinα))= cosαcos2α...cos2^n α

$${first}\:{way}\::{we}\:{solve}\:{by}\:{induction} \\ $$$${n}=\mathrm{1}\Rightarrow\frac{{sin}\mathrm{2}^{\mathrm{2}} \alpha}{\mathrm{2}^{\mathrm{2}} {sin}\alpha}=\frac{{sin}\mathrm{2}\alpha{cos}\mathrm{2}\alpha}{\mathrm{2}{sin}\alpha}={cos}\alpha{cos}\mathrm{2}\alpha \\ $$$${induction}\:{hypothesis} \\ $$$${n}={k}−\mathrm{1}\Rightarrow{cos}\alpha×{cos}\mathrm{2}\alpha×...×{cos}\mathrm{2}^{{k}−\mathrm{1}} \alpha=\frac{{sin}\mathrm{2}^{{k}} \alpha}{\mathrm{2}^{{k}} {sin}\alpha} \\ $$$${now} \\ $$$${n}={k}\Rightarrow{cos}\alpha×{cos}\mathrm{2}\alpha×...×{cos}\mathrm{2}^{{k}−\mathrm{1}} \alpha×{cos}\mathrm{2}^{{k}} \alpha= \\ $$$$\frac{{sin}\mathrm{2}^{{k}} \alpha{cos}\mathrm{2}^{{k}} \alpha}{\mathrm{2}^{{k}} {sin}\alpha}=\frac{\frac{\mathrm{1}}{\mathrm{2}}{sin}\mathrm{2}^{{k}+\mathrm{1}} \alpha}{\mathrm{2}^{{k}} {sin}\alpha}=\frac{{sin}\mathrm{2}^{{k}+\mathrm{1}} \alpha}{\mathrm{2}^{{k}+\mathrm{1}} {sin}\alpha}. \\ $$$$ \\ $$$$ \\ $$$${second}\:{way}: \\ $$$$ \\ $$$$\frac{{sin}\mathrm{2}^{{n}+\mathrm{1}} \alpha}{\mathrm{2}^{{n}+\mathrm{1}} {sin}\alpha}=\frac{{sin}\mathrm{2}^{{n}} \alpha{cos}\mathrm{2}^{{n}} \alpha}{\mathrm{2}^{{n}} {sin}\alpha}=\frac{{sin}\mathrm{2}^{{n}−\mathrm{1}} \alpha{cos}\mathrm{2}^{{n}−\mathrm{1}} \alpha{cos}\mathrm{2}^{{n}} \alpha}{\mathrm{2}^{{n}−\mathrm{1}} {sin}\alpha}= \\ $$$$...=\frac{{sin}\mathrm{2}^{\mathrm{0}} \alpha{cos}\mathrm{2}^{\mathrm{0}} \alpha{cos}\mathrm{2}\alpha...{cos}\mathrm{2}^{{n}} \alpha}{{sin}\alpha}= \\ $$$${cos}\alpha{cos}\mathrm{2}\alpha...{cos}\mathrm{2}^{{n}} \alpha \\ $$$$ \\ $$

Commented by Maclaurin Stickker last updated on 25/Oct/19

Thank you for your time.

$${Thank}\:{you}\:{for}\:{your}\:{time}. \\ $$

Commented by mathmax by abdo last updated on 25/Oct/19

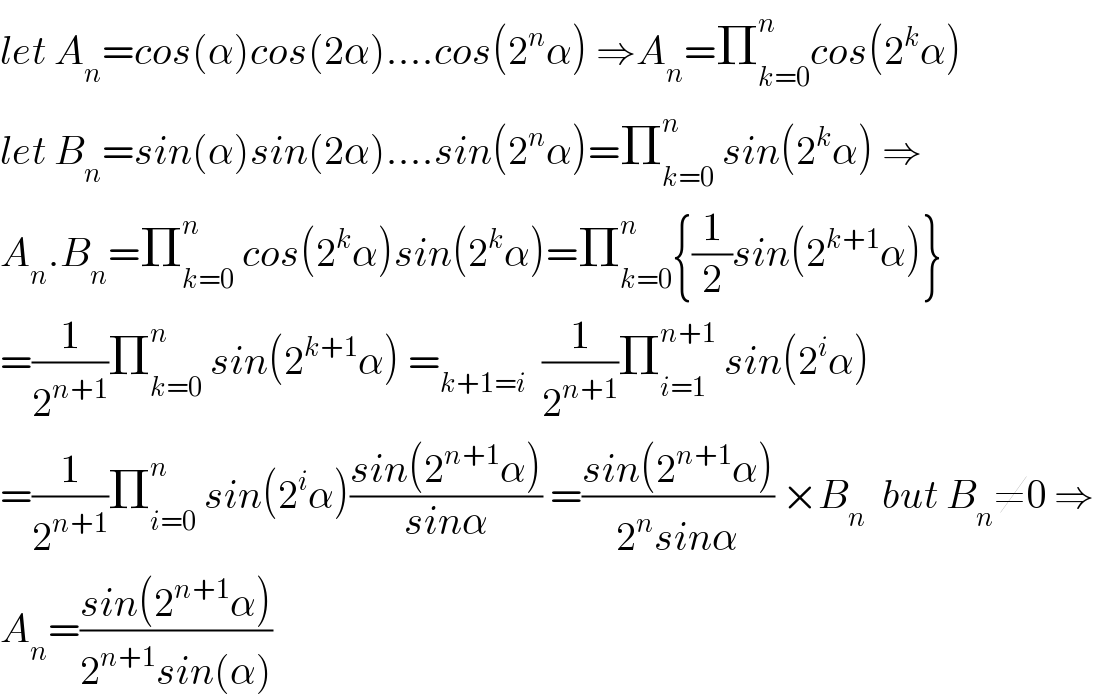
let A_n =cos(α)cos(2α)....cos(2^n α) ⇒A_n =Π_(k=0) ^n cos(2^k α) let B_n =sin(α)sin(2α)....sin(2^n α)=Π_(k=0) ^n sin(2^k α) ⇒ A_n .B_n =Π_(k=0) ^n cos(2^k α)sin(2^k α)=Π_(k=0) ^n {(1/2)sin(2^(k+1) α)} =(1/2^(n+1) )Π_(k=0) ^n sin(2^(k+1) α) =_(k+1=i) (1/2^(n+1) )Π_(i=1) ^(n+1) sin(2^i α) =(1/2^(n+1) )Π_(i=0) ^n sin(2^i α)((sin(2^(n+1) α))/(sinα)) =((sin(2^(n+1) α))/(2^n sinα)) ×B_n but B_n ≠0 ⇒ A_n =((sin(2^(n+1) α))/(2^(n+1) sin(α)))

$${let}\:{A}_{{n}} ={cos}\left(\alpha\right){cos}\left(\mathrm{2}\alpha\right)....{cos}\left(\mathrm{2}^{{n}} \alpha\right)\:\Rightarrow{A}_{{n}} =\prod_{{k}=\mathrm{0}} ^{{n}} {cos}\left(\mathrm{2}^{{k}} \alpha\right) \\ $$$${let}\:{B}_{{n}} ={sin}\left(\alpha\right){sin}\left(\mathrm{2}\alpha\right)....{sin}\left(\mathrm{2}^{{n}} \alpha\right)=\prod_{{k}=\mathrm{0}} ^{{n}} \:{sin}\left(\mathrm{2}^{{k}} \alpha\right)\:\Rightarrow \\ $$$${A}_{{n}} .{B}_{{n}} =\prod_{{k}=\mathrm{0}} ^{{n}} \:{cos}\left(\mathrm{2}^{{k}} \alpha\right){sin}\left(\mathrm{2}^{{k}} \alpha\right)=\prod_{{k}=\mathrm{0}} ^{{n}} \left\{\frac{\mathrm{1}}{\mathrm{2}}{sin}\left(\mathrm{2}^{{k}+\mathrm{1}} \alpha\right)\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} }\prod_{{k}=\mathrm{0}} ^{{n}} \:{sin}\left(\mathrm{2}^{{k}+\mathrm{1}} \alpha\right)\:=_{{k}+\mathrm{1}={i}} \:\:\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} }\prod_{{i}=\mathrm{1}} ^{{n}+\mathrm{1}} \:{sin}\left(\mathrm{2}^{{i}} \alpha\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} }\prod_{{i}=\mathrm{0}} ^{{n}} \:{sin}\left(\mathrm{2}^{{i}} \alpha\right)\frac{{sin}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right)}{{sin}\alpha}\:=\frac{{sin}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right)}{\mathrm{2}^{{n}} {sin}\alpha}\:×{B}_{{n}} \:\:{but}\:{B}_{{n}} \neq\mathrm{0}\:\Rightarrow \\ $$$${A}_{{n}} =\frac{{sin}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right)}{\mathrm{2}^{{n}+\mathrm{1}} {sin}\left(\alpha\right)} \\ $$

Commented by mathmax by abdo last updated on 25/Oct/19

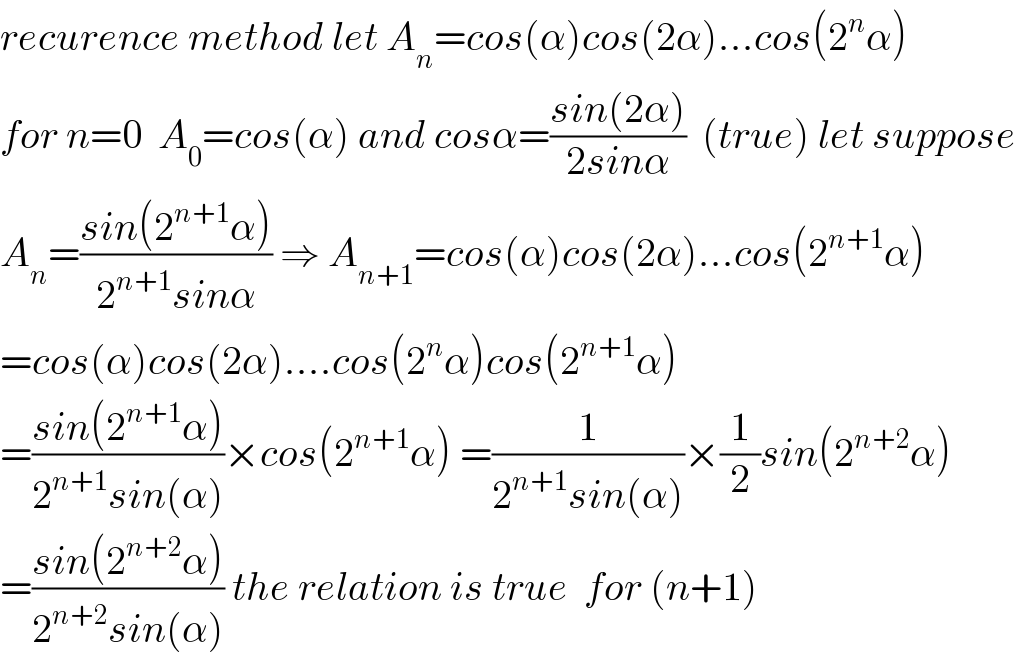
recurence method let A_n =cos(α)cos(2α)...cos(2^n α) for n=0 A_0 =cos(α) and cosα=((sin(2α))/(2sinα)) (true) let suppose A_n =((sin(2^(n+1) α))/(2^(n+1) sinα)) ⇒ A_(n+1) =cos(α)cos(2α)...cos(2^(n+1) α) =cos(α)cos(2α)....cos(2^n α)cos(2^(n+1) α) =((sin(2^(n+1) α))/(2^(n+1) sin(α)))×cos(2^(n+1) α) =(1/(2^(n+1) sin(α)))×(1/2)sin(2^(n+2) α) =((sin(2^(n+2) α))/(2^(n+2) sin(α))) the relation is true for (n+1)

$${recurence}\:{method}\:{let}\:{A}_{{n}} ={cos}\left(\alpha\right){cos}\left(\mathrm{2}\alpha\right)...{cos}\left(\mathrm{2}^{{n}} \alpha\right) \\ $$$${for}\:{n}=\mathrm{0}\:\:{A}_{\mathrm{0}} ={cos}\left(\alpha\right)\:{and}\:{cos}\alpha=\frac{{sin}\left(\mathrm{2}\alpha\right)}{\mathrm{2}{sin}\alpha}\:\:\left({true}\right)\:{let}\:{suppose} \\ $$$${A}_{{n}} =\frac{{sin}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right)}{\mathrm{2}^{{n}+\mathrm{1}} {sin}\alpha}\:\Rightarrow\:{A}_{{n}+\mathrm{1}} ={cos}\left(\alpha\right){cos}\left(\mathrm{2}\alpha\right)...{cos}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right) \\ $$$$={cos}\left(\alpha\right){cos}\left(\mathrm{2}\alpha\right)....{cos}\left(\mathrm{2}^{{n}} \alpha\right){cos}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right) \\ $$$$=\frac{{sin}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right)}{\mathrm{2}^{{n}+\mathrm{1}} {sin}\left(\alpha\right)}×{cos}\left(\mathrm{2}^{{n}+\mathrm{1}} \alpha\right)\:=\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} {sin}\left(\alpha\right)}×\frac{\mathrm{1}}{\mathrm{2}}{sin}\left(\mathrm{2}^{{n}+\mathrm{2}} \alpha\right) \\ $$$$=\frac{{sin}\left(\mathrm{2}^{{n}+\mathrm{2}} \alpha\right)}{\mathrm{2}^{{n}+\mathrm{2}} {sin}\left(\alpha\right)}\:{the}\:{relation}\:{is}\:{true}\:\:{for}\:\left({n}+\mathrm{1}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com