Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 75437 by Master last updated on 11/Dec/19

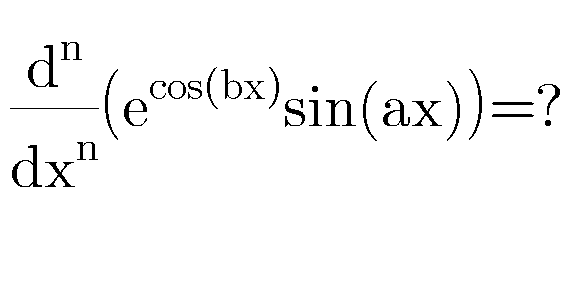
Commented by Master last updated on 11/Dec/19

sir mind is power please

$$\mathrm{sir}\:\boldsymbol{\mathrm{mind}}\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{power}}\:\:\mathrm{please}\: \\ $$

Commented by Master last updated on 11/Dec/19

sir mathmax

$$\mathrm{sir}\:\mathrm{mathmax} \\ $$

Commented by mathmax by abdo last updated on 12/Dec/19

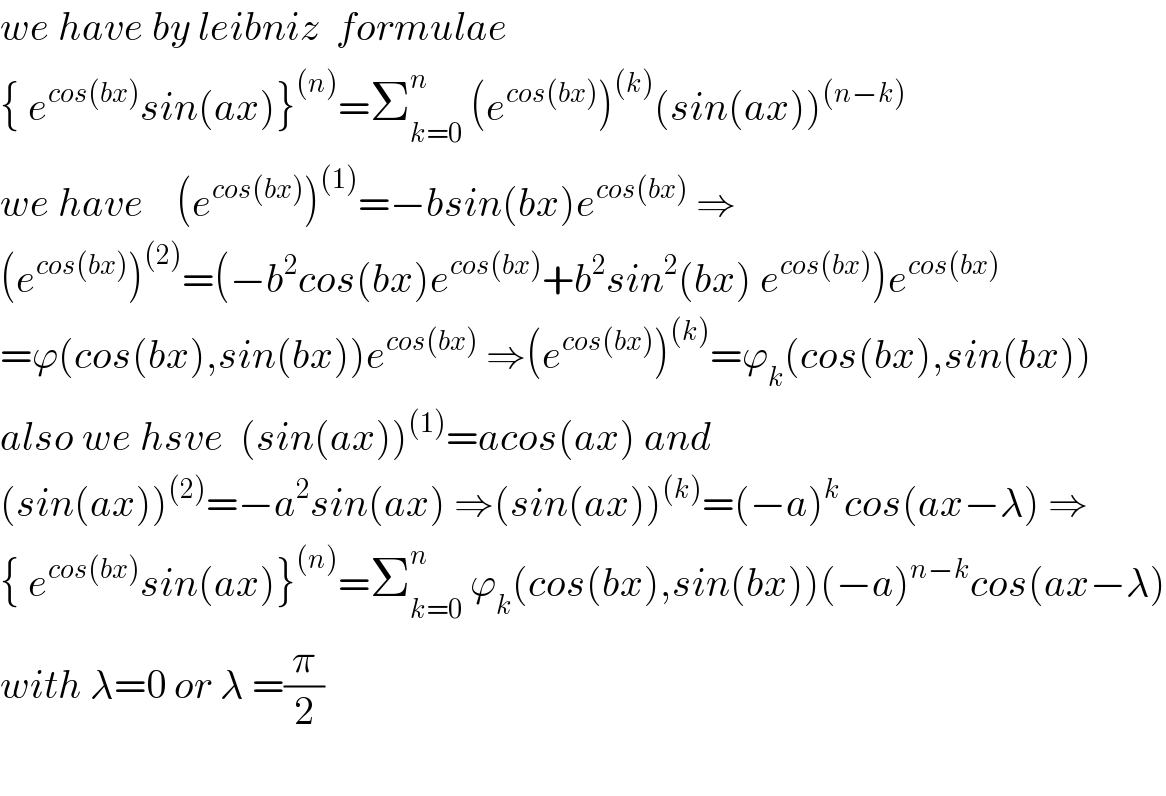
we have by leibniz formulae { e^(cos(bx)) sin(ax)}^((n)) =Σ_(k=0) ^n (e^(cos(bx)) )^((k)) (sin(ax))^((n−k)) we have (e^(cos(bx)) )^((1)) =−bsin(bx)e^(cos(bx)) ⇒ (e^(cos(bx)) )^((2)) =(−b^2 cos(bx)e^(cos(bx)) +b^2 sin^2 (bx) e^(cos(bx)) )e^(cos(bx)) =ϕ(cos(bx),sin(bx))e^(cos(bx)) ⇒(e^(cos(bx)) )^((k)) =ϕ_k (cos(bx),sin(bx)) also we hsve (sin(ax))^((1)) =acos(ax) and (sin(ax))^((2)) =−a^2 sin(ax) ⇒(sin(ax))^((k)) =(−a)^(k ) cos(ax−λ) ⇒ { e^(cos(bx)) sin(ax)}^((n)) =Σ_(k=0) ^n ϕ_k (cos(bx),sin(bx))(−a)^(n−k) cos(ax−λ) with λ=0 or λ =(π/2)

$${we}\:{have}\:{by}\:{leibniz}\:\:{formulae}\: \\ $$$$\left\{\:{e}^{{cos}\left({bx}\right)} {sin}\left({ax}\right)\right\}^{\left({n}\right)} =\sum_{{k}=\mathrm{0}} ^{{n}} \:\left({e}^{{cos}\left({bx}\right)} \right)^{\left({k}\right)} \left({sin}\left({ax}\right)\right)^{\left({n}−{k}\right)} \\ $$$${we}\:{have}\:\:\:\:\left({e}^{{cos}\left({bx}\right)} \right)^{\left(\mathrm{1}\right)} =−{bsin}\left({bx}\right){e}^{{cos}\left({bx}\right)} \:\Rightarrow \\ $$$$\left({e}^{{cos}\left({bx}\right)} \right)^{\left(\mathrm{2}\right)} =\left(−{b}^{\mathrm{2}} {cos}\left({bx}\right){e}^{{cos}\left({bx}\right)} +{b}^{\mathrm{2}} {sin}^{\mathrm{2}} \left({bx}\right)\:{e}^{{cos}\left({bx}\right)} \right){e}^{{cos}\left({bx}\right)} \\ $$$$=\varphi\left({cos}\left({bx}\right),{sin}\left({bx}\right)\right){e}^{{cos}\left({bx}\right)} \:\Rightarrow\left({e}^{{cos}\left({bx}\right)} \right)^{\left({k}\right)} =\varphi_{{k}} \left({cos}\left({bx}\right),{sin}\left({bx}\right)\right) \\ $$$${also}\:{we}\:{hsve}\:\:\left({sin}\left({ax}\right)\right)^{\left(\mathrm{1}\right)} ={acos}\left({ax}\right)\:{and}\: \\ $$$$\left({sin}\left({ax}\right)\right)^{\left(\mathrm{2}\right)} =−{a}^{\mathrm{2}} {sin}\left({ax}\right)\:\Rightarrow\left({sin}\left({ax}\right)\right)^{\left({k}\right)} =\left(−{a}\right)^{{k}\:} {cos}\left({ax}−\lambda\right)\:\Rightarrow \\ $$$$\left\{\:{e}^{{cos}\left({bx}\right)} {sin}\left({ax}\right)\right\}^{\left({n}\right)} =\sum_{{k}=\mathrm{0}} ^{{n}} \:\varphi_{{k}} \left({cos}\left({bx}\right),{sin}\left({bx}\right)\right)\left(−{a}\right)^{{n}−{k}} {cos}\left({ax}−\lambda\right) \\ $$$${with}\:\lambda=\mathrm{0}\:{or}\:\lambda\:=\frac{\pi}{\mathrm{2}} \\ $$$$ \\ $$

Commented by mathmax by abdo last updated on 12/Dec/19

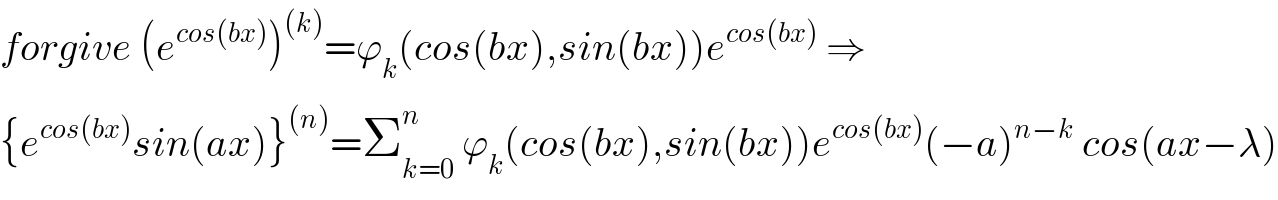
forgive (e^(cos(bx)) )^((k)) =ϕ_k (cos(bx),sin(bx))e^(cos(bx)) ⇒ {e^(cos(bx)) sin(ax)}^((n)) =Σ_(k=0) ^n ϕ_k (cos(bx),sin(bx))e^(cos(bx)) (−a)^(n−k) cos(ax−λ)

$${forgive}\:\left({e}^{{cos}\left({bx}\right)} \right)^{\left({k}\right)} =\varphi_{{k}} \left({cos}\left({bx}\right),{sin}\left({bx}\right)\right){e}^{{cos}\left({bx}\right)} \:\Rightarrow \\ $$$$\left\{{e}^{{cos}\left({bx}\right)} {sin}\left({ax}\right)\right\}^{\left({n}\right)} =\sum_{{k}=\mathrm{0}} ^{{n}} \:\varphi_{{k}} \left({cos}\left({bx}\right),{sin}\left({bx}\right)\right){e}^{{cos}\left({bx}\right)} \left(−{a}\right)^{{n}−{k}} \:{cos}\left({ax}−\lambda\right) \\ $$

Commented by Master last updated on 12/Dec/19

thank you. great!

$$\mathrm{thank}\:\mathrm{you}.\:\mathrm{great}! \\ $$

Commented by mathmax by abdo last updated on 12/Dec/19

you are welcome.

$${you}\:{are}\:{welcome}. \\ $$

Answered by mind is power last updated on 12/Dec/19

hello sir sorry i have not times this days ,let f(x)=e^(cos(bx)) sin(ax) f′(x)={acos(ax)−bsin(bx)sin(ax)}e^(cos(bx)) lets find g_n (x) suche f^((n)) (x)=g_n (x)e^(cos(bx)) ⇒f^((n+1)) (x)=g_(n+1) (x)e^(cos(bx)) ={g′_n (x)−bsin(bx)g_n (x)}e^(cos(bx)) ⇒g_(n+1) (x)=g′_n (x)−bsin(bx)g_n (x) g_0 (x)=sin(ax) ⇒ { ((g_0 (x)=sin(ax))),((g_(n+1) (x)=g′_n (x)−bsin(bx)g_n (x))) :} f^n (x)=g_n (x)e^(cos(bx)) by reccursion

$$\mathrm{hello}\:\mathrm{sir}\:\mathrm{sorry}\:\:\mathrm{i}\:\mathrm{have}\:\:\mathrm{not}\:\mathrm{times}\:\mathrm{this}\:\mathrm{days}\:,\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{e}^{\mathrm{cos}\left(\mathrm{bx}\right)} \mathrm{sin}\left(\mathrm{ax}\right) \\ $$$$ \\ $$$$\mathrm{f}'\left(\mathrm{x}\right)=\left\{\mathrm{acos}\left(\mathrm{ax}\right)−\mathrm{bsin}\left(\mathrm{bx}\right)\mathrm{sin}\left(\mathrm{ax}\right)\right\}\mathrm{e}^{\mathrm{cos}\left(\mathrm{bx}\right)} \\ $$$$\mathrm{lets}\:\mathrm{find}\:\mathrm{g}_{\mathrm{n}} \left(\mathrm{x}\right)\:\mathrm{suche} \\ $$$$\mathrm{f}^{\left(\mathrm{n}\right)} \left(\mathrm{x}\right)=\mathrm{g}_{\mathrm{n}} \left(\mathrm{x}\right)\mathrm{e}^{\mathrm{cos}\left(\mathrm{bx}\right)} \\ $$$$\Rightarrow\mathrm{f}^{\left(\mathrm{n}+\mathrm{1}\right)} \left(\mathrm{x}\right)=\mathrm{g}_{\mathrm{n}+\mathrm{1}} \left(\mathrm{x}\right)\mathrm{e}^{\mathrm{cos}\left(\mathrm{bx}\right)} =\left\{\mathrm{g}'_{\mathrm{n}} \left(\mathrm{x}\right)−\mathrm{bsin}\left(\mathrm{bx}\right)\mathrm{g}_{\mathrm{n}} \left(\mathrm{x}\right)\right\}\mathrm{e}^{\mathrm{cos}\left(\mathrm{bx}\right)} \\ $$$$\Rightarrow\mathrm{g}_{\mathrm{n}+\mathrm{1}} \left(\mathrm{x}\right)=\mathrm{g}'_{\mathrm{n}} \left(\mathrm{x}\right)−\mathrm{bsin}\left(\mathrm{bx}\right)\mathrm{g}_{\mathrm{n}} \left(\mathrm{x}\right) \\ $$$$\mathrm{g}_{\mathrm{0}} \left(\mathrm{x}\right)=\mathrm{sin}\left(\mathrm{ax}\right) \\ $$$$\Rightarrow\begin{cases}{\mathrm{g}_{\mathrm{0}} \left(\mathrm{x}\right)=\mathrm{sin}\left(\mathrm{ax}\right)}\\{\mathrm{g}_{\mathrm{n}+\mathrm{1}} \left(\mathrm{x}\right)=\mathrm{g}'_{\mathrm{n}} \left(\mathrm{x}\right)−\mathrm{bsin}\left(\mathrm{bx}\right)\mathrm{g}_{\mathrm{n}} \left(\mathrm{x}\right)}\end{cases} \\ $$$$\mathrm{f}^{\mathrm{n}} \left(\mathrm{x}\right)=\mathrm{g}_{\mathrm{n}} \left(\mathrm{x}\right)\mathrm{e}^{\mathrm{cos}\left(\mathrm{bx}\right)} \:\:\:\:\mathrm{by}\:\mathrm{reccursion} \\ $$$$ \\ $$$$ \\ $$

Commented by Master last updated on 13/Dec/19

yes sir thank you.

$$\mathrm{yes}\:\mathrm{sir}\:\mathrm{thank}\:\mathrm{you}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com