Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 76077 by vishalbhardwaj last updated on 23/Dec/19

Find the maximum area of an isosceles triangle inscribed in an ellipse (x^2 /a^2 ) + (y^2 /b^2 ) = 1with its vetrex at one end of the major axis ? ?

$$\boldsymbol{\mathrm{Find}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{maximum}}\:\boldsymbol{\mathrm{area}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{an}}\:\boldsymbol{\mathrm{isosceles}}\:\boldsymbol{\mathrm{triangle}} \\ $$$$\boldsymbol{\mathrm{inscribed}}\:\boldsymbol{\mathrm{in}}\:\boldsymbol{\mathrm{an}}\:\boldsymbol{\mathrm{ellipse}}\:\frac{\boldsymbol{{x}}^{\mathrm{2}} }{\boldsymbol{{a}}^{\mathrm{2}} }\:+\:\frac{\boldsymbol{{y}}^{\mathrm{2}} }{\boldsymbol{{b}}^{\mathrm{2}} }\:=\:\mathrm{1}\boldsymbol{\mathrm{with}}\:\boldsymbol{\mathrm{its}}\:\boldsymbol{\mathrm{vetrex}}\: \\ $$$$\boldsymbol{\mathrm{at}}\:\boldsymbol{\mathrm{one}}\:\boldsymbol{\mathrm{end}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{major}}\:\boldsymbol{\mathrm{axis}}\:?\:? \\ $$

Commented by MJS last updated on 23/Dec/19

the centroid of the triangle is in the center of the ellipse the idea is: construct a circle with r=a insert an equilateral triangle with one vertex in ((a),(0) ) now multiply all y−values by (b/a) ⇒ the circle becomes the ellipse ⇒ the triangle becomes the one you′re searching

$$\mathrm{the}\:\mathrm{centroid}\:\mathrm{of}\:\mathrm{the}\:\mathrm{triangle}\:\mathrm{is}\:\mathrm{in}\:\mathrm{the}\:\mathrm{center} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{ellipse} \\ $$$$\mathrm{the}\:\mathrm{idea}\:\mathrm{is}: \\ $$$$\mathrm{construct}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{with}\:{r}={a} \\ $$$$\mathrm{insert}\:\mathrm{an}\:\mathrm{equilateral}\:\mathrm{triangle}\:\mathrm{with}\:\mathrm{one}\:\mathrm{vertex} \\ $$$$\mathrm{in}\:\begin{pmatrix}{{a}}\\{\mathrm{0}}\end{pmatrix} \\ $$$$\mathrm{now}\:\mathrm{multiply}\:\mathrm{all}\:{y}−\mathrm{values}\:\mathrm{by}\:\frac{{b}}{{a}} \\ $$$$\Rightarrow\:\mathrm{the}\:\mathrm{circle}\:\mathrm{becomes}\:\mathrm{the}\:\mathrm{ellipse} \\ $$$$\Rightarrow\:\mathrm{the}\:\mathrm{triangle}\:\mathrm{becomes}\:\mathrm{the}\:\mathrm{one}\:\mathrm{you}'\mathrm{re} \\ $$$$\:\:\:\:\:\mathrm{searching} \\ $$

Commented by MJS last updated on 23/Dec/19

btw all triangles you construct this way, out of an equilateral triangle, no matter where you put the vertices on the circle will share the same maximum area

$$\mathrm{btw}\:\mathrm{all}\:\mathrm{triangles}\:\mathrm{you}\:\mathrm{construct}\:\mathrm{this}\:\mathrm{way}, \\ $$$$\mathrm{out}\:\mathrm{of}\:\mathrm{an}\:\mathrm{equilateral}\:\mathrm{triangle},\:\mathrm{no}\:\mathrm{matter} \\ $$$$\mathrm{where}\:\mathrm{you}\:\mathrm{put}\:\mathrm{the}\:\mathrm{vertices}\:\mathrm{on}\:\mathrm{the}\:\mathrm{circle} \\ $$$$\mathrm{will}\:\mathrm{share}\:\mathrm{the}\:\mathrm{same}\:\mathrm{maximum}\:\mathrm{area} \\ $$

Commented by vishalbhardwaj last updated on 23/Dec/19

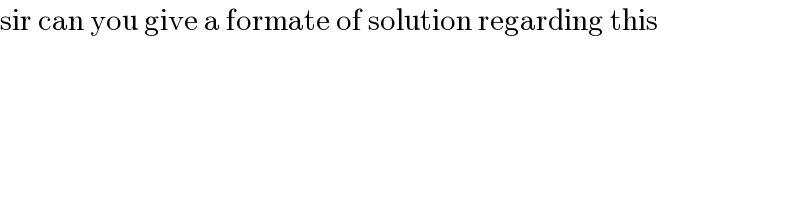
sir can you give a formate of solution regarding this

$$\mathrm{sir}\:\mathrm{can}\:\mathrm{you}\:\mathrm{give}\:\mathrm{a}\:\mathrm{formate}\:\mathrm{of}\:\mathrm{solution}\:\mathrm{regarding}\:\mathrm{this} \\ $$

Answered by MJS last updated on 23/Dec/19

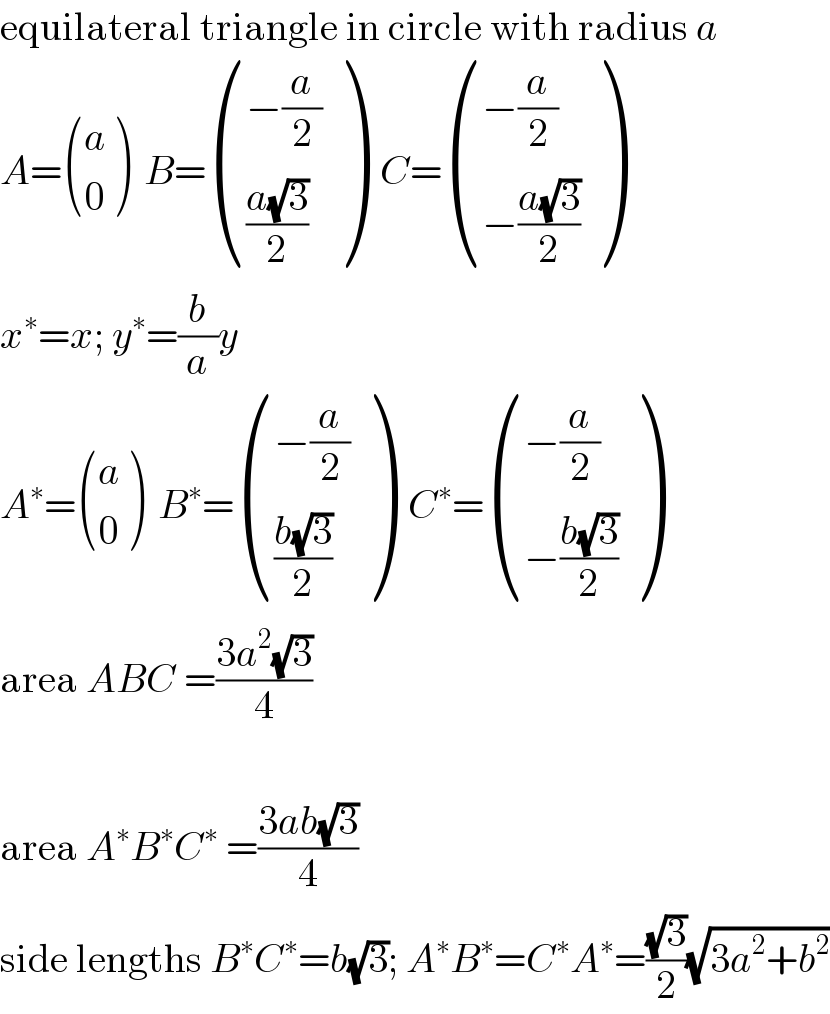
equilateral triangle in circle with radius a A= ((a),(0) ) B= (((−(a/2))),(((a(√3))/2)) ) C= (((−(a/2))),((−((a(√3))/2))) ) x^∗ =x; y^∗ =(b/a)y A^∗ = ((a),(0) ) B^∗ = (((−(a/2))),(((b(√3))/2)) ) C^∗ = (((−(a/2))),((−((b(√3))/2))) ) area ABC =((3a^2 (√3))/4) area A^∗ B^∗ C^∗ =((3ab(√3))/4) side lengths B^∗ C^∗ =b(√3); A^∗ B^∗ =C^∗ A^∗ =((√3)/2)(√(3a^2 +b^2 ))

$$\mathrm{equilateral}\:\mathrm{triangle}\:\mathrm{in}\:\mathrm{circle}\:\mathrm{with}\:\mathrm{radius}\:{a}\: \\ $$$${A}=\begin{pmatrix}{{a}}\\{\mathrm{0}}\end{pmatrix}\:\:{B}=\begin{pmatrix}{−\frac{{a}}{\mathrm{2}}}\\{\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{2}}}\end{pmatrix}\:\:{C}=\begin{pmatrix}{−\frac{{a}}{\mathrm{2}}}\\{−\frac{{a}\sqrt{\mathrm{3}}}{\mathrm{2}}}\end{pmatrix} \\ $$$${x}^{\ast} ={x};\:{y}^{\ast} =\frac{{b}}{{a}}{y} \\ $$$${A}^{\ast} =\begin{pmatrix}{{a}}\\{\mathrm{0}}\end{pmatrix}\:\:{B}^{\ast} =\begin{pmatrix}{−\frac{{a}}{\mathrm{2}}}\\{\frac{{b}\sqrt{\mathrm{3}}}{\mathrm{2}}}\end{pmatrix}\:\:{C}^{\ast} =\begin{pmatrix}{−\frac{{a}}{\mathrm{2}}}\\{−\frac{{b}\sqrt{\mathrm{3}}}{\mathrm{2}}}\end{pmatrix} \\ $$$$\mathrm{area}\:{ABC}\:=\frac{\mathrm{3}{a}^{\mathrm{2}} \sqrt{\mathrm{3}}}{\mathrm{4}} \\ $$$$ \\ $$$$\mathrm{area}\:{A}^{\ast} {B}^{\ast} {C}^{\ast} \:=\frac{\mathrm{3}{ab}\sqrt{\mathrm{3}}}{\mathrm{4}} \\ $$$$\mathrm{side}\:\mathrm{lengths}\:{B}^{\ast} {C}^{\ast} ={b}\sqrt{\mathrm{3}};\:{A}^{\ast} {B}^{\ast} ={C}^{\ast} {A}^{\ast} =\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\sqrt{\mathrm{3}{a}^{\mathrm{2}} +{b}^{\mathrm{2}} } \\ $$

Commented by vishalbhardwaj last updated on 23/Dec/19

Dear sir, can we solve this question by using differentiation(maxima and minima condition)

$$\mathrm{Dear}\:\mathrm{sir},\:\mathrm{can}\:\mathrm{we}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{question}\:\mathrm{by}\:\mathrm{using}\: \\ $$$$\mathrm{differentiation}\left(\mathrm{maxima}\:\mathrm{and}\:\mathrm{minima}\:\mathrm{condition}\right) \\ $$$$ \\ $$$$ \\ $$

Answered by MJS last updated on 23/Dec/19

A= ((a),(0) ) B= ((x),(((b/a)(√(a^2 −x^2 )))) ) C= ((x),((−(b/a)(√(a^2 −x^2 )))) ) ∣AB∣^2 =∣CA∣^2 =(x−a)(x−((a(a^2 +b^2 ))/(a^2 −b^2 ))) ∣BC∣^2 =((4b^2 )/a^2 )(a^2 −x^2 ) area (o, p, q) = =(1/4)(√((o+p+q)(−o+p+q)(o−p+q)(o+p−q))) =(1/4)(√(2(o^2 p^2 +o^2 q^2 +p^2 q^2 −(o^4 +p^4 +q^4 ))) area (o, p, p) =(1/4)o(√(4p^2 −o^2 )) o^2 =((4b^2 )/a^2 )(a^2 −x^2 )∧p^2 =(x−a)(x−((a(a^2 +b^2 ))/(a^2 −b^2 ))) area (o, p, p) =(b/a)∣x−a∣(√(a^2 −x^2 )) (d/dx)[(b/a)∣x−a∣(√(a^2 −x^2 ))]=±((b(2x^2 −ax−a^2 ))/(a(√(a^2 −x^3 ))))=0 ⇒ x=−(a/2)

$${A}=\begin{pmatrix}{{a}}\\{\mathrm{0}}\end{pmatrix}\:\:{B}=\begin{pmatrix}{{x}}\\{\frac{{b}}{{a}}\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }}\end{pmatrix}\:\:{C}=\begin{pmatrix}{{x}}\\{−\frac{{b}}{{a}}\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }}\end{pmatrix} \\ $$$$\mid{AB}\mid^{\mathrm{2}} =\mid{CA}\mid^{\mathrm{2}} =\left({x}−{a}\right)\left({x}−\frac{{a}\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }\right) \\ $$$$\mid{BC}\mid^{\mathrm{2}} =\frac{\mathrm{4}{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} }\left({a}^{\mathrm{2}} −{x}^{\mathrm{2}} \right) \\ $$$$\mathrm{area}\:\left({o},\:{p},\:{q}\right)\:= \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\left({o}+{p}+{q}\right)\left(−{o}+{p}+{q}\right)\left({o}−{p}+{q}\right)\left({o}+{p}−{q}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{2}\left({o}^{\mathrm{2}} {p}^{\mathrm{2}} +{o}^{\mathrm{2}} {q}^{\mathrm{2}} +{p}^{\mathrm{2}} {q}^{\mathrm{2}} −\left({o}^{\mathrm{4}} +{p}^{\mathrm{4}} +{q}^{\mathrm{4}} \right)\right.} \\ $$$$\mathrm{area}\:\left({o},\:{p},\:{p}\right)\:=\frac{\mathrm{1}}{\mathrm{4}}{o}\sqrt{\mathrm{4}{p}^{\mathrm{2}} −{o}^{\mathrm{2}} } \\ $$$${o}^{\mathrm{2}} =\frac{\mathrm{4}{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} }\left({a}^{\mathrm{2}} −{x}^{\mathrm{2}} \right)\wedge{p}^{\mathrm{2}} =\left({x}−{a}\right)\left({x}−\frac{{a}\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }\right) \\ $$$$\mathrm{area}\:\left({o},\:{p},\:{p}\right)\:=\frac{{b}}{{a}}\mid{x}−{a}\mid\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} } \\ $$$$\frac{{d}}{{dx}}\left[\frac{{b}}{{a}}\mid{x}−{a}\mid\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }\right]=\pm\frac{{b}\left(\mathrm{2}{x}^{\mathrm{2}} −{ax}−{a}^{\mathrm{2}} \right)}{{a}\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{3}} }}=\mathrm{0} \\ $$$$\Rightarrow\:{x}=−\frac{{a}}{\mathrm{2}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com