Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 77229 by Maclaurin Stickker last updated on 04/Jan/20

Commented by Maclaurin Stickker last updated on 04/Jan/20

ABC is a triangle. Radius circumferences shown in the figure are drawn internally to the triangle tanging two of its sides and the incircle. Determine the radius of the incircle.

$${ABC}\:{is}\:{a}\:{triangle}.\:{Radius}\:{circumferences} \\ $$$${shown}\:{in}\:{the}\:{figure}\:{are}\:{drawn} \\ $$$${internally}\:{to}\:{the}\:{triangle}\:{tanging} \\ $$$${two}\:{of}\:{its}\:{sides}\:{and}\:{the}\:{incircle}. \\ $$$${Determine}\:{the}\:{radius}\:{of}\:{the}\:{incircle}. \\ $$$$ \\ $$

Answered by mr W last updated on 04/Jan/20

Commented by mr W last updated on 05/Jan/20

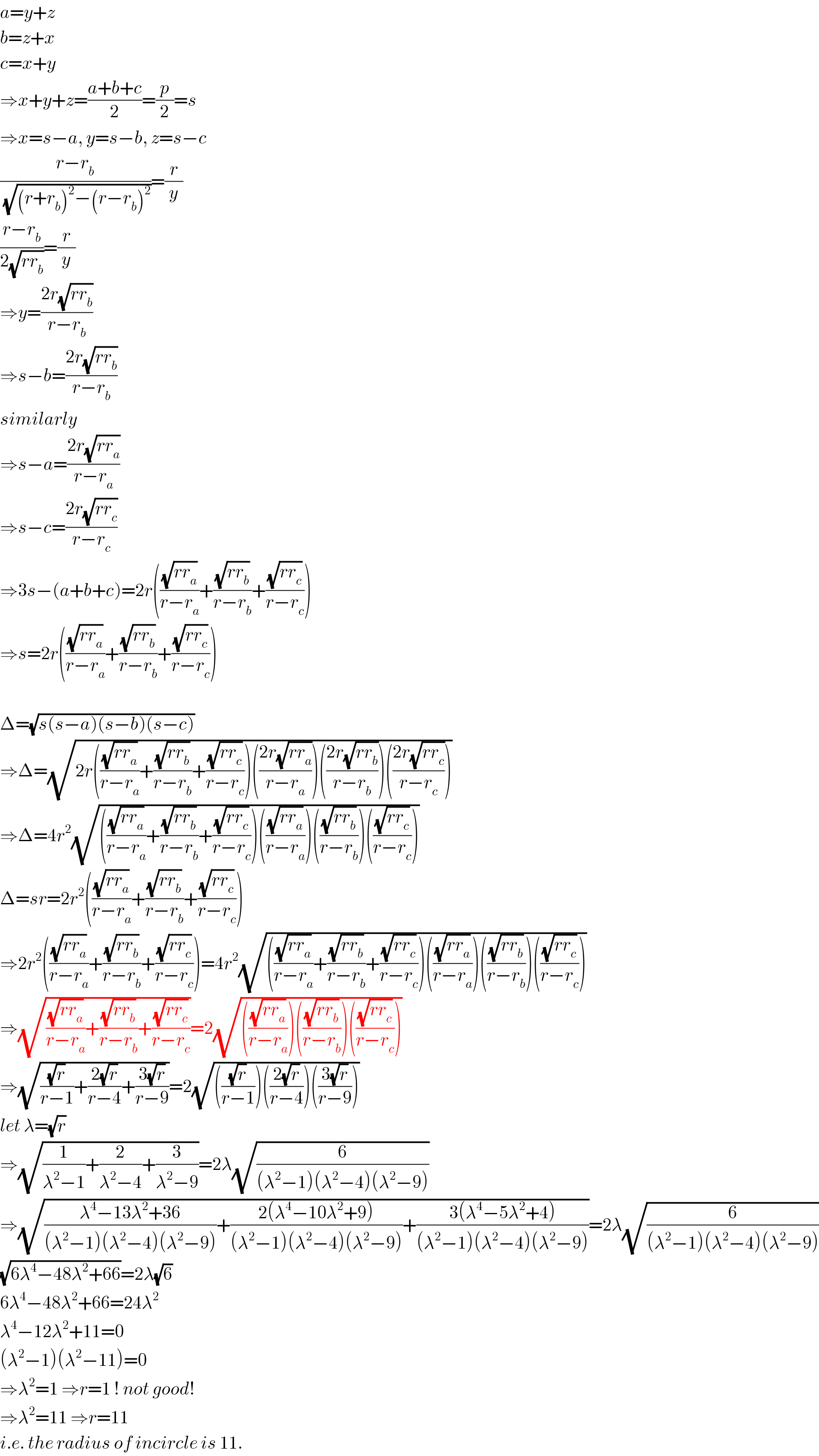
a=y+z b=z+x c=x+y ⇒x+y+z=((a+b+c)/2)=(p/2)=s ⇒x=s−a, y=s−b, z=s−c ((r−r_b )/(√((r+r_b )^2 −(r−r_b )^2 )))=(r/y) ((r−r_b )/(2(√(rr_b ))))=(r/y) ⇒y=((2r(√(rr_b )))/(r−r_b )) ⇒s−b=((2r(√(rr_b )))/(r−r_b )) similarly ⇒s−a=((2r(√(rr_a )))/(r−r_a )) ⇒s−c=((2r(√(rr_c )))/(r−r_c )) ⇒3s−(a+b+c)=2r(((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c ))) ⇒s=2r(((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c ))) Δ=(√(s(s−a)(s−b)(s−c))) ⇒Δ=(√(2r(((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c )))(((2r(√(rr_a )))/(r−r_a )))(((2r(√(rr_b )))/(r−r_b )))(((2r(√(rr_c )))/(r−r_c ))))) ⇒Δ=4r^2 (√((((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c )))(((√(rr_a ))/(r−r_a )))(((√(rr_b ))/(r−r_b )))(((√(rr_c ))/(r−r_c ))))) Δ=sr=2r^2 (((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c ))) ⇒2r^2 (((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c )))=4r^2 (√((((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c )))(((√(rr_a ))/(r−r_a )))(((√(rr_b ))/(r−r_b )))(((√(rr_c ))/(r−r_c ))))) ⇒(√(((√(rr_a ))/(r−r_a ))+((√(rr_b ))/(r−r_b ))+((√(rr_c ))/(r−r_c ))))=2(√((((√(rr_a ))/(r−r_a )))(((√(rr_b ))/(r−r_b )))(((√(rr_c ))/(r−r_c ))))) ⇒(√(((√r)/(r−1))+((2(√r))/(r−4))+((3(√r))/(r−9))))=2(√((((√r)/(r−1)))(((2(√r))/(r−4)))(((3(√r))/(r−9))))) let λ=(√r) ⇒(√((1/(λ^2 −1))+(2/(λ^2 −4))+(3/(λ^2 −9))))=2λ(√(6/((λ^2 −1)(λ^2 −4)(λ^2 −9)))) ⇒(√(((λ^4 −13λ^2 +36)/((λ^2 −1)(λ^2 −4)(λ^2 −9)))+((2(λ^4 −10λ^2 +9))/((λ^2 −1)(λ^2 −4)(λ^2 −9)))+((3(λ^4 −5λ^2 +4))/((λ^2 −1)(λ^2 −4)(λ^2 −9)))))=2λ(√(6/((λ^2 −1)(λ^2 −4)(λ^2 −9)))) (√(6λ^4 −48λ^2 +66))=2λ(√6) 6λ^4 −48λ^2 +66=24λ^2 λ^4 −12λ^2 +11=0 (λ^2 −1)(λ^2 −11)=0 ⇒λ^2 =1 ⇒r=1 ! not good! ⇒λ^2 =11 ⇒r=11 i.e. the radius of incircle is 11.

$${a}={y}+{z} \\ $$$${b}={z}+{x} \\ $$$${c}={x}+{y} \\ $$$$\Rightarrow{x}+{y}+{z}=\frac{{a}+{b}+{c}}{\mathrm{2}}=\frac{{p}}{\mathrm{2}}={s} \\ $$$$\Rightarrow{x}={s}−{a},\:{y}={s}−{b},\:{z}={s}−{c} \\ $$$$\frac{{r}−{r}_{{b}} }{\sqrt{\left({r}+{r}_{{b}} \right)^{\mathrm{2}} −\left({r}−{r}_{{b}} \right)^{\mathrm{2}} }}=\frac{{r}}{{y}} \\ $$$$\frac{{r}−{r}_{{b}} }{\mathrm{2}\sqrt{{rr}_{{b}} }}=\frac{{r}}{{y}} \\ $$$$\Rightarrow{y}=\frac{\mathrm{2}{r}\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} } \\ $$$$\Rightarrow{s}−{b}=\frac{\mathrm{2}{r}\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} } \\ $$$${similarly} \\ $$$$\Rightarrow{s}−{a}=\frac{\mathrm{2}{r}\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} } \\ $$$$\Rightarrow{s}−{c}=\frac{\mathrm{2}{r}\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} } \\ $$$$\Rightarrow\mathrm{3}{s}−\left({a}+{b}+{c}\right)=\mathrm{2}{r}\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right) \\ $$$$\Rightarrow{s}=\mathrm{2}{r}\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right) \\ $$$$ \\ $$$$\Delta=\sqrt{{s}\left({s}−{a}\right)\left({s}−{b}\right)\left({s}−{c}\right)} \\ $$$$\Rightarrow\Delta=\sqrt{\mathrm{2}{r}\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)\left(\frac{\mathrm{2}{r}\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }\right)\left(\frac{\mathrm{2}{r}\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }\right)\left(\frac{\mathrm{2}{r}\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)} \\ $$$$\Rightarrow\Delta=\mathrm{4}{r}^{\mathrm{2}} \sqrt{\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }\right)\left(\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }\right)\left(\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)} \\ $$$$\Delta={sr}=\mathrm{2}{r}^{\mathrm{2}} \left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right) \\ $$$$\Rightarrow\mathrm{2}{r}^{\mathrm{2}} \left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)=\mathrm{4}{r}^{\mathrm{2}} \sqrt{\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }\right)\left(\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }\right)\left(\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)} \\ $$$$\Rightarrow\sqrt{\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }+\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }+\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }}=\mathrm{2}\sqrt{\left(\frac{\sqrt{{rr}_{{a}} }}{{r}−{r}_{{a}} }\right)\left(\frac{\sqrt{{rr}_{{b}} }}{{r}−{r}_{{b}} }\right)\left(\frac{\sqrt{{rr}_{{c}} }}{{r}−{r}_{{c}} }\right)} \\ $$$$\Rightarrow\sqrt{\frac{\sqrt{{r}}}{{r}−\mathrm{1}}+\frac{\mathrm{2}\sqrt{{r}}}{{r}−\mathrm{4}}+\frac{\mathrm{3}\sqrt{{r}}}{{r}−\mathrm{9}}}=\mathrm{2}\sqrt{\left(\frac{\sqrt{{r}}}{{r}−\mathrm{1}}\right)\left(\frac{\mathrm{2}\sqrt{{r}}}{{r}−\mathrm{4}}\right)\left(\frac{\mathrm{3}\sqrt{{r}}}{{r}−\mathrm{9}}\right)} \\ $$$${let}\:\lambda=\sqrt{{r}} \\ $$$$\Rightarrow\sqrt{\frac{\mathrm{1}}{\lambda^{\mathrm{2}} −\mathrm{1}}+\frac{\mathrm{2}}{\lambda^{\mathrm{2}} −\mathrm{4}}+\frac{\mathrm{3}}{\lambda^{\mathrm{2}} −\mathrm{9}}}=\mathrm{2}\lambda\sqrt{\frac{\mathrm{6}}{\left(\lambda^{\mathrm{2}} −\mathrm{1}\right)\left(\lambda^{\mathrm{2}} −\mathrm{4}\right)\left(\lambda^{\mathrm{2}} −\mathrm{9}\right)}} \\ $$$$\Rightarrow\sqrt{\frac{\lambda^{\mathrm{4}} −\mathrm{13}\lambda^{\mathrm{2}} +\mathrm{36}}{\left(\lambda^{\mathrm{2}} −\mathrm{1}\right)\left(\lambda^{\mathrm{2}} −\mathrm{4}\right)\left(\lambda^{\mathrm{2}} −\mathrm{9}\right)}+\frac{\mathrm{2}\left(\lambda^{\mathrm{4}} −\mathrm{10}\lambda^{\mathrm{2}} +\mathrm{9}\right)}{\left(\lambda^{\mathrm{2}} −\mathrm{1}\right)\left(\lambda^{\mathrm{2}} −\mathrm{4}\right)\left(\lambda^{\mathrm{2}} −\mathrm{9}\right)}+\frac{\mathrm{3}\left(\lambda^{\mathrm{4}} −\mathrm{5}\lambda^{\mathrm{2}} +\mathrm{4}\right)}{\left(\lambda^{\mathrm{2}} −\mathrm{1}\right)\left(\lambda^{\mathrm{2}} −\mathrm{4}\right)\left(\lambda^{\mathrm{2}} −\mathrm{9}\right)}}=\mathrm{2}\lambda\sqrt{\frac{\mathrm{6}}{\left(\lambda^{\mathrm{2}} −\mathrm{1}\right)\left(\lambda^{\mathrm{2}} −\mathrm{4}\right)\left(\lambda^{\mathrm{2}} −\mathrm{9}\right)}} \\ $$$$\sqrt{\mathrm{6}\lambda^{\mathrm{4}} −\mathrm{48}\lambda^{\mathrm{2}} +\mathrm{66}}=\mathrm{2}\lambda\sqrt{\mathrm{6}} \\ $$$$\mathrm{6}\lambda^{\mathrm{4}} −\mathrm{48}\lambda^{\mathrm{2}} +\mathrm{66}=\mathrm{24}\lambda^{\mathrm{2}} \\ $$$$\lambda^{\mathrm{4}} −\mathrm{12}\lambda^{\mathrm{2}} +\mathrm{11}=\mathrm{0} \\ $$$$\left(\lambda^{\mathrm{2}} −\mathrm{1}\right)\left(\lambda^{\mathrm{2}} −\mathrm{11}\right)=\mathrm{0} \\ $$$$\Rightarrow\lambda^{\mathrm{2}} =\mathrm{1}\:\Rightarrow{r}=\mathrm{1}\:!\:{not}\:{good}! \\ $$$$\Rightarrow\lambda^{\mathrm{2}} =\mathrm{11}\:\Rightarrow{r}=\mathrm{11} \\ $$$${i}.{e}.\:{the}\:{radius}\:{of}\:{incircle}\:{is}\:\mathrm{11}. \\ $$

Commented by Maclaurin Stickker last updated on 04/Jan/20

wow! perfect∙

$${wow}!\:{perfect}\centerdot \\ $$

Commented by mr W last updated on 04/Jan/20

it can be simplified to r=(√(r_a r_b ))+(√(r_b r_c ))+(√(r_c r_a )) =(√(1×4))+(√(4×9))+(√(9×1)) =2+6+3 =11

$${it}\:{can}\:{be}\:{simplified}\:{to} \\ $$$${r}=\sqrt{{r}_{{a}} {r}_{{b}} }+\sqrt{{r}_{{b}} {r}_{{c}} }+\sqrt{{r}_{{c}} {r}_{{a}} } \\ $$$$=\sqrt{\mathrm{1}×\mathrm{4}}+\sqrt{\mathrm{4}×\mathrm{9}}+\sqrt{\mathrm{9}×\mathrm{1}} \\ $$$$=\mathrm{2}+\mathrm{6}+\mathrm{3} \\ $$$$=\mathrm{11} \\ $$

Commented by otchereabdullai@gmail.com last updated on 04/Jan/20

you are fantastic prof w

$$\mathrm{you}\:\mathrm{are}\:\mathrm{fantastic}\:\mathrm{prof}\:\:\mathrm{w} \\ $$

Commented by otchereabdullai@gmail.com last updated on 04/Jan/20

you are fantastic prof w

$$\mathrm{you}\:\mathrm{are}\:\mathrm{fantastic}\:\mathrm{prof}\:\:\mathrm{w} \\ $$

Commented by otchereabdullai@gmail.com last updated on 04/Jan/20

you are fantastic prof w

$$\mathrm{you}\:\mathrm{are}\:\mathrm{fantastic}\:\mathrm{prof}\:\:\mathrm{w} \\ $$

Commented by otchereabdullai@gmail.com last updated on 04/Jan/20

you are fantastic prof w

$$\mathrm{you}\:\mathrm{are}\:\mathrm{fantastic}\:\mathrm{prof}\:\:\mathrm{w} \\ $$

Commented by jagoll last updated on 05/Jan/20

waw amazing sir

$$\mathrm{waw}\:\:\mathrm{amazing}\:\mathrm{sir}\: \\ $$

Commented by peter frank last updated on 05/Jan/20

your genius

$${your}\:{genius} \\ $$

Commented by mr W last updated on 06/Jan/20

see Q77425 for an other way to prove.

$${see}\:{Q}\mathrm{77425}\:{for}\:{an}\:{other}\:{way}\:{to} \\ $$$${prove}. \\ $$

Commented by Tawa11 last updated on 29/Dec/21

Great sir

$$\mathrm{Great}\:\mathrm{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com