
Question Number 77252 by Maclaurin Stickker last updated on 04/Jan/20

Commented by Maclaurin Stickker last updated on 04/Jan/20
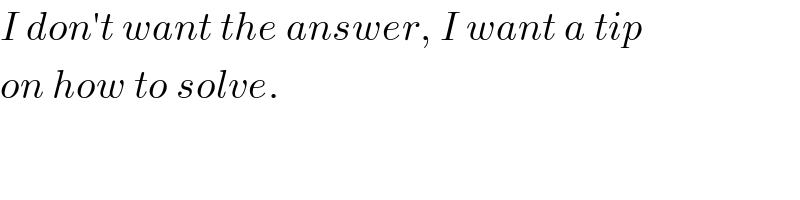
$${I}\:{don}'{t}\:{want}\:{the}\:{answer},\:{I}\:{want}\:{a}\:{tip} \\ $$$${on}\:{how}\:{to}\:{solve}.\: \\ $$
Commented by Maclaurin Stickker last updated on 04/Jan/20

$${I}\:{tried}\:{to}\:{find}\:{the}\:{radius}\:{of}\:{the} \\ $$$${largest}\:{circumference}\:{as}\:{a}\:{function} \\ $$$${of}\:{the}\:{radius}\:{of}\:{the}\:{yellow} \\ $$$${circumference}\:{but}\:{the}\:{results}\:{weren}'{t} \\ $$$${good}... \\ $$
Answered by mr W last updated on 05/Jan/20

Commented by Maclaurin Stickker last updated on 05/Jan/20
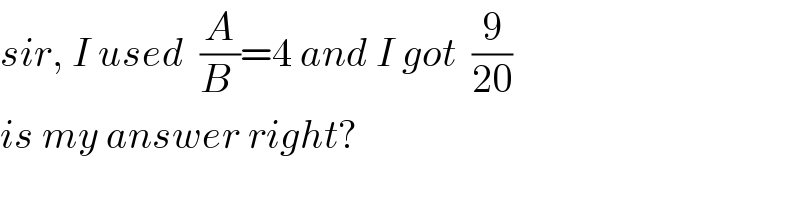
$${sir},\:{I}\:{used}\:\:\frac{{A}}{{B}\:}=\mathrm{4}\:{and}\:{I}\:{got}\:\:\frac{\mathrm{9}}{\mathrm{20}}\:\: \\ $$$${is}\:{my}\:{answer}\:{right}? \\ $$$$ \\ $$
Commented by mr W last updated on 05/Jan/20

$${O}\:{as}\:{origin}\:{and}\:{OF}\:{as}\:{x}−{axis} \\ $$$${x}_{{A}} =−{R}+{r}_{{a}} \\ $$$${x}_{{C}} =\mathrm{2}{r}_{{a}} −{R} \\ $$$${x}_{{B}} ^{\mathrm{2}} +{y}_{{B}} ^{\mathrm{2}} =\left({R}−{r}_{{b}} \right)^{\mathrm{2}} \\ $$$$\left({x}_{{B}} +{R}−{r}_{{a}} \right)^{\mathrm{2}} +{y}_{{B}} ^{\mathrm{2}} =\left({r}_{{a}} +{r}_{{b}} \right)^{\mathrm{2}} \\ $$$$\left({x}_{{B}} +{R}−{r}_{{a}} \right)^{\mathrm{2}} −{x}_{{B}} ^{\mathrm{2}} =\left({r}_{{a}} +{r}_{{b}} \right)^{\mathrm{2}} −\left({R}−{r}_{{b}} \right)^{\mathrm{2}} \\ $$$$\Rightarrow\left(\mathrm{2}{x}_{{B}} +{R}−{r}_{{a}} \right)\left({R}−{r}_{{a}} \right)=\left({R}+{r}_{{a}} \right)\left({r}_{{a}} +\mathrm{2}{r}_{{b}} −{R}\right) \\ $$$$\Rightarrow{x}_{{B}} =\frac{\left({R}+{r}_{{a}} \right)\left(\mathrm{2}{r}_{{b}} −{R}+{r}_{{a}} \right)−\left({R}−{r}_{{a}} \right)^{\mathrm{2}} }{\mathrm{2}\left({R}−{r}_{{a}} \right)} \\ $$$$\Rightarrow{y}_{{B}} =\sqrt{\left({R}−{r}_{{b}} \right)^{\mathrm{2}} −{x}_{{B}} ^{\mathrm{2}} } \\ $$$$ \\ $$$${CF}=\mathrm{2}\left({R}−{r}_{{a}} \right) \\ $$$$\frac{{EF}}{{GF}}=\frac{\mathrm{2}{R}}{{EF}}\:\Rightarrow\:{EF}^{\mathrm{2}} =\mathrm{2}{R}\left({R}−{r}_{{a}} \right) \\ $$$${EG}^{\mathrm{2}} ={EF}^{\mathrm{2}} −{GF}^{\mathrm{2}} =\mathrm{2}{R}\left({R}−{r}_{{a}} \right)−\left({R}−{r}_{{a}} \right)^{\mathrm{2}} \\ $$$${EG}^{\mathrm{2}} =\left({R}−{r}_{{a}} \right)\left({R}+{r}_{{a}} \right)={R}^{\mathrm{2}} −{r}_{{a}} ^{\mathrm{2}} \\ $$$$\Rightarrow{EG}=\sqrt{{R}^{\mathrm{2}} −{r}_{{a}} ^{\mathrm{2}} } \\ $$$${m}=\mathrm{tan}\:\angle{ECG}=\frac{{EG}}{{CG}}=\frac{\sqrt{{R}^{\mathrm{2}} −{r}_{{a}} ^{\mathrm{2}} }}{{R}−{r}_{{a}} }=\sqrt{\frac{{R}+{r}_{{a}} }{{R}−{r}_{{a}} }} \\ $$$${x}_{{C}} =\mathrm{2}{r}_{{a}} −{R} \\ $$$${eqn}.\:{of}\:{CE}: \\ $$$${y}={m}\left({x}−{x}_{{C}} \right) \\ $$$$\Rightarrow{mx}−{y}−{m}\left(\mathrm{2}{r}_{{a}} −{R}\right)=\mathrm{0} \\ $$$${r}_{{b}} =\frac{{m}\left({x}_{{B}} +{R}−\mathrm{2}{r}_{{a}} \right)−{y}_{{B}} }{\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }} \\ $$$${r}_{{b}} =\frac{\sqrt{\frac{{R}−{r}_{{a}} }{{R}+{r}_{{a}} }}\left({x}_{{B}} +{R}−\mathrm{2}{r}_{{a}} \right)−{y}_{{B}} }{\sqrt{\frac{\mathrm{2}{R}}{{R}+{r}_{{a}} }}} \\ $$$$\Rightarrow{r}_{{b}} =\sqrt{\frac{{R}−{r}_{{a}} }{\mathrm{2}{R}}}\left({x}_{{B}} +{R}−\mathrm{2}{r}_{{a}} \right)−\sqrt{\frac{{R}+{r}_{{a}} }{\mathrm{2}{R}}}{y}_{{B}} \\ $$$${let}\:\alpha=\frac{{r}_{{a}} }{{R}},\:\beta=\frac{{r}_{{b}} }{{R}} \\ $$$$\frac{{x}_{{B}} }{{R}}=\mu=\frac{\left(\mathrm{1}+\alpha\right)\left(\mathrm{2}\beta−\mathrm{1}+\alpha\right)−\left(\mathrm{1}−\alpha\right)^{\mathrm{2}} }{\mathrm{2}\left(\mathrm{1}−\alpha\right)} \\ $$$$\frac{{y}_{{B}} }{{R}}=\nu=\sqrt{\left(\mathrm{1}−\beta\right)^{\mathrm{2}} −\mu^{\mathrm{2}} } \\ $$$$\Rightarrow\beta=\sqrt{\frac{\mathrm{1}−\alpha}{\mathrm{2}}}\left(\mu+\mathrm{1}−\mathrm{2}\alpha\right)−\sqrt{\frac{\mathrm{1}+\alpha}{\mathrm{2}}}\nu \\ $$$$...... \\ $$
Commented by mr W last updated on 05/Jan/20
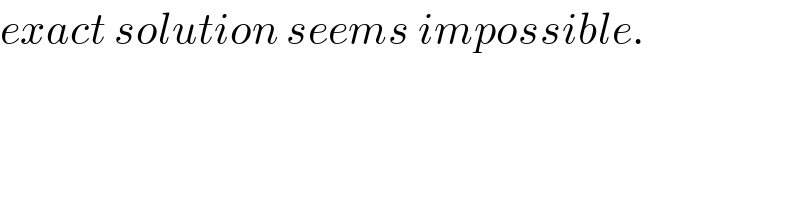
$${exact}\:{solution}\:{seems}\:{impossible}. \\ $$
Commented by Maclaurin Stickker last updated on 05/Jan/20
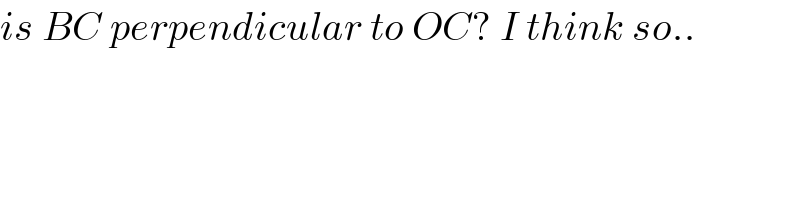
$${is}\:{BC}\:{perpendicular}\:{to}\:{OC}?\:{I}\:{think}\:{so}.. \\ $$
Commented by Maclaurin Stickker last updated on 05/Jan/20
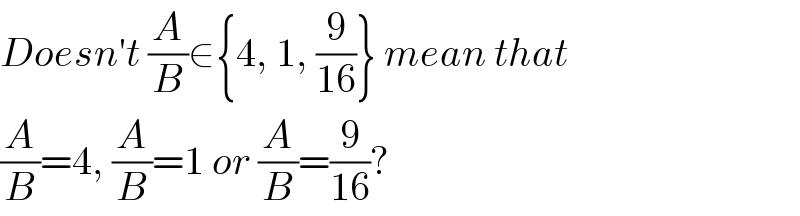
$${Doesn}'{t}\:\frac{{A}}{{B}}\in\left\{\mathrm{4},\:\mathrm{1},\:\frac{\mathrm{9}}{\mathrm{16}}\right\}\:{mean}\:{that} \\ $$$$\frac{{A}}{{B}}=\mathrm{4},\:\frac{{A}}{{B}}=\mathrm{1}\:{or}\:\frac{{A}}{{B}}=\frac{\mathrm{9}}{\mathrm{16}}? \\ $$
Commented by mr W last updated on 05/Jan/20

$${BC}\:{is}\:{not}\:{perpendicular}\:{to}\:{OC}! \\ $$$${you}\:{can}\:{see}\:{this}\:{clearly}\:{if}\:{r}_{{b}} ={r}_{{a}.} \\ $$
Commented by Maclaurin Stickker last updated on 05/Jan/20

$${If}\:{r}_{{a}} \neq{r}_{{b}} \:{BC}\:{could}\:{be}\:{perpendicular} \\ $$$${to}\:{OC}? \\ $$
Commented by mr W last updated on 06/Jan/20

$${not}\:{generally}! \\ $$
Commented by Tawa11 last updated on 29/Dec/21

$$\mathrm{Great}\:\mathrm{sir} \\ $$
